Intermittency in the Hodgkin-Huxley model

Abstract

We show that action potentials in the Hodgkin-Huxley neuron model result from a type I intermittency phenomenon that occurs in the proximity of a saddle-node bifurcation of limit cycles. For the Hodgkin-Huxley spatially extended model, describing propagation of action potential along axons, we show the existence of type I intermittency and a new type of chaotic intermittency, as well as space propagating regular and chaotic diffusion waves. Chaotic intermittency occurs in the transition from a turbulent regime to the resting regime of the transmembrane potential and is characterised by the existence of a sequence of action potential spikes occurring at irregular time intervals.

Appendix: The Bautin bifurcation normal form and the Hodgkin-Huxley equation

The normal form of the Bautin bifurcation in polar coordinates is Kuznetsov (2004)

$$ \left\{ \begin{array}{lcl}\displaystyle \dot r&=&\displaystyle r (\beta_{1}+\beta_{2} r^{2}-r^{4})=f(r)\\ \displaystyle \dot \phi&=&1, \end{array}\right. $$

(6)

where β ₁ and β ₂ are real parameters.

One of the features of the normal form Eq. (6) of the Bautin bifurcation is the existence of a SNLC bifurcation, a subcritical and a supercritical Hopf bifurcations, when the parameters β ₁ and β ₂ are varied. As it is shown numerically in Fig. 2, these features are present in the Hodgkin-Huxley model Eq. (1), when the parameter i is varied.

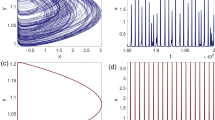
To show that the bifurcation behaviour of the diffusion free Hodgkin-Huxley Eqs. (1)–(2), is well described by the bifurcation behaviour of Eq. (6), we take the new parameters $\rho =\sqrt {{\beta _{1}^{2}}+{\beta _{2}^{2}}}$ and θ = arctan (−β ₂/β ₁). The bifurcation diagram of fixed points and limit cycles as a function of θ and for ρ = 1 is presented in Fig. 11.

Fig. 11

Bifurcation diagram of Eq. (6) as a function of θ = arctan(−β ₂/β ₁), for ρ = 1. The maximum values of the radial coordinate r for the stable and unstable limit cycles are represented, along with its unique fixed point r ^∗ = 0. Dashed lines indicate unstable limit sets. For other values of ρ, the bifurcation diagram is similar

We note that the normal form of the Bautin bifurcation is unfolded as a two-dimensional dynamical system, whereas the diffusion free Hodgkin-Huxley equations define a four-dimensional vector field. This is due to the fact that the fixed point of Eq. (1) has a two-dimensional stable manifold, and the limit sets or attractors of the diffusion free Hodgkin-Huxley equations live in a two-dimensional space embedded in the four-dimensional phase space. All the analyses we have done show that the intermittency phenomena found in Eq. (1) are compatible with the intermittency phenomena and the bifurcation behaviour of the normal form of the Bautin bifurcation, near a SNLC bifurcation.

1.1 Intermittency near a SNLC bifurcation

A simple analysis shows that, if β ₂ > 0 and β ₁ < 0, there is a saddle node bifurcation in the radial variable r, which corresponds to a SNLC bifurcation in the cartesian coordinates. The codimension 2 SLNC bifurcation occurs for $\beta _{1}=\beta _{1}^{*}=-{\beta _{2}^{2}}/4$ and a limit cycle is created with radial coordinate $r^{*}=\sqrt {\beta _{2}/2}$. We take now the new parameter ε defined by

$$ \beta_{1}=-\frac{1}{4}{\beta_{2}^{2}}-\varepsilon. $$

(7)

For β ₂ > 0, the system of Eq. (6) has a SNLC bifurcation for ε = 0. If ε > 0, the system of Eq. (6) has no limit cycles and, if ε < 0, the system of Eq. (6) has two limit cycles with radial coordinates $r_{\text {s}}=\sqrt {(\beta _{2}+2\sqrt {-\varepsilon })/2}$ and $r_{\text {u}}=\sqrt {(\beta _{2}-2\sqrt {-\varepsilon })/2}$, where the subscripts “s” and “u” stand for stable and unstable, respectively.

Along any line β ₂ = constant with β ₂ > 0, for ε < 0, the asymptotic solutions of Eq. (6) with initial condition r ₀ > r _s converge to a stable limit cycle. For ε > 0 but close to zero, the graph of y = f(r) has a local maximum near $r_{max}=\sqrt {\beta _{2}/2}$ which is very close to the line y = 0. For initial conditions r ₀ > r _s and by the continuity of the solutions of the differential equation in order to ε, the radial solution of Eq. (6) will be near r = r _{m a x} for a long interval of time. This interval of time goes to infinity, as ε → 0. So, during this period of time, the solution of the differential equation will resemble the solution along a limit cycle that does not exist. This is the phenomenon of type I intermittency, Pomeau and Manneville (1980) and Guckenheimer and Holmes (2002).

As a function of ε, we estimate the time of permanence of the solution of Eq. (6) near the point $r_{max}=\sqrt {\beta _{2}/2}$, the radius of the limit cycle at bifurcation. As this analysis is local, we take u = r − r _{m a x} and, by Eq. (7), the first equation in Eq. (6) is rewritten as

$$ \dot u=-\varepsilon (r_{max}+u)-\sqrt{2}\beta_{2}^{3/2} u^{2}+{\mathcal O}(u^{3})=g(u)+{\mathcal O}(u^{3}). $$

(8)

In this new coordinate, the SNLC bifurcation occurs for ε = 0 and the limit cycle has u-radial coordinate u = r − r _{m a x} = 0.

For an initial condition u(0) = d > 0, the time of permanence of the solutions of Eq. (8) in the interval [−d, d] is

$$ t={\int}_{-d}^{d} \frac{du}{g(u)}=\left. \frac{2}{\sqrt{\varepsilon}}\frac{1}{\sqrt{4 {\beta_{2}^{2}}-\varepsilon}}\arctan{\frac{\varepsilon+2\sqrt{2}u\beta_{2}^{3/2}}{\sqrt{\varepsilon}\sqrt{4 {\beta_{2}^{2}}-\varepsilon}}}\right|_{-d}^{d}. $$

(9)

In the limit ε → 0, arctan(.) → π/2 and the previous expression becomes

$$ t=\frac{\pi}{\beta_{2}\sqrt{\varepsilon}}. $$

(10)

Thus, we have proved that in the left vicinity of a codimension 2 SNLC bifurcation, the orbits follow a trajectory resembling a limit cycle oscillation during a time that goes to infinity as the bifurcation is approached.

1.2 Stability of the steady state of the HH equations

Here, we analyse the stability of the homogeneous stable state p ^∗(0) = (V ^∗(0), n ^∗(0), m ^∗(0), h ^∗(0)) of the second and third equations in Eq. (4), with the boundary conditions Eq. (5).

Due to the zero flux boundary conditions Eq. (5), the solutions of the second and third equations in Eq. (4) can be written in the form

$$\begin{array}{@{}rcl@{}} V(x,t)&=& V^{*}(0)+{\sum}_{k\ge 1} a_{k}(t) \cos{2\pi k x/L}\\ n(x,t)&=& n^{*}(0)+{\sum}_{k\ge 1} b_{k}(t) \cos{2\pi k x/L}\\ m(x,t)&=& m^{*}(0)+{\sum}_{k\ge 1} c_{k}(t) \cos{2\pi k x/L}\\ h(x,t)&=& m^{*}(0)+{\sum}_{k\ge 1} d_{k}(t) \cos{2\pi k x/L}, \end{array} $$

(11)

where a _k (t), b _k (t), c _k (t) and d _k (t) are time dependent unknown functions of time, and V (x, t), n(x, t), m(x, t), h(x, t) ∈ L ²([0, L]), for every t ≥ 0. As usual, a _k (t), b _k (t), c _k (t) and d _k (t) are the k-eigenmode solutions of the extended HH equations. To analyse the stability of p ^∗(0), following (Dilão 2005), we introduce (11) into the first equation in (4) and, up to first order in the phase space variables we obtain

$$ \left( \begin{array}{llll} {\dot a}_{k}\\ {\dot b}_{k}\\ {\dot c}_{k}\\ {\dot d}_{k} \end{array}\right) = \left( \begin{array}{cccc} \frac{\partial F}{\partial V}-D\frac{4\pi^{2} k^{2}}{L^{2}}& \frac{\partial F}{\partial n}& \frac{\partial F}{\partial m}& \frac{\partial F}{\partial h}\\[1em] \frac{\partial G_{n}}{\partial V}& \frac{\partial G_{n}}{\partial n}& 0& 0\\[1em] \frac{\partial G_{m}}{\partial V}& 0& \frac{\partial G_{m}}{\partial m}& 0\\[1em] \frac{\partial G_{h}}{\partial V} &0 &0 & \frac{\partial G_{h}}{\partial h} \end{array}\right) \left( \begin{array}{llll} {a}_{k}\\ {b}_{k}\\ {c}_{k}\\ {d}_{k} \end{array}\right), $$

(12)

for every k ≥ 1 and the matrix is evaluated at p ^∗(0). If, for every k > 1, the real parts of the eigenvalues of the jacobian matrix in Eq. (12) are negative, the homogeneous spatial solution (V (x, t), n(x, t)) = p ^∗(0) of the first and third equations in Eq. (4) is stable. Due to the complex form of the vector field components, the jacobian matrix is difficult to calculate. So, we have analysed numerically the real part of its eigenvalues, for a large range of the index k. For values of the eigenmode number k, up to k = 2 000, and for D ∈ [10⁻⁶, 10], all the eigenvalues of the matrix in Eq. (12) have negative real parts, implying that the homogeneous steady state of the extended HH equation is stable.