Nonclassical Properties of a Hybrid NAAN Quantum State

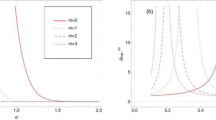
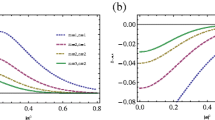
Abstract

We introduce a two-mode hybrid entangled state (NAAN) which is constructed by two n-photon Fock states and two coherent states with an arbitrary relative phase. We show that the NAAN can be considered as the superpositions of NOON states when $\alpha \ne 0$. In the special case, when $\alpha = 0$, the NAAN degenerates to the general NOON. The most interesting nonclassical properties of this state are its strong violations of the CHSH inequality. In addition, we show explicitly some typical nonclassical properties of the NAAN state, such as entanglement, sub-Poissonian distribution, phase fluctuation and squeezing. These findings suggest that the even NAAN states exhibit a high degree of entanglement, while the odd NAAN states have a distinct sub-Poissonian distribution and the optimal phase sensitivity.

Appendices

Appendix 1. Derivation of expectation values in Eq. (16)

Using Eq. (1), the average value of the $a_{1}^{\dag } a_{1}$ is

$$\begin{gathered} \left\langle {a_{1}^{\dag } a_{1} } \right\rangle = N_{c}^{ - 1} \left( {\left\langle {N_{2} } \right|\left\langle {\alpha_{1} } \right|e^{ - i\varphi } + \left\langle {\alpha_{2} } \right|\left\langle {N_{1} } \right|} \right)a_{1}^{\dag } a_{1} \left( {\left| {N_{1} } \right\rangle \left| {\alpha_{2} } \right\rangle + e^{i\varphi } \left| {\alpha_{1} } \right\rangle \left| {N_{2} } \right\rangle } \right) \hfill \\ = N_{c}^{ - 1} (\left\langle {\alpha_{2} } \right|\left. {\alpha_{2} } \right\rangle \left\langle {N_{1} } \right|a_{1}^{\dag } a_{1} \left| {N_{1} } \right\rangle + \left\langle {N_{2} } \right|\left. {N_{2} } \right\rangle \left\langle {\alpha_{1} } \right|a_{1}^{\dag } a_{1} \left| {\alpha_{1} } \right\rangle \hfill \\ + e^{i\varphi } \left\langle {\alpha_{2} } \right|\left. {N_{2} } \right\rangle \left\langle {N_{1} } \right|a_{1}^{\dag } a_{1} \left| {\alpha_{1} } \right\rangle + e^{ - i\varphi } \left\langle {N_{2} } \right|\left. {\alpha_{2} } \right\rangle \left\langle {\alpha_{1} } \right|a_{1}^{\dag } a_{1} \left| {N_{1} } \right\rangle ). \hfill \\ \end{gathered}$$

(A1)

According $a\left| \alpha \right\rangle = \alpha \left| \alpha \right\rangle ,a^{\dag } a\left| n \right\rangle = n\left| n \right\rangle$ and $\left\langle \alpha \right.\left| n \right\rangle = \frac{{\alpha^{ * n} }}{{\sqrt {n!} }}e^{{ - \frac{1}{2}\left| \alpha \right|^{2} }}$, we have

$$\left\langle {a_{1}^{\dag } a_{1} } \right\rangle = N_{c}^{ - 1} \left( {\frac{2}{{\left( {n - 1} \right)!}}\left| \alpha \right|^{2} e^{{ - \left| \alpha \right|^{2} }} \cos \varphi + \left| \alpha \right|^{2} + n} \right).$$

(A2)

In a similar way, one readily obtain the average values of $a_{2}^{\dag } a_{2} ,a_{1} a_{2} ,a_{1}$ and $a_{2}$ as shown in Eq. (16).

Appendix 2. Derivation of Wigner function in Eq. (22)

We first calculate the inner product part of the Wigner function as

$$\begin{gathered} \left\langle { - z_{1} , - z_{2} } \right|\rho \left| {z_{1} ,z_{2} } \right\rangle \hfill \\ = N_{c}^{ - 1} \left\langle { - z_{1} , - z_{2} } \right|\left( {\left| {N_{1} } \right\rangle \left| {\alpha_{2} } \right\rangle + e^{{i^{\varphi } }} \left| {\alpha_{1} } \right\rangle \left| {N_{2} } \right\rangle } \right) \hfill \\ \left( {\left\langle {N_{2} } \right|\left\langle {\alpha_{1} } \right|e^{ - i\varphi } + \left\langle {\alpha_{2} } \right|\left\langle {N_{1} } \right|} \right)\left| {z_{1} ,z_{2} } \right\rangle \hfill \\ = N_{c}^{ - 1} \frac{1}{n!}\left( { - 1} \right)^{n} \left[ {z_{1}^{ * n} z_{1}^{n} e^{{ - \left| {z_{1} } \right|^{2} - \left| {z_{2} } \right|^{2} - \alpha z_{2}^{ * } + \alpha^{ * } z_{2} }} + z_{2}^{ * n} z_{2}^{n} e^{{ - \left| {z_{1} } \right|^{2} - \left| {z_{2} } \right|^{2} - z_{1}^{ * } \alpha + \alpha^{ * } z_{1} }} } \right. \hfill \\ \left. { + e^{{i^{\varphi } }} z_{1}^{n} z_{2}^{ * n} e^{{ - \left| {z_{1} } \right|^{2} - \left| {z_{2} } \right|^{2} - \alpha z_{1}^{ * } + \alpha z_{2}^{ * } }} + e^{ - i\varphi } \left( {z_{1}^{ * } } \right)^{n} z_{2}^{n} e^{{ - \left| {z_{1} } \right|^{2} - \left| {z_{2} } \right|^{2} + \alpha^{ * } z_{1} - \alpha z_{2}^{ * } }} } \right], \hfill \\ \end{gathered}$$

(B1)

where the overlap between two coherent states $\left\langle \alpha \right|\left. \beta \right\rangle = \exp \left[ { - \frac{1}{2}\left( {\left| \alpha \right|^{2} + \left| \beta \right|^{2} } \right) + \alpha^{ * } \beta } \right]$ has been used.

Using the definition of the Wigner function in Eq. (20), the first integral term is obtained as

$$\begin{gathered} F_{1}^{^{\prime}} = \frac{{\left( { - 1} \right)^{n} }}{n!}\frac{{2e^{{2\left( {\left| {\gamma_{1} } \right|^{2} + \left| {\gamma_{2} } \right|^{2} } \right)}} }}{{\pi^{2} }}N_{c}^{ - 1} \int \frac{{d^{2} z_{1} d^{2} z_{2} }}{{\pi^{2} }}z_{1}^{ * n} z_{1}^{n} e^{{ - \alpha z_{2}^{ * } + \alpha^{ * } z_{2} }} e^{{ - \left| {z_{1} } \right|^{2} - \left| {z_{2} } \right|^{2} }} \hfill \\ .\exp \left[ {2\left( {z_{1}^{ * } \gamma_{1} - z_{1} \gamma_{1}^{ * } } \right) + 2\left( {z_{2}^{ * } \gamma_{2} - z_{2} \gamma_{2}^{ * } } \right)} \right] \hfill \\ = \frac{{\left( { - 1} \right)^{n} }}{n!}\frac{{2e^{{2\left( {\left| {\gamma_{1} } \right|^{2} + \left| {\gamma_{2} } \right|^{2} } \right)}} }}{{\pi^{2} }}N_{c}^{ - 1} \int \frac{{d^{2} z_{1} }}{\pi }z_{1}^{ * n} z_{1}^{n} \exp \left( { - \left| {z_{1} } \right|^{2} + 2z_{1}^{ * } \gamma_{1} - 2z_{1} \gamma_{1}^{ * } } \right) \hfill \\ .\int \frac{{d^{2} z_{2} }}{\pi }\exp \left[ { - \left| {z_{2} } \right|^{2} + \left( {2\gamma_{2} - \alpha } \right)z_{2}^{ * } + \left( {\alpha^{ * } - 2\gamma_{2}^{ * } } \right)z_{2} } \right] \hfill \\ = \frac{2}{{\pi^{2} }}e^{{ - 2\left( {\left| {\gamma_{1} } \right|^{2} + \left| {\gamma_{2} } \right|^{2} } \right)}} \left( { - 1} \right)^{n} N_{c}^{ - 1} L_{m} \left( { - 4\left| {\gamma_{1} } \right|^{2} } \right)\exp \left( {2\alpha \gamma_{2}^{ * } + 2\gamma_{2} \alpha^{ * } - \left| \alpha \right|^{2} } \right), \hfill \\ \end{gathered}$$

(B2)

where we have used the integral formula of complex function

$$\int \frac{{d^{2} z}}{\pi }z^{m} z^{ * m} \exp \left( { - \left| z \right|^{2} + \xi z + \eta z^{ * } } \right) = m!e^{\xi \eta } L_{m} \left( {\xi \eta } \right),$$

(B3)

and

$$\int \frac{{d^{2} z}}{\pi }\exp \left( {\zeta \left| z \right|^{2} + \xi z + \eta z^{ * } } \right) = - \frac{1}{\zeta }\exp \left( { - \frac{\xi \eta }{\zeta }} \right),\;Re\left( \xi \right) < 0.$$

(B4)

Similarly, using Eqs. (22) and (B1) and the integral formula

$$\begin{gathered} \int \frac{{d^{2} z}}{\pi }z^{n} \exp \left( { - \left| z \right|^{2} + \xi z + \eta z^{ * } } \right) = \eta^{n} e^{\xi \eta } , \hfill \\ \int \frac{{d^{2} z}}{\pi }z^{ * n} \exp \left( { - \left| z \right|^{2} + \xi z + \eta z^{ * } } \right) = \xi^{n} e^{\xi \eta } , \hfill \\ \end{gathered}$$

(B5)

we have

$$\begin{gathered} F_{2}^{^{\prime}} = e^{ - i\varphi } \frac{{\left( { - 1} \right)^{n} }}{n!}\frac{{2e^{{2\left( {\left| {\gamma_{1} } \right|^{2} + \left| {\gamma_{2} } \right|^{2} } \right)}} }}{{\pi^{2} }}N_{c}^{ - 1} \hfill \\ \int \frac{{d^{2} z_{1} d^{2} z_{2} }}{{\pi^{2} }}z_{1}^{ * n} z_{2}^{n} e^{{ - \left| {z_{1} } \right|^{2} - \left| {z_{2} } \right|^{2} - \alpha z_{2}^{ * } + \alpha^{ * } z_{1} }} \hfill \\ \exp \left[ {2\left( {z_{1}^{ * } \gamma_{1} - z_{1} \gamma_{1}^{ * } } \right) + 2\left( {z_{2}^{ * } \gamma_{2} - z_{2} \gamma_{2}^{ * } } \right)} \right] \hfill \\ = e^{ - i\varphi } \frac{{\left( { - 1} \right)^{n} }}{n!}\frac{{2e^{{ - 2\left( {\left| {\gamma_{1} } \right|^{2} + \left| {\gamma_{2} } \right|^{2} } \right)}} }}{{\pi^{2} }}N_{c}^{ - 1} \hfill \\ \left( {2\gamma_{2} - \alpha } \right)^{n} \left( {\alpha^{ * } - 2\gamma_{1}^{ * } } \right)^{n} \exp \left( {2\gamma_{1} \alpha + 2\alpha \gamma_{2}^{ * } } \right) \hfill \\ \end{gathered}$$

(B6)

and

$$\begin{gathered} F_{3}^{^{\prime}} = \frac{{\left( { - 1} \right)^{n} }}{n!}\frac{{2e^{{2\left( {\left| {\gamma_{1} } \right|^{2} + \left| {\gamma_{2} } \right|^{2} } \right)}} }}{{\pi^{2} }}N_{c}^{ - 1} \int \frac{{d^{2} z_{1} d^{2} z_{2} }}{{\pi^{2} }}z_{2}^{n} z_{2}^{ * n} e^{{ - \alpha z_{1}^{ * } }} e^{{\alpha^{ * } z_{1} }} \hfill \\ \exp \left[ {2\left( {z_{1}^{ * } \gamma_{1} - z_{1} \gamma_{1}^{ * } } \right) + 2\left( {z_{2}^{ * } \gamma_{2} - z_{2} \gamma_{2}^{ * } } \right)} \right] \hfill \\ = \frac{{\left( { - 1} \right)^{n} }}{n!}\frac{2}{{\pi^{2} }}e^{{ - 2\left( {\left| {\gamma_{1} } \right|^{2} + \left| {\gamma_{2} } \right|^{2} } \right)}} N_{c}^{ - 1} \hfill \\ n!L_{n} \left( {4\left| {\gamma_{2} } \right|} \right)^{2} \exp \left( {2\alpha \gamma_{1}^{ * } + 2\gamma_{1} \alpha^{ * } - \left| \alpha \right|^{2} } \right) \hfill \\ \end{gathered}$$

(B7)

Substituting Eqs.(B2),(B6) and (B7) into (20) and after some simplifications, Eq. (22) is obtained.