Skip to main content
SpringerLink
Log in

Atom-Atom Entanglement in a Hybrid Fiber-Atom-Optomechanical System

  • Published:
International Journal of Theoretical Physics Aims and scope Submit manuscript

Abstract

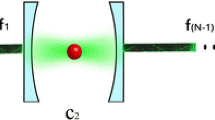
In this paper, we consider a hybrid fiber-atom-optomechanical system consisting of two optomechanical cavities where they are connected together with an optical fiber. Moreover, each cavity interacts separately with a two-level atom. Obtaining the effective Hamiltonian of the whole system, we find the state vector of the system numerically. Afterward, we study the degree of entanglement (DEM) between two atoms with the benefit of concurrence. We show that the DEM between the atoms can be appropriately controlled by adopting cavity-fiber and optomechanical couplings. Furthermore, we observe from the numerical results that the so-called phenomena of entanglement sudden death and birth (ESD and ESB) happen in the considered system.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

References

  1. Gröblacher, S., Hammerer, K., Vanner, M., Aspelmeyer, M.: Observation of strong coupling between a micromechanical resonator and an optical cavity field. Nature 460, 724 (2009)

    Article  ADS  Google Scholar 

  2. Huang, S., Agarwal, G.S.: Normal-mode splitting in a coupled system of a nanomechanical oscillator and a parametric amplifier cavity. Phys. Rev. A 80, 033807 (2009)

    Article  ADS  Google Scholar 

  3. Asghari Nejad, A., Ranjbar Askari, H., Baghshahi, H.R.: Normal mode splitting in an optomechanical system: effects of Coulomb and parametric interactions . J. Opt. Soc. Am. B 35, 2237 (2018)

    Article  ADS  Google Scholar 

  4. He, B., Yang, L., Lin, Q., Xiao, M.: Radiation pressure cooling as a quantum dynamical process. Phys. Rev. Lett. 118, 233604 (2017)

    Article  ADS  Google Scholar 

  5. Asghari Nejad, A., Ranjbar Askari, H., Baghshahi, H.R.: Cooling of a nanomechanical resonator in a hybrid optomechanical system: effect of parametric interactions. Physica E: Low-dimensional Systems and Nanostructures 102, 83 (2018)

    Article  ADS  Google Scholar 

  6. Asghari Nejad, A., Baghshahi, H.R., Ranjbar Askari, H.: Effect of second-order coupling on optical bistability in a hybrid optomechanical system . Eur. Phys. J. D 71, 267 (2017)

    Article  ADS  Google Scholar 

  7. Zhu, X.-F., Wang, L.-D., Yan, J.-K., Chen, B.: Controllable optical bistability in an optomechanical system assisted by microwave. Optik 154, 139 (2018)

    Article  ADS  Google Scholar 

  8. Asghari Nejad, A., Baghshahi, H.R., Ranjbar Askari, H.: Bistability in a hybrid optomechanical system: effect of a gain medium. Laser Phys. 27, 115202 (2017)

    Article  ADS  Google Scholar 

  9. Asghari Nejad, A., Ranjbar Askari, H., Baghshahi, H.R.: Optical bistability in coupled optomechanical cavities in the presence of Kerr effect. Appl. Opt. 56, 2816 (2017)

    Article  ADS  Google Scholar 

  10. Asghari Nejad, A., Ranjbar Askari, H., Baghshahi, H.R.: Optical tristability in a hybrid optomechanical system. Opt. Laser Technol. 101, 279 (2018)

    Article  ADS  Google Scholar 

  11. Kronwald, A., Marquardt, F.: Optomechanically induced transparency in the nonlinear quantum regime. Phys. Rev. Lett. 111, 133601 (2013)

    Article  ADS  Google Scholar 

  12. Asghari Nejad, A., Ranjbar Askari, H., Baghshahi, H.R.: Optomechanical electromagnetically induced transparency in inverted atomic configurations: a comparative view. Laser Phys. 27, 035202 (2017)

    Article  ADS  Google Scholar 

  13. Parvaz, M., Askari, H.R., Baghshahi, H.R.: Double electromagnetically induced transparency windows in the Λ-type three-level atoms-assisted optomechanical system. Optik 220, 164974 (2020)

    Article  ADS  Google Scholar 

  14. Jennewein, T., Simon, C., Weihs, G., Weinfurter, H., Zeilinger, A.: Quantum cryptography with entangled photons. Phys. Rev. Lett. 84, 4729 (2000)

    Article  ADS  Google Scholar 

  15. Cirac, J.I., Zoller, P.: Quantum computations with cold trapped ions. Phys. Rev. Lett. 74, 4091 (1995)

    Article  ADS  Google Scholar 

  16. Ou, Z.Y.: Enhancement of the phase-measurement sensitivity beyond the standard quantum limit by a nonlinear interferometer. Phys. Rev. A 85, 023815 (2012)

    Article  ADS  Google Scholar 

  17. Li, X., Pan, Q., Jing, J., Zhang, J., Xie, C., Peng, K.: Quantum dense coding exploiting a bright Einstein-Podolsky-Rosen beam. Phys. Rev. Lett. 88, 047904 (2002)

    Article  ADS  Google Scholar 

  18. Abdi, M., Pirandola, S., Tombesi, P., Vitali, D.: Entanglement swapping with local certification: application to remote micromechanical resonators. Phys. Rev. Lett. 109, 143601 (2012)

    Article  ADS  Google Scholar 

  19. Yönaç, M., Yu, T., Eberly, J.H.: Sudden death of entanglement of two Jaynes-Cummings atoms. J. Phys. B: At. Mol. Opt. Phys. 39, S621 (2006)

    Article  ADS  Google Scholar 

  20. Abdel-Khalek, S., Halawani, S.H.A.: New features of the stationary and moving atom-atom entanglement. Optik 127, 9020 (2016)

    Article  ADS  Google Scholar 

  21. Qiang, W.-C., Sun, G.-H., Dong, Q., Camacho-Nieto, O., Dong, S.-H.: Concurrence of three Jaynes-Cummings systems. quantum Inf. Process. 17, 90 (2018)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  22. Bashkirov, E.K., Mastyugin, M.S.: Influence of Stark shift on entanglement of two atoms with degenerate two-photon transitions for entangled and disentangled initial states. Optik 126, 1787 (2015)

    Article  ADS  Google Scholar 

  23. Baghshahi, H.R., Tavassoly, M.K.: Entanglement, quantum statistics and squeezing of two Ξ-type three-level atoms interacting nonlinearly with a single-mode field. Phys. Scr. 89, 075101 (2014)

    Article  ADS  Google Scholar 

  24. Mohamed, A.B.A., Hessian, H.A., Eleuch, H.: Dynamics of a dissipative two-qubit system interacting non-linearly with a generalized field: entanglement and mixedness. Optik 202, 163500 (2020)

    Article  ADS  Google Scholar 

  25. Faraji, E., Baghshahi, H.R., Tavassoly, M.K.: The influence of atomic dipole-dipole interaction on the dynamics of the population inversion and entanglement of two atoms interacting non-resonantly with two coupled modes field. Mod. Phys. Lett. B 31, 1750038 (2017)

    Article  ADS  Google Scholar 

  26. Zhang, B.: Entanglement between two atoms in two distant cavities connected by an optical fiber beyond strong fiber-cavity coupling. Opt. Commun. 283, 196 (2010)

    Article  ADS  Google Scholar 

  27. Genes, C., Vitali, D., Tombesi, P.: Emergence of atom-light-mirror entanglement inside an optical cavity. Phys. Rev. A 77, 050307 (2008)

    Article  ADS  Google Scholar 

  28. De Chiara, G., Paternostro, M., Palma, G.M.: Entanglement detection in hybrid optomechanical systems . Phys. Rev. A 83, 052324 (2011)

    Article  ADS  Google Scholar 

  29. Li, J., Li, G., Zippilli, S., Vitali, D., Zhang, T.: Enhanced entanglement of two different mechanical resonators via coherent feedback. Phys. Rev. A 95, 043819 (2017)

    Article  ADS  Google Scholar 

  30. Asghari Nejad, A., Ranjbar Askari, H., Baghshahi, H.R.: Optomechanical detection of weak microwave signals with the assistance of a plasmonic wave. Phys. Rev. A 97, 053839 (2018)

    Article  ADS  Google Scholar 

  31. Joshi, C., Larson, J., Jonson, M., Andersson, E., Öhberg, P.: Entanglement of distant optomechanical systems. Phys. Rev. A 85, 033805 (2012)

    Article  ADS  Google Scholar 

  32. Liao, Q., Nie, W., Xu, J., Liu, Y., Zhou, N., Yan, Q., Chen, A., Liu, N., Ahmad, M.: Properties of linear entropy of the atom in a tripartite cavity-optomechanical system. Laser Phys. 26, 055201 (2016)

    Article  ADS  Google Scholar 

  33. Nadiki, M.H., Tavassoly, M.K.: Collapse-revival in entanglement and photon statistics: the interaction of a three-level atom with a two-mode quantized field in cavity optomechanics. Laser Phys. 26, 125204 (2016)

    Article  ADS  Google Scholar 

  34. Momenabadi, F.M., Baghshahi, H.R., Faghihi, M.J., Mirafzali, S.Y.: Stable entanglement in a quadripartite cavity optomechanics. Eur. Phys. J. Plus 136, 7 (2021)

    Article  Google Scholar 

  35. Liao, Q., Yuan, L., Fu, Y., Zhou, N.: Properties of entanglement between the JC model and atom-cavity-optomechanical system. Int. J. Theor. Phys. 58, 2641 (2019)

    Article  MATH  Google Scholar 

  36. Hu, X., Hou, B.P., Tang, B.: Enhanced mechanical entanglement in an optomechanical cavity with a Coulomb interaction. Optik 159, 368 (2019)

    Article  ADS  Google Scholar 

  37. Horodecki, R., Horodecki, P., Horodecki, M., Horodecki, K.: Quantum entanglement. Rev. Mod. Phys. 81, 865 (2009)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  38. Wootters, W.K.: Entanglement of formation of an arbitrary state of two qubits. Phys. Rev. Lett. 80, 2245 (1998)

    Article  ADS  MATH  Google Scholar 

  39. Coffman, V., Kundu, J., Wootters, W.K.: Distributed entanglement . Phys. Rev. A 61, 052306 (2000)

    Article  ADS  Google Scholar 

  40. Ikram, M., Li, F., Zubairy, M.: Disentanglement in a two-qubit system subjected to dissipation environments. Phys. Rev. A 75, 062336 (2007)

    Article  ADS  Google Scholar 

  41. Gamel, O., James, D.F.V.: Time-averaged quantum dynamics and the validity of the effective Hamiltonian model. Phys. Rev. A 82, 052106 (2010)

    Article  ADS  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Physics, Faculty of Science, Vali-e-Asr University of Rafsanjan, Rafsanjan, Iran

    Mohammad Ali Fathi, Hamid Reza Baghshahi, Mohammad Khanzadeh & Sayyed Yahya Mirafzali

Authors
  1. Mohammad Ali Fathi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Hamid Reza Baghshahi
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Mohammad Khanzadeh
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Sayyed Yahya Mirafzali
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Hamid Reza Baghshahi.

Additional information

Publisher’s Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix

Appendix

Here, we want to demonstrate how the effective Hamiltonian of the whole system, which is introduced in (7), can be obtained. To this end, first suppose that an interaction Hamiltonian could be written as follows

$$ \begin{array}{@{}rcl@{}} \hat{H}_{\mathrm{I}}(t) = \sum\limits_{{ n}=0}^{{ N}} \hat{h}_{n} { e}^{-i \omega^{\prime}_{n} t} +\hat{h}^{\dagger}_{n} \mathrm{e}^{i \omega^{\prime}_{n} t}, \end{array} $$
(15)

where N is the total number of different harmonic terms,which make up the interaction Hamiltonian, with oscillating frequency ωn > 0. So the effective Hamiltonian reduces to:

$$ \begin{array}{@{}rcl@{}} \hat{H}_{\text{eff}}(t) = \sum\limits_{{n,m}=0}^{N} \frac{1}{\hbar \bar{\omega}^{\prime}_{mn}} [\hat{h}^{\dagger}_{m},\hat{h}_{n}] {e}^{i(\omega^{\prime}_{m}-\omega^{\prime}_{n})t} \end{array} $$
(16)

where \(\bar {\omega }^{\prime }_{mn}\) is the harmonic average of \({\omega }^{\prime }_{m}\) and \({\omega }^{\prime }_{n}\), defines as \(\frac {1}{\bar {\omega }^{\prime }_{mn}} = \frac {1}{2}(\frac {1}{{\omega }^{\prime }_{m}}+\frac {1}{{\omega }^{\prime }_{n}})\) [41].

Now, we use the above equations and calculate the effective Hamiltonian for our considered model. In our system, the interaction Hamiltonian reads as (\(\hbar =1\)):

$$ \begin{array}{@{}rcl@{}} \hat{H}_{\mathrm{I}}&=& \sum\limits_{{j}=1}^{2} \lambda_{j} (\hat{a}_{j} \hat{\sigma}_{j+} {e}^{-i ({\varOmega}_{j} - \omega_{j}) t} + \hat{a}^{\dagger}_{j} \hat{\sigma}_{j-} {e}^{i ({\varOmega}_{j} - \omega_{j} ) t} ) \\ &+& \sum\limits_{{j}=1}^{2} {J}(\hat{a}_{j}\hat{b}^{\dagger} {e}^{-i ({\varOmega}_{j} - {\varOmega}_{f}) t}+\hat{a}^{\dagger}_{j}\hat{b} {e}^{i ({\varOmega}_{j} - {\varOmega}_{f}) t}) \\ &-& \sum\limits_{{j}=1}^{2} {G}_{j}\hat{a}^{\dagger}_{j} \hat{a}_{j} (\hat{c}_{j} { e}^{-i\omega_{jm} t}+\hat{c}^{\dagger}_{j} {e}^{i \omega_{jm} t}). \end{array} $$
(17)

Comparing (15) with that of (17), one would be able to find the operators hi(i = 1, 2,...6) and frequencies \({\omega }^{\prime }_{i} (i=1,2,...6)\), associated with the Hamiltonian (17), as the following form:

$$ \begin{array}{@{}rcl@{}} \hat{h}_{1} &=& \lambda_{1} \hat{a}_{1} \hat{\sigma}_{1+}, \quad \quad \quad \quad \hat{h}_{2} = \lambda_{2} \hat{a}_{2} \hat{\sigma}_{2+},\\ \hat{h}_{3} &=& J \hat{a}_{1}\hat{b}^{\dagger}, \quad \quad \quad \quad \quad \hat{h}_{4} = J \hat{a}_{2}\hat{b}^{\dagger},\\ \hat{h}_{5} &=& -G_{1} \hat{a}^{\dagger}_{1} \hat{a}_{1} \hat{c}_{1}, \quad \quad \quad \hat{h}_{6} = -G_{2} \hat{a}^{\dagger}_{2} \hat{a}_{2} \hat{c}_{2}, \\ {\omega}^{\prime}_{1} &=& {\varOmega}_{1} - \omega_{1}, \quad \quad \quad \quad {\omega}^{\prime}_{2} = {\varOmega}_{2} - \omega_{2}, \\ \omega_{3}^{\prime} &=& {\varOmega}_{1}-{\varOmega}_{f}, \quad \quad \quad \quad \omega_{4}^{\prime} = {\varOmega}_{2}-{\varOmega}_{f}, \\ \omega_{5}^{\prime}&=& \omega_{5m}, \quad \quad \quad \quad \quad \quad \omega_{6}^{\prime} = \omega_{6m}. \end{array} $$
(18)

Now, using the (16), we get to the following formula:

$$ \begin{array}{@{}rcl@{}} \hat{H}_{\text{eff}} &=& \frac{1}{\bar{\omega}^{\prime}_{11}} [\hat{h}^{\dagger}_{1},\hat{h}_{1}]+\frac{1}{\bar{\omega}^{\prime}_{12}} [\hat{h}^{\dagger}_{1},\hat{h}_{2}]e^{i(\omega_{1}^{\prime} - \omega_{2}^{\prime})t}+\frac{1}{\bar{\omega}^{\prime}_{13}} [\hat{h}^{\dagger}_{1},\hat{h}_{3}]e^{i(\omega_{1}^{\prime} - \omega_{3}^{\prime})t} \\ &+&\frac{1}{\bar{\omega}^{\prime}_{14}} [\hat{h}^{\dagger}_{1},\hat{h}_{4}]e^{i(\omega_{1}^{\prime} - \omega_{4}^{\prime})t}+\frac{1}{\bar{\omega}^{\prime}_{15}} [\hat{h}^{\dagger}_{1},\hat{h}_{5}]e^{i(\omega_{1}^{\prime} - \omega_{5}^{\prime})t} \\ &+&\frac{1}{\bar{\omega}^{\prime}_{16}} [\hat{h}^{\dagger}_{1},\hat{h}_{6}]e^{i(\omega_{1}^{\prime} - \omega_{6}^{\prime})t}+ \frac{1}{\bar{\omega}^{\prime}_{21}} [\hat{h}^{\dagger}_{2},\hat{h}_{1}]e^{i(\omega_{2}^{\prime} - \omega_{1}^{\prime})t}+\frac{1}{\bar{\omega}^{\prime}_{22}} [\hat{h}^{\dagger}_{2},\hat{h}_{2}]\\ &+&\frac{1}{\bar{\omega}^{\prime}_{23}} [\hat{h}^{\dagger}_{2},\hat{h}_{3}]e^{i(\omega_{2}^{\prime} - \omega_{3}^{\prime})t}+\frac{1}{\bar{\omega}^{\prime}_{24}}[\hat{h}^{\dagger}_{2},\hat{h}_{4}]e^{i(\omega_{2}^{\prime} - \omega_{4}^{\prime})t} \\ &+&\frac{1}{\bar{\omega}^{\prime}_{25}} [\hat{h}^{\dagger}_{2},\hat{h}_{5}]e^{i(\omega_{2}^{\prime} - \omega_{5}^{\prime})t}+ \frac{1}{\bar{\omega}^{\prime}_{26}} [\hat{h}^{\dagger}_{2},\hat{h}_{6}]e^{i(\omega_{2}^{\prime} - \omega_{6}^{\prime})t}\\ &+&\frac{1}{\bar{\omega}^{\prime}_{31}} [\hat{h}^{\dagger}_{3},\hat{h}_{1}]e^{i(\omega_{3}^{\prime} - \omega_{1}^{\prime})t}+\frac{1}{\bar{\omega}^{\prime}_{32}} [\hat{h}^{\dagger}_{3},\hat{h}_{2}]e^{i(\omega_{3}^{\prime} - \omega_{2}^{\prime})t}+\frac{1}{\bar{\omega}^{\prime}_{33}} [\hat{h}^{\dagger}_{3},\hat{h}_{3}] \\ &+&\frac{1}{\bar{\omega}^{\prime}_{34}} [\hat{h}^{\dagger}_{3},\hat{h}_{4}]e^{i(\omega_{3}^{\prime} - \omega_{4}^{\prime})t}+\frac{1}{\bar{\omega}^{\prime}_{35}} [\hat{h}^{\dagger}_{3},\hat{h}_{5}]e^{i(\omega_{3}^{\prime} - \omega_{5}^{\prime})t}\\ &+&\frac{1}{\bar{\omega}^{\prime}_{36}} [\hat{h}^{\dagger}_{3},\hat{h}_{6}]e^{i(\omega_{3}^{\prime} - \omega_{6}^{\prime})t}+\frac{1}{\bar{\omega}^{\prime}_{41}} [\hat{h}^{\dagger}_{4},\hat{h}_{1}]e^{i(\omega_{4}^{\prime} - \omega_{1}^{\prime})t}\\ &+&\frac{1}{\bar{\omega}_{42}} [\hat{h}^{\dagger}_{4},\hat{h}_{2}]e^{i(\omega_{4}^{\prime} - \omega_{2}^{\prime})t} +\frac{1}{\bar{\omega}_{43}} [\hat{h}^{\dagger}_{4},\hat{h}_{3}]e^{i(\omega_{4}^{\prime} - \omega_{3}^{\prime})t}+\frac{1}{\bar{\omega}^{\prime}_{44}} [\hat{h}^{\dagger}_{4},\hat{h}_{4}]\\ &+&\frac{1}{\bar{\omega}^{\prime}_{45}} [\hat{h}^{\dagger}_{4},\hat{h}_{5}]e^{i(\omega_{4}^{\prime} - \omega_{5}^{\prime})t}+\frac{1}{\bar{\omega}^{\prime}_{46}} [\hat{h}^{\dagger}_{4},\hat{h}_{6}]e^{i(\omega_{4}^{\prime} - \omega_{6}^{\prime})t}\\ &+&\frac{1}{\bar{\omega}^{\prime}_{51}} [\hat{h}^{\dagger}_{5},\hat{h}_{1}]e^{i(\omega_{5}^{\prime} - \omega_{1}^{\prime})t} +\frac{1}{\bar{\omega}^{\prime}_{52}} [\hat{h}^{\dagger}_{5},\hat{h}_{2}]e^{i(\omega_{5}^{\prime} - \omega_{2}^{\prime})t}\\ &+&\frac{1}{\bar{\omega}^{\prime}_{53}} [\hat{h}^{\dagger}_{5},\hat{h}_{3}]e^{i(\omega_{5}^{\prime} - \omega_{3}^{\prime})t}+\frac{1}{\bar{\omega}^{\prime}_{54}} [\hat{h}^{\dagger}_{5},\hat{h}_{4}]e^{i(\omega_{5}^{\prime} - \omega_{4}^{\prime})t}+\frac{1}{\bar{\omega}^{\prime}_{55}} [\hat{h}^{\dagger}_{5},\hat{h}_{5}] \\ &+&\frac{1}{\bar{\omega}^{\prime}_{56}} [\hat{h}^{\dagger}_{5},\hat{h}_{6}]e^{i(\omega_{5}^{\prime} - \omega_{6}^{\prime})t} + \frac{1}{\bar{\omega}^{\prime}_{61}} [\hat{h}^{\dagger}_{6},\hat{h}_{1}]e^{i(\omega_{6}^{\prime} - \omega_{1}^{\prime})t} \\ &+&\frac{1}{\bar{\omega}^{\prime}_{62}} [\hat{h}^{\dagger}_{6},\hat{h}_{2}]e^{i(\omega_{6}^{\prime} - \omega_{2}^{\prime})t}+\frac{1}{\bar{\omega}^{\prime}_{63}} [\hat{h}^{\dagger}_{6},\hat{h}_{3}]e^{i(\omega_{6}^{\prime} - \omega_{3}^{\prime})t} \\ &+&\frac{1}{\bar{\omega}^{\prime}_{64}} [\hat{h}^{\dagger}_{6},\hat{h}_{4}]e^{i(\omega_{6}^{\prime} - \omega_{4}^{\prime})t}+\frac{1}{\bar{\omega}^{\prime}_{65}} [\hat{h}^{\dagger}_{6},\hat{h}_{5}]e^{i(\omega_{6}^{\prime} - \omega_{5}^{\prime})t} +\frac{1}{\bar{\omega}^{\prime}_{66}} [\hat{h}^{\dagger}_{6},\hat{h}_{6}]. \end{array} $$
(19)

By substituting the defined parameters hi and \({\omega }^{\prime }_{i}\) from (18) into (19) and evaluating the commutators, we arrive at the effective Hamiltonian as follows:

$$ \begin{array}{@{}rcl@{}} \hat{H}_{\text{eff}}&=& \sum\limits_{{j}=1}^{2} \frac{\lambda_{j}{G}_{j}}{2} \left( \frac{1}{{\varOmega}_{j} - \omega_{j}} + \frac{1}{\omega_{jm}}\right) (\hat{a}^{\dagger}_{j} \hat{c}_{j} \hat{\sigma}_{j-} {e}^{i({\varOmega}_{j} - \omega_{j} -\omega_{jm}t )} + H.C) \\ &+& \sum\limits_{{j}=1}^{2} \frac{{J} {G}_{j}}{2} \left( \frac{1}{\omega_{jm}} + \frac{1}{{\varOmega}_{j} - {\varOmega}_{f}}\right) (\hat{a}^{\dagger}_{j} \hat{c}_{j} \hat{b} e^{i({\varOmega}_{j}-{\varOmega}_{f}-\omega_{jm}) t} + H.C ) \end{array} $$
$$ \begin{array}{@{}rcl@{}} &-& \sum\limits_{{j}=1}^{2} \frac{\lambda_{j} {J}}{2}\left( \frac{1}{{\varOmega}_{j} - \omega_{j}} +\frac{1}{{\varOmega}_{j} - {\varOmega}_{f}}\right) (\hat{b}^{\dagger} \hat{\sigma}_{j-} {e}^{-i (\omega_{j} - {\varOmega}_{f}) t} + H.C ) \\ &+& \frac{J^{2}}{2} \left( \frac{1}{{\varOmega}_{1} - \omega_{f}} + \frac{1}{{\varOmega}_{2} - \omega_{f}}\right) (\hat{a}^{\dagger}_{1} \hat{a}_{2} {e}^{i({\varOmega}_{1} - {\varOmega}_{2} )t} + H.C) \\ &-& \sum\limits_{{j}=1}^{2} \frac{{\lambda_{j}^{2}}}{{\varOmega}_{j} - \omega_{j}} (\hat{a}^{\dagger}_{j} \hat{a}_{j} \hat{\sigma}_{jz} + \hat{\sigma}_{j+} \hat{\sigma}_{j-}) \\ &-& \sum\limits_{{j}=1}^{2} \frac{{G}^{2}_{j}}{\omega_{jm}} (\hat{a}^{\dagger}_{j} \hat{a}_{j})^{2}\\ &+& \sum\limits_{{j}=1}^{2} \frac{{J}^{2}}{{\varOmega}_{j} - {\varOmega}_{f}}(\hat{a}^{\dagger}_{j}\hat{a}_{j} - \hat{b}^{\dagger}\hat{b}), \end{array} $$
(20)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Fathi, M.A., Baghshahi, H.R., Khanzadeh, M. et al. Atom-Atom Entanglement in a Hybrid Fiber-Atom-Optomechanical System. Int J Theor Phys 61, 62 (2022). https://doi.org/10.1007/s10773-022-05056-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10773-022-05056-3

Keywords

Search

Navigation