Unidimensional Continuous-variable Quantum Key Distribution Based on Basis-encoding Coherent States Protocol

Abstract

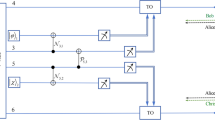
We propose the unidimensional continuous-variable quantum key distribution(UDCVQKD) protocol based on the basis-encoding of Gaussian modulated coherent states. the UDCVQKD protocol disregards the necessity in one of the quadrature modulations in coherent states. On that basis, we propose our scheme by encoding the secret keys on the either randomly selected measurement bases: the phase quadrature (X) or the amplitude quadrature (P), in order to slightly weaken the effects of reconciliation efficiency and channel excess noise compare to the present UCVQKD protocol. The new protocol with a view to simplify the precedent unidimensional protocols in the decoding procedure, meanwhile ensure the security of the quantum communication.

Appendix

In the BEUD CVQKD protocols, Alice sends the single modulated key information through the lossy and noisy channel to Bob. We focus on the Gaussian attacks in this paper, in which we assume the parameters ε_x = 𝜖_p = ε as the channel excess noise, we suppose that Eve would replace the quantum channel with a perfect displacement with no noise and lose, then she entangle ρ_b0 with her own states ∣ψ_E〉 and resends to Bob as camouflage, so that the entangled ρ_b0 would be the same as the state that passed through the noisy and lossy channel. Suppose that Bob uses homodyne detection with the electronics noise v and efficiency η, then the quadratures received by Bob can be calculated as

$$ \begin{array}{@{}rcl@{}} X_{B} &=& \sqrt{\eta T}X_{A}+\sqrt{\eta T}X_{ex}+\delta X_{v}+\delta X_{el},\\ P_{B} &=& \sqrt{\eta T}P_{A}+\sqrt{\eta T}P_{ex}+\delta P_{v}+\delta P_{el}. \end{array} $$

(18)

Where X_ex(P_ex), δX_v(δP_v), δX_el(δP_el) are generated from homodyne detection and denoted to the channel excess noise, shot noise and electronic noise, respectively. where$\left \langle {X_{ex}^{2}} \right \rangle$ = $\left \langle P_{ex}^{2} \right \rangle$ = ε_c,$\left \langle {X_{v}^{2}} \right \rangle$ = $\left \langle {P_{v}^{2}} \right \rangle$ = 1, and$\left \langle \delta X_{el}^{2} \right \rangle$ = $\left \langle \delta P_{el}^{2} \right \rangle$ = v in shot noise units, respectively.

After identify the quadrature of the output mode in proposed scheme, we will calculate the QBER of Alice’s decoding according to Bob’s measurement results. Because of the symmetry of the jugement rule for either X or P quadratures, we neglect the unmodulated quadrature and just focus on the case of X_A > P_A for simplicity. Accroding to (18), the inequality ${{\upbeta }_{A}^{x}}X_{B}<C_{A}$ and ${{\upbeta }_{A}^{p}}P_{B}>C_{A}$ can be expanded to [28].

$$ \begin{array}{@{}rcl@{}} X_{A}-P_{A} &=& -2\delta X_{ex}-\frac{2}{\sqrt{\eta T}}(\delta X_{v}+\delta X_{el}),\\ X_{A}-P_{A} &=& -2\delta P_{ex}-\frac{2}{\sqrt{\eta T}}(\delta P_{v}+\delta P_{el}). \end{array} $$

(19)

We set M = X_A − P_A, $\text {N}_{\text {x}}=-2\delta X_{ex}-\frac {2}{\sqrt {\eta T}}(\delta X_{v}+\delta X_{el})$ and $\text {N}_{\text {p}}=-2\delta \text {P}_{\text {ex}}-\frac {2}{\sqrt {\eta T}}(\delta P_{v}+\delta P_{el})$, the variables follow the normal distribution as

$$ M\sim(0,{\sigma_{m}^{2}}),N_{x},N_{p}\sim(0,{\sigma_{n}^{2}}). $$

(20)

where ${\sigma _{m}^{2}}=2V_{M}$ and ${\sigma _{n}^{2}}=4\left (\frac {\varepsilon _{c}\eta T+1+v}{\eta T}\right )$, The QBER between Alice and Bob can be derived as

$$ \begin{array}{@{}rcl@{}} P_{A B}& =&\frac{1}{2}[P(M<N_{x}\mid M>0)+P(M<N_{p}\mid M>0)]\\ & =&\frac{1}{2}[P(0<M<N_{x})/P(M>0) +P(0<M<N_{p})/P(M>0)]\\ & =&P(0<M<N_{x})+P(0<M<N_{p})\\ & =&\iint_{0<m<n_{x}} \frac{e^{\frac{m^{2}}{2{\sigma_{m}^{2}}}-\frac{{n_{x}^{2}}}{2\sigma_{n_{1}}^{2}}}}{2\pi\sigma_{m}\sigma_{n_{1}}} \text{dm} \text{dn}_{\text{x}}+ \iint_{0<m<n_{x}} \frac{e^{\frac{m^{2}}{2{\sigma_{m}^{2}}}-\frac{{n_{p}^{2}}}{2\sigma_{n_{1}}^{2}}}}{2\pi\sigma_{m}\sigma_{n_{1}}} \text{dm} \text{dn}_{\text{p}}\\ & =&\frac{1}{2\pi\sigma_{n_{1}}\sigma_{m}}{\int}_{n_{x}}^{0} e^{\frac{m^{2}}{2{\sigma_{m}^{2}}}} \text{dm}{\int}_{-\infty}^{0} e^{\frac{{n_{x}^{2}}}{2\sigma_{n_{1}}^{2}} } \text{dn}_{\text{x}}+ \quad\ \frac{1}{2\pi\sigma_{n_{1}}\sigma_{m}}{\int}_{n_{p}}^{0} e^{\frac{m^{2}}{2{\sigma_{m}^{2}}}} dm{\int}_{-\infty}^{0} e^{\frac{{n_{p}^{2}}}{2\sigma_{n_{1}}^{2}}} \text{dn}_{\text{p}}\\ & =&{\int}_{0}^{+\infty} \frac{1}{\sqrt{2\pi}\sigma_{n_{1}}}erf\left( \frac{n}{\sqrt{2}\sigma_{m}}\right)e^{-\frac{n^{2}}{2\sigma_{n_{1}}^{2}}} \text{dn}\\ & =&\text{arctan}\left( \frac{\sigma_{n_{1}}}{\sigma_{m}}\right)/\pi. \end{array} $$

(21)

where $\text {erf}(x)={{\int \limits }_{0}^{x}}e^{-t^{2}}dt$ is the error function.