Security Analysis of Practical Continuous-Variable Quantum Key Distribution Using a Heralded Noiseless Amplifier

Abstract

The noiseless linear amplifier(NLA) can probabilistically amplify the amplitude of the coherent state while retaining the initial level of noise. It is widely proposed and discussed to enhance various CVQKD protocols. In the previous work, when analyzing the security of the NLA-based CVQKQ protocol, they usually included the impact of NLA in a set of equivalent parameters. In this article, we develop this equivalent method and propose a new one to analyze the protocol with NLA which simplifies the computational complexity. In particular, we have further considered the effect of NLA on entanglement parameter of the EPR state. Then by using our proposed method, we first obtain the secret key rate of the NLA-enhanced CVQKD system with imperfect detection. The results show that the NLA can also improve the transmission distance of CVQKD protocols with imperfect detection by equivalent $20\log _{10}g$ dB of losses.

Appendices

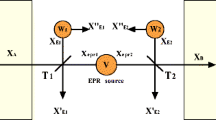
Appendix A: Acquisition of the Equivalent Entanglement Parameter

In this appendix, we discuss the acquisition of the equivalent entanglement parameter λ_t. As we know, if we trace out one of the two output modes of a two-mode squeezed state which has an equivalent parameter λ, we obtain a thermal state ρ. And the state ρ can be decomposed on an ensemble of coherent states using the P function,

$$ \rho=\int P(\alpha)|\alpha\rangle\langle\alpha|d\alpha, $$

(19)

where $P(\alpha )=\frac {1-\lambda ^{2}}{\pi \lambda ^{2}}e^{-\frac {1-\lambda ^{2}}{\lambda ^{2}}|\alpha |^{2}}=Ae^{-\frac {1-\lambda ^{2}}{\lambda ^{2}}|\alpha |^{2}}$. The above equation can be used to EB scheme, where one output mode of a two-mode squeezed state is sent to Bob through the channel, and P(α) is the probability that the coherent state |α〉 is transmitted.

In channel, the coherent state |α〉 is attenuated and simultaneously affected by the channel excess noise, which we can then record as $|\alpha ^{\prime }\rangle$. Here we can obtain the condition that $|\alpha ^{\prime }|^{2}=T|\alpha |^{2}+T\epsilon$, where ϵ is the variance of the channel excess noise. And then the state $|\alpha ^{\prime }\rangle$ is amplified and selected by the NLA. Here we only care about the selection effect since we only wants to find the equivalent parameter λ_t of the EPR state, and we can describe this effect using (3). Substituting $|\alpha ^{\prime }\rangle$ into the (3), we can calculate the successfully amplified probability $P_{sel}(\alpha )=\eta ^{N}e^{-(1-g^{2})|\alpha ^{\prime }|^{2}}$. This probability is equivalent to pre-screening our EPR state, since only the successful amplified case is reserved.

Considering the pre-screening effect of the NLA, the output mode of the EPR state that is sent to Bob can be rewritten as

$$ \begin{array}{@{}rcl@{}} &&\rho^{\prime} \propto\int P(\alpha)P_{sel}(\alpha)|\alpha\rangle\langle\alpha|d\alpha\\ &&\propto\frac{1-\lambda^{2}}{\pi\lambda^{2}}e^{-(1-g^{2})T\epsilon}\int e^{\left( -\frac{1-\lambda^{2}}{\lambda^{2}}-T(1-g^{2})\right)|\alpha|^{2}}|\alpha\rangle\langle\alpha|d\alpha\\ &&=A^{\prime}\int e^{\left( -\frac{1-\lambda^{2}}{\lambda^{2}}-T(1-g^{2})\right)|\alpha|^{2}}|\alpha\rangle\langle\alpha|d\alpha. \end{array} $$

(20)

For the new thermal state $\rho ^{\prime }$, its corresponding entanglement parameter λ_t should satisfy the condition that

$$ P(\alpha)=A^{\prime} e^{-\frac{1-{\lambda_{t}^{2}}}{{\lambda_{t}^{2}}}|\alpha^{2}|}=A^{\prime} e^{\left( -\frac{1-\lambda^{2}}{\lambda^{2}}-T(1-g^{2})\right)|\alpha|^{2}}. $$

(21)

And using it we can find the equivalent entanglement parameter λ_t as follows,

$$ \lambda_{t}=\frac{\lambda}{\sqrt{1-T\lambda^{2}(g^{2}-1)}}. $$

(22)

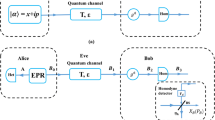
Appendix B: Acquisition of the Channel Equivalent Parameter

In this appendix, we detail the acquisition of the channel equivalent parameter T_t and ϵ_t. And they can be obtained from the following equation which describes the NLA’s influence on a displaced input thermal state $\hat \rho (\lambda _{0})$ and the NLA’s output state $\hat \rho ^{\prime }$ can be described as

$$ \hat\rho^{\prime}\propto \hat D (\widetilde g \beta)\hat\rho(g\lambda_{0})\hat D(\widetilde g \beta), $$

(23)

where β stands for the displacement of the thermal state, the parameter $\widetilde g = g\frac {{1-{\lambda _{0}^{2}}}}{1-g^{2}{\lambda _{0}^{2}}}$, g is the gain of the NLA, λ₀ is the parameter of the input thermal state $\hat \rho (\lambda _{0})=(1-{\lambda _{0}^{2}}){\sum }_{n=0}^{\infty }\lambda _{0}^{2n}|n\rangle \langle n|$, and the $\hat \rho ^{\prime }$ is the state we get at the other end of the NLA. This equation means that an input thermal state $\hat \rho (\lambda _{0})$ displaced by β = β_x + iβ_y will be changed by the NLA to another state $\hat \rho ^{\prime }$, $\hat \rho ^{\prime }$ is proportional to $(1-g^{2}{\lambda _{0}^{2}}){\sum }_{n=0}^{\infty }{(g\lambda _{0})}^{2n}|n\rangle \langle n|$, and its displacement is proportional to $\widetilde g\beta$.

The above equation describes the NLA’s amplification effect on the transmitted thermal state in channel. In the quantum channel a transmitted coherent state along with the excess noise acts as a thermal state. If a NLA is added, it amplifies the thermal state. What we want to find is an equivalent channel to generate the amplified thermal state without the NLA.

First, we consider the change of the state when we modulate nothing. In this condition V_A is zero, the variance of the input thermal state ρ(λ_ch) equals to the sum of the excess noise and shot noise, so we can find that

$$ \frac{1+\lambda_{ch}^{2}}{1-\lambda_{ch}^{2}}=T\epsilon+1. $$

(24)

After NLA’s amplification, the parameter λ_ch will be changed to gλ_ch. And according to (24), $\lambda _{ch}^{2}=\frac {T\epsilon }{2+T\epsilon }$, we find the first function describing the changes of the thermal state,

$$ \begin{array}{@{}rcl@{}} &&\frac{T\epsilon}{2+T\epsilon}\xrightarrow{NLA}g^{2}\frac{T\epsilon}{2+T\epsilon}\\ &&\frac{T_{t}\epsilon_{t}}{2+T_{t}\epsilon_{t}}=g^{2}\frac{T\epsilon}{2+T\epsilon}. \end{array} $$

(25)

Then we only consider the state that is modulated by Alice and ignore the noise. Always a coherent state is generated by displacing a vacuum state. After a coherent state transmitted through the channel, the excess noise changes this displaced vacuum state to a displaced thermal state. If the displaced value we modulated is β = β_x + iβ_y, under the effect of the NLA, it changes to $\widetilde g\beta$ according to (23). The amplification of the states’s amplitude will change the variance of the state received by Bob. Through the NLA, the variance of the transmitted coherent state TV (λ) will be changed to ${\widetilde g}^{2}TV(\lambda )$. Without considering the noise, we can get the second equation describing the changes of the thermal state, here $\lambda _{ch}^{2}=\frac {T\epsilon }{2+T\epsilon }$,

$$ \begin{array}{@{}rcl@{}} \sqrt{T}\beta_{x(y)}\xrightarrow{NLA}g\frac{{1-\lambda_{ch}^{2}}}{1-g^{2}\lambda_{ch}^{2}}\sqrt{T}\beta_{x(y)} \\ T_{t}\frac{1+{\lambda_{t}^{2}}}{1-{\lambda_{t}^{2}}}=(g\frac{{1-\lambda_{ch}^{2}}}{1-g^{2}\lambda_{ch}^{2}})^{2}T\frac{1+\lambda^{2}}{1-\lambda^{2}}. \end{array} $$

(26)

Last, we consider the total thermal state at Bob’s device. In the quantum channel $\hat \rho (\lambda _{tot})=(1-\lambda _{tot}^{2}){\sum }_{n=0}^{\infty }\lambda _{tot}^{2n}|n\rangle \langle n|$, using the same method we can know the NLA will change the parameter λ_tot to gλ_tot, the third equation can be expressed as

$$ \begin{array}{@{}rcl@{}} \frac{T(1+\lambda^{2})+T\epsilon(1-\lambda^{2})}{(2+T\epsilon)(1-\lambda^{2})+T(1+\lambda^{2})}\\ \xrightarrow{NLA}g^{2}\frac{T(1+\lambda^{2})+T\epsilon(1-\lambda^{2})}{(2+T\epsilon)(1-\lambda^{2})+T(1+\lambda^{2})} \\ \frac{T_{t}(1+{\lambda_{t}^{2}})+T_{t}\epsilon_{t}(1-{\lambda_{t}^{2}})}{(2+T_{t}\epsilon_{t})(1-{\lambda_{t}^{2}})+T_{t}(1+{\lambda_{t}^{2}})}\\ =g^{2}\frac{T(1+\lambda^{2})+T\epsilon(1-\lambda^{2})}{(2+T\epsilon)(1-\lambda^{2})+T(1+\lambda^{2})}. \end{array} $$

(27)

Generally speaking, using the three Eqs. (25), (26), (27) above, we can find three corresponding equivalent parameters. But actually, using Eqs. (25), (26) we can deduce the Eq. (27). It means that just considering the effect of NLA only two equivalent parameters can be found. In the previous works, they make mistakes when finding the Eq. (27). So, wrongly effective parameters are deduced, and the solutions don’t match the fact.

In fact, just considering the successfully amplified cases, only two effective parameters can be found, and the effective parameter λ_t should be found from method we proposed in Appendix A. And the two parameters can be expressed as follows,

$$ \begin{array}{@{}rcl@{}} &&T_{t} = \frac{4g^{2}}{[T\epsilon(1-g^{2})+2]^{2}}T \\ &&\epsilon_{t} = \epsilon-\frac{1}{2}T(g^{2}-1)\epsilon^{2}. \end{array} $$

(28)

Through the equations above, the parameters T_t an ϵ_t can be calculated easily. The NLA’s linear amplification effect is included in the (28) when the NLA successfully runs. Together with the (22) in Apendix A, they gives us the three effective parameters. When we calculate with the secret key rate, we can just using the parameters λ_tT_t and ϵ_t instead of λ, T and ϵ, even with the imperfect detection device added.