The Chiral Phase Transition and Thermodynamic Properties in the Nambu-Jona-Lasinio Model Using the Midpoint Technique

Abstract

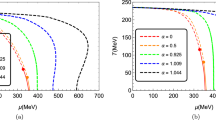
A midpoint method is introduced to calculate the chiral phase transition and thermodynamic properties in the Nambu-Jona-Lasinio (NJL) model with two flavors (u,d). The constituent quark mass, the pressure, the energy density, and the entropy are calculated in the mean-field approximation using the midpoint technique. The phase transition was found to be crossover for all values of bare quark mass. The effect of finite temperature and chemical potential on the thermodynamic properties is studied. A comparison with other models is presented. In addition, the advantages of the midpoint technique are discussed. In conclusion, the midpoint technique successfully predicts the phase transition and thermodynamic properties with a good accuracy for numerical integrals.

Appendix

In this Appendix, we write the detail of the calculation of (14) and the characteristic feature of the midpoint method. To calculate the integral

$$ M={{\int}_{a}^{b}}f(y)dy. $$

(A1)

We divide the interval [a,b] into N subintervals of length

$$ \triangle y=\frac{b\ -a}{N}, $$

(A2)

and the midpoint of the i th interval [y _i ,y _i+1] is

$$ A_{i}=a+\left(i+\frac{1}{2}\right)\triangle y{\kern7pt} ;i=0,1,2,...,N-1. $$

(A2)

The i the midpoint rectangle is the rectangle of height f(A _i ) over the subinterval [y _i ,y _i+1]. This rectangle signed area

$$ f(A_{i})\triangle y. $$

(A3)

Thus, M is equal to the sum of the signed areas of these rectangles

$$\begin{array}{@{}rcl@{}} M &=&\sum\limits_{i=0}^{N-1}f(A_{i})\triangle y, \\ &=&\frac{b\ -a}{N}\sum\limits_{i=0}^{N-1}f(A_{i}). \end{array} $$

(A4)

Now, we apply the above steps on (12), we can rewrite (12) as follows

$$ \rho_{s}=I_{1}+I_{2}^{+\mu }+I_{2}^{-\mu } $$

(A5)

where

$$\begin{array}{@{}rcl@{}} I_{1}&=&-\frac{6M}{\pi^{2}}^{\prime }{{\int}_{0}^{1}}\frac{\ln^{2}ydy}{y\sqrt{ \ln^{2}y+M^{^{\prime }2}}},\\ I_{2}^{\pm \mu^{\prime }} &=&\frac{6M}{\pi^{2} }^{\prime }{{\int}_{0}^{1}}\frac{\ln^{2}ydy}{y\sqrt{\ln^{2}y+M^{^{\prime }2}}\left[ 1+\exp \left[\frac{\left(\sqrt{\ln^{2}y+M^{^{\prime }2}}\pm \mu^{\prime }\right)}{ T^{\prime }}\right]\right]}. \end{array} $$

(A6)

To calculate I ₁, we determine $\triangle y=\frac {1}{N}$ and the midpoint of the i th interval [y _i ,y _i+1]

$$ A_{i}=\left(\frac{i}{N}+\frac{1}{2N}\right){\kern7pt} where~ i=0,1,2,...,N-1. $$

(A7)

Using (A4), we can write the integral I ₁

$$\begin{array}{@{}rcl@{}} I_{1} &=&-\frac{6M}{\pi^{2}}^{\prime }\sum\limits_{i=0}^{n-1}f_{1}(A_{i})\triangle y, \\ &=&-\frac{6M^{\prime }}{n\ \pi^{2}}\sum\limits_{i=0}^{n-1}\frac{\ln^{2}\left( A_{i}\right) }{A_{i}\sqrt{M^{^{\prime }2}+\ln^{2}\left( A_{i}\right) }}, \end{array} $$

(A8)

where

$$ A_{i}=\frac{i}{n}+\frac{1}{2n}. $$

(A9)

Similarly, we can write Integral $I_{2}^{\pm \mu ^{\prime }}$ as follows

$$ I_{2}^{\pm \mu^{\prime }}=\frac{6M}{\pi^{2}}^{\prime }\sum\limits_{i=1}^{n-1}f_{2}(A_{i})\triangle y $$

(A10)

where

$$ f_{2}(A_{i})=\frac{\ln^{2}A_{i}}{A_{i}\sqrt{\ln^{2}A_{i}+M^{^{\prime }2}}\left[ 1+\exp \left[\frac{\left(\sqrt{\ln^{2}A_{i}+M^{^{\prime }2}}\pm \mu^{\prime }\right)}{ T^{\prime }}\right]\right]}, $$

(A11)

therefore, we write (A10) as follows

$$ I_{2}^{\pm \mu^{\prime }}=\frac{6M^{\prime }}{n\ \pi^{2}}\sum\limits_{i=0}^{n-1} \frac{\ln^{2}\left( A_{i}\right) }{A_{i}\sqrt{M^{^{\prime }2}+\ln^{2}\left( A_{i}\right) }}\left[\frac{1}{\left[\exp \left( \frac{1}{T^{\prime }}\left( \pm \mu^{\prime }+\sqrt{M^{^{\prime }2}+\ln^{2}\left( A_{i}\right) } \right) \right) +1\right]}\right]. $$

(A12)

Substituting by (A8) and (A12) into (A5) , we obtain the final form of ρ _s as (14). The characteristic feature of the midpoint method that gives a good accuracy for numerical integrations in comparison with other numerical integrations that used in the calculations of thermodynamics properties in NJL model.