Hawking temperature of black holes with multiple horizons

Abstract

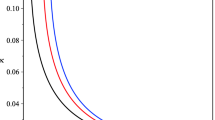
There are several well-established methods for computing thermodynamics in single-horizon spacetimes. However, understanding thermodynamics becomes particularly important when dealing with spacetimes with multiple horizons. Multiple horizons raise questions about the existence of a global temperature for such spacetimes. Recent studies highlight the significant role played by the contribution of all the horizons in determining Hawking’s temperature. Here we explore the Hawking temperature of a rotating and charged black hole in four spacetime dimensions and a rotating BTZ black hole. We also find that each horizon of those black holes contributes to the Hawking temperature. The effective Hawking temperature for a four-dimensional rotating and charged black hole depends only on its mass. This temperature is the same as the Hawking temperature of a Schwarzschild’s black hole. In contrast, the effective Hawking temperature depends on the black hole’s mass and angular momentum for a rotating BTZ hole.

Appendices

A Derivation of Eq. (25)

Equation (17) is the exact Dirac equation in curved space-time. Now to solve the equation we apply the Hamilton–Jacobi method for that we take the limit $\hbar \rightarrow 0$ and consider the equation upto $O(\hbar )$. Here, also we consider a mass-less charged particle, so in our case, $m=0$. Now upon substituting the ansatz (24) into the Eq. (17), it becomes evident that within an approximation up to $O(\hbar )$, we can neglect the spin coefficient $\omega _\mu ^{\alpha \beta }$. So, we start with an approximated Dirac equation by neglecting the spin coefficient,

$$\begin{aligned} -i\hbar \gamma ^\alpha e^\mu _\alpha \bigg (\partial _\mu +\frac{iq}{\hbar }A_\mu \bigg )\psi =0. \end{aligned}$$

(47)

If we consider only nonzero tetrad, then the above equation reduces to the following

$$\begin{aligned} \begin{aligned}&-i\hbar \bigg (\gamma ^0 e^t_0\partial _t+\gamma ^0 e^\phi _0\partial _\phi +\gamma ^1 e^r_1\partial _r+\gamma ^2 e^\theta _2\partial _\theta +\gamma ^3 e^\phi _3\partial _\phi +\gamma ^0 e^t_0\frac{iq}{\hbar }A_t\\&\quad +\gamma ^0 e^\phi _0\frac{iq}{\hbar }A_\phi +\gamma ^3e^\phi _3\frac{iq}{\hbar }A_\phi \bigg )\psi =0. \end{aligned} \end{aligned}$$

(48)

Four Gamma matrices are

$$\begin{aligned} \begin{aligned}&\gamma ^0=\begin{pmatrix} i &{} \quad 0 &{} \quad 0 &{} \quad 0\\ 0 &{} \quad i &{} \quad 0 &{} \quad 0\\ 0 &{} \quad 0 &{} \quad -i &{} \quad 0\\ 0 &{} \quad 0 &{} \quad 0 &{} \quad -i\\ \end{pmatrix} \;\;\; \gamma ^1=\begin{pmatrix} 0 &{} \quad 0 &{} \quad 1 &{} \quad 0\\ 0 &{} \quad 0 &{} \quad 0 &{} \quad -1\\ 1 &{} \quad 0 &{} \quad 0 &{} \quad 0\\ 0 &{} \quad -1 &{} \quad 0 &{} \quad 0\\ \end{pmatrix}\\&\gamma ^2=\begin{pmatrix} 0 &{} \quad 0 &{} \quad 0 &{} \quad -i\\ 0 &{} \quad 0 &{} \quad i &{} \quad 0\\ 0 &{} \quad -i &{} \quad 0 &{} \quad 0\\ i &{} \quad 0 &{} \quad 0 &{} \quad 0\\ \end{pmatrix} \;\; \;\;\; \gamma ^3=\begin{pmatrix} 0 &{} \quad 0 &{} \quad 0 &{} \quad 1\\ 0 &{} \quad 0 &{} \quad 1 &{} \quad 0\\ 0 &{} \quad 1 &{} \quad 0 &{} \quad 0\\ 1 &{} \quad 0 &{} \quad 0 &{} \quad 0\\ \end{pmatrix}. \end{aligned} \end{aligned}$$

(49)

By inserting the values of gamma matrices, we can write the equation (48) as,

$$\begin{aligned} -i\hbar \begin{pmatrix} A &{} \quad 0 &{} \quad B &{} \quad C\\ 0 &{} \quad A &{} \quad D &{} \quad -B\\ B &{} \quad C &{} \quad -A &{} \quad 0\\ D &{} \quad -B &{} \quad 0 &{} \quad -A \\ \end{pmatrix} \begin{pmatrix} \alpha (t,r,\theta ,\phi ) \\ 0 \\ \beta (t,r,\theta ,\phi )\\ 0\\ \end{pmatrix}e^{\frac{i}{\hbar }{\mathcal {I}}(t,r,\theta ,\phi )}= \begin{pmatrix} 0 \\ 0 \\ 0\\ 0\\ \end{pmatrix}~, \end{aligned}$$

(50)

where A, B, and C are

$$\begin{aligned} \begin{aligned}&A=i\bigg (e^t_0\partial _t+e^t_0\frac{iq}{\hbar }A_t +e^\phi _0\partial _\phi +e^\phi _0\frac{iq}{\hbar }A_\phi \bigg ),\\&B=e^r_1\partial _r,\\&C= -ie^\theta _2\partial _\theta +e^\phi _3\partial _\phi +e^\phi _3\frac{iq}{\hbar }A_\phi .\\&D= ie^\theta _2\partial _\theta +e^\phi _3\partial _\phi +e^\phi _3\frac{iq}{\hbar }A_\phi ~ \end{aligned} \end{aligned}$$

(51)

Now, using the expression of A, B, C, and D in equation (50), we get

$$\begin{aligned} \begin{pmatrix} \alpha \bigg \{i(e^t_0\partial _t+e^\phi _0\partial _\phi ){\mathcal {I}}+ie^t_0qA_t+ie^\phi _0qA_\phi \bigg \} +\beta e^r_1\partial _r{\mathcal {I}}+o(\hbar )\\ \beta (ie^\theta _2 \partial _\theta {\mathcal {I}}+e^\phi _3\partial _\phi {\mathcal {I}}+qe^\phi _3A_\phi )+o(\hbar )\\ \alpha e^r_1\partial _r{\mathcal {I}}-\beta \bigg \{i(e^t_0\partial _t+e^\phi _0\partial _\phi ){\mathcal {I}} +ie^t_0qA_t+ie^\phi _0qA_\phi \bigg \}+o(\hbar )\\ \alpha (ie^\theta _2\partial _\theta {\mathcal {I}}+e^\phi _3\partial _\phi {\mathcal {I}}+qe^\phi _3A_\phi )+o(\hbar ) \end{pmatrix}e^{\frac{i}{\hbar }{\mathcal {I}}(t,r,\theta ,\phi )}=\begin{pmatrix} 0 \\ 0 \\ 0\\ 0\\ \end{pmatrix}. \end{aligned}$$

(52)

Thus, we arrive at the following four equations,

$$\begin{aligned} \begin{aligned} \alpha \bigg \{i(e^t_0\partial _t+e^\phi _0\partial _\phi ){\mathcal {I}}+ie^t_0qA_t+ie^\phi _0qA_\phi \bigg \}+\beta e^r_1\partial _r{\mathcal {I}}=0\\ \beta (ie^\theta _2 \partial _\theta {\mathcal {I}}+e^\phi _3\partial _\phi {\mathcal {I}}+qe^\phi _3A_\phi )=0\\ \alpha e^r_1\partial _r{\mathcal {I}}-\beta \bigg \{i(e^t_0\partial _t +e^\phi _0\partial _\phi ){\mathcal {I}}+ie^t_0qA_t+ie^\phi _0qA_\phi \bigg \}=0\\ \alpha (ie^\theta _2\partial _\theta {\mathcal {I}}+e^\phi _3\partial _\phi {\mathcal {I}}+qe^\phi _3A_\phi )=0. \end{aligned} \end{aligned}$$

(53)

B Derivation of Eq. (41)

Here, we apply a similar procedure for writing an approximated Dirac equation for a rotating BTZ black hole. The approximated Dirac equation for the rotating BTZ black hole spacetime is then given by,

$$\begin{aligned} \begin{aligned} -i\hbar \gamma ^\alpha e^\mu _\alpha \partial _\mu \psi =0\\ \implies -i\hbar \bigg (\gamma ^0 e^\mu _0\partial _\mu +\gamma ^1 e^\mu _1\partial _\mu +\gamma ^2 e^\mu _2\partial _\mu \bigg )\psi =0~ \end{aligned}. \end{aligned}$$

(54)

There are three gamma matrices in three dimensions $\gamma ^i =(i\sigma ^2,\sigma ^1,\sigma ^3)$. Where $(\sigma ^1,\sigma ^2,\sigma ^3)$ are the three spin Pauli matrices. Now, considering the nonzero tetrads for BTZ black hole, we can write Eq. (54) as,

$$\begin{aligned}&-i\begin{pmatrix} e^\phi _2\partial _\phi &{} (e^t_0\partial _t+e^\phi _0\partial _\phi )+e^r_1\partial _r\\ e^r_1\partial _r-(e^t_0\partial _t+e^\phi _0\partial _\phi ) &{} - e^\phi _2\partial _\phi \end{pmatrix}\begin{pmatrix} \alpha (t,r,\phi )\\ \beta (t,r,\phi )\end{pmatrix}e^{\frac{i}{\hbar }{\mathcal {I}}(t,\theta ,\phi )}=\begin{pmatrix} 0\\ 0 \end{pmatrix}\nonumber \\&\quad \implies \begin{pmatrix} \alpha e^\phi _2\partial _\phi {\mathcal {I}}+\beta (e^r_1\partial _r{\mathcal {I}} +e^t_0\partial _t{\mathcal {I}}+e^\phi _0\partial _\phi {\mathcal {I}})+o(\hbar )\\ \alpha (e^r_1\partial _r{\mathcal {I}}-e^t_0\partial _t{\mathcal {I}}-e^\phi _0\partial _\phi {\mathcal {I}})-\beta e^\phi _2\partial _\phi {\mathcal {I}}+o(\hbar ) \end{pmatrix}e^{\frac{i}{\hbar }{\mathcal {I}}(t,\theta ,\phi )}=\begin{pmatrix} 0\\ 0 \end{pmatrix}. \end{aligned}$$

(55)

So we get a set of two equations as follows,

$$\begin{aligned} \begin{aligned} \alpha e^\phi _2\partial _\phi {\mathcal {I}}+\beta (e^r_1\partial _r{\mathcal {I}} +e^t_0\partial _t{\mathcal {I}}+e^\phi _0\partial _\phi {\mathcal {I}})=0\\ \alpha (e^r_1\partial _r{\mathcal {I}}-e^t_0\partial _t{\mathcal {I}}-e^\phi _0\partial _\phi {\mathcal {I}})-\beta e^\phi _2\partial _\phi {\mathcal {I}}=0. \end{aligned} \end{aligned}$$

(56)