A transient phase in cosmological evolution : A multi-fluid approximation for a quasi-thermodynamical equilibrium

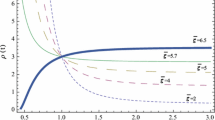
Abstract

This article presents the study of a multi-species fluid characterised by a freeze-out or break-away of one or more species. Whereas single-fluid approximation suffices for modelling of the pre freeze-out period, multi-fluid approximation is required for the post freeze-out period. Embedded in this is a transition period where neither one of the two approximations is singly appropriate. We examine the thermodynamics of this fluid and find that the second law holds before, during and after the freeze-out. Our application to cosmological modelling involving interacting dark-section species indicates that the species’ equations of state are modified.

Appendices

A The Mueller-Israel-Stewart (MIS)Theory

The Mueller-Israel-Stewart (MIS) theory was developed for single-fluid approximation [16,17,18]. the main parameters are the energy-momentum tensor, $T^{\mu \nu }$, and the particel 4-current, $N^{\nu }$, subject to the conservation laws $\nabla _{\mu }T^{\mu \nu }=0, \nabla _{\mu }N^{\mu }=0.$ Given the 4-velocity, $u^{\mu }$, and the projection tensor, $h^{\mu \nu }=g^{\mu \nu }+u^{\mu }u^{\nu }$, the two state parameters decompose into

$$\begin{aligned} N^{\mu }= & {} \mathbf{n} u^{\mu }+n^{\mu } \end{aligned}$$

(25)

$$\begin{aligned} T^{\mu \nu }= & {} \rho u^{\mu }u^{\nu }+Ph^{\mu \nu }+2u^{(\mu }q^{\nu )}+\pi ^{\mu \nu }, \end{aligned}$$

(26)

where $\mathbf{n}=N^{\mu }u_{\mu }$ is the particle number density and $n^{\mu }$ is the diffusion current. $\rho =u_{\nu }u_{\mu }T^{\nu \mu }$ is the energy density, P is the aggregate of the equilibrium, p, and the bulk viscosity pressures, $\varPi$. The aggregate gives the isotropic pressure. The anisotropic pressure is given by $\pi ^{\nu \mu }$ while $q^{\mu }$ is the heat flux vector. The extra quantities, $q^{\mu },\pi ^{\mu }, \varPi$ are the dissipative quantities. But this theory is for short range interactions within hydrodynamics regime. It’s extension to multifluid, which extends beyond the hydrodynamics regime, is considered in [23]. We will refer to this as the extended MIS theory. In this extended theory, the primary extensive parameters are taken to be the species number flux current $N^{\mu }_{i}$, the stress momentum tensor $T^{\mu \nu }_{(i)}$, and the entropy flux vector $S^{\mu }_{(i)}$. In this case, the total stress-energy momentum is conserved but not the individual i.e. $\nabla _{\mu }T^{\mu \nu }=\nabla _{\mu }(\sum _{i}T^{\mu \nu }_{(i)} +\bar{T}^{\mu \nu })=0\ne \nabla _{\mu }T^{\mu \nu }_{(i)}$, where $\bar{T}^{\mu \nu }$ is the momentum due to the interactions. The decompositions take the form;

$$\begin{aligned} N^{\mu }_{(i)}=\mathbf{n}_{(i)}u^{\mu }+n^{\mu }_{(i)}, \end{aligned}$$

(27)

where $\mathbf{n}_{i}$ is the species number density and $n^{\mu }_{i}$ is the species diffusion current. Similarly

$$\begin{aligned} T^{\mu \nu }_{(i)}=\rho _{(i)}u^{\mu }u^{\nu }+p_{(i)}h^{\mu \nu } +2u^{(\mu }_{(i)}q^{\nu )}_{(i)}+\pi ^{\mu \nu }_{(i)}, \end{aligned}$$

(28)

where $h^{\mu \nu }=g^{\mu \nu }+u^{\mu }u^{\nu }$ is the projection tensor to the rest frame of the various fluid species. This projection is only applicable upon the separation of species. Here too, $\rho _{(i)}=u_{\nu }u_{\mu }T^{\nu \mu }_{(i)}$ is the energy density, $p_{(i)}$ is the isotropic pressure, $\pi ^{\nu \mu }_{(i)}$ is the anisotropic pressure, $q^{\mu }_{(i)}$ is the heat flux vector. The use of different velocities have also been considered in single-fluid approximation, for example in the comparison of the energy and the particle frames in [16], or the rest frame and the boosted frame in [37]. The entropy vector takes the form:

$$\begin{aligned} S^{\mu }_{(i)}= & {} s_{(i)}u^{\mu }+s^{\mu }_{(i)} \end{aligned}$$

(29)

$$\begin{aligned}= & {} s_{(i)}u^{\mu }_{(i)}+\frac{q^{\mu }}{T}\nonumber \\&-\left( \beta _{(i)}\varPi ^2+\beta _{1(i)}q_{(i)\nu }q^{\nu }_{(i)} +\beta _{2(i)}\pi _{(i)\gamma \delta }\pi ^{\gamma \delta }_{(i)}\right) \frac{u^{\mu }}{2T}\nonumber \\&+\left( \alpha _{0(i)}\varPi q^{\mu }_{(i)}+\alpha _{1(i)}\pi ^{\mu \nu }_{(i)}q_{(i)\nu }\right) \frac{1}{T}. \end{aligned}$$

(30)

$s_{(i)}$ is the entropy density, $s^{\mu }_{(i)}$ is the entropy flux with respect to $u^{\mu }$ such that $s_{\mu (i)}u^{\mu }=0$. $\varPi _{(i)}$ is the bulk viscosity. The complexity of the detailed interactions is immense but tractable as will be demonstrated in [23]. In this regard, the total entropy vector takes the phenomenological expressions

$$\begin{aligned} S^{\mu }=\sum _{i}S^{\mu }_{i}+\bar{S}^{\mu }, \end{aligned}$$

(31)

where, as in the stress-energy-momentum tensor, the term with the bar denotes interaction effects. It is important to reflect on the dynamics and changes that take place in this approximation. We postulate that there is a gradual change that sees the terms with the bars being important at $t_{qe}$ to having no effect at some $t>t_{qe}$. In other words, transiting to truly separate fluids requiring a full multifluid approximation. We now consider an application to cosmology.

B An evolving apparent horizon

We gave a definition of the horizon radius

$$\begin{aligned} {\tilde{r}}_{A}= & {} \frac{1}{\sqrt{H^2+\frac{\kappa }{a^2}}} \end{aligned}$$

(32)

in section (2). It will be noticed that one can replace the radius using Eq.(3). In particular,

$$\begin{aligned} H^2+\frac{\kappa }{a^2}= & {} \frac{1}{{\tilde{r}}^{2}_{A}} =\frac{1}{3}(\rho _{DE}+\rho _{M}+\rho _{\chi }). \end{aligned}$$

(33)

The evolution with respect to proper time, t, yields

$$\begin{aligned} \frac{d}{dt} \left( H^{2}+\frac{\kappa }{a^2} \right)= & {} -2\frac{{\dot{{\tilde{r}}}}_{A}}{{\tilde{r}}^3_{A}}\nonumber \\= & {} \frac{1}{3}({\dot{\rho }}_{DE}+{\dot{\rho }}_{DM}+{\dot{\rho }}_{X}), \end{aligned}$$

(34)

from which it follows that

$$\begin{aligned} {\dot{{\tilde{r}}}}_{A}= & {} -\frac{{\tilde{r}}^3_{A}}{6}({\dot{\rho }}_{DE}+{\dot{\rho }}_{DM} +{\dot{\rho }}_{\chi }) \end{aligned}$$

(35)