Stable exponential cosmological solutions with two factor spaces in the Einstein–Gauss–Bonnet model with a $\Lambda$-term

Abstract

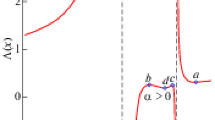
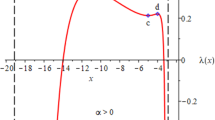
We study D-dimensional Einstein–Gauss–Bonnet gravitational model including the Gauss–Bonnet term and the cosmological term $\Lambda$. We find a class of solutions with exponential time dependence of two scale factors, governed by two Hubble-like parameters $H >0$ and h, corresponding to factor spaces of dimensions $m >2$ and $l > 2$, respectively. These solutions contain a fine-tuned $\Lambda = \Lambda (x, m, l, \alpha )$, which depends upon the ratio $h/H = x$, dimensions of factor spaces m and l, and the ratio $\alpha = \alpha _2/\alpha _1$ of two constants ($\alpha _2$ and $\alpha _1$) of the model. The master equation $\Lambda (x, m, l,\alpha ) = \Lambda$ is equivalent to a polynomial equation of either fourth or third order and may be solved in radicals. The explicit solution for $m = l$ is presented in “Appendix”. Imposing certain restrictions on x, we prove the stability of the solutions in a class of cosmological solutions with diagonal metrics. We also consider a subclass of solutions with small enough variation of the effective gravitational constant G and show the stability of all solutions from this subclass.

Appendices

Appendix

The analytical solution for $m=l$

For any $m = l > 2$ the master Eq. (3.33) reads

$$\begin{aligned} A x^4+B x^3 + C x^2 + B x + A = 0, \end{aligned}$$

(A.1)

where

$$\begin{aligned} A= & {} 8 \lambda (m-2)^2(m-1) + m(m+1)(m-2), \end{aligned}$$

(A.2)

$$\begin{aligned} B= & {} 32 \lambda (m-2)(m-1)^2 + 4 m (m-1)^2, \end{aligned}$$

(A.3)

$$\begin{aligned} C= & {} 16 \lambda (m-1)(3m^2-8m+6) + 2m(m-1)(3m -4). \end{aligned}$$

(A.4)

It may be readily solved in radicals, by using the substitution $y = x + \frac{1}{x}$ [33]. For $A \ne 0$ we obtain

$$\begin{aligned} \begin{aligned} x= \frac{1}{4A} \left( - B + \nu _1 \sqrt{E - 2 B \nu _2 \sqrt{d}} + \nu _2 \sqrt{d} \right) , \end{aligned} \end{aligned}$$

(A.5)

where $\nu _1 = \pm \,1$, $\nu _2 = \pm \,1$ and

$$\begin{aligned} d = 8A^2 - 4 C A + B^2, \qquad E = - 8A^2 - 4CA + 2 B^2. \end{aligned}$$

(A.6)

We get

$$\begin{aligned} d= & {} 16 m^2(2m^2-7m+7) - 128 m(m-1)(m-2) (2m-3) \lambda , \end{aligned}$$

(A.7)

$$\begin{aligned} E= & {} 1024 \lambda ^2(m-2)^2(m-1)^2(2m-3) \nonumber \\&+\,128 \lambda (m-2)(m-1)m(4m-7) \nonumber \\&-\,16 m^2 (2 m^3- 11 m^2+15 m-4). \end{aligned}$$

(A.8)

For $A = 0$, the solution reads

$$\begin{aligned} \begin{aligned} x= \frac{1}{2B} \left[ - C \pm \sqrt{C^2 -4 B^2 } \right] , \quad \mathrm{or} \ x=0, \end{aligned} \end{aligned}$$

(A.9)

where

$$\begin{aligned} \begin{aligned} B = - 8 m (m-1), \qquad C = - \frac{4m}{m-2} (4 m^2-10m+7). \end{aligned} \end{aligned}$$

(A.10)

The special solution for $m=3$ was considered recently in ref. [27].

The proof of the Lemma

Here we give the proof of the Lemma from Sect. 2. The calculations (by using Mathematica) lead us to following relations

$$\begin{aligned} {\mathcal {R}}_{\pm }(m,l)={\mathcal {R}}(x_{\pm }(m,l),m,l)=\frac{A(m,l) \pm B(m,l)\sqrt{\Delta (m,l)}}{C(l)} \end{aligned}$$

(B.11)

where

$$\begin{aligned} A(m,l)&=-2(m-1)(l+m-3)A_{*}(m,l),\\ A_{*}(m,l)&=l^2m^2+4lm^2-4m^2+l^3m-4l^2m-8lm+8m-2l^3+8l^2-4l,\\ B(m,l)&=8l(m-1)^2(l+m-3)>0,\\ \Delta (m,l)&=(m-1)(l-1)(l+m-3)>0,\\ C(l)&= (l - 2)^3 (l -1) > 0. \end{aligned}$$

In order to prove ${\mathcal {R}}_{-}(m,l) <0$ it is sufficient to prove that $A_{*}(m,l) > 0$ for $m>2$ and $l>2$.

Let $m \ge 4$. Then we group $A_*(m,l)$ as the sum of the non-negative terms:

$$\begin{aligned} A_*(m,l)= & {} (l^2m^2-4l^2m)_1+(4lm^2-4m^2-8lm)_2 \\&+(l^3m-2l^3)_3+(8m)_4+(8l^2-4l)_5; \end{aligned}$$

where

$$\begin{aligned} (.)_1&=l^2m^2-4l^2m=l^2m(m-4)\ge 0, \\ (.)_2&=4lm^2-4m^2-8lm=2(l-2)m^2+2lm(m-4)>0, \\ (.)_3&=l^3(m-2)>0, \\ (.)_4&>0, \\ (.)_5&=4l(2l-1)>0. \end{aligned}$$

Thus, we get $A_{*}(m,l) > 0$ for $m \ge 4$ and $l > 2$. For $m=3$ we have $A_*(3,l)=l^3+5l^2+8l-12 \ge 84$ (as $l\ge 3$). Thus, ${\mathcal {R}}_{-}(m,l) < 0$ ($m>2$, $l>2$) is proved.

Now we prove ${\mathcal {R}}_{+}(m,l) < 0$ ($m>2$, $l>2$)). By using the identities (3.18), (3.25) and definitions of ${\mathcal {R}}_{\pm }(m,l)$ we obtain

$$\begin{aligned} {\mathcal {R}}_{+}(m,l)= & {} {\mathcal {R}}(x_{+}(m,l),m,l) = (x_{+}(m,l))^4 {\mathcal {R}}\left( \frac{1}{x_{+}(m,l)},l,m\right) \nonumber \\= & {} (x_{+}(m,l))^4 {\mathcal {R}}(x_{-}(l,m),l,m) = (x_{+}(m,l))^4 {\mathcal {R}}_{-}(l,m) < 0.\nonumber \\ \end{aligned}$$

(B.12)

By this we complete the proof of the Lemma.