Motion of charged particles in a NUTty Einstein–Maxwell spacetime and causality violation

Abstract

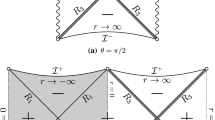
We investigate the motion of electrically charged test particles in spacetimes with closed timelike curves, a subset of the black hole or wormhole Reissner–Nordström-NUT spacetimes without periodic identification of time. We show that, while in the wormhole case there are closed worldlines inside a potential well, the wordlines of initially distant charged observers moving under the action of the Lorentz force can never close or self-intersect. This means that for these observers causality is preserved, which is an instance of our weak chronology protection criterion.

Appendix: Circular orbits with \(r\ne 0\)

From (3.15) and (3.16), $\beta$ and $\delta$ can be expressed in terms of z as

$$\begin{aligned} \beta = \frac{\alpha + \overline{l}^2z^2}{z}, \quad \delta = \frac{\alpha - \overline{l}^2z^2}{z}. \end{aligned}$$

(A.1)

Using this, we obtain from (3.10)

$$\begin{aligned} y_0 = \frac{-\delta (\alpha +\overline{l}^{2}) + \beta (\alpha -\overline{l}^{2})}{2\alpha \overline{l}^{2}\delta } = \frac{1-z^2}{\overline{l}^2z^2-\alpha }, \end{aligned}$$

(A.2)

which can be inverted to

$$\begin{aligned} z^2 = \frac{1+\alpha y_0}{1+\overline{l}^2 y_0}. \end{aligned}$$

(A.3)

We also obtain from (A.1) and (A.2) the value of the effective energy $\mathcal{E}(r_0)=E_0+(\beta /2)y_0$:

$$\begin{aligned} \mathcal{E}(r_0) = \frac{(\overline{l}^2-\alpha )z}{\overline{l}^2z^2-\alpha } = (1+\overline{l}^2y_0)z, \end{aligned}$$

(A.4)

so that the effective energy is positive provided

$$\begin{aligned} z>0. \end{aligned}$$

(A.5)

In the black-hole case or extreme-black-hole case, $-2\le \alpha \le -1$, (A.3) is positive definite provided

$$\begin{aligned} 0< y_0 < y_h = - \frac{1}{\alpha }, \end{aligned}$$

(A.6)

so that the circular orbits must be outside the horizon ($r_0>r_h$). The allowed range of z is then from (A.2)

$$\begin{aligned} 0< z < 1, \end{aligned}$$

(A.7)

leading from (A.1) to the condition for the existence of these circular orbits:

$$\begin{aligned} \overline{l}^2 > \beta -\alpha . \end{aligned}$$

(A.8)

In the wormhole case, $\alpha >-1$, $y_0$ can vary in the full range $0<y_0<1$, leading to the allowed range of z

$$\begin{aligned} \begin{array}{lcc} \displaystyle \frac{\overline{b}}{\gamma }< z< 1 &{}\qquad \mathrm{if} &{}\quad \overline{l}^2>\alpha , \\ 1< z< \displaystyle \frac{\overline{b}}{\gamma }&{}\qquad \mathrm{if} &{}\quad \overline{l}^2<\alpha \end{array} \end{aligned}$$

(A.9)

[where $\overline{b}$ and $\gamma$ are related to $\alpha$ and $\overline{l}^2$ by (3.8)]. Both cases lead to the same bounds for the existence of an unstable circular orbit of radius $r=\pm r_0$,

$$\begin{aligned} \frac{\alpha + \overline{l}^2 + 2\alpha \overline{l}^2}{\overline{b}\gamma }< \beta < \alpha + \overline{l}^2. \end{aligned}$$

(A.10)

For $\alpha >0$, the lower bound ensures that the first existence condition (3.13) is satisfied, due to the identity

$$\begin{aligned} (\alpha + \overline{l}^2 + 2\alpha \overline{l}^2)^2 = 4\alpha \overline{l}^2\overline{b}^2\gamma ^2 + (\alpha - \overline{l}^2)^2. \end{aligned}$$

(A.11)

Note that in the parameter range (A.10) there is also from (3.9) a stable circular orbit at $r=0$.