Skip to main content
SpringerLink
Log in

Generating the mapping class group of a nonorientable surface by three torsions

  • Original Paper
  • Published:
Geometriae Dedicata Aims and scope Submit manuscript

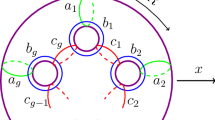
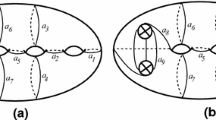
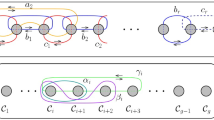
Abstract

We prove that the mapping class group \(\mathcal {M}(N_g)\) of a closed nonorientable surface of genus g different than 4 is generated by three torsion elements. Moreover, for every even integer \(k\ge 12\) and g of the form \(g=pk+2q(k-1)\) or \(g=pk+2q(k-1)+1\), where p, q are non-negative integers and p is odd, \(\mathcal {M}(N_g)\) is generated by three conjugate elements of order k. Analogous results are proved for the subgroup of \(\mathcal {M}(N_g)\) generated by Dehn twists.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

Data availability

Data sharing not applicable to this article as no datasets were generated or analysed during the current study.

References

  1. Altunöz, T., Pamuk, M., Yildiz, O.: Generating the mapping class group of a nonorientable surface by two elements or by three involutions. Preprint 2021. arXiv:2104.10958

  2. Altunöz, T., Pamuk, M., Yildiz, O.: Torsion generators of the twist subgroup. Preprint 2020. arxiv:2001.06326

  3. Altunöz, T., Pamuk, M., Yildiz, O.: Generating the twist subgroup by involutions. Preprint 2020. arXiv:1912.10685

  4. Birman, J.S., Chillingworth, D.R.J.: On the homeotopy group of a non-orientable surface. Proc. Camb. Philos. Soc. 71, 437–448 (1972)

    Article  MathSciNet  Google Scholar 

  5. Du, X.: The torsion generating set of the mapping class groups and the Dehn twist subgroups of nonorientable surfaces of odd genus. Hiroshima Math. J. 50, 199–206 (2020)

  6. Epstein, D.B.A.: Curves on 2-manifolds and isotopies. Acta Math. 115, 83–107 (1966)

    Article  MathSciNet  Google Scholar 

  7. Farb, B., Margalit, D.: A Primer on Mapping Class Groups. Princeton University Press, Princeton (2011)

    Book  Google Scholar 

  8. Korkmaz, M.: First homology group of mapping class group of nonorientable surfaces. Math. Proc. Camb. Philos. Soc. 123, 487–499 (1998)

    Article  MathSciNet  Google Scholar 

  9. Korkmaz, M.: Mapping class groups of nonorientable surfaces. Geom. Dedic. 89, 109–133 (2002)

    Article  MathSciNet  Google Scholar 

  10. Korkmaz, M.: Generating the surface mapping class group by two elements. Trans. Am. Math. Soc. 357, 3299–3310 (2005)

    Article  MathSciNet  Google Scholar 

  11. Lanier, J., Margalit, D.: Normal generators for mapping class groups are abundant. Comment. Math. Helv. 97, 1–59 (2022)

  12. Lanier, J.: Generating mapping class groups with elements of fixed finite order. J. Algebra 511, 455–470 (2018)

    Article  MathSciNet  Google Scholar 

  13. Lickorish, W.B.R.: On the homeomorphisms of a non-orientable surface. Proc. Camb. Philos. Soc. 61, 61–64 (1965)

    Article  MathSciNet  Google Scholar 

  14. Leśniak, M. Torsion normal generators of the mapping class group of a non-orientable surface. J. Pure Appl. Algebra 226, 106964 (2022)

  15. McCarthy, J., Papadopoulos, A.: Involutions in surface mapping class groups. Enseign. Math. 33, 275–290 (1987)

    MathSciNet  MATH  Google Scholar 

  16. Maclachlan, C.: Modulus space is simply-connected. Proc. Am. Math. Soc. 29(1), 85–86 (1971)

    Article  MathSciNet  Google Scholar 

  17. Omori, G.: A small generating set for the twist subgroup of the mapping class group of a non-orientable surface by Dehn twists. Hiroshima Math. J. 48, 81–88 (2018)

    Article  MathSciNet  Google Scholar 

  18. Paris, L., Szepietowski, B.: A presentation for the mapping class group of a nonorientable surface. Bull. Soc. Math. France 143, 503–566 (2015)

    Article  MathSciNet  Google Scholar 

  19. Stukow, M.: The twist subgroup of the mapping class group of a non-orientable surface. Osaka J. Math. 46, 717–738 (2009)

    MathSciNet  MATH  Google Scholar 

  20. Stukow, M.: A finite presentation for the twist subgroup of the mapping class group of a nonrientable surface. Bull. Korean Math. Soc. 53, 601–614 (2016)

    Article  MathSciNet  Google Scholar 

  21. Szepietowski, B.: Mapping class group of a non-orientable surface and moduli space of Klein surfaces. C. R. Math. Acad. Sci. Paris 335(12), 1053–1056 (2002)

    Article  MathSciNet  Google Scholar 

  22. Szepietowski, B.: The mapping class group of a nonorientable surface is generated by three elements and by four involutions. Geom. Dedic. 117, 1–9 (2006)

    Article  MathSciNet  Google Scholar 

  23. Szepietowski, B.: A presentation for the mapping class group of a non-orientable surface from the action on the complex of curves. Osaka J. Math. 45, 283–326 (2008)

    MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute of Mathematics, Faculty of Mathematics, Physics and Informatics, University of Gdańsk, 80-308, Gdańsk, Poland

    Marta Leśniak & Błażej Szepietowski

Authors
  1. Marta Leśniak
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Błażej Szepietowski
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Błażej Szepietowski.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Leśniak, M., Szepietowski, B. Generating the mapping class group of a nonorientable surface by three torsions. Geom Dedicata 216, 40 (2022). https://doi.org/10.1007/s10711-022-00698-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10711-022-00698-3

Keywords

Mathematics Subject Classification

Search

Navigation