Interface fracture mechanics: theory and experiment

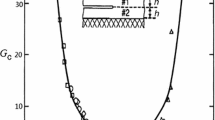
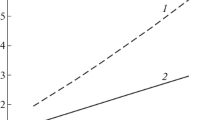
Abstract

The purpose of this paper is to review a methodology for predicting interface crack propagation. The materials will be taken to be isotropic and anisotropic. The first term of the asymptotic expansion for the stress and displacement fields is considered. A deterministic failure criterion derived from the interface energy release rate is described. In order to predict failure by means of a statistical approach, two statistical measures are examined. One makes use of a $t$-distribution for statistical intervals and the other uses the standard variate to determine a failure probability and confidence interval. The failure probability was set to 10 %, that is there is a 10 % probability that the next observation would unexpectedly be below the failure curve or surface in the safe region. For the $t$-statistic, there was a 50 % confidence; for the standard variant model, there was a 95 % confidence. Test results are presented with the failure criteria obtained and examined.

Appendices

Appendix 1: Stress and displacement functions of the first term of the asymptotic expansion of an interface crack between two linear elastic, homogeneous and isotropic materials

The stress and displacement functions for the first term of the asymptotic expansion of an interface crack between two linear elastic, homogeneous and isotropic materials are presented here. For material (1), the upper material, the stress functions in Eq. (1) are

$$\begin{aligned}&_{1}\varSigma _{11}^{(1)}(\theta ,\varepsilon )\nonumber \\&\quad = -\frac{1}{\cosh \pi \varepsilon }\left\{ \left[ \sinh (\pi - \theta )\varepsilon - e^{-(\pi - \theta )\varepsilon }\right] \cos \frac{\theta }{2}\right. \nonumber \\&\qquad \left. +\, \frac{1}{2} e^{-(\pi - \theta )\varepsilon }\sin \theta \left[ \sin \frac{3\theta }{2} - 2\varepsilon \cos \frac{3\theta }{2} \right] \right\} \end{aligned}$$

(49)

$$\begin{aligned}&_{1}\varSigma _{12}^{(1)}(\theta ,\varepsilon )\nonumber \\&\quad = -\frac{1}{\cosh \pi \varepsilon }\left\{ \sinh (\pi - \theta )\varepsilon \;\sin \frac{\theta }{2} \right. \nonumber \\&\qquad \left. -\, \frac{1}{2} e^{-(\pi - \theta )\varepsilon }\sin \theta \left[ \cos \frac{3\theta }{2} + 2\varepsilon \sin \frac{3\theta }{2} \right] \right\} \end{aligned}$$

(50)

$$\begin{aligned}&_{1}\varSigma _{22}^{(1)}(\theta ,\varepsilon ) \nonumber \\&\quad = \frac{1}{\cosh \pi \varepsilon }\left\{ \left[ \sinh (\pi - \theta )\varepsilon + e^{-(\pi - \theta )\varepsilon }\right] \cos \frac{\theta }{2}\right. \nonumber \\&\qquad \left. +\, \frac{1}{2} e^{-(\pi - \theta )\varepsilon }\sin \theta \left[ \sin \frac{3\theta }{2} - 2\varepsilon \cos \frac{3\theta }{2} \right] \right\} \end{aligned}$$

(51)

$$\begin{aligned}&_{1}\varSigma _{11}^{(2)}(\theta ,\varepsilon ) \nonumber \\&\quad = -\frac{1}{\cosh \pi \varepsilon }\left\{ \left[ \cosh (\pi - \theta )\varepsilon +e^{-(\pi - \theta )\varepsilon }\right] \sin \frac{\theta }{2}\right. \nonumber \\&\qquad \left. +\, \frac{1}{2} e^{-(\pi - \theta )\varepsilon }\sin \theta \left[ \cos \frac{3\theta }{2} + 2\varepsilon \sin \frac{3\theta }{2} \right] \right\} \end{aligned}$$

(52)

$$\begin{aligned}&_{1}\varSigma _{12}^{(2)}(\theta ,\varepsilon ) \nonumber \\&\quad = \frac{1}{\cosh \pi \varepsilon }\left\{ \cosh (\pi - \theta )\varepsilon \;\cos \frac{\theta }{2}\right. \nonumber \\&\qquad \left. -\, \frac{1}{2} e^{-(\pi - \theta )\varepsilon }\sin \theta \left[ \sin \frac{3\theta }{2} - 2\varepsilon \cos \frac{3\theta }{2} \right] \right\} \end{aligned}$$

(53)

$$\begin{aligned}&_1\varSigma _{22}^{(2)}(\theta ,\varepsilon ) \nonumber \\&\quad = \frac{1}{\cosh \pi \varepsilon }\left\{ \left[ \cosh (\pi - \theta )\varepsilon - e^{-(\pi - \theta )\varepsilon }\right] \sin \frac{\theta }{2}\right. \nonumber \\&\qquad \left. +\, \frac{1}{2} e^{-(\pi - \theta )\varepsilon }\sin \theta \left[ \cos \frac{3\theta }{2} + 2\varepsilon \sin \frac{3\theta }{2} \right] \right\} . \end{aligned}$$

(54)

For the upper material, the out-of-plane stress functions in Eqs. (5) are given by

$$\begin{aligned} _1\varSigma _{31}^{(III)}(\theta )&= -\sin \frac{\theta }{2}\end{aligned}$$

(55)

$$\begin{aligned} _1\varSigma _{32}^{(III)}(\theta )&= \cos \frac{\theta }{2}. \end{aligned}$$

(56)

For material (1), the upper material, the displacement functions in Eq. (6) are given by

$$\begin{aligned} _{1}U_1^{(1)}&= -\frac{1}{\mu _1 (1 + 4\varepsilon ^2) \cosh \pi \varepsilon } \left\{ \left[ \sinh (\pi - \theta )\varepsilon \right. \right. \nonumber \\&\quad \left. \left. - \frac{\kappa _1 - 1}{2} e^{-(\pi - \theta )\varepsilon }\right] \cos \frac{\theta }{2} -\,\frac{1}{2}(1 + 4\varepsilon ^2)\right. \nonumber \\&\quad \left. \times \, e^{-(\pi - \theta )\varepsilon } \sin \theta \sin \frac{\theta }{2} + 2\varepsilon \left[ \cosh (\pi - \theta )\varepsilon \right. \right. \nonumber \\&\quad \left. \left. +\, \frac{\kappa _1 - 1}{2} e^{-(\pi - \theta )\varepsilon } \right] \sin \frac{\theta }{2} \right\} \end{aligned}$$

(57)

$$\begin{aligned} _{1}U_2^{(1)}&= \frac{1}{\mu _1 (1 + 4\varepsilon ^2) \cosh \pi \varepsilon } \left\{ \left[ \cosh (\pi - \theta )\varepsilon \right. \right. \nonumber \\&\quad \left. \left. +\,\frac{\kappa _1 - 1}{2} e^{-(\pi - \theta )\varepsilon }\right] \sin \frac{\theta }{2} -\frac{1}{2}(1 + 4\varepsilon ^2)\right. \nonumber \\&\quad \left. \times \, e^{-(\pi - \theta )\varepsilon } \sin \theta \cos \frac{\theta }{2} - 2\varepsilon \left[ \sinh (\pi - \theta )\varepsilon \right. \right. \nonumber \\&\quad \left. \left. -\, \frac{\kappa _1 - 1}{2} e^{-(\pi - \theta )\varepsilon } \right] \cos \frac{\theta }{2} \right\} \end{aligned}$$

(58)

$$\begin{aligned} _{1}U_1^{(2)}&= \frac{1}{\mu _1 (1 + 4\varepsilon ^2) \cosh \pi \varepsilon } \left\{ \left[ \cosh (\pi - \theta )\varepsilon \right. \right. \nonumber \\&\quad \left. \left. +\,\frac{\kappa _1 - 1}{2} e^{-(\pi - \theta )\varepsilon }\right] \sin \frac{\theta }{2}+ \frac{1}{2}(1 + 4\varepsilon ^2)\right. \nonumber \\&\quad \left. \times \, e^{-(\pi - \theta )\varepsilon } \sin \theta \cos \frac{\theta }{2} - 2\varepsilon \left[ \sinh (\pi - \theta )\varepsilon \right. \right. \nonumber \\&\quad \left. \left. -\, \frac{\kappa _1 - 1}{2} e^{-(\pi - \theta )\varepsilon } \right] \cos \frac{\theta }{2} \right\} \end{aligned}$$

(59)

$$\begin{aligned} _{1}U_2^{(2)}&= \frac{1}{\mu _1 (1 + 4\varepsilon ^2) \cosh \pi \varepsilon } \left\{ \left[ \sinh (\pi - \theta )\varepsilon \right. \right. \nonumber \\&\quad \left. \left. -\,\frac{\kappa _1 - 1}{2} e^{-(\pi - \theta )\varepsilon }\right] \cos \frac{\theta }{2} +\frac{1}{2}(1 + 4\varepsilon ^2)\right. \nonumber \\&\quad \left. \times \, e^{-(\pi - \theta )\varepsilon } \sin \theta \sin \frac{\theta }{2} + 2\varepsilon \left[ \cosh (\pi - \theta )\varepsilon \right. \right. \nonumber \\&\quad \left. \left. +\, \frac{\kappa _1 - 1}{2} e^{-(\pi - \theta )\varepsilon } \right] \sin \frac{\theta }{2} \right\} . \end{aligned}$$

(60)

where $\mu _1$ is the shear modulus of the upper material and $\kappa _1$ is defined in Eq. (4). For the upper material, the out-of-plane displacement in Eq. (7) is given by

$$\begin{aligned} _1U_3^{(III)} = \frac{2}{\mu _1} \sin \frac{\theta }{2} . \end{aligned}$$

(61)

For material (2), the lower material, replace $\pi$ with $-\pi$ in Eqs. (49)–(54) and (57)–(60). In addition, replace $\mu _1$ by $\mu _2$ and $\kappa _1$ by $\kappa _2$ in Eqs. (57)–(61).

Appendix 2: Stress and displacement functions of the first term of the asymptotic expansion for an interface delamination of a $0^{\circ }/90^{\circ }$ laminate for mode III

In this Appendix, the stress functions $_k\varSigma _{\alpha 3}^{(III)}(\theta )$ in Eq. (5) and the displacement functions $_kU_{3}^{(III)}(\theta )$ in Eq. (7) are presented. For the upper material (fibers in the $0^{\circ }$-direction)

$$\begin{aligned} _1 \varSigma _{13}^{(III)}&= -\frac{\beta _3}{B_3^{1/4}} \sin \frac{\phi _3}{2} \end{aligned}$$

(62)

$$\begin{aligned} _{1} \varSigma _{23}^{(III)}&= \frac{1}{B_3^{1/4}} \cos \frac{\phi _3}{2}\end{aligned}$$

(63)

$$\begin{aligned} _{1} U_3^{(III)}&= \frac{2 B_3^{1/4}}{\beta _3 G_T} \sin \frac{\phi _3}{2} \end{aligned}$$

(64)

where the third eigenvalue of the constitutive equations is $p_3 = \beta _3 i$,

$$\begin{aligned} B_3 = \cos ^2 \theta + \beta _3^2 \sin ^2 \theta \end{aligned}$$

(65)

and

$$\begin{aligned} \phi _3 = \arctan \left( \beta _3 \tan \theta \right) . \end{aligned}$$

(66)

For the lower material (fibers in the $90^{\circ }$-direction),

$$\begin{aligned} _2 \varSigma _{13}^{(III)}&= -\sin \frac{\theta }{2} \end{aligned}$$

(67)

$$\begin{aligned} _{2} \varSigma _{23}^{(III)}&= \cos \frac{\theta }{2} \end{aligned}$$

(68)

$$\begin{aligned} _{2} U_{3}^{(III)}&= \frac{2}{G_A} \sin \frac{\theta }{2}. \end{aligned}$$

(69)