Sustainable partial backordering inventory model under linked-to-order credit policy and all-units discount with capacity constraint and carbon emissions

Abstract

In today’s competitive market environment, providing different sorts of concessions are often observed from the suppliers/manufacturers to their customers for escalating the number of order quantity, as well as work mutually to control the rising level of carbon emission along with long-lasting financial benefits. So, it is challenging for researchers and industry to develop low carbon inventory models that can meet emission reduction targets while maintaining company’s profit. The credit function plays an important role within the organization. Furthermore, with the globalization of the marketing policy, the supplier may provide the retailer a discounted price if the quantity of purchase is large enough, and green inventory management reduces the environmental impact of a business without affecting its profitability. In this paper, we study a profit maximizing green economic order quantity (EOQ) model by considering capacity constraints under order-size dependent trade credit and all-units discount, along with minimizing carbon emissions for a cleaner environment. Shortages are allowed and partially backordered. The paper discusses all the potential cases, which may occur in green inventory models with carbon emission costs under different allowable delay-in-payments. We find that if retailers’ own warehouse capacity is relatively small, they always benefit from enlarging order quantities and renting an extra warehouse. Finally, some numerical examples are presented to illustrate the applicability of the proposed model. Sensitivity analysis of the major parameters is performed and some insights are obtained.

Appendices

Appendix 1 Equivalent transformation of the objective function

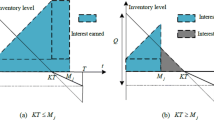
Case 1–1: ${M}_{j}\le KT\le {T}_{w}$

Using $K+\left(1-K\right)\beta =1-\left(1-K\right)\left(1-\beta \right)$ and ${\pi }_{j}=p+{c}_{g}-{c}_{j}$, Eq. (7) can be rewritten as below

$$\begin{array}{l}AT{P}_{11}^{\left(j\right)}\left(K,T\right)=pD-\left[\frac{A+\tau {A}^{^{\prime}}}{T}+\right.\left({c}_{j}+\tau {c}^{^{\prime}}\right)D+{\pi }_{j}D\left(1-K\right)\left(1-\beta \right)+\frac{{c}_{b}\beta D{\left(1-k\right)}^{2}T}{2}\\\qquad\qquad\qquad\qquad +\ \frac{\left({h}_{0}+\tau {h}_{0}^{^{\prime}}\right)D{K}^{2}T}{2}-\left.\frac{{{p{I}_{e}DM}_{j}}^{2}}{2T}-p{I}_{e}D\left(1-K\right)\beta {M}_{j}+\frac{{c}_{j}{I}_{c}D{\left(KT-{M}_{j}\right)}^{2}}{2T}\right]\end{array}$$

(51)

From Eq. (51), $AT{P}_{11}^{\left(j\right)}\left(K,T\right)$ is maximized by minimizing the expression which is

$$\begin{array}{l}AT{C}_{11}^{\left(j\right)}\left(K,T\right)=\left[\frac{A+\tau {A}^{^{\prime}}}{T}+\right.\left({c}_{j}+\tau {c}^{^{\prime}}\right)D+{\pi }_{j}D\left(1-K\right)\left(1-\beta \right)+\frac{{c}_{b}\beta D{\left(1-k\right)}^{2}T}{2}\\\qquad\qquad\qquad\qquad +\ \frac{\left({h}_{0}+\tau {h}_{0}^{^{\prime}}\right)D{K}^{2}T}{2}-\left.\frac{{{p{I}_{e}DM}_{j}}^{2}}{2T}-p{I}_{e}D\left(1-K\right)\beta {M}_{j}+\frac{{c}_{j}{I}_{c}D{\left(KT-{M}_{j}\right)}^{2}}{2T}\right]\end{array}$$

(52)

Further, making some algebraic manipulation, Eq. (52) can be rearranged to Eq. (53),

$$\begin{array}{l}AT{C}_{11}^{\left(j\right)}\left(K,T\right)={\underbrace{\frac{D}{2}\left[{h}_{0}+\tau {h}_{0}^{^{\prime}}+{c}_{b}\beta +{c}_{j}{I}_{c}\right]}_{{\varphi }_{111}}}{K}^{2}T-{\underbrace{{c}_{b}\beta D}_{{\varphi }_{112}}}KT\\\qquad\qquad\qquad\quad -{\underbrace{\left[{\pi }_{j}D\left(1-\beta \right)+\left\{{c}_{j}{I}_{c}-p\beta {I}_{e}\right\}D{M}_{j}\right]}_{{\varphi }_{113}}}K+{\underbrace{\frac{{c}_{b}\beta D}{2}}_{{\varphi }_{114}}}T\\\qquad\qquad\qquad\quad +\frac{1}{T}{\underbrace{\left[A+{c}_{j}{A}^{^{\prime}}+\frac{\left\{{c}_{j}{I}_{c}-p{I}_{e}\right\}D{{M}_{j}}^{2}}{2}\right]}_{{\varphi }_{115}}}+{\underbrace{\left[\left({c}_{j}+{c}_{j}{c}^{^{\prime}}\right)D+{\pi }_{j}D\left(1-\beta \right)-p\beta {I}_{e}{DM}_{j}\right]}_{{\varphi }_{116}}}\end{array}$$

(53)

Case 1–2: $KT\le {M}_{j}\le {T}_{w}$ or $KT\le {T}_{w}\le {M}_{j}$

$$\begin{array}{c}AT{P}_{12}^{\left(j\right)}\left(K,T\right)=pD-\left[\frac{A+\tau {A}^{^{\prime}}}{T}+\right.\left({c}_{j}+\tau {c}^{^{\prime}}\right)D+{\pi }_{j}D\left(1-K\right)\left(1-\beta \right)+\frac{{c}_{b}\beta D{\left(1-k\right)}^{2}T}{2}\\\qquad\qquad +\ \frac{\left({h}_{0}+\tau {h}_{0}^{^{\prime}}\right)D{K}^{2}T}{2}-\left.p{I}_{e}D\left\{K\left({M}_{j}-\frac{KT}{2}\right)+\left(1-K\right){\beta M}_{j}\right\}\right]\end{array}$$

(54)

Maximizing Eq. (54) is equivalent to minimizing the following function

$$\begin{array}{l}AT{C}_{12}^{\left(j\right)}\left(K,T\right)={\underbrace{\frac{D}{2}\left\{\left({h}_{0}+\tau {h}_{0}^{^{\prime}}\right)+{c}_{b}\beta +p{I}_{e}\right\}}_{{\varphi }_{121}}}{K}^{2}T-{\underbrace{{c}_{b}\beta D}_{{\varphi }_{122}}}KT\\\qquad\qquad\qquad\quad -\ {\underbrace{\left[{\pi }_{j}D\left(1-\beta \right)+\left(1-\beta \right) p{I}_{e}{M}_{j}D\right]}_{{\varphi }_{123}}}K+{\underbrace{\frac{{c}_{b}\beta D}{2}}_{{\varphi }_{124}}}T+\frac{1}{T}{\underbrace{A+\tau {A}^{^{\prime}}}_{{\varphi }_{125}}}\\\qquad\qquad\qquad\quad +{\underbrace{\left\{\left({c}_{j}+\tau {c}^{^{\prime}}\right)D+{\pi }_{j}D\left(1-\beta \right)-p\beta {I}_{e}{DM}_{j}\right\}}_{{\varphi }_{126}}}\end{array}$$

(55)

Case 2–1: ${M}_{j}\le {T}_{w}\le KT$ or ${T}_{w}\le {M}_{j}\le KT$

$$\begin{array}{l}AT{P}_{21}^{\left(j\right)}\left(K,T\right)=pD-\left[\frac{A+\tau {A}^{^{\prime}}}{T}+\right.\left({c}_{j}+\tau {c}^{^{\prime}}\right)D+{\pi }_{j}D\left(1-K\right)\left(1-\beta \right)+\frac{{c}_{b}\beta D{\left(1-k\right)}^{2}T}{2}\\ +\frac{\left({h}_{r}+\tau {h}_{r}^{^{\prime}}\right){\left(KDT-W\right)}^{2}}{2DT}+\left.\frac{\left({h}_{0}+\tau {h}_{0}^{^{\prime}}\right)\left(2DKT-W\right)W}{2DT}-p{I}_{e}D\left\{\frac{{{M}_{j}}^{2}}{2T}+\left(1-K\right)\beta {M}_{j}\right\}+\frac{{c}_{j}{I}_{c}D{\left(KT-{M}_{j}\right)}^{2}}{2T}\right]\end{array}$$

(56)

Maximizing Eq. (56) is equivalent to minimizing the following function

$$\begin{array}{l}AT{C}_{21}^{\left(j\right)}\left(K,T\right)=\frac{D}{2}\left[{h}_{r}+\tau {h}_{r}^{^{\prime}}+{c}_{b}\beta +{c}_{j}{I}_{c}\right]{\varphi }_{211}{K}^{2}T-{c}_{b}\beta D{\varphi }_{212}KT\\ -\left[{\pi }_{j}D\left(1-\beta \right)+\left({h}_{r}+\tau {h}_{r}^{^{\prime}}-{h}_{0}-\tau {h}_{0}^{^{\prime}}\right)W+\left\{{c}_{j}{I}_{c}-p\beta {I}_{e}\right\}D{M}_{j}]\right.{\varphi }_{213}K\\ +\frac{{c}_{b}\beta D}{2}{\varphi }_{214}T+\frac{1}{T}\left\{A+\tau {A}^{^{\prime}}+\frac{{\left({h}_{r}+\tau {h}_{r}^{^{\prime}}-{h}_{0}-\tau {h}_{0}^{^{\prime}}\right)W}^{2}}{2D}+\frac{\left\{{c}_{j}{I}_{c}-p{I}_{e}\right\}D{{M}_{j}}^{2}}{2}\right\}{\varphi }_{215}\\ +\left\{\left({c}_{j}+\tau {c}^{^{\prime}}\right)D+{\pi }_{j}D\left(1-\beta \right)-p\beta {I}_{e}{DM}_{j}\right\}{\varphi }_{216}\end{array}$$

(57)

Case 2–2: ${T}_{w}\le KT\le {M}_{j}$

$$\begin{array}{l}AT{P}_{22}^{\left(j\right)}\left(K,T\right)=pD-\left[\frac{A+\tau {A}^{^{\prime}}}{T}+\right.\left({c}_{j}+\tau {c}^{^{\prime}}\right)D+{\pi }_{j}D\left(1-K\right)\left(1-\beta \right)+\frac{{c}_{b}\beta D{\left(1-k\right)}^{2}T}{2}\\ +\frac{\left({h}_{r}+\tau {h}_{r}^{^{\prime}}\right){\left(KDT-W\right)}^{2}}{2DT}+\left.\frac{\left({h}_{0}+\tau {h}_{0}^{^{\prime}}\right)\left(2DKT-W\right)W}{2DT}-p{I}_{e}D\left\{K\left({M}_{j}-\frac{KT}{2}\right)+\left(1-K\right){\beta M}_{j}\right\}\right]\end{array}$$

(58)

Maximizing Eq. (58) is equivalent to minimizing the following function

$$\begin{array}{l}AT{C}_{22}^{\left(j\right)}\left(K,T\right)=\frac{D}{2}\left[{h}_{r}+\tau {h}_{r}^{^{\prime}}+{c}_{b}\beta +p{I}_{e}\right]{\varphi }_{221}{K}^{2}T-{c}_{b}\beta D{\varphi }_{222}KT\\ -\left\{{\pi }_{j}D\left(1-\beta \right)+\left({h}_{r}+\tau {h}_{r}^{^{\prime}}-{h}_{0}-\tau {h}_{0}^{^{\prime}}\right)W+\left(1-\beta \right)p{I}_{e}{M}_{j}D\right\}{\varphi }_{223}K\\ +\frac{{c}_{b}\beta D}{2}{\varphi }_{224}T+\frac{1}{T}A+{A}^{^{\prime}}+\frac{\left({h}_{r}+\tau {h}_{r}^{^{\prime}}-{h}_{0}-\tau {h}_{0}^{^{\prime}}\right){W}^{2}}{2D}{\varphi }_{225}\\ +\left\{\left({c}_{j}+\tau {c}^{^{\prime}}\right)D+{\pi }_{j}D\left(1-\beta \right)-p\beta {I}_{e}{DM}_{j}\right\}{\varphi }_{226}\end{array}$$

(59)

Appendix 2 Find the optimal values when ${\varphi }_{115}\le 0$  and ${\varphi }_{215}\le 0$

For Case 1–1, if ${\varphi }_{115}\le 0,$ we have ${\theta }_{11}\left(K\right)={\varphi }_{111}{K}^{2}-{\varphi }_{112}K+{\varphi }_{114}>0,$ which always holds for any $K\in \left[0, 1\right]$. So if ${\varphi }_{115}\le 0,$ then

$$AT{C}_{11}^{\left(j\right)}\left(K,T\right)={\varphi }_{111}{K}^{2}-{\varphi }_{112}K+{\varphi }_{114}-\frac{{\varphi }_{115}}{{T}^{2}}>0$$

(60)

From Eq. (60), we can conclude that $AT{C}_{11}^{\left(j\right)}\left(K,T\right)$ is a strictly increasing function of T. Therefore, $AT{C}_{11}^{\left(j\right)}\left(K,T\right)$ reaches its minimum at $T=\frac{{M}_{j}}{K}$. Then substituting $T=\frac{{M}_{j}}{K}$ into Eq. (11) lead to

$$AT{C}_{11}^{\left(j\right)}\left(K,\frac{{M}_{j}}{K}\right)={\varphi }_{111}K{M}_{j}-{\varphi }_{112}{M}_{j}-{\varphi }_{113}K+{\varphi }_{114}\frac{{M}_{j}}{K}+\frac{{\varphi }_{115}K}{{M}_{j}}+{\varphi }_{116}$$

(61)

Taking the first and second derivatives of Eq. (70) with respect to K, we have

$$AT{C}_{11}^{{^{\prime}}\left(j\right)}\left(K,\frac{{M}_{j}}{K}\right)={\varphi }_{111}{M}_{j}-{\varphi }_{113}-{\varphi }_{114}\frac{{M}_{j}}{{K}^{2}}+\frac{{\varphi }_{115}}{{M}_{j}}$$

(62)

$$AT{C}_{11}^{{^{\prime}}{^{\prime}}\left(j\right)}\left(K,\frac{{M}_{j}}{K}\right)=\frac{2{M}_{j{\varphi }_{114}}}{{K}^{3}}>0$$

(63)

Clearly, $AT{C}_{11}^{\left(j\right)}\left(K,T\right)$ is a strictly convex function of K. Setting $AT{C}_{11}^{\left(j\right)}\left(K,\frac{{M}_{j}}{K}\right)=0$ yields

$${K{^{\prime}}}_{11}=\sqrt{\frac{{\varphi }_{114}{{M}_{j}}^{2}}{{\varphi }_{111}{{M}_{j}}^{2}-{\varphi }_{113}{M}_{j}+{\varphi }_{115}}}$$

(64)

Therefore, from Eq. (64), if ${{K}^{^{\prime}}}_{11}$ is feasible, the optimal solution in this case is $\left({T}_{11}, {K}_{11}\right)=\left(\frac{{M}_{j}}{{{K}^{^{\prime}}}_{11}}, {{K}^{^{\prime}}}_{11}\right).$ Otherwise the optimal solution is $\left({T}_{11}, {K}_{11}\right)=({M}_{j},1)$.

Similarly, for Case 2–1, if ${\varphi }_{215}\le 0,$ following the same steps used in Case 1–1 to develop ${{K}^{^{\prime}}}_{21}$, we have

$${K{^{\prime}}}_{21}=\sqrt{\frac{{\varphi }_{214}{\left(max\left\{{T}_{w},{M}_{j}\right\}\right)}^{2}}{{\varphi }_{211}{\left(max\left\{{T}_{w},{M}_{j}\right\}\right)}^{2}-{\varphi }_{213}\left\{{T}_{w},{M}_{j}\right\}+{\varphi }_{215}}}$$

(65)

For Eq. (65), if ${{K}^{^{\prime}}}_{21}$ is feasible, the optimal solution in this case is $\left({T}_{21}, {K}_{21}\right)=\left(\frac{max\left\{{T}_{w},{M}_{j}\right\}}{{K{^{\prime}}}_{21}}, {K{^{\prime}}}_{21}\right)$.

Otherwise the optimal solution is $\left({T}_{21}, {K}_{21}\right)=(max\left\{{T}_{w},{M}_{j}\right\},1)$.

Appendix 3 Find the roots $\left({K}_{11}, {T}_{11}\right)$, $\left({K}_{12}, {T}_{12}\right)$, $\left({K}_{21}, {T}_{21}\right)$, and $\left({K}_{22}, {T}_{22}\right)$

Case 1–1: ${M}_{j}\le KT\le {T}_{w}$

From Eq. (11), differentiating $AT{C}_{11}^{\left(j\right)}\left(K,T\right)$ with respect to $K\mathrm{and}T$, we have

$$\frac{\partial AT{C}_{11}^{\left(j\right)}\left(K,T\right)}{\partial K}=2{\varphi }_{111}KT-{\varphi }_{112}T-{\varphi }_{113}\to K=\frac{{\varphi }_{112}T + {\varphi }_{113}}{2{\varphi }_{111}T}$$

(66)

$$\frac{\partial AT{C}_{11}^{\left(j\right)}\left(K,T\right)}{\partial T}={\varphi }_{111}{K}^{2}-{\varphi }_{112}K+{\varphi }_{114}-\frac{{\varphi }_{115}}{{T}^{2}}\to {T}^{2}=\frac{{\varphi }_{115}}{{\varphi }_{111}{K}^{2}-{\varphi }_{112}K+{\varphi }_{114}}$$

(67)

After some algebra

$${T}_{11}=\sqrt{\frac{4{\varphi }_{111}{\varphi }_{115}-{\varphi }_{113}^{2}}{4{\varphi }_{111}{\varphi }_{114}-{\varphi }_{112}^{2}}}$$

(68)

and

$${K}_{11}=\frac{{\varphi }_{112}}{2{\varphi }_{111}}+\frac{{\varphi }_{113}}{2{\varphi }_{111}}\sqrt{\frac{4{\varphi }_{111}{\varphi }_{114}-{\varphi }_{112}^{2}}{4{\varphi }_{111}{\varphi }_{115}-{\varphi }_{113}^{2}}}$$

(69)

Similarly, for Case 1–2, Case 2–1 and Case 2–2, the roots $\left({K}_{12}, {T}_{12}\right)$, $\left({K}_{21}, {T}_{21}\right)$, and $\left({K}_{22}, {T}_{22}\right)$ can be obtained easily.

Appendix 4 Find the optimal values of T ^# when K = 1

Case 1–1:${M}_{j}\le KT\le {T}_{w}$

Substituting ${K}_{11}=1$ into Eq. (10) leads to

$$AT{C}_{11}^{\left(j\right)}\left(1,T\right)={\varphi }_{111}T-{\varphi }_{112}T-{\varphi }_{113}+{\varphi }_{114}T+\frac{{\varphi }_{115}}{T}+{\varphi }_{116}$$

(70)

Taking the first and second derivatives of Eq. (70) with respect to $T$, we have

$$\frac{dAT{C}_{11}^{\left(j\right)}\left(1,T\right)}{dT}={\varphi }_{111}-{\varphi }_{112}+{\varphi }_{114}-\frac{{\varphi }_{115}}{{T}^{2}}$$

(71)

$$\frac{{d}^{2}AT{C}_{11}^{\left(j\right)}\left(1,T\right)}{{dT}^{2}}=\frac{2{\varphi }_{115}}{{T}^{3}}$$

(72)

Obviously, if ${\varphi }_{115}>0,$ then $AT{C}_{11}^{\left(j\right)}\left(1,T\right)$ is a strictly convex function of $T$. Setting $AT{C}_{11}^{{^{\prime}}\left(j\right)}\left(1,T\right)=0$ yields

$${T}_{11}=\sqrt{\frac{{\varphi }_{115}}{{\varphi }_{111}-{\varphi }_{112}+{\varphi }_{114}}}$$

(73)

Similarly, for the other cases, ${T}$ can be obtained when ${K}_{12}=1$, ${K}_{21}=1$, and ${K}_{22}=1$,

$${T}_{12}=\sqrt{\frac{{\varphi }_{125}}{{\varphi }_{121}-{\varphi }_{122}+{\varphi }_{124}}}$$

(74)

$${T}_{21}=\sqrt{\frac{{\varphi }_{215}}{{\varphi }_{211}-{\varphi }_{212}+{\varphi }_{214}}}$$

(75)

$${T}_{11}=\sqrt{\frac{{\varphi }_{225}}{{\varphi }_{221}-{\varphi }_{222}+{\varphi }_{224}}}$$

(76)

Appendix 5 Find the optimal values of T' and K'

For the solution of ${K}_{11}$ and ${T}_{11}$ derived for Case 1–1, if the relationship ${K}_{11}{T}_{11}<{M}_{j}$ is established, it shows that the optimal values will be obtained on the boundary point. Thus, we may set $T=\frac{{M}_{j}}{{K}_{11}}$ and then substitute it into Eq. (11), which leads to

$$AT{C}_{11}^{\left(j\right)}\left({K}_{11},\frac{{M}_{j}}{{K}_{11}}\right)={\varphi }_{111}{{K}_{11}}^{2}\frac{{M}_{j}}{{K}_{11}}-{\varphi }_{112}{K}_{11}\frac{{M}_{j}}{{K}_{11}}-{\varphi }_{113}{K}_{11}+{\varphi }_{114}\frac{{M}_{j}}{{K}_{11}}+\frac{{\varphi }_{115}{K}_{11}}{{M}_{j}}+{\varphi }_{116}$$

(77)

Taking the first and second derivatives of Eq. (77) with respect to ${K}_{11}$, we have

$$\frac{dAT{C}_{11}^{\left(j\right)}\left({K}_{11},\frac{{M}_{j}}{{K}_{11}}\right)}{d{K}_{11}}={\varphi }_{111}{M}_{j}-{\varphi }_{113}-{\varphi }_{114}\frac{{M}_{j}}{{{K}_{11}}^{2}}+\frac{{\varphi }_{115}}{{M}_{j}}$$

(78)

$$\frac{{d}^{2}AT{C}_{11}^{\left(j\right)}\left({K}_{11},\frac{{M}_{j}}{{K}_{11}}\right)}{{d{K}_{11}}^{2}}=\frac{2{\varphi }_{114}{M}_{j}}{{{K}_{11}}^{3}}>0$$

(79)

From Eq. (79), $AT{C}_{11}^{\left(j\right)}\left({K}_{11},\frac{{M}_{j}}{{K}_{11}}\right)$ is a strictly convex function of ${K}_{11}$. Setting $AT{C}_{11}^{{^{\prime}}\left(j\right)}\left({K}_{11},\frac{{M}_{j}}{{K}_{11}}\right)=0$ yields

$${K{^{\prime}}}_{11}=\sqrt{\frac{{\varphi }_{114}{{M}_{j}}^{2}}{{\varphi }_{111}{{M}_{j}}^{2}-{\varphi }_{113}{M}_{j}+{\varphi }_{115}}}$$

(80)

Noticing that, if ${K{^{\prime}}}_{11}$ is feasible, the optimal solution in this case is $\left({T}_{11}, {K}_{11}\right)=\left(\frac{{M}_{j}}{{K{^{\prime}}}_{11}}, {K{^{\prime}}}_{11}\right),$ Otherwise the optimal solution is $\left({T}_{11}, {K}_{11}\right)=\left({M}_{j},1\right).$

In addition, if the relationship ${K}_{11}{T}_{11}>{T}_{w}$ is established, use the same approach to develop${K{^{\prime}}}_{11}$, ${K{^{\prime}}}_{11}=\sqrt{\frac{{\varphi }_{114}{{T}_{w}}^{2}}{{\varphi }_{111}{{T}_{w}}^{2}-{\varphi }_{113}{T}_{w}+{\varphi }_{115}}}$.

In the same way, we can analyze Case 1–2, Case 2–1, Case 2–2. The specific computational results are summarized in Table 2.

Appendix 6 Find the optimal values of T'' and K'' when optimal order quantity ${Q}_{j}\notin [{q}_{j},{q}_{j+1})$

If ${Q}_{j}\notin [{q}_{j},{q}_{j+1})$, there are two situations:

• 1. if ${Q}_{j}\ge {q}_{j+1},$ the optimal solution does not exist and then the retailer needs to adjust the order quantity;

• 2. if ${Q}_{j}<{q}_{j}$, the optimal values will be obtained at point $T=\frac{{q}_{j}}{D\left[\left(1-\beta \right)K+\beta \right]}$.

Based on the analysis above, we only need to discuss the case of ${Q}_{j}<{q}_{j}$.

First, for Case 1–1, substituting $T=\frac{{q}_{j}}{D\left[\left(1-K\right)\beta +K\right]}=\frac{{q}_{j}}{D\left[\left(1-\beta \right)K+\beta \right]}$ into Eq. (52) leads to

$$\begin{array}{l}AT{C}_{11}^{\left(j\right)}\left(K\right)=\frac{\left[2\left(A+\tau {A}^{^{\prime}}\right)D+\left\{{c}_{j}{I}_{c}-p{I}_{e}\right\}{D}^{2}{{M}_{j}}^{2}\right]\left[\left(1-\beta \right)K+\beta \right]}{2{q}_{j}}+{(c}_{j}+\tau c{^{\prime}})D+\pi D\left(1-K\right)\left(1-\beta \right)\\\qquad\qquad\qquad -\left(1-K\right)p{I}_{e}\beta {M}_{j}D-{c}_{j}{I}_{c}D{M}_{j}K+\frac{{c}_{b}{q}_{j}\beta {\left(1-K\right)}^{2}}{2\left[\left(1-\beta \right)K+\beta \right]}+\frac{\left\{{h}_{0}+\tau {h}_{0}^{^{\prime}}+{c}_{j}{I}_{c}\right\}{q}_{j}{K}^{2}}{2\left[\left(1-\beta \right)K+\beta \right]}\end{array}$$

(81)

Taking the first and second derivatives of Eq. (81) with respect to K, we have

$$\begin{array}{c}\frac{dAT{C}_{11}^{\left(j\right)}\left(K\right)}{dK}=\frac{\left[2\left(A+\tau {A}^{^{\prime}}\right)D+\left\{{c}_{j}{I}_{c}-p{I}_{e}\right\}{D}^{2}{{M}_{j}}^{2}\right]\left(1-\beta \right)}{2{q}_{j}}-K\left(1-\beta \right)+p{I}_{e}\beta {M}_{j}D-{c}_{j}{I}_{c}D{M}_{j}\\ -\frac{{c}_{b}{q}_{j}\beta \left[\left(1-K\right)\left(1+K\right)\left(1-\beta \right)+2\left(1-K\right)\beta \right]}{2{\left[\left(1-\beta \right)K+\beta \right]}^{2}}+\frac{\left\{{h}_{0}+\tau {h}_{0}^{^{\prime}}{+c}_{j}{I}_{c}\right\}{q}_{j}\left[{\left(1-\beta \right)K}^{2}+2K\beta \right]}{2{\left[\left(1-\beta \right)K+\beta \right]}^{2}}\end{array}$$

(82)

$$\begin{array}{c}\frac{{d}^{2}AT{P}_{11}^{\left(j\right)}\left(K\right)}{{dK}^{2}}={c}_{b}{q}_{j}\beta \left[\frac{1}{\left(1-\beta \right)K+\beta }+\frac{\left(1-\beta \right)\left\{\left(1-K\right)\left(1+K\right)\left(1-\beta \right)+2\left(1-K\right)\beta \right\}}{{\left[\left(1-\beta \right)K+\beta \right]}^{3}}\right]\\ +\frac{\left\{{h}_{0}+\tau {h}_{0}^{^{\prime}}{+c}_{j}{I}_{c}\right\}{q}_{j}{\beta }^{2}}{{\left[\left(1-\beta \right)K+\beta \right]}^{3}}>0.\end{array}$$

(83)

From Eq. (83), we know that $AT{C}_{11}^{\left(j\right)}\left(K\right)$ is convex. Setting $dAT{C}_{11}^{\left(j\right)}\left(K\right)/dK=0$ yields

$$\begin{array}{c}\frac{\left[2\left(A+\tau {A}^{^{\prime}}\right)D+\left\{{c}_{j}{I}_{c}-p{I}_{e}\right\}{D}^{2}{{M}_{j}}^{2}\right]\left(1-\beta \right)}{2{q}_{j}}-K\left(1-\beta \right)+p{I}_{e}\beta {M}_{j}D-{c}_{j}{I}_{c}D{M}_{j}\\ -\frac{{c}_{b}{q}_{j}\beta \left[\left(1-K\right)\left(1+K\right)\left(1-\beta \right)+2\left(1-K\right)\beta \right]}{2{\left[\left(1-\beta \right)K+\beta \right]}^{2}}+\frac{\left\{{h}_{0}+\tau {h}_{0}^{^{\prime}}{+c}_{j}{I}_{c}\right\}{q}_{j}\left[{\left(1-\beta \right)K}^{2}+2K\beta \right]}{2{\left[\left(1-\beta \right)K+\beta \right]}^{2}}=0\end{array}$$

(84)

After some transformation, the Eq. (84) can be simplified to

$${\mu }_{111}{K}^{2}+{\mu }_{112}K+{\mu }_{113}=0,$$

(85)

where,

$${\mu }_{111}=2{\left(1-\beta \right)}^{2}{\omega }_{111}+\left({h}_{0}+\tau {h}_{0}^{^{\prime}}+{c}_{b}\beta +{c}_{j}{I}_{c}\right){q}_{j}\left(1-\beta \right)$$

(86)

$${\mu }_{112}={4\beta \left(1-\beta \right)\omega }_{111}+2{c}_{b}{q}_{j}{\beta }^{2}+2\left({h}_{0}+\tau {h}_{0}^{^{\prime}}+{c}_{j}{I}_{c}\right){q}_{j}\beta$$

(87)

$${\mu }_{113}=2{\omega }_{111}{\beta }^{2}-{c}_{b}\beta {q}_{j}\left(1+\beta \right)$$

(88)

$${\omega }_{111}=\frac{D\left(1-\beta \right)}{{q}_{j}}\left\{A+\tau A{^{\prime}}+\frac{\left({c}_{j}{I}_{c}-p{I}_{e}\right)D{{M}_{j}}^{2}}{2}\right\}-\left[{\pi }_{j}D\left(1-\beta \right)+\left\{{c}_{j}{I}_{c}-p\beta {I}_{e}\right\}D{M}_{j}\right]$$

(89)

For the quadratic equation (F5), if it has roots $\left(\mathrm{i}.\mathrm{e}., \Delta ={\mu }_{112}-4{\mu }_{111}{\mu }_{113}\ge 0\right)$, then we have

$${{K}^{^{\prime}}}_{11}=max\left\{\frac{-{\mu }_{112}+\sqrt{{\mu }_{112}^{2}-4{\mu }_{111}{\mu }_{113}}}{2{\mu }_{111}},\frac{-{\mu }_{112}-\sqrt{{\mu }_{112}^{2}-4{\mu }_{111}{\mu }_{113}}}{2{\mu }_{111}}\right\}$$

(90)

If ${{K}^{^{\prime}}}_{11}$ is feasible, then we obtain the retailer’s replenishment cycle

$${{T}^{^{\prime}}}_{11}=\frac{{q}_{j}}{D\left[\left(1-\beta \right){K{^{\prime}}{^{\prime}}}_{11}+\beta \right]}$$

(91)

If ${{K}^{^{\prime}}}_{11}$ is not feasible or Eq. (85) has no root, we may set ${K{^{\prime}}{^{\prime}}}_{11}=0$ or ${K{^{\prime}}{^{\prime}}}_{11}=1$.

In summary, for the solution of ${K{^{\prime}}{^{\prime}}}_{11}$ and ${T{^{\prime}}{^{\prime}}}_{11}$ derived for Case 1–1, we also need to check whether the constraint ${M}_{j}\le {K{^{\prime}}{^{\prime}}}_{11}{T{^{\prime}}{^{\prime}}}_{11}\le {T}_{w}$ is satisfied. If the constraint is valid, the optimal solution is obtained. Otherwise, the optimal solution does not exist.

Following the same steps used in Case 1–1, we can analyze Case 1–2, Case 2–1 and Case 2–2 separately.

$${{K}^{^{\prime}}}_{12}=max\left\{\frac{-{\mu }_{122}+\sqrt{{\mu }_{122}^{2}-4{\mu }_{121}{\mu }_{123}}}{2{\mu }_{121}},\frac{-{\mu }_{122}-\sqrt{{\mu }_{122}^{2}-4{\mu }_{121}{\mu }_{123}}}{2{\mu }_{121}}\right\}$$

(92)

where,

$${\mu }_{121}=2{\left(1-\beta \right)}^{2}{\omega }_{121}+{q}_{j}\left(1-\beta \right)\left({h}_{0}+\tau {h}_{0}^{^{\prime}}+{c}_{b}\beta +p{I}_{e}\right)$$

(93)

$${\mu }_{122}={4\beta \left(1-\beta \right)\omega }_{121}+2{{c}_{b}q}_{j}{\beta }^{2}+2\left({h}_{0}+\tau {h}_{0}^{^{\prime}}+p{I}_{e}\right)\beta {q}_{j}$$

(94)

$${\mu }_{123}=2{\omega }_{121}{\beta }^{2}-{{c}_{b}\beta q}_{j}\left(1+\beta \right)$$

(95)

$${\omega }_{121}=\frac{D\left(1-\beta \right)}{{2q}_{j}}\left\{A+\tau A{^{\prime}}\right\}-\left[{\pi }_{j}D\left(1-\beta \right)+\left(1-\beta \right) p{I}_{e}{M}_{j}D\right]$$

(96)

$${{K}^{^{\prime}}}_{21}=max\left\{\frac{-{\mu }_{212}+\sqrt{{\mu }_{212}^{2}-4{\mu }_{211}{\mu }_{213}}}{2{\mu }_{211}},\frac{-{\mu }_{212}-\sqrt{{\mu }_{212}^{2}-4{\mu }_{211}{\mu }_{213}}}{2{\mu }_{211}}\right\}$$

(97)

where,

$${\mu }_{211}=2{\left(1-\beta \right)}^{2}{\omega }_{211}+{q}_{j}\left(1-\beta \right)\left({h}_{r}+\tau {h}_{r}^{^{\prime}}+{c}_{b}\beta +{c}_{j}{I}_{c}\right)$$

(98)

$${\mu }_{212}={4\beta \left(1-\beta \right)\omega }_{211}+2{q}_{j}{c}_{b}{\beta }^{2}+2\left({h}_{r}+\tau {h}_{r}^{^{\prime}}+{c}_{j}{I}_{c}\right)\beta {q}_{j}$$

(99)

$${\mu }_{213}=2{\omega }_{211}{\beta }^{2}-{c}_{b}\beta {q}_{j}\left(1+\beta \right)$$

(100)

$$\begin{array}{l}{\omega }_{211}=\frac{\left(A+A{^{\prime}}\right)D\left(1-\beta \right)}{{q}_{j}}-{\pi }_{j}D\left(1-\beta \right)+\frac{\left({h}_{r}+\tau {h}_{r}^{^{\prime}}-{h}_{0}-\tau {h}_{0}^{^{\prime}}\right){W}^{2}\left(1-\beta \right)}{2{q}_{j}}-\left({h}_{r}+\tau {h}_{r}^{^{\prime}}-{h}_{0}-\tau {h}_{0}^{^{\prime}}\right)W\\\qquad\quad -\left({c}_{j}{I}_{c}-p\beta {I}_{e}\right)D{M}_{j}+\frac{\left({c}_{j}{I}_{c}-p{I}_{e}\right){D}^{2}{{M}_{j}}^{2}\left(1-\beta \right)}{2{q}_{j}}\end{array}$$

(101)

$${{K}^{^{\prime}}}_{22}=max\left\{\frac{-{\mu }_{222}+\sqrt{{\mu }_{222}^{2}-4{\mu }_{221}{\mu }_{223}}}{2{\mu }_{221}},\frac{-{\mu }_{222}-\sqrt{{\mu }_{222}^{2}-4{\mu }_{221}{\mu }_{223}}}{2{\mu }_{221}}\right\}$$

(102)

where,

$${\mu }_{221}=2{\left(1-\beta \right)}^{2}{\omega }_{221}+{q}_{j}\left(1-\beta \right)\left({h}_{r}+\tau {h}_{r}^{^{\prime}}+{c}_{b}\beta +p{I}_{e}\right)$$

(103)

$${\mu }_{222}={4\beta \left(1-\beta \right)\omega }_{221}+2{c}_{b}{q}_{j}{\beta }^{2}+2\left({h}_{r}+\tau {h}_{r}^{^{\prime}}+p{I}_{e}\right){q}_{j}\beta$$

(104)

$${\mu }_{223}=2{\omega }_{221}{\beta }^{2}-{{c}_{b}\beta q}_{j}\left(1+\beta \right)$$

(105)

$$\begin{array}{l}{\omega }_{221}=\frac{D\left(1-\beta \right)}{{q}_{j}}\left\{A+{A}^{^{\prime}}+\frac{\left({h}_{r}+\tau {h}_{r}^{^{\prime}}-{h}_{0}-\tau {h}_{0}^{^{\prime}}\right){W}^{2}}{2D}\right\}\\\qquad\quad -\left\{{\pi }_{j}D\left(1-\beta \right)+\left({h}_{r}+\tau {h}_{r}^{^{\prime}}-{h}_{0}-\tau {h}_{0}^{^{\prime}}\right)W+\left(1-\beta \right)p{I}_{e}{M}_{j}D\right\}\end{array}$$

(106)

Appendix 7

Algorithm A: Determine (${K}_{11}^{**},{T}_{11}^{**}$) and ${ATP}_{11}^{(j)}\left({K}_{11}^{**}, {T}_{11}^{**}\right)$

• A1. Calculate ${\varphi }_{11i}\left(i=\mathrm{1,2},\dots ,6\right)$ from Eqs.(14)-(19). If ${\varphi }_{115}>0,$ go to step A2; if not, go to step A6.

• A2. Calculate ${\beta }_{11}$ from Eq. (28), if ${\beta \le \beta }_{11}$, go to step A4; else if ${\beta >\beta }_{11},$ calculate ${T}_{11}$ from Eq. (29). If ${T}_{11}$ is feasible, go to step A3; if not, go to step A4.

• A3. Compute ${K}_{11}$ from Eq. (30), if ${K}_{11}\le 1,$ go to step A5; if not, go to step A4.

• A4. Set ${K}_{11}=1,$ determine ${T}_{11}$ from Eq.(D4) in Appendix 4. If ${T}_{11}>{T}_{w}$, set $\left({K}_{11}^{*},{T}_{11}^{*}\right)=(1,{T}_{w})$ and go to step A7; else if ${T}_{11}<{M}_{j}$, set $\left({K}_{11}^{*},{T}_{11}^{*}\right)=(1,{M}_{j})$ and go to step A7; otherwise, set $\left({K}_{11}^{*},{T}_{11}^{*}\right)=(1,{T}_{11})$ and go to step A7.

• A5. If ${M}_{j}\le {K}_{11}{T}_{11}\le {T}_{w}$, set $\left({K}_{11}^{*},{T}_{11}^{*}\right)=({K}_{11},{T}_{11})$ and go to step A7; if not, go to step A6.

• A6. If ${K}_{11}{T}_{11}>{T}_{w},$ obtain $\left({K}_{11}^{*},{T}_{11}^{*}\right)=\left({K}_{11}^{^{\prime}},{T}_{11}^{^{\prime}}\right)$ by employing Table 2. Then if ${T}_{11}^{*}$ and ${K}_{11}^{*}$ are feasible, go to step A7; if not, go to step A4. On the other hand, if ${K}_{11}{T}_{11}<{M}_{j},$ obtain $\left({K}_{11}^{*},{T}_{11}^{*}\right)=\left({K}_{11}^{^{\prime}},{T}_{11}^{^{\prime}}\right)$ using Table 2. Now, if ${T}_{11}^{*}$ and ${K}_{11}^{*}$ are feasible, go to step A7; if not, go to step A4.

• A7. Calculate order quantity ${Q}_{j}=D{T}_{12}^{*}\left[\left(1-{K}_{12}^{*}\right)\beta +{K}_{12}^{*}\right]$ from Eq. (31), and go to step A8.

• A8. Determine the relationship between ${Q}_{j}$ and $\left[{q}_{j},\left.{q}_{j+1}\right)\right.$ using the following sub-steps.

• A8.1 If ${q}_{j}\le {Q}_{j}<{q}_{j+1},$ set $\left({K}_{11}^{**},{T}_{11}^{**}\right)=\left({K}_{11}^{*},{T}_{11}^{*}\right).$ Calculate the retailer’s annual profit ${ATP}_{11}^{(j)}\left({K}_{11}^{**}, {T}_{11}^{**}\right)$ using Eq. (7) and go to step A9.

• A8.2 If ${Q}_{j}\ge {q}_{j+1},$ then ${T}_{11}^{*}$ and ${K}_{11}^{*}$ are not feasible solutions, set ${ATP}_{11}^{(j)}\left(K,T\right)=-\mathrm{inf}.$

• A8.3 If ${Q}_{j}<{q}_{j},$ then ${T}_{11}^{*}$ and ${K}_{11}^{*}$ are not feasible solutions. However, ${ATP}_{11}^{(j)}\left(K,T\right)$ at point $T=\frac{{q}_{j}}{D\left[\left(1-\beta \right)K+\beta \right]}$ has a maximum value. Thus, calculate ${K}_{11}^{{^{\prime}}{^{\prime}}}$ from Eq. (86) in Appendix 6. If ${K}_{11}^{{^{\prime}}{^{\prime}}}$ is feasible, go to step A8.3.1; if not, go to step A8.3.2.

• A8.3.1 If ${M}_{j}\le {K{^{\prime}}{^{\prime}}}_{11}{T{^{\prime}}{^{\prime}}}_{11}\le {T}_{w}$, set $\left({K}_{11}^{**},{T}_{11}^{**}\right)=\left({K}_{11}^{{^{\prime}}{^{\prime}}},{T}_{11}^{{^{\prime}}{^{\prime}}}\right),$ and calculate the retailer’s annual profit ${ATP}_{11}^{(j)}\left({K}_{11}^{**}, {T}_{11}^{**}\right)$ using Eq. (7), go to step A9. Otherwise,${T}_{11}^{{^{\prime}}{^{\prime}}}$ and ${K}_{11}^{{^{\prime}}{^{\prime}}}$ are not feasible solutions, set ${ATP}_{11}^{(j)}\left(K,T\right)=-\mathrm{inf},$ go to step A9.

• A8.3.2 Let ${K}_{11}^{\mathrm{^{\prime}}\mathrm{^{\prime}}}=1$ and ${T}_{11}^{\mathrm{^{\prime}}\mathrm{^{\prime}}}={q}_{j}/D.$ If ${M}_{j}\le {K\mathrm{^{\prime}}\mathrm{^{\prime}}}_{11}{T\mathrm{^{\prime}}\mathrm{^{\prime}}}_{11}\le {T}_{w},$ set $\left({K}_{11}^{**},{T}_{11}^{**}\right)=(1,{q}_{j}/D),$ and calculate the retailer’s annual profit ${ATP}_{11}^{(j)}\left({K}_{11}^{**}, {T}_{11}^{**}\right)$ using Eq. (7) and go to step A9.

  Otherwise, ${T}_{11}^{^{\prime}}$ and ${K}_{11}^{^{\prime}}$ are not feasible solutions, set ${ATP}_{11}^{(j)}\left(K,T\right)=-\mathrm{inf},$ go to step A9.

• A9. If ${ATP}_{11}^{(j)}\left({K}_{11}^{**}, {T}_{11}^{**}\right)\ge -{\pi }_{j}D,$ the optimal solutions ${K}_{11}^{**}$ and ${T}_{11}^{**}$ are found and stop. Otherwise, go to step A9.

• A10. Set $\left({K}_{11}^{**},{T}_{11}^{**}\right)=\left(0,\infty \right),{ATP}_{11}^{(j)}\left({K}_{11}^{**}, {T}_{11}^{**}\right)=-{\uppi }_{j}D.$

Appendix 8

Algorithm B: Determine (${K}_{12}^{**},{T}_{12}^{**}$) and ${ATP}_{12}^{(j)}\left({K}_{12}^{**}, {T}_{12}^{**}\right)$

• B1. Calculate ${\varphi }_{12i} \left(i=\mathrm{1,2},\dots ,6\right)$ from Eqs. (35)-(40), go to step B2.

• B2. Calculate ${\beta }_{12}$ from Eq. (42), if ${\beta \le \beta }_{12}$, go to step B4; else if ${\beta >\beta }_{12}$, calculate ${T}_{12}$ from Eq. (43). If ${T}_{12}$ is feasible, go to step B3; if not, go to step B4.

• B3. Compute ${K}_{12}$ from Eq. (44), if ${K}_{12}\le 1,$ go to step B5; if not, go to step B4.

• B4. Set ${K}_{12}=1,$ determine ${T}_{12}$ from Eq.(D5) in Appendix 4. If ${T}_{12}>\mathrm{min}\{{T}_{w},{M}_{j}\}$, set $\left({K}_{12}^{*},{T}_{12}^{*}\right)=(1,\mathrm{min}\{{T}_{w},{M}_{j}\})$ and go to step B7; otherwise, set $\left({K}_{12}^{*},{T}_{12}^{*}\right)=(1,{T}_{12})$ and go to step B7.

• B5. If ${K}_{12}{T}_{12}\le \mathrm{min}\{{T}_{w},{M}_{j}\}$, set $\left({K}_{12}^{*},{T}_{12}^{*}\right)=({K}_{12},{T}_{12})$ and go to step B7; if not, go to step B6.

• B6. If If ${K}_{12}{T}_{12}>\mathrm{min}\{{T}_{w},{M}_{j}\},$ obtain $\left({K}_{12}^{*},{T}_{12}^{*}\right)=\left({K}_{12}^{^{\prime}},{T}_{12}^{^{\prime}}\right)$ by employing Table 2.Then if ${T}_{12}^{*}$ and ${K}_{12}^{*}$ are feasible, go to step B7; if not, go to step B4.

• B7. Calculate order quantity ${Q}_{j}=D{T}_{12}^{*}\left[\left(1-{K}_{12}^{*}\right)\beta +{K}_{12}^{*}\right]$, and go to step B8.

• B8. Determine the relationship between ${Q}_{j}$ and $\left[{q}_{j},\left.{q}_{j+1}\right)\right.$ using the following sub-steps.

• B8.1 If ${q}_{j}\le {Q}_{j}<{q}_{j+1},$ set $\left({K}_{12}^{**},{T}_{12}^{**}\right)=\left({K}_{12}^{*},{T}_{12}^{*}\right).$ Calculate the retailer’s annual profit ${ATP}_{12}^{(j)}\left({K}_{12}^{**}, {T}_{12}^{**}\right)$ using Eq. (8) and go to step B9.

• B8.2 If ${Q}_{j}\ge {q}_{j+1},$ then ${T}_{12}^{*}$ and ${K}_{12}^{*}$ are not feasible solutions, set ${ATP}_{12}^{(j)}\left({K}_{12}^{*},{T}_{12}^{*}\right)=-\mathrm{inf}.$

• B8.3 If ${Q}_{j}<{q}_{j},$ then ${T}_{12}^{*}$ and ${K}_{12}^{*}$ are not feasible solutions. However, ${ATP}_{12}^{(j)}\left(K,T\right)$ at point $T=\frac{{q}_{j}}{D\left[\left(1-\beta \right)K+\beta \right]}$ has a maximum value. Thus, calculate ${K}_{12}^{{^{\prime}}{^{\prime}}}$ from Eq. (88) in Appendix 6. If ${K}_{12}^{{^{\prime}}{^{\prime}}}$ is feasible, go to step B8.3.1; if not, go to step B8.3.2.

• B8.3.1 If ${{K}^{^{\prime}}}_{12}{{T}^{^{\prime}}}_{12}\le \mathrm{min}\{{T}_{w},{M}_{j}\}$, set $\left({K}_{12}^{**},{T}_{12}^{**}\right)=\left({K}_{12}^{{^{\prime}}{^{\prime}}},{T}_{12}^{{^{\prime}}{^{\prime}}}\right),$ and calculate the retailer’s annual profit ${ATP}_{12}^{(j)}\left({K}_{12}^{**}, {T}_{12}^{**}\right)$ using Eq. (8), go to step B9. Otherwise,${T}_{12}^{{^{\prime}}{^{\prime}}}$ and ${K}_{12}^{{^{\prime}}{^{\prime}}}$ are not feasible solutions, set ${ATP}_{12}^{(j)}\left({K}_{12}^{**},{T}_{12}^{**}\right)=-\mathrm{inf},$ go to step B9.

• B8.3.2 Let ${K}_{12}^{\mathrm{^{\prime}}\mathrm{^{\prime}}}=1$ and ${T}_{12}^{\mathrm{^{\prime}}\mathrm{^{\prime}}}={q}_{j}/D.$ If ${{K}_{12}^{\mathrm{^{\prime}}\mathrm{^{\prime}}}T\mathrm{^{\prime}}\mathrm{^{\prime}}}_{12}\le \mathrm{min}\{{T}_{w},{M}_{j}\},$ set $\left({K}_{12}^{**},{T}_{12}^{**}\right)=(1,{q}_{j}/D),$ and calculate the retailer’s annual profit ${ATP}_{12}^{(j)}\left({K}_{12}^{**}, {T}_{12}^{**}\right)$ using Eq. (8) and go to step B9.

  Otherwise, ${T}_{11}^{{^{\prime}}{^{\prime}}}$ and ${K}_{11}^{{^{\prime}}{^{\prime}}}$ are not feasible solutions, set ${ATP}_{12}^{(j)}\left({K}_{12}^{**},{T}_{12}^{**}\right)=-\mathrm{inf},$ go to step B9.

• B9. If ${ATP}_{12}^{(j)}\left({K}_{12}^{**}, {T}_{12}^{**}\right)\ge -{\pi }_{j}D,$ the optimal solutions ${K}_{12}^{**}$ and ${T}_{12}^{**}$ are found and stop. Otherwise, go to step B10.

• B10. Set $\left({K}_{12}^{**},{T}_{12}^{**}\right)=\left(0,\infty \right),{ATP}_{12}^{(j)}\left({K}_{12}^{**}, {T}_{12}^{**}\right)=-{\uppi }_{j}D.$

Appendix 9

Algorithm C: Determine (${K}_{21}^{**},{T}_{21}^{**}$) and ${ATP}_{21}^{(j)}\left({K}_{21}^{**}, {T}_{21}^{**}\right)$

• C1. Calculate ${\varphi }_{21i}\left(i=\mathrm{1,2},\dots ,6\right)$ from Eqs.(48)-(53). If ${\varphi }_{215}>0,$ go to step C2; if not, go to step C6.

• C2. Calculate ${\beta }_{21}$ from Eq. (55), if ${\beta \le \beta }_{21}$, go to step C4; else if ${\beta >\beta }_{21}$, calculate ${T}_{21}$ from Eq. (56). If ${T}_{21}$ is feasible, go to step C3; if not, go to step C4.

• C3. Compute ${K}_{21}$ from Eq. (57), if ${K}_{21}\le 1,$ go to step C5; if not, go to step C4.

• C4. Set ${K}_{21}=1,$ determine ${T}_{21}$ from Eq.(D6) in Appendix 4. If ${T}_{21}<\mathrm{max}\{{T}_{w},{M}_{j}\}$, set $\left({K}_{21}^{*},{T}_{21}^{*}\right)=(1,\mathrm{max}\{{T}_{w},{M}_{j}\})$ and go to step C7. Otherwise, set $\left({K}_{21}^{*},{T}_{21}^{*}\right)=(1,{T}_{21})$ and go to step C7.

• C5. If $\mathrm{max}\{{T}_{w},{M}_{j}\}\le {K}_{21}{T}_{21}$, set $\left({K}_{21}^{*},{T}_{21}^{*}\right)=({K}_{21},{T}_{21})$ and go to step C7; if not, go to step C6.

• C6. If ${K}_{21}{T}_{21}<\mathrm{max}\{{T}_{w},{M}_{j}\},$ obtain $\left({K}_{21}^{*},{T}_{21}^{*}\right)=\left({K}_{21}^{^{\prime}},{T}_{21}^{^{\prime}}\right)$ by employing Table 2.Then if ${T}_{21}^{*}$ and ${K}_{21}^{*}$ are feasible, go to step C7; if not, go to step C4.

• C7. Calculate order quantity ${Q}_{j}=D{T}_{21}^{*}\left[\left(1-{K}_{21}^{*}\right)\beta +{K}_{21}^{*}\right]$, and go to step C8.

• C8. Determine the relationship between ${Q}_{j}$ and $\left[{q}_{j},\left.{q}_{j+1}\right)\right.$ using the following sub-steps.

• C8.1 If ${q}_{j}\le {Q}_{j}<{q}_{j+1},$ set $\left({K}_{21}^{**},{T}_{21}^{**}\right)=\left({K}_{21}^{*},{T}_{21}^{*}\right).$ Calculate the retailer’s annual profit ${ATP}_{21}^{(j)}\left({K}_{21}^{**}, {T}_{21}^{**}\right)$ using Eq. (9) and go to step C9.

• C8.2. If ${Q}_{j}\ge {q}_{j+1},$ then ${T}_{21}^{*}$ and ${K}_{21}^{*}$ are not feasible solutions, set ${ATP}_{21}^{(j)}\left({K}_{21}^{*},{T}_{21}^{*}\right)=-\mathrm{inf}.$

• C8.3. If ${Q}_{j}<{q}_{j},$ then ${T}_{21}^{*}$ and ${K}_{21}^{*}$ are not feasible solutions. However, ${ATP}_{21}^{(j)}\left(K,T\right)$ at point $T=\frac{{q}_{j}}{D\left[\left(1-\beta \right)K+\beta \right]}$ has a maximum value. Thus, calculate ${K}_{21}^{{^{\prime}}{^{\prime}}}$ from Eq. (89) in Appendix 6. If ${K}_{21}^{{^{\prime}}{^{\prime}}}$ is feasible, go to step C8.3.1; if not, go to step C8.3.2.

• C8.3.1 If $\mathrm{max}\{{T}_{w},{M}_{j}\}\le {{K}^{^{\prime}}}_{21}{{T}^{^{\prime}}}_{21}$, set $\left({K}_{21}^{**},{T}_{21}^{**}\right)=\left({K}_{21}^{{^{\prime}}{^{\prime}}},{T}_{21}^{{^{\prime}}{^{\prime}}}\right),$ and calculate the retailer’s annual profit ${ATP}_{21}^{(j)}\left({K}_{21}^{**}, {T}_{21}^{**}\right)$ using Eq. (9), go to step C9. Otherwise,${T}_{21}^{{^{\prime}}{^{\prime}}}$ and ${K}_{21}^{{^{\prime}}{^{\prime}}}$ are not feasible solutions, set ${ATP}_{21}^{(j)}\left({K}_{21}^{**},{T}_{21}^{**}\right)=-\mathrm{inf},$ go to step C9.

• C8.3.2 Let ${K}_{21}^{\mathrm{^{\prime}}\mathrm{^{\prime}}}=1$ and ${T}_{21}^{\mathrm{^{\prime}}\mathrm{^{\prime}}}={q}_{j}/D.$ If $\mathrm{max}\{{T}_{w},{M}_{j}\}\le {K\mathrm{^{\prime}}\mathrm{^{\prime}}}_{21}{T\mathrm{^{\prime}}\mathrm{^{\prime}}}_{21},$ set $\left({K}_{21}^{**},{T}_{21}^{**}\right)=(1,{q}_{j}/D),$ and calculate the retailer’s annual profit ${ATP}_{21}^{(j)}\left({K}_{21}^{**}, {T}_{21}^{**}\right)$ using Eq. (9) and go to step C9. Otherwise, ${T}_{21}^{\mathrm{^{\prime}}\mathrm{^{\prime}}}$ and ${K}_{21}^{\mathrm{^{\prime}}\mathrm{^{\prime}}}$ are not feasible solutions, set ${ATP}_{21}^{(j)}\left({K}_{21}^{**},{T}_{21}^{**}\right)=-\mathrm{inf},$ go to step C9.

• C9. If ${ATP}_{21}^{(j)}\left({K}_{21}^{**}, {T}_{21}^{**}\right)\ge -{\pi }_{j}D,$ the optimal solutions ${K}_{21}^{**}$ and ${T}_{21}^{**}$ are found and stop. Otherwise, go to step C10.

• C10. Set $\left({K}_{21}^{**}, {T}_{21}^{**}\right)=\left(0,\infty \right), {ATP}_{21}^{(j)}\left({K}_{21}^{**}, {T}_{21}^{**}\right)=-{\uppi }_{j}D.$

Appendix 10

Algorithm D: Determine (${K}_{22}^{**},{T}_{22}^{**}$) and ${ATP}_{22}^{(j)}\left({K}_{22}^{**}, {T}_{22}^{**}\right)$

• D1. Calculate ${\varphi }_{22i}\left(i=\mathrm{1,2},\dots ,6\right)$ from Eqs.(61)-(66), go to step D6.

• D2. Calculate ${\beta }_{22}$ from Eq. (68), if ${\beta \le \beta }_{22}$, go to step D4; else if ${\beta >\beta }_{22}$, calculate ${T}_{22}$ from Eq. (69). If ${T}_{22}$ is feasible, go to step D3; if not, go to step D4.

• D3. Compute ${K}_{22}$ from Eq. (70), if ${K}_{22}\le 1,$ go to step D5; if not, go to step D4.

• D4. Set ${K}_{22}=1,$ determine ${T}_{22}$ from Eq.(D7) in Appendix 4. If ${T}_{22}<{T}_{w},$ set $\left({K}_{22}^{*},{T}_{22}^{*}\right)=(1,{T}_{w})$ and go to step D7; else if ${T}_{22}>{M}_{j},$ set $\left({K}_{22}^{*},{T}_{22}^{*}\right)=(1,{M}_{j})$ and go to step D7; Otherwise, set $\left({K}_{22}^{*},{T}_{22}^{*}\right)=(1,{T}_{22})$ and go to step D7.

• D5. If ${{T}_{w}\le K}_{22}{T}_{22}\le {M}_{j},$ set $\left({K}_{22}^{*},{T}_{22}^{*}\right)=({K}_{22},{T}_{22})$ and go to step D7; if not, go to step D6.

• D6. If If ${K}_{22}{T}_{22}<{T}_{w},$ obtain $\left({K}_{22}^{*},{T}_{22}^{*}\right)=\left({K}_{22}^{^{\prime}},{T}_{22}^{^{\prime}}\right)$ by employing Table 2.Then if ${T}_{22}^{*}$ and ${K}_{22}^{*}$ are feasible, go to step D7; if not, go to step D4. On the other hand, if ${K}_{22}{T}_{22}>{M}_{j}$, obtain $\left({K}_{22}^{*},{T}_{22}^{*}\right)=\left({K}_{22}^{^{\prime}},{T}_{22}^{^{\prime}}\right)$ using Table 2. Now, if ${T}_{22}^{*}$ and ${K}_{22}^{*}$ are feasible, go to step D7; if not, go to step D4.

• D7. Calculate order quantity ${Q}_{j}=D{T}_{22}^{*}\left[\left(1-{K}_{22}^{*}\right)\beta +{K}_{22}^{*}\right]$, and go to step D8.

• D8. Determine the relationship between ${Q}_{j}$ and $\left[{q}_{j},\left.{q}_{j+1}\right)\right.$ using the following sub-steps.

• D8.1 If ${q}_{j}\le {Q}_{j}<{q}_{j+1},$ set $\left({K}_{22}^{**},{T}_{22}^{**}\right)=\left({K}_{22}^{*},{T}_{22}^{*}\right).$ Calculate the retailer’s annual profit ${ATP}_{22}^{(j)}\left({K}_{22}^{**}, {T}_{22}^{**}\right)$ using Eq. (10) and go to step D9.

• D8.2 If ${Q}_{j}\ge {q}_{j+1},$ then ${T}_{22}^{*}$ and ${K}_{22}^{*}$ are not feasible solutions, set ${ATP}_{22}^{(j)}\left({K}_{22}^{*},{T}_{22}^{*}\right)=-\mathrm{inf}.$

• D8.3 If ${Q}_{j}<{q}_{j},$ then ${T}_{22}^{*}$ and ${K}_{22}^{*}$ are not feasible solutions. However, ${ATP}_{22}^{(j)}\left({K}_{22}^{*},{T}_{22}^{*}\right)$ at point $T=\frac{{q}_{j}}{D\left[\left(1-\beta \right)K+\beta \right]}$ has a maximum value. Thus, calculate ${K}_{22}^{{^{\prime}}{^{\prime}}}$ from Eq. (90) in Appendix 6. If ${K}_{22}^{{^{\prime}}{^{\prime}}}$ is feasible, go to step D8.3.1; if not, go to step D8.3.2.

• D8.3.1 If ${T}_{w}{\le {K}^{^{\prime}}}_{22}{{T}^{^{\prime}}}_{22}\le {M}_{j}$, set $\left({K}_{22}^{**},{T}_{22}^{**}\right)=\left({K}_{22}^{{^{\prime}}{^{\prime}}},{T}_{22}^{{^{\prime}}{^{\prime}}}\right),$ and calculate the retailer’s annual profit ${ATP}_{22}^{(j)}\left({K}_{22}^{**}, {T}_{22}^{**}\right)$ using Eq. (10), go to step D9. Otherwise,${T}_{22}^{^{\prime}}$ and ${K}_{22}^{^{\prime}}$ are not feasible solutions, set ${ATP}_{22}^{(j)}\left({K}_{22}^{**},{T}_{22}^{**}\right)=-\mathrm{inf},$ go to step D9.

• D8.3.2 Let ${K}_{22}^{\mathrm{^{\prime}}}=1$ and ${T}_{22}^{\mathrm{^{\prime}}}={q}_{j}/D.$ If ${T}_{w}{\le {K}^{\mathrm{^{\prime}}}}_{22}{{T}^{\mathrm{^{\prime}}}}_{22}\le {M}_{j},$ set $\left({K}_{22}^{**},{T}_{22}^{**}\right)=(1,{q}_{j}/D),$ and calculate the retailer’s annual profit ${ATP}_{22}^{(j)}\left({K}_{22}^{**}, {T}_{22}^{**}\right)$ using Eq. (10) and go to step D9.

  Otherwise, ${T}_{22}^{^{\prime}}$ and ${K}_{22}^{^{\prime}}$ are not feasible solutions, set ${ATP}_{22}^{(j)}\left({K}_{22}^{**},{T}_{22}^{**}\right)=-\mathrm{inf},$ go to step D9.

• D9. If ${ATP}_{22}^{(j)}\left({K}_{22}^{**}, {T}_{22}^{**}\right)\ge {-\pi }_{j}D,$ the optimal solutions ${K}_{22}^{**}$ and ${T}_{22}^{**}$ are found and stop. Otherwise, go to step D10.

• D10. Set $\left({K}_{22}^{**},{T}_{22}^{**}\right)=\left(0,\infty \right),{ATP}_{22}^{(j)}\left({K}_{22}^{**}, {T}_{22}^{**}\right)=-{\uppi }_{j}D.$