Combined Newton–Raphson and Streamlines-Upwind Petrov–Galerkin iterations for nanoparticles transport in buoyancy-driven flow

Abstract

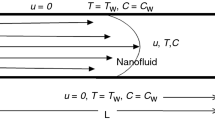
The present study deals with the finite element discretization of nanofluid convective transport in an enclosure with variable properties. We study the Buongiorno model, which couples the Navier–Stokes equations for the base fluid, an advective-diffusion equation for the heat transfer, and an advection-dominated nanoparticle fraction concentration subject to thermophoresis and Brownian motion forces. We develop an iterative numerical scheme that combines Newton’s method (dedicated to the resolution of the momentum and energy equations) with the transport equation that governs the nanoparticles concentration in the enclosure. We show that the Stream-Upwind Petrov–Galerkin regularization approach is required to solve properly the transport equation in Buongiorno’s model, in the Finite Element framework. Indeed, we formulate this ill-posed equation as a variational problem under mean value constraint. Numerical analysis and computations are reported to show the effectiveness of our proposed numerical approach in its ability to provide reasonably good agreement with the experimental results available in the literature.

Appendices

Appendix 1: Density dimensionless derivation

Following Eq.(2.22.3) we have

$$\begin{aligned} \rho _\text {nf}= (1-\phi ^{\star }) \rho _\text {bf}+\phi ^{\star }\rho _\text {np}\end{aligned}$$

note also from Eq. (2.4),

$$\begin{aligned} (\rho \beta )_\text {nf} = (\rho \beta )_\text {bf}(1-\phi ^{\star }) + (\rho \beta )_\text {np}\phi ^{\star }, \end{aligned}$$

where $(\rho \beta )_\text {bf}=\rho _\text {bf}\beta _\text {bf}$ and $(\rho \beta )_\text {np}=\rho _\text {np}\beta _\text {np}$. Hence

$$\begin{aligned} \dfrac{(\rho \beta )_\text {nf} }{\rho _\text {nf}}= & {} \dfrac{\rho _\text {bf}\beta _\text {bf}(1-\phi ^{\star })}{ (1-\phi ^{\star }) \rho _\text {bf}+\phi ^{\star }\rho _\text {np}} + \dfrac{ \rho _\text {np}\beta _\text {np}\phi ^{\star }}{ (1-\phi ^{\star }) \rho _\text {bf}+\phi ^{\star }\rho _\text {np}}\\= & {} \dfrac{\beta _\text {bf}(1-\phi ^{\star })}{ (1-\phi ^{\star })+\phi ^{\star }\dfrac{\rho _\text {np}}{\rho _\text {bf}}} + \dfrac{ \phi ^{\star }}{ (1-\phi ^{\star }) +\phi ^{\star }\dfrac{\rho _\text {np}}{\rho _\text {bf}}}\beta _\text {np}= \beta _\text {bf}\left( \dfrac{(1-\phi ^{\star })}{ (1-\phi ^{\star })+\phi ^{\star }\dfrac{\rho _\text {np}}{\rho _\text {bf}}} + \dfrac{ \phi ^{\star }}{ (1-\phi ^{\star }) +\phi ^{\star }\dfrac{\rho _\text {np}}{\rho _\text {bf}}}\dfrac{\beta _\text {np}}{\beta _\text {bf}} \right) .\\ \end{aligned}$$

Let

$$\begin{aligned} \mathcal {M}:=\left( \dfrac{(1-\phi ^{\star })}{ (1-\phi ^{\star })+\phi ^{\star }\dfrac{\rho _\text {np}}{\rho _\text {bf}}} + \dfrac{ \phi ^{\star }}{ (1-\phi ^{\star }) +\phi ^{\star }\dfrac{\rho _\text {np}}{\rho _\text {bf}}}\dfrac{\beta _\text {np}}{\beta _\text {bf}} \right) . \end{aligned}$$

Appendix 2: Momentum equation

$$\begin{aligned} \left( \mathbf{u} ^{\star }\cdot \nabla ^{\star }\,\right) \mathbf{u} ^{\star }= \dfrac{-1}{\rho _\text {nf}}\nabla ^{\star }\,p^{\star }+ \dfrac{1}{\rho _\text {nf}} \nabla ^{\star }\,\cdot \left( \mu ^{\star }_\text {nf}\left( \nabla ^{\star }\,\mathbf{u} ^{\star }+ (\nabla ^{\star }\,\mathbf{u} ^{\star })^{t}\right) \right) + \dfrac{{\mathbf{g}}}{\rho _\text {nf}}(\rho _{\infty }-\rho _{c}). \end{aligned}$$

(A.1)

Moving toward dimensionless variables the above equation becomes

$$\begin{aligned} \dfrac{\alpha ^2}{L^3} \left( \mathbf {u}\cdot \nabla \right) \mathbf {u}= \dfrac{-\rho _\text {bf}\alpha ^2}{L^{2}\rho _\text {nf}}\nabla ^{\star }\,p^{\star }+ \dfrac{\alpha \mu _\text {bf}}{L^3\rho _\text {nf}} \nabla ^{\star }\,\cdot \left( \mu _\text {nf}\left( \nabla ^{\star }\,\mathbf{u} ^{\star }+ (\nabla ^{\star }\,\mathbf{u} ^{\star })^{t}\right) \right) + \dfrac{{\mathbf{g}}\beta _\text {nf}}{\rho _\text {nf}}\rho _{\infty }(\theta _{h}-\theta _{c})\theta \end{aligned}$$

(A.2)

Multiplying the above equation by $\dfrac{L^3}{\alpha ^2}$ we obtain

$$\begin{aligned} \left( \mathbf {u}\cdot \nabla \right) \mathbf {u}= & {} \dfrac{\rho _\text {bf}}{\rho _\text {nf}} \nabla p + \dfrac{\mu _\text {bf}}{\alpha \rho _\text {bf}\left( 1-\phi +\phi \dfrac{\rho _\text {np}}{\rho _\text {bf}}\right) } \nabla \cdot \left( \mu _\text {nf}\left( \nabla \mathbf {u}+ (\nabla \mathbf {u})^{t}\right) \right) +\dfrac{\mathcal {M}L^3{\mathbf{g}}\beta _\text {nf}}{\alpha ^2\rho _\text {nf}}\rho _{\infty }(\theta _{h}-\theta _{c})\theta , \end{aligned}$$

which rewrites using the non-dimensional constants as follows:

$$\begin{aligned} \left( \mathbf {u}\cdot \nabla \right) \mathbf {u}= & {} \pi ^{m}_{1}(\phi ) \nabla p + \pi ^{m}_{2}(\phi ) \nabla \cdot \left( \mu _\text {nf}\left( \nabla \mathbf {u}+ (\nabla \mathbf {u})^{t}\right) \right) +\pi ^{m}_{3}(\phi )\theta , \end{aligned}$$

(A.3)

where

$$\begin{aligned} \pi ^{m}_{1}(\phi )= & {} \left( 1-\phi +\phi \dfrac{\rho _\text {np}}{\rho _\text {bf}}\right) ^{-1}, \end{aligned}$$

(A.4)

$$\begin{aligned} \pi ^{m}_{2}(\phi )= & {} \text {Pr}\left( 1-\phi +\phi \dfrac{\rho _\text {np}}{\rho _\text {bf}}\right) ^{-1}, \end{aligned}$$

(A.5)

$$\begin{aligned} \pi ^{m}_{3}(\phi )= & {} \text {Pr}\text {Ra}_\text {nf} \mathcal {M}. \end{aligned}$$

(A.6)

Appendix 3: Energy equation

The dimensional energy equation writes

$$\begin{aligned} \left( \mathbf{u} ^{\star }\cdot \nabla ^{\star }\,\theta ^{\star }\right) = \dfrac{1}{c_\text {nf}\rho _\text {nf}} \nabla ^{\star }\,\cdot \left( k^{\star }_\text {nf}\nabla ^{\star }\,\theta ^{\star }\right) + \left( \dfrac{\rho _\text {np}c_\text {np}}{\rho _\text {nf}c_\text {nf}}\right) \left( \dfrac{D^{\star }_{\theta }}{\theta ^{\star }_{C}} \nabla ^{\star }\,\theta ^{\star }\cdot \nabla ^{\star }\,\theta ^{\star }+D_{\omega }^{\star }\nabla ^{\star }\,\phi \cdot \nabla ^{\star }\,\theta ^{\star }\right) . \end{aligned}$$

Moving toward dimensionless variables the above equation writes

$$\begin{aligned} \dfrac{\alpha (\theta ^{\star }_{H}-\theta ^{\star }_{C})}{L^2}\left( \mathbf {u}\cdot \nabla \theta \right)= & {} \dfrac{k_\text {bf}(\theta ^{\star }_{H}-\theta ^{\star }_{C})}{L^2c_\text {nf}\rho _\text {nf}} \nabla \cdot \left( k_\text {nf}\nabla \theta \right) + \left( \dfrac{\rho _\text {np}c_\text {np}}{\rho _\text {nf}c_\text {nf}}\right) \dfrac{D_{\theta _{c}} (\theta ^{\star }_{H}-\theta ^{\star }_{C})^{2}}{\theta ^{\star }_{C}L^2} \left( D_{\theta }\nabla \theta \cdot \nabla \theta \right) \\&+ \left( \dfrac{\rho _\text {np}c_\text {np}}{\rho _\text {nf}c_\text {nf}}\right) \dfrac{\phi _\text {b}D_{\omega _{c}} (\theta ^{\star }_{H}-\theta ^{\star }_{C})}{L^2} \left( D_{\omega }\nabla \phi \cdot \nabla \theta \right) . \end{aligned}$$

Multiplying the above equation by $\dfrac{L^2}{\alpha (\theta ^{\star }_{H}-\theta ^{\star }_{C})}$ we obtain

$$\begin{aligned} \left( \mathbf {u}\cdot \nabla \theta \right)= & {} \dfrac{k_\text {bf}}{\alpha c_\text {nf}\rho _\text {nf}} \nabla \cdot \left( k_\text {nf}\nabla \theta \right) + \left( \dfrac{\rho _\text {np}c_\text {np}}{\rho _\text {nf}c_\text {nf}}\right) \dfrac{D_{\theta _{c}} (\theta ^{\star }_{H}-\theta ^{\star }_{C})}{\alpha \theta ^{\star }_{C}} \left( D_{\theta }\nabla \theta \cdot \nabla \theta \right) \\&+\left( \dfrac{\rho _\text {np}c_\text {np}}{\rho _\text {nf}c_\text {nf}}\right) \dfrac{\phi _\text {b}D_{\omega _{c}}}{\alpha } \left( D_{\omega }\nabla \phi \cdot \nabla \theta \right) , \end{aligned}$$

which rewrites using non-dimensional variables as follows:

$$\begin{aligned} \left( \mathbf {u}\cdot \nabla \theta \right)= & {} \pi ^{e}_{1} \nabla \cdot \left( k_\text {nf}\nabla \theta \right) +\pi ^{e}_{2} \left( D_{\theta }\nabla \theta \cdot \nabla \theta \right) +\pi ^{e}_{3} \left( D_{\omega }\nabla \phi \cdot \nabla \theta \right) , \end{aligned}$$

where

Appendix 4: Nanoparticle transport equation

The particle dimensional equation writes

$$\begin{aligned} \nabla ^{\star }\,\cdot \nabla ^{\star }\,\phi ^{\star }= \nabla ^{\star }\,\cdot \left( D_{\omega }^{\star } \nabla ^{\star }\,\phi ^{\star }+\dfrac{D_{\theta ^{\star }}^{\star }}{\theta ^{\star }_{C}}\nabla ^{\star }\,\theta ^{\star }\right) \end{aligned}$$

using the non-dimensional equations the above equation writes

$$\begin{aligned} \dfrac{\alpha }{L^2}\phi _\text {b}\nabla \cdot \nabla \phi = \dfrac{\phi _\text {b}D_{\omega _{c}}}{L^2}\nabla \cdot \left( D_{\omega } \nabla \phi \right) +\dfrac{D_{\theta ^{\star }_{C}} (\theta ^{\star }_{H}-\theta ^{\star }_{C})}{ L^2\theta ^{\star }_{C}} \nabla \cdot \left( D_{\theta }\nabla \theta \right) . \end{aligned}$$

Multiplying the above by $\dfrac{L^2}{\alpha \phi _\text {b}}$ we obtain

$$\begin{aligned} \phi _\text {b}\nabla \cdot \nabla \phi= & {} \dfrac{D_{\omega _{c}}}{\alpha }\nabla \cdot \left( D_{\omega } \nabla \phi \right) +\dfrac{D_{\theta ^{\star }_{C}}^{\star } (\theta ^{\star }_{H}-\theta ^{\star }_{C})}{\phi _\text {b}\alpha \theta ^{\star }_{C}} \nabla \cdot \left( D_{\theta }\nabla \theta ^{\star }\right) .\\ \nabla \cdot \nabla \phi= & {} \pi ^{p}_{1}\nabla \cdot \left( D_{\omega } \nabla \phi \right) +\pi ^{p}_{2} \nabla \cdot \left( D_{\theta }\nabla \theta ^{\star }\right) , \end{aligned}$$

where

$$\begin{aligned}&\pi ^{p}_{1} = \dfrac{D_{\omega _{c}}}{\alpha }=\dfrac{1}{\text {Le}},\\&\pi ^{p}_{2} = \dfrac{D_{\theta ^{\star }_{C}} (\theta ^{\star }_{H}-\theta ^{\star }_{C})}{ L^2\theta ^{\star }_{C}}=\dfrac{\text {St}\text {Pr}}{\text {Sc}}\dfrac{\theta ^{\star }_{H}-\theta ^{\star }_{C}}{\theta ^{\star }_{C}} \dfrac{1}{\phi _\text {b}}. \end{aligned}$$