Pure Torsion for Stretch-Based Constitutive Models for Incompressible Isotropic Hyperelastic Soft Materials

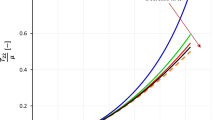
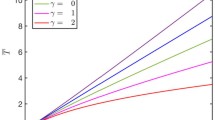
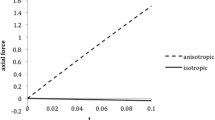
Abstract

Stretch-based constitutive models for isotropic hyperelastic materials as alternatives to the classical strain invariant models have been the subject of considerable recent attention largely motivated by application to modelling the mechanical response of soft tissues. One such four-parameter constitutive model was proposed recently by Anssari-Benam (J. Elast. 153:219–244, 2023) for incompressible isotropic hyperelastic soft materials. The model was deemed to be comprehensive in that several well-known strain-energies may be recovered for some particular and limiting values of some of the parameters. The model is a generalization of several related simpler models based on microstructural considerations that have been shown to match well with experimental data for a wide variety of soft materials. In particular, the celebrated one-term Ogden model is obtained as a special case. Here we examine the response of the new model for the problem of pure torsion for a solid circular cylinder with particular emphasis on the Poynting effects governing the lengthening or shortening of the cylinder.

Appendices

Appendix A: Evaluation of the Integrals (3.26), (3.27)

We recall from Sect. 3 that

$$ K_{1} = \int _{1}^{\lambda _{A}} g(x)H(x)dx + \int _{1}^{\frac{1}{\lambda _{A}}} g(x)H(x)dx - \frac{1}{\alpha} \int _{1}^{\lambda _{A}} (x - x^{ - 3})H(x)dx $$

(A.1)

and

$$ K_{2} = \int _{1}^{\lambda _{A}} h(x)H(x)dx + \int _{1}^{\frac{1}{\lambda _{A}}} h(x)H(x)dx, $$

(A.2)

where $H$ is given by

$$ H \equiv - \frac{3(n - 1)N}{x^{\alpha} + x^{ - \alpha} + 1 - 3N} \ge 0, $$

(A.3)

and where $g(x)$ and $h(x)$ are given by

$$ g(x) = \frac{\left ( x^{2} - 2 \right )\left ( 1 - x^{2} \right )x^{\alpha - 3}}{\alpha},\qquad h(x) = \frac{\left ( x^{2} - 1 \right )^{2}x^{\alpha - 3}}{\alpha}. $$

(A.4)

Case 1 $n = 3, \alpha = 2$: Verification of (4.9), (4.10)

Thus we have

$$ g(x) = \frac{\left ( x^{2} - 2 \right )\left ( 1 - x^{2} \right )}{2x}, h(x) = \frac{\left ( x^{2} - 1 \right )^{2}}{2x}, H = - \frac{6N}{x^{2} + x^{ - 2} + 1 - 3N}. $$

(A.5)

To evaluate $K_{1}$, it proves convenient to combine the first and third integrals in (A.1) to get

$$ I_{1} \equiv - 3N\int _{1}^{\lambda _{A}} \frac{\left ( - x^{3} + x^{ - 3} - 2x^{ - 1} + 2x \right )dx}{x^{2} + x^{ - 2} + 1 - 3N}. $$

(A.6)

The second integral in (A.1), denoted by $I_{2}$, can be converted to an integral with the same upper limit as in (A.6) on using an obvious change of variable to read

$$ I_{2} \equiv 3N\int _{1}^{\lambda _{A}} \frac{\left ( 3x^{ - 3} - x^{ - 5} - 2x^{ - 1} \right )dx}{x^{2} + x^{ - 2} + 1 - 3N}. $$

(A.7)

Thus we find that

$$ K_{1} = I_{1} + I_{2} = 3N\int _{1}^{\lambda _{A}} \frac{\left ( x^{3} + 2x^{ - 3} - x^{ - 5} - 2x \right )dx}{x^{2} + x^{ - 2} + 1 - 3N}. $$

(A.8)

It is convenient to rewrite this as

$$ K_{1} = - 9(1 - N)N\int _{1}^{\lambda _{A}} \frac{\left ( x - x^{ - 3} \right )dx}{x^{2} + x^{ - 2} + 1 - 3N} + 3N\int _{1}^{\lambda _{A}} \left ( x - x^{ - 3} \right )dx, $$

(A.9)

so that we obtain

$$ K_{1} = - \frac{9(1 - N)N}{2}\ln \left ( x^{2} + x^{ - 2} + 1 - 3N \right )|_{1}^{\lambda _{A}} + \frac{3N}{2}\left ( x^{2} + x^{ - 2} \right )|_{1}^{\lambda _{A}}. $$

(A.10)

On evaluation and using the fact that $\lambda _{A}^{2} + \lambda _{A}^{ - 2} = 2 + \psi ^{2}A^{2}$, we find that

$$ K_{1} = \frac{3N}{2}\psi ^{2}A^{2} + \frac{9N(N - 1)}{2}\ln \left ( \frac{\psi ^{2}A^{2} - 3N + 3}{3 - 3N} \right ). $$

(A.11)

On substitution into (4.6) and using the first of (4.8), we recover (4.9) as desired. The derivation of (4.10) follows along similar lines.

Case 2 $n \to \infty , \alpha = 2$:Verification of (4.15).

When $\alpha = 2$, we have, on recalling (3.15), (3.18) and (4.12),

$$ g(x) = \frac{\left ( x^{2} - 2 \right )\left ( 1 - x^{2} \right )}{2x}, h(x) = \frac{\left ( x^{2} - 1 \right )^{2}}{2x}, G = - \frac{3(N - 1)}{x^{2} + x^{ - 2} + 1 - 3N}. $$

(A.12)

Proceeding from (4.13) as in (A.6)–(A.11), and setting $J_{m} = 3\left ( N - 1 \right )$ one finds that

$$ \hat{N} = \frac{\mu \pi J_{m}A^{2}}{2}\left [ 1 + \frac{J_{m}}{\psi ^{2}A^{2}}\ln (1 - \frac{\psi ^{2}A^{2}}{J_{m}}) \right ], $$

(A.13)

which is the result (4.15). This is exactly the result obtained in [27] for the Gent model derived on using the classical invariant approach.

Appendix B: Evaluation of the Integrals $K_{3}$, $K_{4}$

$\alpha = 6$: We recall from (3.26) in Sect. 3 that in this case

$$ K_{3} = \int _{1}^{\lambda _{A}} g(x)H(x)dx + \int _{1}^{\frac{1}{\lambda _{A}}} g(x)H(x)dx - \frac{1}{6}\int _{1}^{\lambda _{A}} (x - x^{ - 3})H(x)dx $$

(B.1)

where $H$ is given by

$$ H \equiv - \frac{3(n - 1)N}{x^{6} + x^{ - 6} + 1 - 3N} \ge 0, $$

(B.2)

and where $g(x)$ is given by

$$ g(x) = \frac{\left ( x^{2} - 2 \right )\left ( 1 - x^{2} \right )x^{3}}{6}. $$

(B.3)

To evaluate $K_{3}$, it proves convenient to combine the first and third integrals in (B.1) to get

$$ I_{3} \equiv - \frac{(n - 1)N}{2}\int _{1}^{\lambda _{A}} \frac{\left ( 3x^{5} - x^{7} - 2x^{3} - x + x^{ - 3} \right )dx}{x^{6} + x^{ - 6} + 1 - 3N}. $$

(B.4)

The second integral in (B.1) can be combined with (B.4) on using a change of variable and so one obtains the analog of (A.8) namely

$$ K_{3} = \frac{(n - 1)N}{2}\int _{1}^{\lambda _{A}} \frac{\left ( 3x^{ - 7} - 3x^{5} - x^{ - 9} - 2x^{ - 5} + x^{7} + 2x^{3} + x - x^{ - 3} \right )dx}{x^{6} + x^{ - 6} + 1 - 3N}. $$

(B.5)

This can be rewritten as

$$\begin{aligned} K_{3} = \frac{(n - 1)N}{2}\int _{1}^{\lambda _{A}} \frac{ - 3\left ( x^{5} - x^{ - 7} \right )dx}{x^{6} + x^{ - 6} + 1 - 3N} + \frac{(n - 1)N}{2}\int _{1}^{\lambda _{A}} \left ( x - x^{ - 3} \right )dx \\ + \frac{3(n - 1)N}{2}\int _{1}^{\lambda _{A}} \frac{x^{3} - x^{ - 5} + N\left ( x - x^{ - 3} \right )dx}{x^{6} + x^{ - 6} + 1 - 3N}. \end{aligned}$$

(B.6)

The first two integrals are readily evaluated to give

$$ K_{3} = \frac{(n - 1)N}{2}\left [ - \frac{1}{2}\ln \left ( \frac{9\psi ^{2}A^{2} + 6\psi ^{4}A^{4} + \psi ^{6}A^{6} - 3N + 3}{3 - 3N} \right ) + \frac{\psi ^{2}A^{2}}{2} + 3J_{1} \right ], $$

(B.7)

where $J_{1}$ is given by

$$ J_{1} = \int _{1}^{\lambda _{A}} \frac{x^{3} - x^{ - 5} + N\left ( x - x^{ - 3} \right )dx}{x^{6} + x^{ - 6} + 1 - 3N}. $$

(B.8)

$\alpha = 8$:

In this case, the analog of (B.5) is

$$ K_{4} = \frac{3(n - 1)N}{8}\int _{1}^{\lambda _{A}} \frac{\left ( 3x^{ - 9} - 3x^{7} - x^{ - 11} - 2x^{ - 7} + x^{9} + 2x^{5} + x - x^{ - 3} \right )dx}{x^{8} + x^{ - 8} + 1 - 3N}. $$

(B.9)

This can be rewritten as

$$\begin{aligned} K_{4} = \frac{3(n - 1)N}{8}\int _{1}^{\lambda _{A}} \frac{ - 3\left ( x^{7} - x^{ - 9} \right )dx}{x^{8} + x^{ - 8} + 1 - 3N} + \frac{3(n - 1)N}{8}\int _{1}^{\lambda _{A}} \left ( x - x^{ - 3} \right )dx \\ + \frac{9(n - 1)N}{8}\int _{1}^{\lambda _{A}} \frac{x^{5} - x^{ - 7} + N\left ( x - x^{ - 3} \right )dx}{x^{8} + x^{ - 8} + 1 - 3N}. \end{aligned}$$

(B.10)

Thus we obtain

$$\begin{aligned} K_{4} = {}&\frac{3(n - 1)N}{8}\left [ - \frac{3}{8}\ln \left ( \frac{16\psi ^{2}A^{2} + 20\psi ^{4}A^{4} + 8\psi ^{6}A^{6} + \psi ^{8}A^{8} - 3N + 3}{3 - 3N} \right ) \right. \\ &{}\left.+ \frac{\psi ^{2}A^{2}}{2} + 3J_{2} \right ] \end{aligned}$$

(B.11)

where $J_{2}$ is given by

$$ J_{2} = \int _{1}^{\lambda _{A}} \frac{x^{5} - x^{ - 7} + N\left ( x - x^{ - 3} \right )dx}{x^{8} + x^{ - 8} + 1 - 3N}. $$

(B.12)