Strategic analysis of RFID adoption sequences in a supply chain with Cournot competition: effects of ordering-timing strategies

Abstract

We investigate the effects of ordering-timing strategies on radio-frequency identification (RFID) adoption sequences and firms’ profitability in a supply chain with a manufacturer and two retailers, both of whom suffer from misplaced inventory problems. The manufacturer is the Stackelberg leader, the two retailers engage in a quantity competition and simultaneously/sequentially make decisions on RFID adoption under two ordering-timing strategies. We derive the equilibrium strategies for RFID adoption and characterize each partner’s optimal order quantity and profit. We show that the equilibrium strategies for RFID adoption are identical regardless of the two competing retailers’ RFID adoption sequences. The retailer with a smaller misplacement rate will never adopt RFID alone. However, for the retailer with a higher misplacement rate: the more intense the competition is, the more likely he is to adopt RFID; ordering later always promotes him to adopt RFID; ordering earlier is more attractive than adopting RFID when the tagging cost is intermediate. Furthermore, as long as one or both retailers adopt RFID, ordering simultaneously can achieve Pareto improvement for both retailers. When neither retailer adopts RFID, the optimal ordering strategies depend on the misplacement rates and competition intensity.

Appendix

Proof of Proposition 1

• (a) For Nash quantity game, we solve the problem by backward induction with $p_{i - y}^{NN} = a - (1 - \alpha_{i} )q_{i - y}^{NN} - \gamma (1 - \alpha_{j} )q_{j - y}^{NN}$. In the second stage of the output game, both retailers decide on their order quantities $q_{1 - n}^{NN}$ and $q_{2 - n}^{NN}$ on the basis of the manufacturer’s wholesale price $w_{n}^{NN}$. With ${{\partial^{2} \pi_{i - n}^{NN} } \mathord{\left/ {\vphantom {{\partial^{2} \pi_{i - n}^{NN} } {\partial^{2} q_{i - n}^{NN} }}} \right. \kern-0pt} {\partial^{2} q_{i - n}^{NN} }} = - 2(1 - \alpha_{i} )^{2} < 0$, we set ${{\partial \pi_{i - n}^{NN} } \mathord{\left/ {\vphantom {{\partial \pi_{i - n}^{NN} } {\partial q_{i - n}^{NN} }}} \right. \kern-0pt} {\partial q_{i - n}^{NN} }} = 0$ and generate the retailer $i$‘s order quantity $q_{i - n}^{NN} = \frac{a}{{(2 + \gamma )(1 - \alpha_{i} )}} - \frac{{[2(1 - \alpha_{j} ) - \gamma (1 - \alpha_{i} )]w_{n}^{NN} }}{{(4 - \gamma^{2} )(1 - \alpha_{i} )^{2} (1 - \alpha_{j} )}}$. In the first stage, the manufacturer determines her wholesale price taking into account the two retailers’ order quantity decisions. With $\frac{{\partial^{2} \pi_{m - n}^{NN} }}{{\partial^{2} w_{n}^{NN} }} = - \frac{{4(2 - \gamma )(1 - \alpha_{1} )(1 - \alpha_{2} ) + 4[(1 - \alpha_{1} ) - (1 - \alpha_{2} )]^{2} }}{{(4 - \gamma^{2} )(1 - \alpha_{1} )^{2} (1 - \alpha_{2} )^{2} }} < 0$, we set ${{\partial \pi_{m - n}^{NN} } \mathord{\left/ {\vphantom {{\partial \pi_{m - n}^{NN} } {\partial w_{n}^{NN} }}} \right. \kern-0pt} {\partial w_{n}^{NN} }} = 0$ and generate the optimal wholesale price $w_{n}^{{NN^{*} }} = v_{n} a + {{c_{m} } \mathord{\left/ {\vphantom {{c_{m} } 2}} \right. \kern-0pt} 2}$.

• (b) For Stackelberg quantity game, we solve the problem by backward induction with $p_{i - y}^{NN} = a - (1 - \alpha_{i} )q_{i - y}^{NN} - \gamma (1 - \alpha_{j} )q_{j - y}^{NN}$. In the third stage, the retailer 2 decides on his order quantity on the basis of the retailer 1’s order quantity. With ${{\partial^{2} \pi_{2 - s}^{NN} } \mathord{\left/ {\vphantom {{\partial^{2} \pi_{2 - s}^{NN} } {\partial^{2} q_{2 - s}^{NN} }}} \right. \kern-0pt} {\partial^{2} q_{2 - s}^{NN} }} = - 2(1 - \alpha_{1} )^{2} < 0$, we set ${{\partial \pi_{2 - s}^{NN} } \mathord{\left/ {\vphantom {{\partial \pi_{2 - s}^{NN} } {\partial q_{2 - s}^{NN} }}} \right. \kern-0pt} {\partial q_{2 - s}^{NN} }} = 0$ and generate the retailer 2’s order quantity $q_{2 - s}^{NN} = \frac{{(1 - \alpha_{2} )[a - q_{1 - s}^{NN} \gamma (1 - \alpha_{1} )] - w_{s}^{NN} }}{{2(1 - \alpha_{2} )^{2} }}$. In the second stage, anticipating the retailer 2’s response function $q_{2 - s}^{NN}$, the optimum $q_{1 - s}^{NN}$ is determined by ${{\partial \pi_{1 - s}^{NN} } \mathord{\left/ {\vphantom {{\partial \pi_{1 - s}^{NN} } {\partial q_{1 - s}^{NN} }}} \right. \kern-0pt} {\partial q_{1 - s}^{NN} }} = 0$ because ${{\partial^{2} \pi_{1 - s}^{NN} } \mathord{\left/ {\vphantom {{\partial^{2} \pi_{1 - s}^{NN} } {\partial^{2} q_{1 - s}^{NN} }}} \right. \kern-0pt} {\partial^{2} q_{1 - s}^{NN} }} = - (2 - \gamma^{2} )(1 - \alpha_{1} )(1 - \alpha_{2} ) < 0$, leading to the retailer 1’s order quantity is $q_{1 - s}^{NN} = \frac{(2 - \gamma )}{{2(1 - \alpha_{1} )(2 - \gamma^{2} )}}a + \frac{{[\gamma (1 - \alpha_{1} ) - 2(1 - \alpha_{2} )]}}{{2(1 - \alpha_{2} )(1 - \alpha_{1} )^{2} (2 - \gamma^{2} )}}w_{s}^{NN}$. In the first stage, the manufacturer determines her wholesale price taking into account the two retailers’ order quantity decisions. The optimum $w_{s}^{NN}$ is determined by ${{\partial \pi_{m - s}^{NN} } \mathord{\left/ {\vphantom {{\partial \pi_{m - s}^{NN} } {\partial w_{s}^{NN} }}} \right. \kern-0pt} {\partial w_{s}^{NN} }} = 0$ because $\frac{{\partial^{2} \pi_{m - s}^{NN} }}{{\partial^{2} w_{s}^{NN} }} = - \frac{{2(2 - \gamma )(1 - \alpha_{1} )^{2} + [2(1 - \alpha_{1} ) - \gamma (1 - \alpha_{2} )]^{2} }}{{2(2 - \gamma^{2} )(1 - \alpha_{1} )^{2} (1 - \alpha_{2} )^{2} }} < 0$, resulting in the optimal wholesale price is $w_{s}^{{NN^{*} }} = v_{s} a + {{c_{m} } \mathord{\left/ {\vphantom {{c_{m} } 2}} \right. \kern-0pt} 2}$.

1.1 Summary of abbreviations in Proposition 1

$$ v_{n} = \frac{{(2 - \gamma )(1 - \alpha_{1} )(1 - \alpha_{2} )}}{4}\left( {\frac{{(1 - \alpha_{1} ) + (1 - \alpha_{2} )}}{{(\alpha_{1} - \alpha_{2} )^{2} + (2 - \gamma )(1 - \alpha_{1} )(1 - \alpha_{2} )}}} \right), $$

$$ v_{s} = \frac{{(1 - \alpha_{1} )(1 - \alpha_{2} )}}{2}\left( {\frac{{(4 - 2\gamma - \gamma^{2} )(1 - \alpha_{1} ) + (4 - 2\gamma )(1 - \alpha_{2} )}}{{(4 - \gamma^{2} )(1 - \alpha_{1} )^{2} - 4\gamma (1 - \alpha_{1} )(1 - \alpha_{2} ) + 4(1 - \alpha_{2} )^{2} }}} \right), $$

$$ \left\{ \begin{aligned} \pi_{i - n}^{NN*} = \left( {\frac{1}{{(4 - \gamma^{2} )}}\left[ {(2 - \gamma )a + (2v_{n} a + c_{m} )\left( {\frac{\gamma }{{2(1 - \alpha_{j} )}} - \frac{1}{{1 - \alpha_{i} }}} \right)} \right]} \right)^{2} \hfill \\ \pi_{m - n}^{NN *} = \frac{{\left( {2v_{n} a - c_{m} } \right)}}{{2(2 + \gamma )(1 - \alpha_{1} )(1 - \alpha_{2} )}}\left[ {\left( {2 - \alpha_{1} - \alpha_{2} } \right)a - \frac{{\left( {2v_{n} a + c_{m} } \right)}}{2 - \gamma }\left( {\frac{{1 - \alpha_{2} }}{{1 - \alpha_{1} }} + \frac{{1 - \alpha_{1} }}{{1 - \alpha_{2} }} - \gamma } \right)} \right] \hfill \\ \end{aligned} \right., $$

$$ \left\{ \begin{aligned} \pi_{1 - s}^{NN*} = \frac{1}{{8(2 - \gamma^{2} )}}\left[ {(2 - \gamma )a - (2v_{s} a + c_{m} )\left( {\frac{1}{{1 - \alpha_{1} }} - \frac{\gamma }{{2(1 - \alpha_{2} )}}} \right)} \right]^{2} \hfill \\ \pi_{2 - s}^{NN*} = \left[ {\frac{{(4 - \gamma^{2} - 2\gamma )a}}{{4(2 - \gamma^{2} )}} - \frac{{(2v_{s} a + c_{m} )}}{{4(2 - \gamma^{2} )}}\left( {\frac{{4 - \gamma^{2} }}{{2(1 - \alpha_{2} )}} - \frac{\gamma }{{1 - \alpha_{1} }}} \right)} \right]^{2} \hfill \\ \pi_{m - s}^{NN*} = \left( {\frac{{2v_{s} a - c_{m} }}{{16(2 - \gamma^{2} )(1 - \alpha_{2} )}}} \right)\left[ {(4 + \gamma^{2} - 2\gamma )a - 2(2v_{s} a + c_{m} )\left( {\frac{2 - \gamma }{{1 - \alpha_{1} }} + \frac{{4 - \gamma^{2} - 2\gamma }}{{2(1 - \alpha_{2} )}}} \right)} \right] \hfill \\ \end{aligned} \right.. $$

1.2 Summary of abbreviations in Corollary 1

$$ \begin{aligned} \overline{\alpha }_{i - n}^{NN} & = \frac{{\gamma + \alpha_{j} + (1 - \alpha_{j} )\sqrt {\gamma + 2} }}{1 + \gamma }, \\ \overline{\alpha }_{1 - s}^{NN} & = \frac{{\gamma (8 - 4\gamma - \gamma^{2} ) + 2(4 - 2\gamma - \gamma^{2} )\alpha_{2} + 2\sqrt {2(8 - 4\gamma - \gamma^{2} )(2 - \gamma^{2} )} (1 - \alpha_{2} )}}{{8 + 4\gamma - 6\gamma^{2} - \gamma^{3} }}, \\ \overline{\alpha }_{2 - s}^{NN} & = \frac{{\gamma (8 - 4\gamma - \gamma^{2} ) + (8 - 4\gamma - 2\gamma^{2} + \gamma^{3} )\alpha_{1} + \sqrt {2(8 - 4\gamma - \gamma^{2} )(4 - \gamma^{2} )(2 - \gamma^{2} )} (1 - \alpha_{1} )}}{{8 + 4\gamma - 6\gamma^{2} }}, \\ \overline{\alpha }_{1 - s(q1)}^{NN} & = \, 1 - \frac{{2w_{s}^{NN*} \sqrt {1 - \alpha_{2} } }}{{\sqrt {\left( {(1 - \alpha_{2} )*{{\partial w_{s}^{NN*} } \mathord{\left/ {\vphantom {{\partial w_{s}^{NN*} } {\partial \alpha_{1} }}} \right. \kern-0pt} {\partial \alpha_{1} }} + 2\gamma w_{s}^{NN*} } \right)*{{\partial w_{s}^{NN*} } \mathord{\left/ {\vphantom {{\partial w_{s}^{NN*} } {\partial \alpha_{1} }}} \right. \kern-0pt} {\partial \alpha_{1} }}} - \sqrt {1 - \alpha_{2} } *{{\partial w_{s}^{NN*} } \mathord{\left/ {\vphantom {{\partial w_{s}^{NN*} } {\partial \alpha_{1} }}} \right. \kern-0pt} {\partial \alpha_{1} }}}}, \\ \overline{\alpha }_{1 - s(q2)}^{NN} & = 1 - \frac{{2\gamma w_{s}^{NN*} \sqrt {1 - \alpha_{2} } }}{{\sqrt {\gamma \left( {w_{s}^{NN*} (8 - 2\gamma^{2} ) + \gamma (1 - \alpha_{2} )} \right)*{{\partial w_{s}^{NN*} } \mathord{\left/ {\vphantom {{\partial w_{s}^{NN*} } {\partial \alpha_{1} }}} \right. \kern-0pt} {\partial \alpha_{1} }}} - \gamma \sqrt {1 - \alpha_{2} } *{{\partial w_{s}^{NN*} } \mathord{\left/ {\vphantom {{\partial w_{s}^{NN*} } {\partial \alpha_{1} }}} \right. \kern-0pt} {\partial \alpha_{1} }}}}, \\ \overline{\alpha }_{2 - s(q1)}^{NN} & = 1 - \frac{{4\gamma w_{s}^{NN*} (1 - \alpha_{1} )}}{{2w_{s}^{NN*} - \xi_{1} \, + \sqrt {(\xi_{1} )^{2} - 4w_{s}^{NN*} \left( {\xi_{1} - 4(1 - \alpha_{1} )\gamma *{{\partial w_{s}^{NN*} } \mathord{\left/ {\vphantom {{\partial w_{s}^{NN*} } {\partial \alpha_{2} }}} \right. \kern-0pt} {\partial \alpha_{2} }} - w_{s}^{NN*} } \right)} }}, \\ \overline{\alpha }_{2 - s(q2)}^{NN} & = 1 - \frac{{8w_{s}^{NN*} (4 - \gamma^{2} )(1 - \alpha_{1} )}}{{4\gamma w_{s}^{NN*} + \xi_{2} + \sqrt {(\xi_{2} )^{2} + 8w_{s}^{NN*} \left[ {\left( {\xi_{2} \gamma + 2(1 - \alpha_{1} )(3 + \gamma )(4 - \gamma^{2} )*{{\partial w_{s}^{NN*} } \mathord{\left/ {\vphantom {{\partial w_{s}^{NN*} } {\partial \alpha_{2} }}} \right. \kern-0pt} {\partial \alpha_{2} }}} \right) + 2\gamma^{2} w_{s}^{NN*} } \right]} }}, \\ \xi_{1} & = (1 - \alpha_{1} )\left( {(2 - \gamma )a + \gamma *{{\partial w_{s}^{NN*} } \mathord{\left/ {\vphantom {{\partial w_{s}^{NN*} } {\partial \alpha_{2} }}} \right. \kern-0pt} {\partial \alpha_{2} }}} \right),\quad \xi_{2} = (1 - \alpha_{1} )\left( {(4 - 2\gamma - \gamma^{2} )a - 2(4 - \gamma^{2} )*{{\partial w_{s}^{NN*} } \mathord{\left/ {\vphantom {{\partial w_{s}^{NN*} } {\partial \alpha_{2} }}} \right. \kern-0pt} {\partial \alpha_{2} }}} \right). \\ \end{aligned} $$

Proof of Proposition 2

• (a) For Nash quantity game, we solve the problem by backward induction with $p_{1 - y}^{AN} = a - q_{1 - y}^{AN} - \gamma (1 - \alpha_{2} )q_{2 - y}^{AN}$ and $p_{2 - y}^{AN} = a - (1 - \alpha_{2} )q_{2 - y}^{AN} - \gamma q_{1 - y}^{AN}$. The two stage of the output game are similar to that of Proposition 1 and hence are omitted. We only discuss the first stage of the pricing game. The manufacturer determines the wholesale price $w_{n}^{AN}$ and the cost-sharing coefficient $\theta_{n}^{AN}$, with consideration of the two retailers’ order quantity decisions. We obtain the Hessian matrix $H_{n}^{AN} = {{4c_{t}^{2} } \mathord{\left/ {\vphantom {{4c_{t}^{2} } {(4 - \gamma^{2} )(1 - \alpha_{2} )^{2} }}} \right. \kern-0pt} {(4 - \gamma^{2} )(1 - \alpha_{2} )^{2} }} > 0$, where $\frac{{\partial^{2} \pi_{m - n}^{AN} }}{{\partial^{2} w_{n}^{AN} }} = - \frac{{4[(2 - \gamma )(1 - \alpha_{2} ) + \alpha_{2}^{2} ]}}{{(4 - \gamma^{2} )(1 - \alpha_{2} )^{2} }} < 0$, $\frac{{\partial^{2} \pi_{m - n}^{AN} }}{{\partial w_{n}^{AN} \partial \theta_{n}^{AN} }} = \frac{{2(\gamma + 2\alpha_{2} - 2)c_{t} }}{{(4 - \gamma^{2} )(1 - \alpha_{2} )}}$, $\frac{{\partial^{2} \pi_{m - n}^{AN} }}{{\partial \theta_{n}^{AN} \partial w_{n}^{AN} }} = \frac{{2(\gamma + 2\alpha_{2} - 2)c_{t} }}{{(4 - \gamma^{2} )(1 - \alpha_{2} )}}$, and $\frac{{\partial^{2} \pi_{m - n}^{AN} }}{{\partial^{2} \theta_{n}^{AN} }} = \frac{{4c_{t}^{2} }}{{(\gamma^{2} - 4)}}$. On this basis, we know that $H_{n}^{AN}$ is negative definite, hence the manufacturer has the maximum profit. The optimum $w_{n}^{AN}$ and $\theta_{n}^{AN}$ are determined by ${{\partial \pi_{m - n}^{AN} } \mathord{\left/ {\vphantom {{\partial \pi_{m - n}^{AN} } {\partial w_{n}^{AN} }}} \right. \kern-0pt} {\partial w_{n}^{AN} }} = 0$ and ${{\partial \pi_{m - n}^{AN} } \mathord{\left/ {\vphantom {{\partial \pi_{m - n}^{AN} } {\partial \theta_{n}^{AN} }}} \right. \kern-0pt} {\partial \theta_{n}^{AN} }} = 0$.

• (b) For Stackelberg quantity game, we solve the problem by backward induction with $p_{1 - y}^{AN} = a - q_{1 - y}^{AN} - \gamma (1 - \alpha_{2} )q_{2 - y}^{AN}$ and $p_{2 - y}^{AN} = a - (1 - \alpha_{2} )q_{2 - y}^{AN} - \gamma q_{1 - y}^{AN}$. The third stage and the two stage of the output game are similar to those of Proposition 1 and hence are omitted. We only discuss the first stage of the pricing game. The manufacturer determines the wholesale price $w_{s}^{AN}$ and the cost-sharing coefficient $\theta_{s}^{AN}$, with consideration of the two retailers’ order quantity decisions. We obtain the Hessian matrix $H_{s}^{AN} = {{2c_{t}^{2} } \mathord{\left/ {\vphantom {{2c_{t}^{2} } {(2 - \gamma^{2} )(1 - \alpha_{2} )^{2} }}} \right. \kern-0pt} {(2 - \gamma^{2} )(1 - \alpha_{2} )^{2} }} > 0$, where $\frac{{\partial^{2} \pi_{m - s}^{AN} }}{{\partial^{2} w_{s}^{AN} }} = - \frac{{4(1 - \alpha_{2} )(2 - \gamma ) + 4\alpha_{2}^{2} - \gamma^{2} }}{{2(2 - \gamma^{2} )(1 - \alpha_{2} )^{2} }} < 0$, $\frac{{\partial^{2} \pi_{m - s}^{AN} }}{{\partial w_{s}^{AN} \partial \theta_{s}^{AN} }} = \frac{{(\gamma + 2\alpha_{2} - 2)c_{t} }}{{(2 - \gamma^{2} )(1 - \alpha_{2} )}}$, $\frac{{\partial^{2} \pi_{m - s}^{AN} }}{{\partial \theta_{s}^{AN} \partial w_{s}^{AN} }} = \frac{{(\gamma + 2\alpha_{2} - 2)c_{t} }}{{(2 - \gamma^{2} )(1 - \alpha_{2} )}}$, and $\frac{{\partial^{2} \pi_{m - s}^{AN} }}{{\partial^{2} \theta_{s}^{AN} }} = \frac{{2c_{t}^{2} }}{{(\gamma^{2} - 2)}}$. On this basis, we know that $H_{s}^{AN}$ is negative definite, hence the manufacturer has the maximum profit. The optimum $w_{s}^{AN}$ and $\theta_{s}^{AN}$ are determined by ${{\partial \pi_{m - s}^{AN} } \mathord{\left/ {\vphantom {{\partial \pi_{m - s}^{AN} } {\partial w_{s}^{AN} }}} \right. \kern-0pt} {\partial w_{s}^{AN} }} = 0$ and ${{\partial \pi_{m - s}^{AN} } \mathord{\left/ {\vphantom {{\partial \pi_{m - s}^{AN} } {\partial \theta_{s}^{AN} }}} \right. \kern-0pt} {\partial \theta_{s}^{AN} }} = 0$. We therefore generate the Proposition 2.

1.3 Summary of abbreviations in Proposition 2

$$ \left\{ \begin{aligned} \pi_{1 - n}^{AN*} = \left( {\frac{a}{2(2 + \gamma )} - \frac{{c_{m} { + }c_{t} }}{{4 - \gamma^{2} }} + \frac{{\gamma c_{m} }}{{2(4 - \gamma^{2} )(1 - \alpha_{2} )}}} \right)^{2} \hfill \\ \pi_{2 - n}^{AN*} { = }\left( {\frac{a}{2(2 + \gamma )} - \frac{{c_{m} }}{{(4 - \gamma^{2} )(1 - \alpha_{2} )}} + \frac{{\gamma (c_{m} + c_{t} )}}{{2(4 - \gamma^{2} )}}} \right)^{2} \hfill \\ \pi_{m - n}^{AN*} = \frac{{(2 - \gamma )(a - c_{t} - c_{m} )a + (c_{m} + c_{t} )^{2} }}{{2(4 - \gamma^{2} )}} - \frac{{(a + c_{m} + \gamma c_{t} )c_{m} - \gamma }}{{2(2 + \gamma )(1 - \alpha_{2} )}} + \frac{{\left( {c_{m} } \right)^{2} }}{{2(4 - \gamma^{2} )(1 - \alpha_{2} )^{2} }} \hfill \\ \end{aligned} \right.' $$

$$ \left\{ \begin{aligned} \pi_{1 - s}^{AN*} = \frac{1}{{2(2 - \gamma^{2} )}}\left( {\frac{{2(1 - \alpha_{2} )(a - c_{m} - c_{t} ) - \gamma [(1 - \alpha_{2} )a - c_{m} ]}}{{4(1 - \alpha_{2} )}}} \right)^{2} \hfill \\ \pi_{2 - s}^{AN*} = \left( {\frac{{(4 - \gamma^{2} )[(1 - \alpha_{2} )a - c_{m} ] - 2\gamma (1 - \alpha_{2} )(a - c_{m} - c_{t} )}}{{8(2 - \gamma^{2} )(1 - \alpha_{2} )}}} \right)^{2} \hfill \\ \pi_{m - s}^{AN*} = \frac{{(4 - \gamma^{2} )c_{m}^{2} }}{{16(2 - \gamma^{2} )(1 - \alpha_{2} )^{2} }} + \frac{{4(2 - \gamma )(a - c_{m} - c_{t} )a + 4(c_{m} - c_{t} )^{2} - \gamma^{2} a^{2} }}{{16(2 - \gamma^{2} )}} - \frac{{\left( {(4 - \gamma^{2} )a - 2\gamma (a - c_{m} - c_{t} )} \right)c_{m} }}{{8(2 - \gamma^{2} )(1 - \alpha_{2} )}} \hfill \\ \end{aligned} \right.. $$

1.4 Summary of abbreviations in Corollary 2

$$ \begin{aligned} \bar{\alpha }_{2 - s}^{AN} & = \frac{{(4 - \gamma^{2} - 2\gamma )a + (2\gamma - 8 + \gamma^{2} )c_{m} + 2\gamma c_{t} }}{{(4 - \gamma^{2} - 2\gamma )a + 2\gamma (c_{m} + c_{t} )}}, \\ \bar{\gamma }_{1 - s}^{AN} & = \frac{{[(1 - \alpha_{2} )a - c_{m} ] + \sqrt {[(1 - \alpha_{2} )a - c_{m} ]^{2} - 2(a - c_{m} - c_{t} )^{2} (1 - \alpha_{2} )^{2} } }}{{(a - c_{m} - c_{t} )(1 - \alpha_{2} )}}. \\ \end{aligned} $$

Proof of Proposition 3

• (a) For Nash quantity game, the proof is similar to that of Proposition 2 and hence is omitted.

• (b) For Stackelberg quantity game, the proof is similar to that of Proposition 2 and hence is omitted. We only discuss the Hessian matrix. The manufacturer determines the wholesale price $w_{s}^{NA}$ and the cost-sharing coefficient $\theta_{s}^{NA}$, with consideration of the two retailers’ order quantity decisions. We obtain the Hessian matrix $H_{s}^{NA} = {{2c_{t}^{2} } \mathord{\left/ {\vphantom {{2c_{t}^{2} } {(2 - \gamma^{2} )(1 - \alpha_{1} )^{2} }}} \right. \kern-0pt} {(2 - \gamma^{2} )(1 - \alpha_{1} )^{2} }} > 0$, where $\frac{{\partial^{2} \pi_{m - s}^{NA} }}{{\partial^{2} w_{s}^{NA} }} = \frac{{\gamma^{2} (1 - \alpha_{1} )^{2} - 4(2 - \gamma )(1 - \alpha_{1} ) - 4\alpha_{1}^{2} }}{{2(2 - \gamma^{2} )(1 - \alpha_{1} )^{2} }} < 0$, $\frac{{\partial^{2} \pi_{m - s}^{NA} }}{{\partial w_{s}^{NA} \partial \theta_{s}^{NA} }} = \frac{{[2\gamma - (4 - \gamma^{2} )(1 - \alpha_{1} )]c_{t} }}{{2(2 - \gamma^{2} )(1 - \alpha_{1} )}}$, $\frac{{\partial^{2} \pi_{m - s}^{NA} }}{{\partial \theta_{s}^{NA} \partial w_{s}^{NA} }} = \frac{{[2\gamma - (4 - \gamma^{2} )(1 - \alpha_{1} )]c_{t} }}{{2(2 - \gamma^{2} )(1 - \alpha_{1} )}}$, and $\frac{{\partial^{2} \pi_{m - s}^{NA} }}{{\partial^{2} \theta_{s}^{NA} }} = - \frac{{(4 - \gamma^{2} )c_{t}^{2} }}{{2(2 - \gamma^{2} )}}$. On this basis, we know that $H_{s}^{NA}$ is negative definite, therefore the manufacturer has the maximum profit.

1.5 Summary of abbreviations in Proposition 3

$$ \left\{ \begin{aligned} \pi_{1 - n}^{NA*} { = }\left( {\frac{a}{2(2 + \gamma )} - \frac{{c_{m} }}{{(4 - \gamma^{2} )(1 - \alpha_{1} )}} + \frac{{\gamma (c_{m} + c_{t} )}}{{2(4 - \gamma^{2} )}}} \right)^{2} \hfill \\ \pi_{2 - n}^{NA*} = \left( {\frac{a}{2(2 + \gamma )} - \frac{{c_{m} { + }c_{t} }}{{4 - \gamma^{2} }} + \frac{{\gamma c_{m} }}{{2(4 - \gamma^{2} )(1 - \alpha_{1} )}}} \right)^{2} \hfill \\ \pi_{m - n}^{NA*} = \frac{{\left( {2 - \gamma } \right)\left( {a - c_{m} - c_{t} } \right)a + \left( {c_{m} + c_{t} } \right)^{2} }}{{2(4 - \gamma^{2} )}} - \frac{{(a + c_{m} + \gamma c_{t} )c_{m} - \gamma }}{{2\left( {2 + \gamma } \right)(1 - \alpha_{1} )}} + \frac{{\left( {c_{m} } \right)^{2} }}{{2(4 - \gamma^{2} )(1 - \alpha_{1} )^{2} }} \hfill \\ \end{aligned} \right., $$

$$ \left\{ \begin{aligned} \pi_{1 - s}^{NA*} = \frac{1}{{2(2 - \gamma^{2} )}}\left( {\frac{{2[(1 - \alpha_{1} )a - c_{m} ] - \gamma (1 - \alpha_{1} )(a - c_{m} - c_{t} )}}{{4(1 - \alpha_{1} )}}} \right)^{2} \\ \pi_{2 - s}^{NA*} = \left( {\frac{{(4 - \gamma^{2} )(1 - \alpha_{1} )(a - c_{m} - c_{t} ) - 2\gamma [(1 - \alpha_{1} )a - c_{m} ]}}{{8(2 - \gamma^{2} )(1 - \alpha_{1} )}}} \right)^{2} \\ \pi_{m - s}^{NA*} = \left( {\frac{{(1 - \alpha_{1} )a - c_{m} }}{2}} \right)\left( {\frac{{\gamma (1 - \alpha_{1} )(a - c_{m} - c_{t} ) - 2\left( {(1 - \alpha_{1} )a - c_{m} } \right)}}{{4(2 - \gamma^{2} )(1 - \alpha_{1} )^{2} }}} \right) + \left( {\frac{{(2\alpha_{1} + 1)a + c_{m} - c_{t} }}{2}} \right)* \\ \left( {\frac{{(4 - \gamma^{2} )(1 - \alpha_{1} )(a - c_{m} - c_{t} ) - 2\gamma [(1 - \alpha_{1} )a - c_{m} ]}}{{8(2 - \gamma^{2} )(1 - \alpha_{1} )}}} \right) \\ \end{aligned} \right.. $$

1.6 Summary of abbreviations in Corollary 3

$$ \overline{{\alpha_{1 - n}^{NA} }} = \frac{{2a - 4c_{m} - (a - c_{m} - c_{t} )\gamma }}{{2a - (a - c_{m} - c_{t} )\gamma }},\quad \bar{\gamma }_{1 - s}^{NA} = \frac{{2[a(1 - \alpha_{1} ) - c_{m} ] - \sqrt {4[(1 - \alpha_{1} )a - c_{m} ]^{2} - 2(1 - \alpha_{1} )^{2} (a - c_{m} - c_{t} )^{2} } }}{{(a - c_{m} - c_{t} )(1 - \alpha_{1} )}}. $$

Proof of Proposition 4

• (a) For Nash quantity game, the proof is similar to that of Proposition 2 and hence is omitted. We only discuss the Hessian matrix. The manufacturer determines the wholesale price $w_{n}^{AA}$ and the cost-sharing coefficient $\theta_{n}^{AA}$, with consideration of the two retailers’ order quantity decisions. We obtain the Hessian matrix $H_{n}^{AA} = 0$, where $\frac{{\partial^{2} \pi_{m - n}^{AA} }}{{\partial^{2} w_{n}^{AA} }} = - \frac{4}{(\gamma + 2)}$, $\frac{{\partial^{2} \pi_{m - n}^{AA} }}{{\partial w_{n}^{AA} \partial \theta_{n}^{AA} }} = - \frac{{4c_{t} }}{(\gamma + 2)}$, $\frac{{\partial^{2} \pi_{m - n}^{AA} }}{{\partial \theta_{n}^{AA} \partial w_{n}^{AA} }} = - \frac{{4c_{t} }}{(\gamma + 2)}$, and $\frac{{\partial^{2} \pi_{m - n}^{AA} }}{{\partial^{2} \theta_{n}^{AA} }} = - \frac{{4c_{t}^{2} }}{(\gamma + 2)}$. The optimum $w_{n}^{AA}$ and $\theta_{n}^{AA}$ are determined by ${{\partial \pi_{m - n}^{AA} } \mathord{\left/ {\vphantom {{\partial \pi_{m - n}^{AA} } {\partial w_{n}^{AA} }}} \right. \kern-0pt} {\partial w_{n}^{AA} }} = {{\partial \pi_{m - n}^{AA} } \mathord{\left/ {\vphantom {{\partial \pi_{m - n}^{AA} } {\partial \theta_{n}^{AA} }}} \right. \kern-0pt} {\partial \theta_{n}^{AA} }} = 0$.

• (b) For Stackelberg quantity game, the proof is similar to that of Proposition 2 and hence is omitted. We only discuss the Hessian matrix. The manufacturer determines the wholesale price $w_{s}^{AA}$ and the cost-sharing coefficient $\theta_{s}^{AA}$, with consideration of the two retailers’ order quantity decisions. We obtain the Hessian matrix $H_{s}^{AA} = 0$, where $\frac{{\partial^{2} \pi_{m - s}^{AA} }}{{\partial^{2} w_{s}^{AA} }} = \frac{{\gamma^{2} + 4\gamma - 8}}{{2(2 - \gamma^{2} )}}$, $\frac{{\partial^{2} \pi_{m - s}^{AA} }}{{\partial w_{s}^{AA} \partial \theta_{s}^{AA} }} = \frac{{(\gamma^{2} + 4\gamma - 8)c_{t} }}{{2(2 - \gamma^{2} )}}$, $\frac{{\partial^{2} \pi_{m - s}^{AA} }}{{\partial \theta_{s}^{AA} \partial w_{s}^{AA} }} = \frac{{(\gamma^{2} + 4\gamma - 8)c_{t} }}{{2(2 - \gamma^{2} )}}$, and $\frac{{\partial^{2} \pi_{m - s}^{AA} }}{{\partial^{2} \theta_{s}^{AA} }} = \frac{{(\gamma^{2} + 4\gamma - 8)c_{t}^{2} }}{{2(2 - \gamma^{2} )}}$. The optimum $w_{s}^{AA}$ and $\theta_{s}^{AA}$ are determined by ${{\partial \pi_{m - s}^{AA} } \mathord{\left/ {\vphantom {{\partial \pi_{m - s}^{AA} } {\partial w_{s}^{AA} }}} \right. \kern-0pt} {\partial w_{s}^{AA} }} = {{\partial \pi_{m - s}^{AA} } \mathord{\left/ {\vphantom {{\partial \pi_{m - s}^{AA} } {\partial \theta_{s}^{AA} }}} \right. \kern-0pt} {\partial \theta_{s}^{AA} }} = 0$.

1.7 Summary of abbreviations in Proposition 4

$$ \left\{ \begin{aligned} \pi_{i - n}^{AA*} = \left( {\frac{{a - c_{m} - c_{t} }}{2(2 + \gamma )}} \right)^{2} \hfill \\ \pi_{m - n}^{AA*} = \frac{{(a - c_{m} - c_{t} )^{2} }}{2(2 + \gamma )} \hfill \\ \end{aligned} \right.,\quad \left\{ \begin{aligned} \pi_{1 - s}^{AA*} = \frac{1}{{2(2 - \gamma^{2} )}}\left( {\frac{{(2 - \gamma )(a - c_{m} - c_{t} )}}{4}} \right)^{2} \hfill \\ \pi_{2 - s}^{AA*} = \left( {\frac{{(4 - \gamma^{2} - 2\gamma )(a - c_{m} - c_{t} )}}{{8(2 - \gamma^{2} )}}} \right)^{2} \hfill \\ \pi_{m - s}^{AA*} = \frac{{(8 - \gamma^{2} - 4\gamma )(a - c_{m} - c_{t} )^{2} }}{{16(2 - \gamma^{2} )}} \hfill \\ \end{aligned} \right. $$

Proof of proposition 5

(I) We first analyze the equilibrium strategies for RFID adoption when both retailers simultaneously make order quantity decisions.

(i) Given that two retailers’ RFID adoption sequences are simultaneously:

AA will be an equilibrium strategy if and only if $\left\{ \begin{aligned} \pi_{1 - n}^{AA*} \ge \pi_{1 - n}^{NA*} \hfill \\ \pi_{2 - n}^{AA*} \ge \pi_{2 - n}^{AN*} \hfill \\ \end{aligned} \right.$. Thus, we derive that both retailers will adopt RFID when $c_{t} \le \hbox{min} (c_{t1 - n}^{A} ,c_{t2 - n}^{A} )$. We compare the relative sizes of $c_{t1 - n}^{A}$ and $c_{t2 - n}^{A}$, and obtain $c_{t2 - n}^{A} - c_{t1 - n}^{A} = \frac{{(\alpha_{2} - \alpha_{1} )c_{m} }}{{(1 - \alpha_{2} )(1 - \alpha_{1} )}}$. Specifically, if $\alpha_{1} = \alpha_{2} = \alpha$, we derive $c_{t2 - n}^{A} = c_{t1 - n}^{A} = \bar{c}_{t - n}$, leading to that AA is an equilibrium strategy when $c_{t} \in (0,\bar{c}_{t - n} ]$; if $\alpha_{1} < \alpha_{2}$, we derive $c_{t2 - n}^{A} - c_{t1 - n}^{A} > 0$, resulting in that AA is an equilibrium strategy when $c_{t} \in (0,c_{t1 - n}^{A} ]$; if $\alpha_{1} > \alpha_{2}$, we derive $c_{t2 - n}^{A} - c_{t1 - n}^{A} < 0$, bringing about that AA is an equilibrium strategy when $c_{t} \in (0,c_{t2 - n}^{A} ]$. Where $c_{t1 - n}^{A} = {{\alpha_{1} c_{m} } \mathord{\left/ {\vphantom {{\alpha_{1} c_{m} } {(1 - \alpha_{1} )}}} \right. \kern-0pt} {(1 - \alpha_{1} )}}$, $c_{t2 - n}^{A} = {{\alpha_{2} c_{m} } \mathord{\left/ {\vphantom {{\alpha_{2} c_{m} } {(1 - \alpha_{2} )}}} \right. \kern-0pt} {(1 - \alpha_{2} )}}$ and $\overline{c}_{t - n} = {{\alpha c_{m} } \mathord{\left/ {\vphantom {{\alpha c_{m} } {(1 - \alpha )}}} \right. \kern-0pt} {(1 - \alpha )}}$.

AN will be an equilibrium strategy if $\left\{ \begin{aligned} \pi_{1 - n}^{AN*} \ge \pi_{1 - n}^{NN*} \hfill \\ \pi_{2 - n}^{AN*} > \pi_{2 - n}^{AA*} \hfill \\ \end{aligned} \right.$. Thus, we derive that only retailer 1 will adopt RFID when $c_{t2 - n}^{A} < c_{t} \le c_{t1 - n}^{N}$. We compare the relative sizes of $c_{t2 - n}^{A}$ and $c_{t1 - n}^{N}$, and then obtain $c_{t1 - n}^{N} - c_{t2 - n}^{A} = \left( {\frac{{(2 + \gamma )(2 - \gamma )(1 - \alpha_{1} )a}}{{4\left( {(\alpha_{1} - \alpha_{2} )^{2} + (2 - \gamma )(1 - \alpha_{1} )(1 - \alpha_{2} )} \right)}} + \frac{{c_{m} }}{{(1 - \alpha_{1} )(1 - \alpha_{2} )}}} \right)(\alpha_{1} - \alpha_{2} )$. Specifically, if $\alpha_{1} = \alpha_{2} = \alpha$, we derive $c_{t1 - n}^{N} - c_{t2 - n}^{A} = 0$, leading to that AN will not exist; if $\alpha_{1} < \alpha_{2}$, we derive $c_{t1 - n}^{N} - c_{t2 - n}^{A} < 0$, resulting in that AN will not exist; if $\alpha_{1} > \alpha_{2}$, we derive $c_{t1 - n}^{N} - c_{t2 - n}^{A} > 0$, bringing about that AN is an equilibrium strategy when $c_{t} \in (c_{t2 - n}^{A} ,c_{t1 - n}^{N} ]$. Where $c_{t1 - n}^{N} = \left( {{{2v_{n} } \mathord{\left/ {\vphantom {{2v_{n} } {(1 - \alpha_{1} )}}} \right. \kern-0pt} {(1 - \alpha_{1} )}} - {{\gamma v_{n} } \mathord{\left/ {\vphantom {{\gamma v_{n} } {(1 - \alpha_{2} )}}} \right. \kern-0pt} {(1 - \alpha_{2} )}} - {{(2 - \gamma )} \mathord{\left/ {\vphantom {{(2 - \gamma )} 2}} \right. \kern-0pt} 2}} \right)a + {{\alpha_{1} c_{m} } \mathord{\left/ {\vphantom {{\alpha_{1} c_{m} } {(1 - \alpha_{1} )}}} \right. \kern-0pt} {(1 - \alpha_{1} )}}$.

NA will be an equilibrium strategy if $\left\{ \begin{aligned} \pi_{1 - n}^{NA*} > \pi_{1 - n}^{AA*} \hfill \\ \pi_{2 - n}^{NA*} \ge \pi_{2 - n}^{NN*} \hfill \\ \end{aligned} \right.$. Thus, we derive that only retailer 2 will adopt RFID when $c_{t1 - n}^{A} < c_{t} \le c_{t2 - n}^{N}$. We compare the relative sizes of $c_{t1 - n}^{A}$ and $c_{t2 - n}^{N}$, and then obtain $c_{t2 - n}^{N} - c_{t1 - n}^{A} = \left( {\frac{{(2 + \gamma )(2 - \gamma )(1 - \alpha_{2} )a}}{{4\left( {(\alpha_{2} - \alpha_{1} )^{2} + (2 - \gamma )(1 - \alpha_{1} )(1 - \alpha_{2} )} \right)}} + \frac{{c_{m} }}{{(1 - \alpha_{1} )(1 - \alpha_{2} )}}} \right)(\alpha_{2} - \alpha_{1} )$. Specifically, if $\alpha_{1} = \alpha_{2} = \alpha$, we derive $c_{t2 - n}^{N} - c_{t1 - n}^{A} = 0$, leading to that NA will not exist; if $\alpha_{1} > \alpha_{2}$, we derive $c_{t2 - n}^{N} - c_{t1 - n}^{A} < 0$, resulting in that NA will not exist; if $\alpha_{1} < \alpha_{2}$, we derive $c_{t2 - n}^{N} - c_{t1 - n}^{A} > 0$, bringing about that NA is an equilibrium strategy when $c_{t} \in (c_{t1 - n}^{A} ,c_{t2 - n}^{N} ]$. Where $c_{t2 - n}^{N} = \left( {{{2v_{n} } \mathord{\left/ {\vphantom {{2v_{n} } {(1 - \alpha_{2} )}}} \right. \kern-0pt} {(1 - \alpha_{2} )}} - {{\gamma v_{n} } \mathord{\left/ {\vphantom {{\gamma v_{n} } {(1 - \alpha_{1} )}}} \right. \kern-0pt} {(1 - \alpha_{1} )}} - {{(2 - \gamma )} \mathord{\left/ {\vphantom {{(2 - \gamma )} 2}} \right. \kern-0pt} 2}} \right)a + {{\alpha_{2} c_{m} } \mathord{\left/ {\vphantom {{\alpha_{2} c_{m} } {(1 - \alpha_{2} )}}} \right. \kern-0pt} {(1 - \alpha_{2} )}}$.

NN will be an equilibrium strategy if $\left\{ \begin{aligned} \pi_{1 - n}^{NN*} > \pi_{1 - n}^{AN*} \hfill \\ \pi_{2 - n}^{NN*} > \pi_{2 - n}^{NA*} \hfill \\ \end{aligned} \right.$. Thus, we derive that both retailers will forgo RFID adoption when $c_{t} > \hbox{max} (c_{t1 - n}^{N} ,c_{t2 - n}^{N} )$. We compare the relative sizes of $c_{t1 - n}^{N}$ and $c_{t2 - n}^{N}$, and then obtain $c_{t2 - n}^{N} - c_{t1 - n}^{N} = \frac{{(2av_{n} + av_{n} \gamma + \alpha_{2} c_{m} )(\alpha_{2} - \alpha_{1} )}}{{(1 - \alpha_{2} )(1 - \alpha_{1} )}}$. Specifically, if $\alpha_{1} = \alpha_{2} = \alpha$, we derive that $c_{t2 - n}^{N} = c_{t1 - n}^{N} = \bar{c}_{t - n}$, leading to that NN is an equilibrium strategy when $c_{t} \in (\bar{c}_{t - n} , + \infty )$; if $\alpha_{1} < \alpha_{2}$, we can derive $c_{t2 - n}^{N} - c_{t1 - n}^{N} > 0$, resulting in that NN is an equilibrium strategy when $c_{t} \in (c_{t2 - n}^{N} , + \infty )$; if $\alpha_{1} > \alpha_{2}$, we derive $c_{t2 - n}^{N} - c_{t1 - n}^{N} < 0$, bringing about that NN is an equilibrium strategy when $c_{t} \in (c_{t1 - n}^{N} , + \infty )$.

Consequently, we obtain the equilibrium strategies for RFID adoption when both retailers simultaneously make decisions on RFID adoption and order quantity. If $\alpha_{1} = \alpha_{2} = \alpha$: AA is an equilibrium strategy when $c_{t} \in (0,\bar{c}_{t - n} ]$, NN is an equilibrium strategy when $c_{t} \in (\bar{c}_{t - n} , + \infty )$; if $\alpha_{1} < \alpha_{2}$, AA is an equilibrium strategy when $c_{t} \in (0,c_{t1 - n}^{A} ]$, NA is an equilibrium strategy when $c_{t} \in (c_{t1 - n}^{A} ,c_{t2 - n}^{N} ]$, NN is an equilibrium strategy when $c_{t} \in (c_{t2 - n}^{N} , + \infty )$; if $\alpha_{1} > \alpha_{2}$, AA is an equilibrium strategy when $c_{t} \in (0,c_{t2 - n}^{A} ]$, AN is an equilibrium strategy when $c_{t} \in (c_{t2 - n}^{A} ,c_{t1 - n}^{N} ]$, NN is an equilibrium strategy when $c_{t} \in (c_{t1 - n}^{N} , + \infty )$. Thus, we obtain the results of proposition 5.

(ii) Given that two retailers’ RFID adoption sequences are sequentially:

If the retailer 1 has adopted RFID: AA will be an equilibrium strategy if the retailer 2 adopts RFID, AN will be an equilibrium strategy if the retailer 2 refuses to adopt RFID. We take $\Delta_{2 - n}^{A} = \pi_{2 - n}^{AA*} - \pi_{2 - n}^{AN*}$ as the retailer 2’s profit variation between AA and AN, and obtain that the retailer 2 adopts RFID when $c_{t} \in (0,c_{t2 - n}^{A} ]$ while forgoes RFID adoption when $c_{t} \in (c_{t2 - n}^{A} , + \infty )$.

If the retailer 1 has not adopted RFID: NA will be an equilibrium strategy if the retailer 2 adopts RFID, NN will be an equilibrium strategy if the retailer 2 refuses to adopt RFID. We take $\Delta_{2 - n}^{N} = \pi_{2 - n}^{NA*} - \pi_{2 - n}^{NN*}$ as the retailer 2’s profit variation between NA and NN, and obtain that the retailer 2 adopts RFID when $c_{t} \in (0,c_{t2 - n}^{N} ]$ while forgoes RFID adoption when $c_{t} \in (c_{t2 - n}^{N} , + \infty )$.

Using backward induction, we first characterize the retailer 2’s RFID adoption decisions. According to comparing the relative sizes of $c_{t2 - n}^{A}$ and $c_{t2 - n}^{N}$, we obtain $c_{t2 - n}^{N} - c_{t2 - n}^{A} = \frac{{(\alpha_{2} - \alpha_{1} )(1 - \alpha_{2} )(4 - \gamma^{2} )a}}{{4\left( {(\alpha_{1} - \alpha_{2} )^{2} + (2 - \gamma )(1 - \alpha_{1} )(1 - \alpha_{2} )} \right)}}$. Specifically, if $\alpha_{1} = \alpha_{2} = \alpha$, we derive $c_{t2 - n}^{N} = c_{t2 - n}^{A} = \bar{c}_{t - n}$, leading to that the retailer 2 always adopts RFID when $c_{t} \in (0,\bar{c}_{t - n} ]$ while forgoes RFID adoption when $c_{t} \in (\bar{c}_{t - n} , + \infty )$, regardless of whether the retailer 1 adopts RFID; if $\alpha_{1} < \alpha_{2}$, we derive $c_{t2 - n}^{N} - c_{t2 - n}^{A} > 0$, hence, the retailer 2 always adopts RFID when $c_{t} \in (0,c_{t2 - n}^{A} ]$, the two retailers have the opposite decisions on RFID adoption when $c_{t} \in (c_{t2 - n}^{A} ,c_{t2 - n}^{N} ]$, and the retailer 2 forgoes RFID adoption when $c_{t} \in (c_{t2 - n}^{N} , + \infty )$; if $\alpha_{1} > \alpha_{2}$, we derive $c_{t2 - n}^{N} - c_{t2 - n}^{A} < 0$, hence, the retailer 2 always adopts RFID when $c_{t} \in (0,c_{t2 - n}^{N} ]$, the two retailers have the same decisions on RFID adoption when $c_{t} \in (c_{t2 - n}^{N} ,c_{t2 - n}^{A} ]$, and the retailer 2 forgoes RFID adoption when $c_{t} \in (c_{t2 - n}^{A} , + \infty )$.

We turn to deal with the equilibrium RFID adoption strategies.

If $\alpha_{1} = \alpha_{2} = \alpha$: given $c_{t} \in (0,\bar{c}_{t - n} ]$, the retailer 2 always adopts RFID. Under such a circumstance, we take $\Delta_{1 - n}^{A} = \pi_{1 - n}^{AA*} - \pi_{1 - n}^{NA*}$ as the retailer 1’s profit variation between AA and NA, and derive that the retailer 1 adopts RFID when $c_{t} \in (0,\bar{c}_{t - n} ]$, leading to that AA is an equilibrium strategy when $c_{t} \in (0,\bar{c}_{t - n} ]$.

Given $c_{t} \in (\bar{c}_{t - n} , + \infty )$, the retailer 2 always refuses to adopt RFID. Under such a circumstance, we take $\Delta_{1 - n}^{N} = \pi_{1 - n}^{AN*} - \pi_{1 - n}^{NN*}$ as the retailer 1’s profit variation between AN and NN, and derive that the retailer 1 adopts RFID when $c_{t} \in (\bar{c}_{t - n} , + \infty )$, resulting in that NN is an equilibrium strategy when $c_{t} \in (\bar{c}_{t - n} , + \infty )$.

If $\alpha_{1} < \alpha_{2}$: given $c_{t} \in (0,c_{t2 - n}^{A} ]$, the retailer 2 always adopts RFID. Under such a circumstance, we take $\Delta_{1 - n}^{A} = \pi_{1 - n}^{AA*} - \pi_{1 - n}^{NA*}$ as the retailer 1’s profit variation between AA and NA, and derive that the retailer 1 adopts RFID when $c_{t} \in (0,c_{t1 - n}^{A} ]$ while refuses to adopt RFID when $c_{t} \in (c_{t1 - n}^{A} , + \infty )$. We compare the relative sizes of $c_{t1 - n}^{A}$ and $c_{t2 - n}^{A}$, and then obtain $c_{t2 - n}^{A} - c_{t1 - n}^{A} = \frac{{(\alpha_{2} - \alpha_{1} )c_{m} }}{{(1 - \alpha_{2} )(1 - \alpha_{1} )}} > 0$, leading to that AA is an equilibrium strategy when $c_{t} \in (0,c_{t1 - n}^{A} ]$ and NA is an equilibrium strategy when $c_{t} \in (c_{t1 - n}^{A} ,c_{t2 - n}^{A} ]$.

Given $c_{t} \in (c_{t2 - n}^{A} ,c_{t2 - n}^{N} ]$, the two retailers have the opposite decisions on RFID adoption. Under such a circumstance, we take $\Delta_{1 - n}^{NA} = \pi_{1 - n}^{AN*} - \pi_{1 - n}^{NA*}$ as the retailer 1’s profit variation between AN and NA, and derive that the retailer 1 adopts RFID when $c_{t} \in (0,c_{t1 - n}^{NA} ]$ while refuses to adopt RFID when $c_{t} \in (c_{t1 - n}^{NA} , + \infty )$. We compare the relative sizes of $c_{t2 - n}^{A}$ and $c_{t1 - n}^{NA}$, and then obtain $c_{t2 - n}^{A} - c_{t1 - n}^{NA} = \frac{{2(\alpha_{2} - \alpha_{1} )c_{m} }}{{(2 + \gamma )(1 - \alpha_{1} )(1 - \alpha_{2} )}} > 0$, resulting in that NA is an equilibrium strategy when $c_{t} \in (c_{t2 - n}^{A} ,c_{t2 - n}^{N} ]$. Where $c_{t1 - n}^{NA} = \frac{{\alpha_{2} c_{m} }}{{(1 - \alpha_{2} )}} + \frac{{2(\alpha_{1} - \alpha_{2} )c_{m} }}{{(2 + \gamma )(1 - \alpha_{1} )(1 - \alpha_{2} )}}$.

Given $c_{t} \in (c_{t2 - n}^{N} , + \infty )$, the retailer 2 always refuses to adopt RFID. Under such a circumstance, we take $\Delta_{1 - n}^{N} = \pi_{1 - n}^{AN*} - \pi_{1 - n}^{NN*}$ as the retailer 1’s profit variation between AN and NN, and derive that the retailer 1 adopts RFID when $c_{t} \in (0,c_{t1 - n}^{N} ]$ while refuses to adopt RFID when $c_{t} \in (c_{t1 - n}^{N} , + \infty )$. We compare the relative sizes of $c_{t2 - n}^{N}$ and $c_{t1 - n}^{N}$, and then obtain $c_{t2 - n}^{N} - c_{t1 - n}^{N} = \frac{{(2av_{n} + av_{n} \gamma + \alpha_{2} c_{m} )(\alpha_{2} - \alpha_{1} )}}{{(1 - \alpha_{2} )(1 - \alpha_{1} )}} > 0$, bring about that NN is an equilibrium strategy when $c_{t} \in (c_{t2 - n}^{N} , + \infty )$.

If $\alpha_{1} > \alpha_{2}$: given $c_{t} \in (0,c_{t2 - n}^{N} ]$, the retailer 2 always adopts RFID. Under such a circumstance, we take $\Delta_{1 - n}^{A} = \pi_{1 - n}^{AA*} - \pi_{1 - n}^{NA*}$ as the retailer 1’s profit variation between AA and NA, and derive that the retailer 1 adopts RFID when $c_{t} \in (0,c_{t1 - n}^{A} ]$ while refuses to adopt RFID when $c_{t} \in (c_{t1 - n}^{A} , + \infty )$. We compare the relative sizes of $c_{t2 - n}^{N}$ and $c_{t1 - n}^{A}$, and then obtain $c_{t2 - n}^{N} - c_{t1 - n}^{A} = \frac{{(2 - \gamma )(2 + \gamma )(1 - \alpha_{2} )(\alpha_{2} - \alpha_{1} )a}}{{4\left[ {(1 - \alpha_{1} )^{2} - \gamma (1 - \alpha_{1} )(1 - \alpha_{2} ) + (1 - \alpha_{2} )^{2} } \right]}} + \left( {\frac{{\alpha_{2} - \alpha_{1} }}{{(1 - \alpha_{1} )(1 - \alpha_{2} )}}} \right)c_{m} < 0$, leading to that AA is an equilibrium strategy when $c_{t} \in (0,c_{t2 - n}^{N} ]$.

Given $c_{t} \in (c_{t2 - n}^{N} ,c_{t2 - n}^{A} ]$, the two retailers have the same decisions on RFID adoption. Under such a circumstance, we take $\Delta_{1 - n}^{AN} = \pi_{1 - n}^{AA*} - \pi_{1 - n}^{NN*}$ as the retailer 1’s profit variation between AA and NN, and derive that the retailer 1 adopts RFID when $c_{t} \in (0,c_{t1 - n}^{AN} ]$ while refuses to adopt RFID when $c_{t} \in (c_{t1 - n}^{AN} , + \infty )$. We compare the relative size of $c_{t1 - n}^{AN}$ and $c_{t2 - n}^{A}$. With $c_{t1 - n}^{AN} - c_{t2 - n}^{A} = \left( {\frac{{2(1 - \alpha_{2} )[2v_{n} - (1 - \alpha_{1} )] - \gamma (1 - \alpha_{1} )[2v_{n} - (1 - \alpha_{2} )]}}{{(2 - \gamma )(1 - \alpha_{1} )(1 - \alpha_{2} )}}} \right)a + \frac{{2(\alpha_{1} - \alpha_{2} )c_{m} }}{{(2 - \gamma )(1 - \alpha_{1} )(1 - \alpha_{2} )}} > 0$, we obtain that AA is an equilibrium strategy when $c_{t} \in (c_{t2 - n}^{N} ,c_{t2 - n}^{A} ]$. Where $c_{t1 - n}^{AN} = \left( {\frac{{2(1 - \alpha_{2} )[2v_{n} - (1 - \alpha_{1} )] - \gamma (1 - \alpha_{1} )[2v_{n} - (1 - \alpha_{2} )]}}{{(2 - \gamma )(1 - \alpha_{1} )(1 - \alpha_{2} )}}} \right)a + \left( {\frac{{2(1 - \alpha_{2} )\alpha_{1} - \gamma (1 - \alpha_{1} )\alpha_{2} }}{{(2 - \gamma )(1 - \alpha_{1} )(1 - \alpha_{2} )}}} \right)c_{m}$.

Given $c_{t} \in (c_{t2 - n}^{A} , + \infty )$, the retailer 2 always refuses to adopt RFID. Under such a circumstance, we take $\Delta_{1 - n}^{N} = \pi_{1 - n}^{AN*} - \pi_{1 - n}^{NN*}$ as the retailer 1’s profit variation between AN and NN, and derive that the retailer 1 adopts RFID when $c_{t} \in (0,c_{t1 - n}^{N} ]$ while refuses to adopt RFID when $c_{t} \in (c_{t1 - n}^{N} , + \infty )$. We compare the relative sizes of $c_{t1 - n}^{N}$ and $c_{t2 - n}^{A}$. With $c_{t1 - n}^{N} - c_{t2 - n}^{A} = \left( {\frac{{(2 - \gamma )(2 + \gamma )(1 - \alpha_{1} )a}}{{4\left[ {(1 - \alpha_{1} )^{2} - \gamma (1 - \alpha_{1} )(1 - \alpha_{2} ) + (1 - \alpha_{2} )^{2} } \right]}} + \frac{{c_{m} }}{{(1 - \alpha_{1} )(1 - \alpha_{2} )}}} \right)(\alpha_{1} - \alpha_{2} ) > 0$, we obtain that AN is an equilibrium strategy when $c_{t} \in (c_{t2 - n}^{A} ,c_{t1 - n}^{N} ]$ and NN is an equilibrium strategy when $c_{t} \in (c_{t1 - n}^{N} , + \infty )$.

Consequently, we obtain the equilibrium strategies for RFID adoption when the two retailers sequentially make decisions on RFID adoption and simultaneously make decisions on order quantity. If $\alpha_{1} = \alpha_{2} = \alpha$: AA is an equilibrium strategy when $c_{t} \in (0,\bar{c}_{t - n} ]$, NN is an equilibrium strategy when $c_{t} \in (\bar{c}_{t - n} , + \infty )$; if $\alpha_{1} < \alpha_{2}$, AA is an equilibrium strategy when $c_{t} \in (0,c_{t1 - n}^{A} ]$, NA is an equilibrium strategy when $c_{t} \in (c_{t1 - n}^{A} ,c_{t2 - n}^{N} ]$, NN is an equilibrium strategy when $c_{t} \in (c_{t2 - n}^{N} , + \infty )$; if $\alpha_{1} > \alpha_{2}$, AA is an equilibrium strategy when $c_{t} \in (0,c_{t2 - n}^{A} ]$, AN is an equilibrium strategy when $c_{t} \in (c_{t2 - n}^{A} ,c_{t1 - n}^{N} ]$, NN is an equilibrium strategy when $c_{t} \in (c_{t1 - n}^{N} , + \infty )$. Thus, we find that when both retailers simultaneously make order quantity decisions, whether the two retailers adopt RFID simultaneously or sequentially does not affect the equilibrium strategies for RFID adoption.

(II) We then analyze the equilibrium strategies for RFID adoption when both retailers sequentially make order quantity decisions.

(i) Given that two retailers’ RFID adoption sequences are simultaneously:

AA will be an equilibrium strategy if and only if $\left\{ \begin{aligned} \pi_{1 - s}^{AA*} \ge \pi_{1 - s}^{NA*} \hfill \\ \pi_{2 - s}^{AA*} \ge \pi_{2 - s}^{AN*} \hfill \\ \end{aligned} \right.$. Thus, we derive that both retailers will adopt RFID when $c_{t} \le \hbox{min} (c_{t1 - s}^{A} ,c_{t2 - s}^{A} )$. We compare the relative size of $c_{t1 - s}^{A}$ and $c_{t2 - s}^{A}$, and then obtain $c_{t2 - s}^{A} - c_{t1 - s}^{A} = \frac{{(\alpha_{2} - \alpha_{1} )c_{m} }}{{(1 - \alpha_{2} )(1 - \alpha_{1} )}}$. Specifically, if $\alpha_{1} = \alpha_{2} = \alpha$, we derive $c_{t2 - s}^{A} = c_{t1 - s}^{A} = \bar{c}_{t - s}$, leading to that AA is an equilibrium strategy when $c_{t} \in (0,\bar{c}_{t - s} ]$; if $\alpha_{1} < \alpha_{2}$, we derive $c_{t2 - s}^{A} - c_{t1 - s}^{A} > 0$, resulting in that AA is an equilibrium strategy when $c_{t} \in (0,c_{t1 - s}^{A} ]$; if $\alpha_{1} > \alpha_{2}$, we derive $c_{t2 - s}^{A} - c_{t1 - s}^{A} < 0$, bringing about that AA is an equilibrium strategy when $c_{t} \in (0,c_{t2 - s}^{A} ]$. Where $c_{t1 - s}^{A} = {{\alpha_{1} c_{m} } \mathord{\left/ {\vphantom {{\alpha_{1} c_{m} } {(1 - \alpha_{1} )}}} \right. \kern-0pt} {(1 - \alpha_{1} )}}$, $c_{t2 - s}^{A} = {{\alpha_{2} c_{m} } \mathord{\left/ {\vphantom {{\alpha_{2} c_{m} } {(1 - \alpha_{2} )}}} \right. \kern-0pt} {(1 - \alpha_{2} )}}$ and $\bar{c}_{t - s} = {{\alpha c_{m} } \mathord{\left/ {\vphantom {{\alpha c_{m} } {(1 - \alpha )}}} \right. \kern-0pt} {(1 - \alpha )}}$.

AN will be an equilibrium strategy if $\left\{ \begin{aligned} \pi_{1 - s}^{AN*} \ge \pi_{1 - s}^{NN*} \hfill \\ \pi_{2 - s}^{AN*} \ge \pi_{2 - s}^{AA*} \hfill \\ \end{aligned} \right.$. Thus, we derive that only retailer 1 will adopt RFID when $c_{t2 - s}^{A} < c_{t} \le c_{t1 - s}^{N}$. We compare the relative sizes of $c_{t2 - s}^{A}$ and $c_{t1 - s}^{N}$, as $c_{t1 - s}^{N} - c_{t2 - s}^{A} = \left( {\frac{{(8 - 4\gamma^{2} )(1 - \alpha_{1} )a}}{{2\left[ {(4 - \gamma^{2} )(1 - \alpha_{1} )^{2} - 4\gamma (1 - \alpha_{1} )(1 - \alpha_{2} ) + 4(1 - \alpha_{2} )^{2} } \right]}} + \frac{{c_{m} }}{{(1 - \alpha_{1} )(1 - \alpha_{2} )}}} \right)(\alpha_{1} - \alpha_{2} )$, Specifically, if $\alpha_{1} = \alpha_{2} = \alpha$, we derive $c_{t1 - s}^{N} - c_{t2 - s}^{A} = 0$, leading to that AN will not exist; if $\alpha_{1} < \alpha_{2}$, we derive $c_{t1 - s}^{N} - c_{t2 - s}^{A} < 0$, resulting in that AN will not exist; if $\alpha_{1} > \alpha_{2}$, we derive $c_{t1 - s}^{N} - c_{t2 - s}^{A} > 0$, bringing about that AN is an equilibrium strategy when $c_{t} \in (c_{t2 - s}^{A} ,c_{t1 - s}^{N} ]$. Where $c_{t1 - s}^{N} = \left( {{{2v_{s} } \mathord{\left/ {\vphantom {{2v_{s} } {(1 - \alpha_{1} )}}} \right. \kern-0pt} {(1 - \alpha_{1} )}} - {{\gamma v_{s} } \mathord{\left/ {\vphantom {{\gamma v_{s} } {(1 - \alpha_{2} )}}} \right. \kern-0pt} {(1 - \alpha_{2} )}} - {{(2 - \gamma )} \mathord{\left/ {\vphantom {{(2 - \gamma )} 2}} \right. \kern-0pt} 2}} \right)a + {{\alpha_{1} c_{m} } \mathord{\left/ {\vphantom {{\alpha_{1} c_{m} } {(1 - \alpha_{1} )}}} \right. \kern-0pt} {(1 - \alpha_{1} )}}$.

NA will be an equilibrium strategy if $\left\{ \begin{aligned} \pi_{1 - s}^{NA*} \ge \pi_{1 - s}^{AA*} \hfill \\ \pi_{2 - s}^{NA*} \ge \pi_{2 - s}^{NN*} \hfill \\ \end{aligned} \right.$. Thus, we derive that only retailer 2 will adopt RFID when $c_{t1 - s}^{A} < c_{t} \le c_{t2 - s}^{N}$. We compare the relative sizes of $c_{t1 - s}^{A}$ and $c_{t2 - s}^{N}$, as $c_{t2 - s}^{N} - c_{t1 - s}^{A} = \left( {\frac{{(16 - 8\gamma^{2} )(1 - \alpha_{2} )a}}{{(4 - \gamma^{2} )[(4 - \gamma^{2} )(1 - \alpha_{1} )^{2} - 4\gamma (1 - \alpha_{1} )(1 - \alpha_{2} ) + 4(1 - \alpha_{2} )^{2} ]}} + \frac{{c_{m} }}{{(1 - \alpha_{1} )(1 - \alpha_{2} )}}} \right)(\alpha_{2} - \alpha_{1} )$. Specifically, if $\alpha_{1} = \alpha_{2} = \alpha$, we derive $c_{t2 - s}^{N} - c_{t1 - s}^{A} = 0$, leading to that NA will not exist; if $\alpha_{1} > \alpha_{2}$, we derive $c_{t2 - s}^{N} - c_{t1 - s}^{A} < 0$, resulting in that NA will not exist; if $\alpha_{1} < \alpha_{2}$, we derive $c_{t2 - s}^{N} - c_{t1 - s}^{A} > 0$, bringing about that NA is an equilibrium strategy when $c_{t} \in (c_{t1 - s}^{A} ,c_{t2 - s}^{N} ]$.

Where $c_{t2 - s}^{N} = \left( {{{2v_{s} } \mathord{\left/ {\vphantom {{2v_{s} } {(1 - \alpha_{2} )}}} \right. \kern-0pt} {(1 - \alpha_{2} )}} - {{4\gamma v_{s} } \mathord{\left/ {\vphantom {{4\gamma v_{s} } {(1 - \alpha_{1} )(4 - \gamma^{2} )}}} \right. \kern-0pt} {(1 - \alpha_{1} )(4 - \gamma^{2} )}} - {{(4 - \gamma^{2} - 2\gamma )} \mathord{\left/ {\vphantom {{(4 - \gamma^{2} - 2\gamma )} {(4 - \gamma^{2} )}}} \right. \kern-0pt} {(4 - \gamma^{2} )}}} \right)a + {{\alpha_{2} c_{m} } \mathord{\left/ {\vphantom {{\alpha_{2} c_{m} } {(1 - \alpha_{2} )}}} \right. \kern-0pt} {(1 - \alpha_{2} )}}$.

NN will be an equilibrium strategy if $\left\{ \begin{aligned} \pi_{1 - s}^{NN*} \ge \pi_{1 - s}^{AN*} \hfill \\ \pi_{2 - s}^{NN*} \ge \pi_{2 - s}^{NA*} \hfill \\ \end{aligned} \right.$. Thus, we derive that both retailers will forgo RFID adoption when $c_{t} > \hbox{max} (c_{t1 - s}^{N} ,c_{t2 - s}^{N} )$. We compare the relative sizes of $c_{t1 - s}^{N}$ and $c_{t2 - s}^{N}$, as $c_{t2 - s}^{N} - c_{t1 - s}^{N} = \frac{{(4 - \gamma^{2} )\left[ {(1 - \alpha_{1} )^{2} - (1 - \alpha_{2} )^{2} } \right]a}}{{(4 - \gamma^{2} )(1 - \alpha_{1} )^{2} - 4\gamma (1 - \alpha_{1} )(1 - \alpha_{2} ) + 4(1 - \alpha_{2} )^{2} }} + \frac{{(\alpha_{2} - \alpha_{1} )c_{m} }}{{(1 - \alpha_{1} )(1 - \alpha_{2} )}}$. Specifically, if $\alpha_{1} = \alpha_{2} = \alpha$, we derive $c_{t2 - s}^{N} = c_{t1 - s}^{N} = \bar{c}_{t - s}$, leading to that NN is an equilibrium strategy when $c_{t} \in (\bar{c}_{t - s} , + \infty )$; if $\alpha_{1} < \alpha_{2}$, we derive $c_{t2 - s}^{N} - c_{t1 - s}^{N} > 0$, resulting in that NN is an equilibrium strategy when $c_{t} \in (c_{t2 - s}^{N} , + \infty )$; i f $\alpha_{1} > \alpha_{2}$, we derive $c_{t2 - s}^{N} - c_{t1 - s}^{N} < 0$, bringing about that NN is an equilibrium strategy when $c_{t} \in (c_{t1 - s}^{N} , + \infty )$.

Consequently, we obtain the equilibrium strategies for RFID adoption when both retailers simultaneously make decisions on RFID adoption and sequentially make decisions on order quantity. If $\alpha_{1} = \alpha_{2} = \alpha$: AA is an equilibrium strategy when $c_{t} \in (0,\bar{c}_{t - s} ]$, NN is an equilibrium strategy when $c_{t} \in (\bar{c}_{t - s} , + \infty )$; if $\alpha_{1} < \alpha_{2}$, AA is an equilibrium strategy when $c_{t} \in (0,c_{t1 - s}^{A} ]$, NA is an equilibrium strategy when $c_{t} \in (c_{t1 - s}^{A} ,c_{t2 - s}^{N} ]$, NN is an equilibrium strategy when $c_{t} \in (c_{t2 - s}^{N} , + \infty )$; if $\alpha_{1} > \alpha_{2}$, AA is an equilibrium strategy when $c_{t} \in (0,c_{t2 - s}^{A} ]$, AN is an equilibrium strategy when $c_{t} \in (c_{t2 - s}^{A} ,c_{t1 - s}^{N} ]$, NN is an equilibrium strategy when $c_{t} \in (c_{t1 - s}^{N} , + \infty )$. Thus, we obtain the results of proposition 5.

(ii) Given that two retailers’ RFID adoption sequences are sequentially:

If the retailer 1 has adopted RFID: AA will be an equilibrium strategy if the retailer 2 adopts RFID, AN will be an equilibrium strategy if the retailer 2 refuses to adopt RFID. We take $\Delta_{2 - s}^{A} = \pi_{2 - s}^{AA*} - \pi_{2 - s}^{AN*}$ as the retailer 2’s profit variation between AA and AN, and obtain that the retailer 2 adopts RFID when $c_{t} \in (0,c_{t2 - s}^{A} ]$ while forgoes RFID adoption when $c_{t} \in (c_{t2 - s}^{A} , + \infty )$.

If the retailer 1 has not adopted RFID: NA will be an equilibrium strategy if the retailer 2 adopts RFID, NN will be an equilibrium strategy if the retailer 2 refuses to adopt RFID. We take $\Delta_{2 - s}^{N} = \pi_{2 - s}^{NA*} - \pi_{2 - s}^{NN*}$ as the retailer 2’s profit variation between NA and NN, and obtain that the retailer 2 adopts RFID when $c_{t} \in (0,c_{t2 - s}^{N} ]$ while forgoes RFID adoption when $c_{t} \in (c_{t2 - s}^{N} , + \infty )$.

Using backward induction, we first characterize the retailer 2’s RFID adoption decisions. According to comparing the relative sizes of $c_{t2 - s}^{A}$ and $c_{t2 - s}^{N}$, we obtain $c_{t2 - s}^{N} - c_{t2 - s}^{A} = \frac{{(16 - 8\gamma^{2} )(1 - \alpha_{2} )(\alpha_{2} - \alpha_{1} )a}}{{(4 - \gamma^{2} )[(4 - \gamma^{2} )(1 - \alpha_{1} )^{2} - 4\gamma (1 - \alpha_{1} )(1 - \alpha_{2} ) + 4(1 - \alpha_{2} )^{2} ]}}$. Specifically, if $\alpha_{1} = \alpha_{2} = \alpha$, we derive $c_{t2 - s}^{N} = c_{t2 - s}^{A} = \bar{c}_{t - s}$, leading to that the retailer 2 always adopts RFID when $c_{t} \in (0,\bar{c}_{t - s} ]$ while forgoes RFID adoption when $c_{t} \in (\bar{c}_{t - s} , + \infty )$, regardless of whether the retailer 1 adopts RFID; if $\alpha_{1} < \alpha_{2}$, we derive $c_{t2 - s}^{N} - c_{t2 - s}^{A} > 0$, hence, the retailer 2 always adopts RFID when $c_{t} \in (0,c_{t2 - s}^{A} ]$, the two retailers have the opposite decisions on RFID adoption when $c_{t} \in (c_{t2 - s}^{A} ,c_{t2 - s}^{N} ]$, the retailer 2 forgoes RFID adoption when $c_{t} \in (c_{t2 - s}^{N} , + \infty )$; if $\alpha_{1} > \alpha_{2}$, we derive $c_{t2 - s}^{N} - c_{t2 - s}^{A} < 0$, hence, the retailer 2 always adopts RFID when $c_{t} \in (0,c_{t2 - s}^{N} ]$, the two retailers have the same decisions on RFID adoption when $c_{t} \in (c_{t2 - s}^{N} ,c_{t2 - s}^{A} ]$, and the retailer 2 forgoes RFID adoption when $c_{t} \in (c_{t2 - s}^{A} , + \infty )$.

We turn to deal with the equilibrium RFID adoption strategies.

If $\alpha_{1} = \alpha_{2} = \alpha$: given $c_{t} \in (0,\bar{c}_{t - s} ]$, the retailer 2 always adopts RFID. Under such a circumstance, we take $\Delta_{1 - s}^{A} = \pi_{1 - s}^{AA*} - \pi_{1 - s}^{NA*}$ as the retailer 1’s profit variation between AA and NA, and derive that the retailer 1 adopts RFID when $c_{t} \in (0,\bar{c}_{t - s} ]$, leading to that AA is an equilibrium strategy when $c_{t} \in (0,\bar{c}_{t - s} ]$.

Given $c_{t} \in (\bar{c}_{t - s} , + \infty )$, the retailer 2 always refuses to adopt RFID. Under such a circumstance, we take $\Delta_{1 - s}^{N} = \pi_{1 - s}^{AN*} - \pi_{1 - s}^{NN*}$ as the retailer 1’s profit variation between AN and NN, and derive that the retailer 1 adopts RFID when $c_{t} \in (\bar{c}_{t - s} , + \infty )$, resulting in that NN is an equilibrium strategy when $c_{t} \in (\bar{c}_{t - s} , + \infty )$.

If $\alpha_{1} < \alpha_{2}$: given $c_{t} \in (0,c_{t2 - s}^{A} ]$, the retailer 2 always adopts RFID. Under such a circumstance, we take $\Delta_{1 - s}^{A} = \pi_{1 - s}^{AA*} - \pi_{1 - s}^{NA*}$ as the retailer 1’s profit variation between AA and NA, and derive that the retailer 1 adopts RFID when $c_{t} \in (0,c_{t1 - s}^{A} ]$ while refuses to adopt RFID when $c_{t} \in (c_{t1 - s}^{A} , + \infty )$. We compare the relative sizes of $c_{t1 - s}^{A}$ and $c_{t2 - s}^{A}$, and then obtain $c_{t2 - s}^{A} - c_{t1 - s}^{A} = \frac{{(\alpha_{2} - \alpha_{1} )c_{m} }}{{(1 - \alpha_{2} )(1 - \alpha_{1} )}} > 0$, leading to that AA is an equilibrium strategy when $c_{t} \in (0,c_{t1 - s}^{A} ]$ and NA is an equilibrium strategy when $c_{t} \in (c_{t1 - s}^{A} ,c_{t2 - s}^{A} ]$.

Given $c_{t} \in (c_{t2 - s}^{A} ,c_{t2 - s}^{N} ]$, the two retailers have the opposite decisions on RFID adoption. Under such a circumstance, we take $\Delta_{1 - s}^{NA} = \pi_{1 - s}^{AN*} - \pi_{1 - s}^{NA*}$ as the retailer 1’s profit variation between AN and NA, and derive that the retailer 1 adopts RFID when $c_{t} \in (0,c_{t1 - s}^{NA} ]$ while refuses to adopt RFID when $c_{t} \in (c_{t1 - s}^{NA} , + \infty )$. We compare the relative sizes of $c_{t2 - s}^{A}$ and $c_{t1 - s}^{NA}$, and then obtain $c_{t2 - s}^{A} - c_{t1 - s}^{NA} = \frac{{2c_{m} }}{(2 + \gamma )}\left( {\frac{{\alpha_{2} - \alpha_{1} }}{{(1 - \alpha_{2} )(1 - \alpha_{1} )}}} \right) > 0$, resulting in that NA is an equilibrium strategy when $c_{t} \in (c_{t2 - s}^{A} ,c_{t2 - s}^{N} ]$. Where $c_{t1 - s}^{NA} = \frac{{c_{m} }}{(2 + \gamma )}\left( {\frac{{\gamma \alpha_{2} }}{{(1 - \alpha_{2} )}} + \frac{{2\alpha_{1} }}{{(1 - \alpha_{1} )}}} \right)$.

Given $c_{t} \in (c_{t2 - s}^{N} , + \infty )$, the retailer 2 always refuses to adopt RFID. Under such a circumstance, we take $\Delta_{1 - s}^{N} = \pi_{1 - s}^{AN*} - \pi_{1 - s}^{NN*}$ as the retailer 1’s profit variation between AN and NN, and derive that the retailer 1 adopts RFID when $c_{t} \in (0,c_{t1 - s}^{N} ]$ while refuses to adopt RFID when $c_{t} \in (c_{t1 - s}^{N} , + \infty )$. We compare the relative sizes of $c_{t2 - s}^{N}$ and $c_{t1 - s}^{N}$, and then obtain $c_{t2 - s}^{N} - c_{t1 - s}^{N} = \frac{{(4 - \gamma^{2} )\left[ {(1 - \alpha_{1} )^{2} - (1 - \alpha_{2} )^{2} } \right]a}}{{(4 - \gamma^{2} )(1 - \alpha_{1} )^{2} - 4\gamma (1 - \alpha_{1} )(1 - \alpha_{2} ) + 4(1 - \alpha_{2} )^{2} }} + \frac{{(\alpha_{2} - \alpha_{1} )c_{m} }}{{(1 - \alpha_{1} )(1 - \alpha_{2} )}} > 0$, then NN is an equilibrium strategy when $c_{t} \in (c_{t2 - s}^{N} , + \infty )$.

If $\alpha_{1} > \alpha_{2}$: given $c_{t} \in (0,c_{t2 - s}^{N} ]$, the retailer 2 always adopts RFID. Under such a circumstance, we take $\Delta_{1 - s}^{A} = \pi_{1 - s}^{AA*} - \pi_{1 - s}^{NA*}$ as the retailer 1’s profit variation between AA and NA, and derive that the retailer 1 adopts RFID when $c_{t} \in (0,c_{t1 - s}^{A} ]$ while refuses to adopt RFID when $c_{t} \in (c_{t1 - s}^{A} , + \infty )$. We compare the relative sizes of $c_{t2 - s}^{N}$ and $c_{t1 - s}^{A}$, as $c_{t2 - s}^{N} - c_{t1 - s}^{A} = \left( {\frac{{(16 - 8\gamma^{2} )(1 - \alpha_{2} )a}}{{(4 - \gamma^{2} )[(4 - \gamma^{2} )(1 - \alpha_{1} )^{2} - 4\gamma (1 - \alpha_{1} )(1 - \alpha_{2} ) + 4(1 - \alpha_{2} )^{2} ]}} + \frac{{c_{m} }}{{(1 - \alpha_{2} )(1 - \alpha_{1} )}}} \right)(\alpha_{2} - \alpha_{1} ) < 0$, leading to that AA is an equilibrium strategy when $c_{t} \in (0,c_{t2 - s}^{N} ]$.

Given $c_{t} \in (c_{t2 - s}^{N} ,c_{t2 - s}^{A} ]$, the two retailers have the same decisions on RFID adoption. Under such a circumstance, we take $\Delta_{1 - s}^{AN} = \pi_{1 - s}^{AA*} - \pi_{1 - s}^{NN*}$ as the retailer 1’s profit variation between AA and NN, and derive that the retailer 1 adopts RFID when $c_{t} \in (0,c_{t1 - s}^{AN} ]$ while forgoes RFID when $c_{t} \in (c_{t1 - s}^{AN} , + \infty )$. We compare the relative size of $c_{t1 - s}^{AN}$ and $c_{t2 - s}^{A}$, as $c_{t1 - s}^{AN} - c_{t2 - s}^{A} = \left( {\frac{{2(4 - 2\gamma^{2} )(1 - \alpha_{1} )a}}{{(2 - \gamma )\left[ {(4 - \gamma^{2} )(1 - \alpha_{1} )^{2} - 4\gamma (1 - \alpha_{1} )(1 - \alpha_{2} ) + 4(1 - \alpha_{2} )^{2} } \right]}} + \frac{{2c_{m} }}{{(2 - \gamma )(1 - \alpha_{1} )(1 - \alpha_{2} )}}} \right)(\alpha_{1} - \alpha_{2} ) > 0$, resulting in that AA is an equilibrium strategy when $c_{t} \in (c_{t2 - s}^{N} ,c_{t2 - s}^{A} ]$. Where $c_{t1 - s}^{AN} = \left( {\frac{{4v_{s} }}{{(2 - \gamma )(1 - \alpha_{1} )}} - \frac{{2\gamma v_{s} }}{{(2 - \gamma )(1 - \alpha_{2} )}} - 1} \right)a + \left( {\frac{{2\alpha_{1} }}{{(2 - \gamma )(1 - \alpha_{1} )}} - \frac{{\gamma \alpha_{2} }}{{(2 - \gamma )(1 - \alpha_{2} )}}} \right)c_{m}$.

Given $c_{t} \in (c_{t2 - s}^{A} , + \infty )$, the retailer 2 always refuses to adopt RFID. Under such a circumstance, we take $\Delta_{1 - s}^{N} = \pi_{1 - s}^{AN*} - \pi_{1 - s}^{NN*}$ as the retailer 1’s profit variation between AN and NN, and derive that the retailer 1 adopts RFID when $c_{t} \in (0,c_{t1 - s}^{N} ]$ while refuses to adopt RFID when $c_{t} \in (c_{t1 - s}^{N} , + \infty )$. We compare the relative sizes of $c_{t1 - s}^{N}$ and $c_{t2 - s}^{A}$, as $c_{t1 - s}^{N} - c_{t2 - s}^{A} = \left( {\frac{{(8 - 4\gamma^{2} )(1 - \alpha_{1} )a}}{{2\left[ {(4 - \gamma^{2} )(1 - \alpha_{1} )^{2} - 4\gamma (1 - \alpha_{1} )(1 - \alpha_{2} ) + 4(1 - \alpha_{2} )^{2} } \right]}} + \frac{{c_{m} }}{{(1 - \alpha_{1} )(1 - \alpha_{2} )}}} \right)(\alpha_{1} - \alpha_{2} ) > 0$, bringing about that AN is an equilibrium strategy when $c_{t} \in (c_{t2 - s}^{A} ,c_{t1 - s}^{N} ]$ and NN is an equilibrium strategy when $c_{t} \in (c_{t1 - s}^{N} , + \infty )$.

Consequently, we obtain the equilibrium strategies for RFID adoption when both retailers simultaneously make decisions on RFID adoption and sequentially make decisions on order quantity. If $\alpha_{1} = \alpha_{2} = \alpha$: AA is an equilibrium strategy when $c_{t} \in (0,\bar{c}_{t - s} ]$, NN is an equilibrium strategy when $c_{t} \in (\bar{c}_{t - s} , + \infty )$; if $\alpha_{1} < \alpha_{2}$, AA is an equilibrium strategy when $c_{t} \in (0,c_{t1 - s}^{A} ]$, NA is an equilibrium strategy when $c_{t} \in (c_{t1 - s}^{A} ,c_{t2 - s}^{N} ]$, NN is an equilibrium strategy when $c_{t} \in (c_{t2 - s}^{N} , + \infty )$; if $\alpha_{1} > \alpha_{2}$, AA is an equilibrium strategy when $c_{t} \in (0,c_{t2 - s}^{A} ]$, AN is an equilibrium strategy when $c_{t} \in (c_{t2 - s}^{A} ,c_{t1 - s}^{N} ]$, NN is an equilibrium strategy when $c_{t} \in (c_{t1 - s}^{N} , + \infty )$. Thus, we find that when both retailers sequentially make order quantity decisions, whether the two retailers adopt RFID simultaneously or sequentially does not affect the equilibrium strategies for RFID adoption.

Proof of Lemma 1

When $\alpha_{1} = \alpha_{2} = \alpha$, we have $\bar{c}_{t - n} = \bar{c}_{t - s} = \bar{c}_{t} = {{\alpha c_{m} } \mathord{\left/ {\vphantom {{\alpha c_{m} } {(1 - \alpha )}}} \right. \kern-0pt} {(1 - \alpha )}}$; when $\alpha_{1} < \alpha_{2}$, we have $\left( {{{2v_{n} } \mathord{\left/ {\vphantom {{2v_{n} } {(1 - \alpha_{2} )}}} \right. \kern-0pt} {(1 - \alpha_{2} )}} - {{\gamma v_{n} } \mathord{\left/ {\vphantom {{\gamma v_{n} } {(1 - \alpha_{1} )}}} \right. \kern-0pt} {(1 - \alpha_{1} )}} - {{(2 - \gamma )} \mathord{\left/ {\vphantom {{(2 - \gamma )} 2}} \right. \kern-0pt} 2}} \right)a < 0$ and $\left( {{{2v_{s} } \mathord{\left/ {\vphantom {{2v_{s} } {(1 - \alpha_{2} )}}} \right. \kern-0pt} {(1 - \alpha_{2} )}} - {{4\gamma v_{s} } \mathord{\left/ {\vphantom {{4\gamma v_{s} } {(1 - \alpha_{1} )(4 - \gamma^{2} )}}} \right. \kern-0pt} {(1 - \alpha_{1} )(4 - \gamma^{2} )}} - {{(4 - \gamma^{2} - 2\gamma )} \mathord{\left/ {\vphantom {{(4 - \gamma^{2} - 2\gamma )} {(4 - \gamma^{2} )}}} \right. \kern-0pt} {(4 - \gamma^{2} )}}} \right)a > 0$, hence we obtain $c_{t2 - n}^{N} - c_{t2 - s}^{N} < 0$; when $\alpha_{1} > \alpha_{2}$, we derive $c_{t1 - n}^{N} - c_{t1 - s}^{N} = (v_{n} - v_{s} )\left( {{2 \mathord{\left/ {\vphantom {2 {(1 - \alpha_{1} )}}} \right. \kern-0pt} {(1 - \alpha_{1} )}} - {\gamma \mathord{\left/ {\vphantom {\gamma {(1 - \alpha_{2} )}}} \right. \kern-0pt} {(1 - \alpha_{2} )}}} \right)a > 0$.

Proof of Proposition 6(i)

Given $\alpha_{1} = \alpha_{2} = \alpha$, we compare with the retailer 1’s profits under different ordering-timing strategies.

When $c_{t} \in (0,\bar{c}_{t} ]$, we obtain $\pi_{1 - s}^{AA*} - \pi_{1 - n}^{AA*} = {{\gamma^{4} (a - c_{m} - c_{t} )^{2} } \mathord{\left/ {\vphantom {{\gamma^{4} (a - c_{m} - c_{t} )^{2} } {32(2 - \gamma^{2} )(2 + \gamma )^{2} }}} \right. \kern-0pt} {32(2 - \gamma^{2} )(2 + \gamma )^{2} }} > 0$; when $c_{t} \in (\bar{c}_{t} , + \infty )$, we obtain $\pi_{1 - s}^{NN*} - \pi_{1 - n}^{NN*} = (2 - \gamma )^{2} \left( {{1 \mathord{\left/ {\vphantom {1 {4(16 - 8\gamma^{2} )}}} \right. \kern-0pt} {4(16 - 8\gamma^{2} )}} - {1 \mathord{\left/ {\vphantom {1 {4(4 - \gamma^{2} )^{2} }}} \right. \kern-0pt} {4(4 - \gamma^{2} )^{2} }}} \right)\left( {a - {{c_{m} } \mathord{\left/ {\vphantom {{c_{m} } {(1 - \alpha )}}} \right. \kern-0pt} {(1 - \alpha )}}} \right)^{2} > 0$. Thus, the retailer 1 prefers to order sequentially. In a similar way, we derive that the retailer 2 prefers to order simultaneously.

Proof of Proposition 6(ii)

From Proposition 6(i), we obtain that when $c_{t} \in (0,\bar{c}_{t} ]$, the retailer 1 prefers to order sequentially; for the retailer 2, ordering simultaneously will be more beneficial than ordering sequentially. Therefore, a transfer payment should be existed to achieve a win–win situation. Taking $\pi_{n}^{AA*} = \pi_{1 - n}^{AA*} + \pi_{2 - n}^{AA*}$ and $\pi_{s}^{AA*} = \pi_{1 - s}^{AA*} + \pi_{2 - s}^{AA*}$ as the retailers’ total profits under ordering simultaneously and ordering sequentially, respectively. We obtain $\pi_{n}^{AA*} - \pi_{s}^{AA*} > 0$, hence ordering simultaneously can bring the retailers’ more total profits when $c_{t} \in (0,\bar{c}_{t} ]$. The retailer 2 should share his profit $F \in [\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{F}^{AA*} ,\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{F}^{AA*} ]$ to the retailer 1 to achieve Pareto improvement.

When $c_{t} \in (\bar{c}_{t} , + \infty )$, the proof is similar to the case when $c_{t} \in (0,\bar{c}_{t} ]$. Therefore we derive when $c_{t} \in (\bar{c}_{t} , + \infty )$, ordering simultaneously can bring the retailers’ more total profits. The retailer 2 should share his profit $F \in [\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{F}^{NN*} ,\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{F}^{NN*} ]$ to the retailer 1 to achieve Pareto improvement.

1.8 Summary of abbreviations in Proposition 6

$$ \underline{F}^{NN*} = \left[ {\frac{{(2 - \gamma )^{2} }}{{32(2 - \gamma^{2} )}} - \left( {\frac{2 - \gamma }{{8 - 2\gamma^{2} }}} \right)^{2} } \right]\left( {a - \frac{{c_{m} }}{1 - \alpha }} \right)^{2} ,\quad \underline{F}^{AA*} = \frac{{\gamma^{4} (a - c_{m} - c_{t} )^{2} }}{{32(2 - \gamma^{2} )(2 + \gamma )^{2} }} $$

$$ \bar{F}^{NN*} = \left[ {\left( {\frac{2 - \gamma }{{8 - 2\gamma^{2} }}} \right)^{2} - \left( {\frac{{4 - \gamma^{2} - 2\gamma }}{{16 - 8\gamma^{2} }}} \right)^{2} } \right]\left( {a - \frac{{c_{m} }}{1 - \alpha }} \right)^{2} ,\quad \bar{F}^{AA*} = \frac{{\gamma^{3} (16 - 8\gamma^{2} - \gamma^{3} )(a - c_{m} - c_{t} )^{2} }}{{64(2 - \gamma^{2} )^{2} (2 + \gamma )^{2} }} $$

Proof of Proposition 7(i)

Given $\alpha_{1} < \alpha_{2}$, we compare with the retailer 1’s profits under different ordering-timing strategies.

When $c_{t} \in (0,c_{t2 - n}^{N} ]$, the proof is similar to that of Proposition 6(i) and hence is omitted; when $c_{t} \in (c_{t2 - n}^{N} ,c_{t2 - s}^{N} ]$, we obtain $\pi_{1 - s}^{NA*} - \pi_{1 - n}^{NN*} \le 0$ if $\gamma \in (0,\overline{{\gamma_{1} }} ]$, while $\pi_{1 - s}^{NA} - \pi_{1 - n}^{NN} > 0$ otherwise; when $c_{t} \in (c_{t2 - s}^{N} , + \infty )$, we obtain $\pi_{1 - s}^{NN*} - \pi_{1 - n}^{NN*} \le 0$ if $\gamma \in (0,\overline{{\gamma_{2} }} ]$, while $\pi_{1 - s}^{NN} - \pi_{1 - n}^{NN} > 0$ otherwise. In a similar way, we derive the retailer 2 always obtain higher profits by ordering simultaneously.

Proof of proposition 7(ii)

When $c_{t} \in (0,\bar{c}_{t1}^{A} ]$, the proof is similar to that of proposition 6(ii) and hence is omitted.

When $c_{t} \in (\bar{c}_{t1}^{A} ,c_{t2 - n}^{N} ]$, ordering simultaneously can bring the retailers’ more total profits. The retailer 2 should share his profit $F \in [\underleftarrow F^{NA*} ,\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{F}^{NA*} ]$ to the retailer 1 to achieve Pareto improvement.

When $c_{t} \in (c_{t2 - n}^{N} ,c_{t2 - s}^{N} ]$ and $\gamma \in (\overline{{\gamma_{1} }} ,1]$, we obtain $\pi_{s}^{NN*} < \pi_{n}^{NN*}$. Therefore, ordering simultaneously can bring the retailers’ more total profits when. The retailer 2 should share his profit $F \in [\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{F}^{N*} ,\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{F}^{N*} ]$ to the retailer 1 to achieve Pareto improvement.

When $c_{t} \in (c_{t2 - s}^{N} , + \infty )$ and $\gamma \in (\overline{{\gamma_{1} }} ,1]$: we obtain $\pi_{s}^{NN*} \ge \pi_{n}^{NN*}$ if $k \in (0,\overleftarrow {k} ]$. Therefore, ordering sequentially can bring the retailers’ more total profits. The retailer 1 should share his profit $F \in [\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{F}^{NN*} ,\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{F}^{NN*} ]$ to the retailer 2 to achieve Pareto improvement. In addition, we obtain $\pi_{s}^{NN*} < \pi_{n}^{NN*}$ if $k \in (\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{k} ,1)$, hence ordering simultaneously can bring the retailers’ more total profits. The retailer 2 should share his profit $F \in [\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{F}^{NN*} ,\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{F}^{NN*} ]$ to the retailer 1 to achieve Pareto improvement. Where $\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{F}^{NA*} = \mathop {\arg }\limits_{F} \left\{ {\pi_{1 - s}^{NA*} = \pi_{1 - n}^{NA*} } \right\}$, $\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{F}^{NA*} = \mathop {\arg }\limits_{F} \left\{ {\pi_{2 - n}^{NA*} = \pi_{2 - s}^{NA*} } \right\}$, $\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{F}^{N*} = \mathop {\arg }\limits_{F} \left\{ {\pi_{1 - n}^{NN*} = \pi_{1 - s}^{NA*} } \right\}$, $\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{F}^{N*} = \mathop {\arg }\limits_{F} \left\{ {\pi_{2 - n}^{NN*} = \pi_{2 - s}^{NA*} } \right\}$, $\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{F}^{NN*} = \mathop {\arg }\limits_{F} \left\{ {\pi_{1 - s}^{NN*} = \pi_{1 - n}^{NN*} } \right\}$, $\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{F}^{NN*} = \mathop {\arg }\limits_{F} \left\{ {\pi_{2 - n}^{NN*} = \pi_{2 - s}^{NN*} } \right\}$.

1.9 Summary of abbreviations in Proposition 7

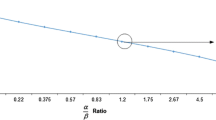
$\overline{{\gamma_{1} }} = \mathop {\arg }\limits_{\gamma } \left\{ {\pi_{1 - s}^{NA*} = \pi_{1 - n}^{NA*} } \right\}$, $\overline{{\gamma_{2} }} = \mathop {\arg }\limits_{\gamma } \left\{ {\pi_{1 - s}^{NN*} = \pi_{1 - n}^{NN*} } \right\}$, $\overleftarrow {k} = \mathop {\arg }\limits_{k} \{ \pi_{1 - s}^{NN*} + \pi_{2 - s}^{NN*} = \pi_{1 - n}^{NN*} + \pi_{2 - n}^{NN*} \}$.

Proof of Proposition 8(i)

Given $\alpha_{1} > \alpha_{2}$, we compare with the retailer 1’s profits under different ordering-timing strategies, and obtain that he always obtain higher profits by ordering sequentially.

We compare with the retailer 2’s profits under different ordering-timing strategies. When $c_{t} \in (0,c_{t1 - s}^{N} ]$, the proof is similar to that of Proposition 6(i) and hence is omitted; when $c_{t} \in (c_{t1 - s}^{N} ,c_{t1 - n}^{N} ]$, we obtain $\pi_{2 - n}^{AN*} - \pi_{2 - s}^{NN*} \le 0$ if $\gamma \in (0,\overline{{\gamma_{3} }} ]$, while $\pi_{2 - n}^{AN*} - \pi_{2 - s}^{NN*} > 0$ otherwise; when $c_{t} \in (c_{t1 - n}^{N} , + \infty )$, we obtain $\pi_{2 - n}^{NN*} - \pi_{2 - s}^{NN*} \le 0$ if $\gamma \in (0,\overline{{\gamma_{4} }} ]$, while $\pi_{2 - n}^{NN*} - \pi_{2 - s}^{NN*} > 0$ otherwise.

Proof of Proposition 8(ii)

When $c_{t} \in (0,\bar{c}_{t2}^{A} ]$, the proof is similar to that of proposition 6(ii) and hence is omitted.

When $c_{t} \in (\bar{c}_{t2}^{A} ,c_{t1 - s}^{N} ]$, ordering simultaneously can bring the retailers’ more total profits. The retailer 2 should share his profit $F \in [\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\leftarrow}$}}{F}^{AN*} ,\vec{F}^{AN*} ]$ to the retailer 1 to achieve Pareto improvement.

When $c_{t} \in (c_{t1 - s}^{N} ,c_{t1 - n}^{N} ]$ and $\gamma \in (\overline{{\gamma_{3} }} ,1]$, we obtain $\pi_{2 - n}^{AN*} - \pi_{2 - s}^{NN*} > 0$. Therefore, ordering sequentially can bring the retailers’ more total profits. The retailer 1 should share his profit $F \in [\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\rightarrow}$}}{F}^{N*} ,\vec{F}^{N*} ]$ to the retailer 2 to achieve Pareto improvement.

When $c_{t} \in (c_{t2 - s}^{N} , + \infty )$ and $\gamma \in (\overline{{\gamma_{4} }} ,1]$: we obtain $\pi_{s}^{NN*} \le \pi_{n}^{NN*}$ if $k \in (1,\vec{k}]$. Therefore, ordering simultaneously can bring the retailers’ more total profits. The retailer 2 should share his profit $F \in [\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\rightarrow}$}}{F}^{NN*} ,\vec{F}^{NN*} ]$ to the retailer 1 to achieve Pareto improvement. Moreover, we obtain $\pi_{s}^{NN*} > \pi_{n}^{NN*}$ if $k \in (\vec{k}, + \infty )$, hence ordering sequentially can bring the retailers’ more total profits. The retailer 1 should share his profit $F \in [\vec{F}^{NN*} ,\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\rightarrow}$}}{F}^{NN*} ]$ to the retailer 2 to achieve Pareto improvement. Where $\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\rightarrow}$}}{F}^{AN*} = \mathop {\arg }\limits_{F} \left\{ {\pi_{1 - s}^{AN*} = \pi_{1 - n}^{AN*} } \right\}$, $\vec{F}^{AN*} = \mathop {\arg }\limits_{F} \left\{ {\pi_{2 - n}^{AN*} = \pi_{2 - s}^{AN*} } \right\}$, $\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\rightarrow}$}}{F}^{NN*} = \mathop {\arg }\limits_{F} \left\{ {\pi_{1 - s}^{NN*} = \pi_{1 - n}^{NN*} } \right\}$, $\vec{F}^{NN*} = \mathop {\arg }\limits_{F} \left\{ {\pi_{2 - n}^{NN*} = \pi_{2 - s}^{NN*} } \right\}$, $\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle\rightarrow}$}}{F}^{N*} = \mathop {\arg }\limits_{F} \left\{ {\pi_{2 - n}^{AN*} = \pi_{2 - s}^{NN*} } \right\}$, $\vec{F}^{N*} = \mathop {\arg }\limits_{F} \left\{ {\pi_{1 - n}^{AN*} = \pi_{1 - s}^{NN*} } \right\}$.

1.10 Summary of abbreviations in Proposition 8

$\overline{{\gamma_{3} }} = \mathop {\arg }\limits_{\gamma } \left\{ {\pi_{2 - s}^{AN*} = \pi_{2 - n}^{AN*} } \right\}$, $\overline{{\gamma_{4} }} = \mathop {\arg }\limits_{\gamma } \left\{ {\pi_{2 - n}^{NN*} = \pi_{2 - s}^{NN*} } \right\}$, $\overrightarrow {k} = \mathop {\arg }\limits_{k} \{ \pi_{1 - s}^{NN*} + \pi_{2 - s}^{NN*} = \pi_{1 - n}^{NN*} + \pi_{2 - n}^{NN*} \}$.

Proof of Proposition 9

The proof is similar to that of Proposition 1 and hence is omitted.

Proof of Proposition 10

The proof is similar to that of Proposition 5 and hence is omitted.

1.11 Summary of abbreviations in Proposition 10

$\tilde{c}_{t} = {{\alpha c_{m} } \mathord{\left/ {\vphantom {{\alpha c_{m} } {(1 - \alpha )}}} \right. \kern-0pt} {(1 - \alpha )}}$, $\tilde{c}_{t1}^{A} = \tilde{c}_{t1}^{N} = {{\alpha_{1} c_{m} } \mathord{\left/ {\vphantom {{\alpha_{1} c_{m} } {(1 - \alpha_{1} )}}} \right. \kern-0pt} {(1 - \alpha_{1} )}}$, $\tilde{c}_{t2}^{A} = \tilde{c}_{t2}^{N} = {{\alpha_{2} c_{m} } \mathord{\left/ {\vphantom {{\alpha_{2} c_{m} } {(1 - \alpha_{2} )}}} \right. \kern-0pt} {(1 - \alpha_{2} )}}$.

Proof of Proposition 11

The proof is similar to those of Propositions 7–8 and hence is omitted.

1.12 Summary of abbreviations in Proposition 11

$\overline{{\tilde{\gamma }_{1} }} = \mathop {\arg }\limits_{{\tilde{\gamma }}} \left\{ {\tilde{\pi }_{1 - s}^{NN*} + \tilde{\pi }_{2 - s}^{NN*} = \tilde{\pi }_{1 - n}^{NN*} + \tilde{\pi }_{2 - n}^{NN*} } \right\}$, $\overline{{\tilde{\gamma }_{2} }} = \mathop {\arg }\limits_{{\tilde{\gamma }}} \left\{ {\tilde{\pi }_{2 - n}^{NN*} = \tilde{\pi }_{2 - s}^{NN*} } \right\}$, $\overleftarrow {{\tilde{k}}} = \mathop {\arg }\limits_{{\tilde{k}}} \{ \tilde{\pi }_{1 - s}^{NN*} + \tilde{\pi }_{2 - s}^{NN*} = \tilde{\pi }_{1 - n}^{NN*} + \tilde{\pi }_{2 - n}^{NN*} \}$, $\overrightarrow {{\tilde{k}}} = \mathop {\arg }\limits_{{\tilde{k}}} \left\{ {\tilde{\pi }_{1 - s}^{NN*} + \tilde{\pi }_{2 - s}^{NN*} = \tilde{\pi }_{1 - n}^{NN*} + \tilde{\pi }_{2 - n}^{NN*} } \right\}$.