Optimal continuous production-inventory systems subject to stockout risk

Abstract

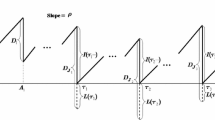
This paper studies a continuous-review production-inventory system with a constant production rate and compound Poisson demands, in which the cost of the system is assessed with holding cost and stockout penalty. For any initial inventory, we derive closed form expression for the expected discounted cost function until stockout occurrence. We quantify the risk of stockout in terms of the average time to stockout occurrence. The objective is to derive the optimal production rate that minimizes the expected discounted system cost subject to a given risk level of stockout. With the aid of the explicit forms of stockout risk and the cost function, we present a computation-efficient algorithm for the optimal solution. For the special cases with proportional stockout penalty function, if the demands follow an exponential distribution, we have a closed form expression for the expected discounted cost. Some numerical studies are conducted to illustrate our results with further insights. Numerically, we show that it is outrageously costly to reduce stockout risk especially when the stockout risk is relatively low. Our results shed light on the inventory risk control and cost optimization. The major results and the developed algorithm can be leveraged to facilitate continuous-production manufacturers, especially pharmaceutical firms, with their Production Process Validation.

Appendices

Appendix 1: Proofs

Proof for Lemma 1

To prove $\phi (z)$ is strictly convex, we compute the first and second order derivative of $\phi (z)$. Then we have

$$\begin{aligned} \phi {'}(z)= & {} \rho -\lambda \int _{0}^{\infty }xe^{-zx}f_D(x)dz \end{aligned}$$

(67)

$$\begin{aligned} \phi {''}(z)= & {} \lambda \int _{0}^{\infty }x^2 \cdot e^{-zx}f_D(z) >0 \end{aligned}$$

(68)

Hence, $\phi (z)$ is strictly convex by Eq. (68).

Note that $\phi (\infty )=\phi (-\infty )=\infty$ and $\phi (0)=-r<0$. Therefore, there must be at least one positive root and one negative root, denoted by $\theta \ge 0$ and $\zeta \le 0$, respectively. Next, we prove the uniqueness by contradiction. To this end, assume that there is another root s, such that $c(s)=0$. In what follows, we assume $\zeta< s < \theta$. For other cases where $s<\zeta$ or $s>\theta$, we can follow the same line to complete the proof. According to Rolle’s theorem, there must be $s_1<s_2$ such that $\phi '(s_1)=\phi '(s_2)=0$, where $\zeta<s_1< s<s_2< \theta$. This is contradictory to the monotonicity of $\phi '(z)$ which is implied by Eq. (68), and thus completes the proof for part (2).

Proof for Theorem 1

By Lemma 1, we have

$$\begin{aligned} \lambda {\hat{f}}_D(\theta )-(r+\lambda )+\rho \theta =0. \end{aligned}$$

(69)

Setting $z=\theta$ in Eq. (15), applying Eq. (69) and rearranging, we complete the proof. $\square$

Proof for Theorem 2

Our effort in the proof is to simplify ${\hat{\alpha }}$ and ${\hat{\beta }}$ given by Eq. (35) and (36), respectively. Then we obtain the result by Eq. (39). First, taking Laplace transform on both sides of Eq. (35) and changing the order of the integral, we have

$$\begin{aligned} \hat{\alpha }(z)= & {} \frac{\lambda }{\rho } \int _0^{\infty }e^{-z x}\left[ \int _x^{\infty }e^{\theta (x-y)}\cdot f_D(y)\cdot dy\right] \cdot dx\nonumber \\= & {} \frac{\lambda }{(\theta -z)\rho }\int _0^{\infty }[e^{(\theta -z)y}-1]e^{-\theta y}f_D(y)dy\nonumber \\= & {} \frac{\lambda }{(\theta -z)\rho }[\hat{f}_D(z)-\hat{f}_D(\theta )]. \end{aligned}$$

(70)

By Eq. (69), Eq. (70) can be further written as

$$\begin{aligned} \hat{\alpha }(z)=\frac{\lambda \hat{f}_D(z)+\rho \theta -r-\lambda }{(\theta -z)\rho }. \end{aligned}$$

(71)

Hence,

$$\begin{aligned} 1-\hat{\alpha }(z)=\frac{ \lambda +r-\lambda \hat{f_D}(z)-\rho z}{\rho \cdot (\theta -z)}=\frac{ \phi (z)}{(z-\theta )\rho }. \end{aligned}$$

(72)

Note that, if $z=\zeta$ in Eq. (72), then by Lemma 1, $1-\hat{\alpha }(\zeta )=0$. In other words, $\rho$ is the root to $\hat{\alpha }(z)=1$.

Second, taking Laplace transform on both sides of Eq. (36) and changing the order of integration, we have

$$\begin{aligned} \hat{\beta }(z)= & {} \frac{1}{\rho } \int _0^{\infty } e^{-z x}\int _x^{\infty }e^{\theta (x-u)} g(u) \cdot du\cdot dx \nonumber \\= & {} \frac{1}{\rho } \int _0^{\infty } e^{-\theta u} g(u) \int _{0}^{u} e^{-(z-\theta ) x} \cdot dx \cdot du \nonumber \\= & {} \frac{1}{\rho } \int _0^{\infty } e^{-\theta u} g(u) \frac{1}{z-\theta }\bigg [1-e^{-(z-\theta )u}\bigg ] \cdot du \nonumber \\= & {} \frac{1}{(z-\theta )\rho } \bigg [\int _0^{\infty } e^{-\theta u} g(u) \cdot du-\int _0^{\infty } e^{-z u} g(u) \cdot du \bigg ] \nonumber \\= & {} \frac{1}{(z-\theta )\rho } \bigg [{\hat{g}}(\theta ) -{\hat{g}}(z)\bigg ] \end{aligned}$$

(73)

Consequently, dividing Eq. (73) by Eq. (72), we complete the proof. $\square$

Proof for Theorem 3

To obtain c(u), we shall apply the inverse Laplace transform of ${\hat{c}}(z)$ provided in Theorem 2. Taking the inverse Laplace transform on both sides of Eq. (42) yields

$$\begin{aligned} c(u)={\hat{g}}(\theta ) \cdot \mathcal {L}^{-1}\big \{\phi ^{-1}\big \}(u) -(g*\mathcal {L}^{-1}\big \{\phi ^{-1}\big \})(u) \end{aligned}$$

(74)

where the first term on the right hand side holds by the linearity property and the second term by the convolution property as given in Table 4. In view of Eq. (18), we have $\phi ^{-1}(z)=1/\phi (z)$ given as

$$\begin{aligned} \phi ^{-1}(z)=\frac{m(z)}{(z-\theta )(z-\zeta )} \end{aligned}$$

(75)

Therefore, its inverse Laplace transform is given as below [cf. Shi et al. 2014],

$$\begin{aligned} \mathcal {L}^{-1}\bigg \{\phi ^{-1}\bigg \}(u)=\frac{m(\theta )}{\theta -\zeta }e^{\theta u}+\frac{m(\zeta )}{\zeta -\theta }e^{\zeta u} \end{aligned}$$

(76)

Consequently, Eq. (45) follows via substituting Eq. (76) into Eq. (74) and the proof is complete with basic algebra. $\square$

Proof for Corollary 1

To prove the result, we specify $h=0$ and $w(u,y)=1_{\{y>0\}}$ in the derivation for $c_{\rho }(u)$. Accordingly, the corresponding results hold for $v_{\rho }(u)$ by replacing g(u) with $\lambda {\bar{F}}(u)$.

To prove Eq. (46), we apply Theorem 1. Then by Eq. (27) and replacing ${\hat{g}}(\theta )$ with $\lambda \, {\hat{\bar{F}}_D}\,(\theta )$, we have

$$\begin{aligned} v_{\rho }(0)=\frac{\lambda {\hat{\bar{F}}_D}(\theta )}{\rho }. \end{aligned}$$

(77)

Furthermore,

$$\begin{aligned} {\hat{\bar{F}}}(z)= & {} \frac{1-\hat{f}_D(z)}{z} =\frac{\rho z-r-\phi (z)}{\lambda z}. \end{aligned}$$

(78)

where the first equality holds by integration by parts in the Laplace transform and the second equality holds by Eq. (16). Finally, Eq. (77) can be rewritten as Eq. (46) by Eq. (78) and the fact that $\phi (\theta )=0$. This completes the proof for Eq. (46).

To prove Eq. (47), we apply Theorem 2. Then we have

$$\begin{aligned} {\hat{v}}_{\rho }(z)= \frac{\lambda {\hat{\bar{F}}}(\theta )-\lambda {\hat{\bar{F}}}(z)}{\phi (z)}. \end{aligned}$$

(79)

Finally, the proof for Eq. (47) completes after substituting Eq. (78) into Eq. (79) and simple rearrangement. $\square$

Proof for Theorem 4

We prove the result via the inverse Laplace transform of ${\hat{v}}_{\rho }(z)$ given by Eq. (47). Accordingly, one has

$$\begin{aligned} v_{\rho }(u)=r\,\int _{0}^{u}\mathcal {L}^{-1}\bigg \{\phi ^{-1}\bigg \}(x)dx -\frac{r}{\theta }\mathcal {L}^{-1} \bigg \{\phi ^{-1}\bigg \}(u) +1. \end{aligned}$$

(80)

where the first term on the right hand side above is obtained by the integration property of Laplace transform Table 4, and the third term is obtained by the fact that $\mathcal {L}\{1\}=1/z$. The proof is complete via substituting $\mathcal {L}^{-1}[\phi ^{-1}](u)$ given by Eq. (76) into Eq. (80) after some basic algebra. $\square$

Proof for Theorem 5

We only need to prove for the first part under stability condition since the second part can be proved in a similar vein. In this case, setting $z=0$ in Eq. (67), we have $\phi '(0)=\rho -\lambda \,\mathbb {E}[D]$. Under the stability condition given by Eq. (50), we have $\phi '(0)<0$, which implies $\theta >0$. It can be further shown $\zeta _{r}\rightarrow 0$ as r approaches 0. Furthermore, by Eq. (69)

$$\begin{aligned} \frac{r}{\zeta _{r}}= & {} \rho -\frac{\lambda }{\zeta _{r}}[1- {\hat{f}}_D(\zeta _{r})]\\= & {} \rho -\frac{\lambda }{\zeta _{r}}\bigg [\int _0^{\infty }f_D(x)\cdot dx- \int _0^{\infty }e^{-\zeta _{r} x}f_D(x)\cdot dx\bigg ]\\= & {} \rho -\lambda \int _0^{\infty }\frac{1-e^{-\zeta _{r} x}}{\theta }f_D(x)\cdot dx \end{aligned}$$

Finally, the proof for part (3) is completed via following Fubini’s Theorem and the fact $\underset{a \rightarrow 0}{\lim } \frac{1-e^{-a x}}{a}=x$. $\square$

Proof for Theorem 6

Note that for zero initial inventory, $v_{\rho }(0)=\mathbb {E}[ e^{-r \tau }\cdot \mathbf{1}(\tau <\infty )|I(0)=0]$, which has an explicit expression given by Corollary 1. Note further that the expected time to stockout is obtained

$$\begin{aligned} {\bar{\tau }}(u)=\mathbb {E}\bigg [\tau \cdot \mathbf{1}(\tau<\infty )\bigg |I(0)=u\bigg ]=-\frac{\partial }{\partial r}\mathbb {E} \bigg [ e^{-r \tau }\cdot \mathbf{1}(\tau <\infty ) \bigg | I(0)=u\bigg ]\bigg |_{r=0}. \end{aligned}$$

For stabilized system, taking derivative of Eq. (46) with respective to r and then sending $r\rightarrow 0$, we reach Eq. (56).

For unstabilized system, since $\tau$ is unbounded almost surely, we have $\bar{\tau }$ unbounded, which completes the proof. $\square$

Proof for Corollary 2

If $w(x,y)=\eta \cdot y$, then $\omega (u)=\eta \int _{u}^{\infty }(x-u) f_D(x)dx$ by Eq. (11). Let $T(u):=\int _{u}^{\infty }(x-u) f_D(x)dx$. Then we have $g(u)=\lambda \cdot \omega (u)+h\cdot u$ by Eq. (13) further written as $g(u)=\lambda \, \eta \cdot T(u)+h\,u.$ First, the Laplace transform of T(u) is given

$$\begin{aligned} {\hat{T}}(z)= & {} \int _{0}^{\infty }e^{-zu} \bigg (\int _{u}^{\infty }(x-u) f_D(x)dx\bigg ) \,du \nonumber \\= & {} \int _{0}^{\infty } f_D(x) \,\bigg (\int _{0}^{x}(x-u) e^{-zu} du\bigg ) dx \nonumber \\= & {} \frac{\mathbb {E}[D]}{z}-\frac{1-{\hat{f}}_{D}(z)}{z^{2}}, \end{aligned}$$

(81)

where the first equality holds via changing the order of integrations and the second equality holds by

$$\begin{aligned} \int _{0}^{x}(x-u) e^{-zu} du=\frac{x}{z}+\frac{e^{-zx}-1}{z^{2}}. \end{aligned}$$

Second, note that $\mathcal {L}\{u\}=\frac{1}{z^{2}}$. Therefore, the Laplace transform of g(u) is given by

$$\begin{aligned} {\hat{g}}(z)= & {} \eta \lambda {\hat{T}}(z)+\frac{h}{z^{2}}\nonumber \\= & {} \eta \, \lambda \bigg ( \frac{\mathbb {E}[D]}{z}-\frac{1-{\hat{f}}_{D}(z)}{z^{2}}\bigg )+\frac{h}{z^{2}}\nonumber \\= & {} \frac{\eta \lambda \mathbb {E}[D]}{z}-\frac{\eta \lambda [1-{\hat{f}}_{D}(z)]}{z^{2}}+\frac{h}{z^{2}} \end{aligned}$$

(82)

The proof for Eq. (59) completes via substituting Eq. (82) into Eq. (42) and following some simple rearrangements. The proof for Eq. (60) immediately follows Eqs. (82) and (27), and the identity $\lambda [1-{\hat{f}}_{D}(\theta )]=\rho \theta -r$. This completes the proof.

Proof for Corollary 3

Substituting Eq. (23) into Eq. (59) and applying the result of the two roots $\theta$ and $\zeta$, we have

$$\begin{aligned} \hat{c}(z)= \frac{\eta \cdot \lambda \cdot (\lambda -\rho \kappa )}{\rho \cdot (z-\theta )(z-\zeta )}\bigg [\frac{1}{\theta }-\frac{1}{z}\bigg ] +\frac{\lambda \cdot \kappa \cdot (h+r \eta ) }{\rho \cdot (z-\theta )(z-\zeta )}\bigg [\frac{1}{\theta ^2}-\frac{1}{z^2}\bigg ]-\eta \frac{1}{z^2}. \end{aligned}$$

After some algebra, the above equation can be further written as

$$\begin{aligned} \hat{c}(z)=b_2\frac{1}{z-\zeta } +b_1 \frac{1}{z^2}+b_0 \frac{1}{z}. \end{aligned}$$

Finally, the proof completes by taking the inverse Laplace transform of the above equation. $\square$

Proof for Theorem 7

First, the expected system cost $c_{\rho }$ is given by Eqs. (27). Further, note that $\rho$ and $\theta$ are one-to-one mapping to each other, which is identified by Eq. (17). Hence, the objective cost $c_{\rho }$ can be expressed in terms of $\theta$ as

$$\begin{aligned} c_{\theta }(0)= \; \frac{\theta \cdot {\hat{g}}(\theta )}{r+\lambda \, \theta \, {\hat{\bar{F}}}_D(\theta )}. \end{aligned}$$

Accordingly, we have the optimal $\rho _{r}^{*}$ that minimizes $c_{\rho }$ can be obtained via $\theta ^{*}$ as given by Eq. (65) directly. This proves Eq. (64).

Next, we consider the stockout risk constraints. Thanks to the monotonicity of $\bar{\tau }$ in $\rho$, we can find the corresponding critical value of $\rho ^{L}$ via Eqs. (58). This completes the proof. $\square$

Appendix 2: Some Properties of Laplace Transform

Table 4 summarizes some key properties of Laplace transform. For more details on Laplace transform and its applications, the reader is referred to Lee and Shi (2010).

Table 4 Properties of Laplace transform ${\hat{f}}(z)=\mathcal {L} \{f(x)\}$