Linearized Boltzmann Collision Operator: I. Polyatomic Molecules Modeled by a Discrete Internal Energy Variable and Multicomponent Mixtures

Abstract

The linearized Boltzmann collision operator appears in many important applications of the Boltzmann equation. Therefore, knowing its main properties is of great interest. This work extends some classical results for the linearized Boltzmann collision operator for monatomic single species to the case of polyatomic single species, while also reviewing corresponding results for multicomponent mixtures of monatomic species. The polyatomicity is modeled by a discrete internal energy variable, that can take a finite number of (given) different values. Results concerning the linearized Boltzmann collision operator being a nonnegative symmetric operator with a finite-dimensional kernel are reviewed.

A compactness result, saying that the linearized operator can be decomposed into a sum of a positive multiplication operator, the collision frequency, and a compact operator, bringing e.g., self-adjointness, is extended from the classical result for monatomic single species, under reasonable assumptions on the collision kernel. With a probabilistic formulation of the collision operator as a starting point, the compactness property is shown by a splitting, such that the terms can be shown to be, or be the uniform limit of, Hilbert-Schmidt integral operators and as such being compact operators. Moreover, bounds on - including coercivity of - the collision frequency are obtained for a hard sphere like model, from which Fredholmness of the linearized collision operator follows, as well as its domain.

1 Introduction

The Boltzmann equation is a fundamental equation in the kinetic theory of gases. It takes binary collisions into account (assuming rarefied gases), while it is assumed that momentum and (kinetic plus possible internal) energy is conserved during collisions. Considering deviations of an equilibrium or a Maxwellian distribution, in the collision integral a linearized collision operator is obtained. The (usually unbounded) linearized Boltzmann collision operator appears in a large number of important applications of the Boltzmann equation [1–4, 6]. Classical results are that it is a nonnegative symmetric operator with a finite-dimensional kernel, while it is less trivial to prove self-adjointness or Fredholmness (for some collision kernels).

The linearized collision operator can in a natural way be written as a sum of a positive multiplication operator, the collision frequency, and an integral operator $-K$. Compactness properties of the integral operator $K$ (for Grad’s cut-off kernels) are extensively studied for monatomic single species [15, 16, 19, 24]. The integral operator can be written as the sum of a Hilbert-Schmidt integral operator and an operator that is the uniform limit of Hilbert-Schmidt integral operators (cf. Lemma 4 in Sect. 2.3) [18], and so compactness of the integral operator $K$ is obtained. More recently, compactness results were also obtained for monatomic multicomponent mixtures by Boudin et al. [10]. For a polyatomic gas, assuming that the translational and vibrational energies can be modelled by a single internal energy variable, one can either consider the internal energy variable to be discrete or continuous [11, 17, 20]. See also [8] where a general framework is exploited. In this work, we consider polyatomic single species, where the polyatomicity is modeled by a discrete internal energy variable [17, 20]. We also review the case of multicomponent mixtures of monatomic species, for which the compactness result is already covered by Boudin et al. [10], while approached from a different starting point. This is a first step to cover the case of multicomponent mixtures of polyatomic species.

Motivated by an approach by Kogan in [23, Sect. 2.8] for monatomic single species case, a probabilistic formulation of the collision operator is considered as the starting point. By starting from the probabilistic formulation, there is no initial parametrization of the velocities based on the momentum and energy conservation, why the parametrization can be chosen from scratch to be more suitable from case to case. From our point of view, this really helps to find appropriate substitutions, by geometrical motivations, for the non-trivial cases, and was crucial for us in the polyatomic case. Using this approach, it is shown, based on ideas from the corresponding proof for monatomic single species by Grad [19], in particular, as presented by Glassey [18], that the integral operator $K$ can be written as a sum of Hilbert-Schmidt integral operators and operators that are the uniform limit of Hilbert-Schmidt integral operators, and hence, compactness of the integral operator $K$ follows for the polyatomic model. The operator $K$ is self-adjoint, as well as the collision frequency, why the linearized collision operator, as the sum of two self-adjoint operators of which one is bounded, is also self-adjoint.

For hard sphere like models, bounds on the collision frequency are obtained, implying its domain. Then the collision frequency is coercive and becomes a Fredholm operator. The set of Fredholm operators is closed under addition with compact operators, why also the linearized collision operator becomes a Fredholm operator by the compactness of the integral operator $K$. For hard sphere like models the linearized collision operator satisfies all the properties of the general linear operator in the abstract half-space problem considered in [4], and, hence, the existence results in [4] apply. The same applies for hard sphere models for multicomponent mixtures, see [3].

Related studies have attracted recent attention. The case of polyatomic single species, where the polyatomicity is modeled by a continuous internal energy variable is considered in [5], see also [9] for the case of molecules undergoing resonant collisions (for which internal energy and kinetic energy, respectively, are conserved under collisions), and [13, 14] for diatomic and polyatomic gases, respectively - with more restrictive assumptions on the collision kernels than in [5], but also a more direct approach.

The rest of the paper is organized as follows. In Sect. 2 polyatomic gases are considered, while multicomponent gases are considered in Sect. 3. The models considered are presented, for polyatomic molecules in Sect. 2.1 and for multicomponent mixtures in Sect. 3.1. The probabilistic formulation of the collision operators considered and its relations to more classical formulations [10, 17, 20] are accounted for in Sect. 2.1.1 for polyatomic molecules and in Sect. 3.1.1 for mixtures. Some classical results for the collision operators in Sects. 2.1.2 and 3.1.2, respectively, and the linearized collision operators in Sects. 2.1.3 and 3.1.3, respectively, are reviewed. Sections 2.2 and 3.2 are devoted to the main results of this paper, while the main proofs are addressed in Sects. 2.3 and 3.3; proofs of compactness of the integral operators $K$ are presented in Sects. 2.3.1 and 3.3.1, respectively, while proofs of the bounds on the collision frequencies appear in Sects. 2.3.2 and 3.3.2, respectively. Finally, the Appendix concerns a new (more basic, as well as, constructive) proof of a crucial - for the compactness in the mixture case - lemma in [10].

2 Polyatomic Molecules Modeled by a Discrete Internal Energy Variable

For a polyatomic gas, assuming that the translational and vibrational energies can be modelled by a single internal energy variable, one can either consider the internal energy variable to be discrete or continuous [11, 17, 20]. See also [8] where a general framework is exploited. Here will the case when the energy variable can take a finite number of different (given) values be considered.

2.1 Model

This section concerns the considered model for a polyatomic single species. A probabilistic formulation of the collision operator, inspired by one for monatomic single species [7, 23, 26], is considered. See also [21] for a probabilistic formulation of the collision operator for polyatomic gases. The relation to a more classical formulation [17, 20] is also accounted for. Known properties of the model and a corresponding linearized collision operator are also reviewed.

Consider a single species of polyatomic molecules with mass $m$, where the polyatomicity is modeled by $r$ different internal energies $I_{1},\ldots,I_{r}$. The internal energies $I_{i}$, $i\in \left \{ 1,\ldots,r\right \}$, are assumed to be nonnegative real numbers; $\left \{ I_{1},\ldots,I_{r}\right \} \subset$ $\mathbb{R}_{+}$. The distribution functions are of the form $f=\left ( f_{1},\ldots,f_{r}\right )$, where the component $f_{i}=f_{i}\left ( t,\mathbf{x},\boldsymbol{\xi }\right ) =f\left ( t,\mathbf{x}, \boldsymbol{\xi },I_{i}\right )$, $i\in \left \{ 1,\ldots,r\right \}$, with $t\in \mathbb{R}_{+}$, $\mathbf{x}=\left ( x,y,z\right ) \in \mathbb{R}^{3}$, and $\boldsymbol{\xi }=\left ( \xi _{x},\xi _{y},\xi _{z}\right ) \in \mathbb{R}^{3}$, is the distribution function for particles with internal energy $I_{i}$, $i\in \left \{ 1,\ldots,r\right \}$.

Moreover, consider the real Hilbert space $\mathcal{\mathfrak{h}}^{(r)}:=\left ( L^{2}\left ( d\boldsymbol{\xi }\right ) \right ) ^{r}$, with inner product

$$ \left ( f,g\right ) =\sum _{i=1}^{r}\int _{\mathbb{R}^{3}}f_{i}g_{i} \,d\boldsymbol{\xi }\text{ for }f,g\in \left ( L^{2}\left ( d \boldsymbol{\xi }\right ) \right ) ^{r}\text{.} $$

The evolution of the distribution functions is (in the absence of external forces) described by the (vector) Boltzmann equation

$$ \frac{\partial f}{\partial t}+\left ( \boldsymbol{\xi }\cdot \nabla _{ \mathbf{x}}\right ) f=Q\left ( f,f\right ) \text{,} $$

(1)

where the (vector) collision operator $Q=\left ( Q_{1},\ldots,Q_{r}\right )$ is a quadratic bilinear operator that accounts for the change of velocities and internal energies of particles due to binary collisions (assuming that the gas is rarefied, such that other collisions are negligible), and where each component $Q_{i}$ is the collision operator for the distribution function $f_{i}$, $i\in \left \{ 1,\ldots,r\right \}$.

A collision can be represented by two pre-collisional pairs, each pair consisting of a microscopic velocity and an internal energy, $\left ( \boldsymbol{\xi },I_{i}\right )$ and $\left ( \boldsymbol{\xi }_{\ast },I_{j}\right )$, and two corresponding post-collisional pairs, $\left ( \boldsymbol{\xi }^{\prime },I_{k}\right )$ and $\left ( \boldsymbol{\xi }_{\ast }^{\prime },I_{l}\right )$, for some $\left \{ i,j,k,l\right \} \subset \left \{ 1,\ldots,r\right \}$. The notation for pre- and post-collisional pairs may, of course, be interchanged as well. Due to momentum and total energy conservation, the following relations have to be satisfied by the pairs

$$\begin{aligned} \boldsymbol{\xi }+\boldsymbol{\xi }_{\ast } =&\boldsymbol{\xi }^{ \prime }+\boldsymbol{\xi }_{\ast }^{\prime } \\ \frac{m}{2}\left \vert \boldsymbol{\xi }\right \vert ^{2}+ \frac{m}{2}\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}+I_{i}+I_{j} =& \frac{m}{2}\left \vert \boldsymbol{\xi }^{\prime }\right \vert ^{2}+ \frac{m}{2}\left \vert \boldsymbol{\xi }_{\ast }^{\prime }\right \vert ^{2}+I_{k}+I_{l}\text{.} \end{aligned}$$

(2)

2.1.1 Collision Operator

The components of the (vector) collision operator $Q=\left ( Q_{1},\ldots,Q_{r}\right )$ can be written in the following form

$$ Q_{i}(f,f)=\sum _{j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3} \right ) ^{3}}W(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j} \left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{ \prime },I_{k},I_{l}\right . )\left ( \frac{f_{k}^{\prime }f_{l\ast }^{\prime }}{\varphi _{k}\varphi _{l}}- \frac{f_{i}f_{j\ast }}{\varphi _{i}\varphi _{j}}\right ) d\boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d \boldsymbol{\xi }_{\ast }^{\prime }\text{,} $$

(3)

for all $i\in \left \{ 1,\ldots,r\right \}$, for some constant $\varphi =\left ( \varphi _{1},\ldots,\varphi _{r}\right ) \in \mathbb{R}^{r}$. Here and below the abbreviations

$$ f_{i\ast }=f_{i}\left ( t,\mathbf{x,}\boldsymbol{\xi }_{\ast }\right ) \text{, }f_{i}^{\prime }=f_{i}\left ( t,\mathbf{x,}\boldsymbol{\xi }^{ \prime }\right ) \text{, and }f_{i\ast }^{\prime }=f_{i}\left ( t, \mathbf{x,}\boldsymbol{\xi }_{\ast }^{\prime }\right ) $$

(4)

are used. In the collision operator (3) the gain term - the term containing the product $f_{k}^{\prime }f_{l\ast }^{\prime }$ - accounts for the gain of particles with microscopic velocity $\boldsymbol{\xi }$ and internal energy $I_{i}$ (at time $t$ and position $\mathbf{x}$) - here $\left ( \boldsymbol{\xi },I_{i}\right )$ and $\left ( \boldsymbol{\xi }_{\ast },I_{j}\right )$ represent the post-collisional particles, while the loss term - the term containing the product $f_{i}f_{j\ast }$ - accounts for the loss of particles with microscopic velocity $\boldsymbol{\xi }$ and internal energy $I_{i}$ - here $\left ( \boldsymbol{\xi },I_{i}\right )$ and $\left ( \boldsymbol{\xi }_{\ast },I_{j}\right )$ represent the pre-collisional particles. The corresponding (signed) internal energy gap is

$$ \Delta I_{ij}^{kl}=I_{k}+I_{l}-I_{i}-I_{j}\text{.} $$

The transition probability $W:\left ( \mathbb{R}^{3}\times \left \{ I_{1},\ldots,I_{r}\right \} \right ) ^{4}\rightarrow \mathbb{R}_{+}:=[0,\infty )$ is of the form, cf. [21], as well as [7, 23, 26] for the monatomic case,

$$\begin{aligned} &W(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l} \right . ) \\ =&4m\varphi _{k}\varphi _{l}\sigma _{kl}^{ij}\left ( \left \vert \mathbf{g}^{\prime }\right \vert ,\left \vert \cos \theta \right \vert \right ) \frac{\left \vert \mathbf{g}^{\prime }\right \vert }{\left \vert \mathbf{g}\right \vert }\delta _{3} \left ( \boldsymbol{\xi }+\boldsymbol{\xi }_{\ast }-\boldsymbol{\xi }^{ \prime }-\boldsymbol{\xi }_{\ast }^{\prime }\right ) \\ &\times \delta _{1}\left ( \frac{m}{2}\left ( \left \vert \boldsymbol{\xi }\right \vert ^{2}+\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}- \left \vert \boldsymbol{\xi }^{\prime }\right \vert ^{2}-\left \vert \boldsymbol{\xi }_{\ast }^{\prime }\right \vert ^{2}\right ) -\Delta I_{ij}^{kl} \right ) \\ =&4m\varphi _{i}\varphi _{j}\sigma _{ij}^{kl}\left ( \left \vert \mathbf{g}\right \vert ,\left \vert \cos \theta \right \vert \right ) \frac{\left \vert \mathbf{g}\right \vert }{\left \vert \mathbf{g}^{\prime }\right \vert }\delta _{3} \left ( \boldsymbol{\xi }+\boldsymbol{\xi }_{\ast }-\boldsymbol{\xi }^{ \prime }-\boldsymbol{\xi }_{\ast }^{\prime }\right ) \\ &\times \delta _{1}\left ( \frac{m}{2}\left ( \left \vert \boldsymbol{\xi }\right \vert ^{2}+\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}- \left \vert \boldsymbol{\xi }^{\prime }\right \vert ^{2}-\left \vert \boldsymbol{\xi }_{\ast }^{\prime }\right \vert ^{2}\right ) -\Delta I_{ij}^{kl} \right ), \\ &\text{with } \sigma _{ij}^{kl}=\sigma _{ij}^{kl}\left ( \left \vert \mathbf{g}\right \vert ,\left \vert \cos \theta \right \vert \right ) >0 \text{ a.e., } \cos \theta = \frac{\mathbf{g}\cdot \mathbf{g}^{\prime }}{\left \vert \mathbf{g}\right \vert \left \vert \mathbf{g}^{\prime }\right \vert } \text{, } \\ &\mathbf{g}=\boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }\text{, } \mathbf{g}^{\prime }=\boldsymbol{\xi }^{\prime }-\boldsymbol{\xi }_{\ast }^{ \prime }\text{, and }\Delta I_{ij}^{kl}=I_{k}+I_{l}-I_{i}-I_{j} \end{aligned}$$

(5)

where $\delta _{3}$ and $\delta _{1}$ denote the Dirac’s delta function in $\mathbb{R}^{3}$ and ℝ, respectively; taking the conservation of momentum and total energy (2) into account. Here and below we use the (inconsistent) shorthanded expressions

$$\begin{aligned} \sigma _{ij}^{kl} =&\sigma (\boldsymbol{\xi },\boldsymbol{\xi }_{ \ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime }, \boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l}\right . )= \widetilde{\sigma }(\left \vert \mathbf{g}\right \vert ,\left \vert \cos \theta \right \vert ,I_{i},I_{j},I_{k},I_{l})\text{ and} \\ \sigma _{kl}^{ij} =&\sigma (\boldsymbol{\xi }^{\prime }, \boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l}\left \vert \boldsymbol{\xi }, \boldsymbol{\xi }_{\ast },I_{i},I_{j}\right . )=\widetilde{\sigma }(\left \vert \mathbf{g}^{\prime }\right \vert ,\left \vert \cos \theta \right \vert ,I_{k},I_{l},I_{i},I_{j}) \text{,} \end{aligned}$$

for a given scattering cross-section $\sigma :\left ( \left ( \mathbb{R}^{3}\right ) ^{2}\times \left \{ I_{1},\ldots,I_{r}\right \} ^{2}\right ) ^{2}\rightarrow \mathbb{R}_{+}$, or in the alternative form $\widetilde{\sigma }:\mathbb{R}_{+}\times \left [ 0,1\right ] \times \left \{ I_{1},\ldots,I_{r} \right \} ^{4}\rightarrow \mathbb{R}_{+}$; assuming the pairs $\left ( \boldsymbol{\xi },I_{i}\right )$, $\left ( \boldsymbol{\xi }_{\ast },I_{j}\right )$, $\left ( \boldsymbol{\xi }^{\prime },I_{k}\right )$, and $\left ( \boldsymbol{\xi }_{\ast }^{\prime },I_{l}\right )$ being given - here, by the arguments of $W$.

The scattering cross sections $\sigma _{ij}^{kl}$, $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots,r\right \}$, are assumed to satisfy the microreversibility conditions

$$ \varphi _{i}\varphi _{j}\left \vert \mathbf{g}\right \vert ^{2} \sigma _{ij}^{kl}\left ( \left \vert \mathbf{g}\right \vert ,\left \vert \cos \theta \right \vert \right ) =\varphi _{k}\varphi _{l} \left \vert \mathbf{g}^{\prime }\right \vert ^{2}\sigma _{kl}^{ij} \left ( \left \vert \mathbf{g}^{\prime }\right \vert ,\left \vert \cos \theta \right \vert \right ) \text{.} $$

(6)

Furthermore, to obtain invariance under exchange of particles in a collision, it is assumed that, the scattering cross sections $\sigma _{ij}^{kl}$, $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots,r\right \}$, satisfy the symmetry relations (fixing the pairs $\left ( \boldsymbol{\xi },I_{i}\right )$, $\left ( \boldsymbol{\xi }_{\ast },I_{j}\right )$, $\left ( \boldsymbol{\xi }^{\prime },I_{k}\right )$, and $\left ( \boldsymbol{\xi }_{\ast }^{\prime },I_{l}\right )$)

$$ \sigma _{ij}^{kl}=\sigma _{ij}^{lk}=\sigma _{ji}^{lk}\text{.} $$

(7)

The invariance under change of particles in a collision, which follows directly by the definition of the transition probability (5) and the symmetry relations (7) for the collision frequency, and the microreversibility of the collisions (6), implies that the transition probabilities (5) satisfy the relations

$$\begin{aligned} W(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l} \right . ) =&W(\boldsymbol{\xi }_{\ast },\boldsymbol{\xi },I_{j},I_{i}\left \vert \boldsymbol{\xi }_{\ast }^{\prime }, \boldsymbol{\xi }^{\prime },I_{l},I_{k}\right . ) \\ W(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l} \right . ) =&W(\boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l}\left \vert \boldsymbol{\xi }, \boldsymbol{\xi }_{\ast },I_{i},I_{j}\right . ) \\ W(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l} \right . ) =&W(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j} \left \vert \boldsymbol{\xi }_{\ast }^{\prime },\boldsymbol{\xi }^{\prime },I_{l},I_{k}\right . )\text{.} \end{aligned}$$

(8)

Applying known properties of Dirac’s delta function $\delta _{n}$ in $\mathbb{R}^{n}$, $n\in \left \{ 1,2,\ldots\right \}$, e.g., that $\delta _{n}\left ( a\mathbf{x}\right ) =\delta _{n}\left ( \mathbf{x}\right ) /\left \vert a\right \vert ^{n}$ for any non-zero real number $a$ and $\mathbf{x}\in \mathbb{R}^{n}$, the transition probabilities may - aiming to obtain expressions for $\mathbf{G}^{\prime }$ and $\left \vert \mathbf{g}^{\prime }\right \vert$ in the arguments of the delta-functions - be transformed to

$$\begin{aligned} &W(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l} \right . ) \\ =&4m\varphi _{k}\varphi _{l}\sigma _{kl}^{ij} \frac{\left \vert \mathbf{g}^{\prime }\right \vert }{\left \vert \mathbf{g}\right \vert }\delta _{3} \left ( 2\left ( \mathbf{G}-\mathbf{G}^{\prime }\right ) \right ) \delta _{1}\left ( \frac{m}{4}\left ( \left \vert \mathbf{g}\right \vert ^{2}-\left \vert \mathbf{g}^{\prime }\right \vert ^{2}\right ) -\Delta I_{ij}^{kl}\right ) \\ =&2\varphi _{k}\varphi _{l}\sigma _{kl}^{ij} \frac{\left \vert \mathbf{g}^{\prime }\right \vert }{\left \vert \mathbf{g}\right \vert }\delta _{3} \left ( \mathbf{G}-\mathbf{G}^{\prime }\right ) \delta _{1}\left ( \left \vert \mathbf{g}\right \vert ^{2}-\left \vert \mathbf{g}^{\prime }\right \vert ^{2} -\frac{4}{m}\Delta I_{ij}^{kl} \right ) \\ =&\varphi _{k}\varphi _{l}\sigma _{kl}^{ij} \frac{1}{\left \vert \mathbf{g}\right \vert }\mathbf{1}_{m\left \vert \mathbf{g}\right \vert ^{2}>4 \Delta I_{ij}^{kl}}\delta _{3}\left ( \mathbf{G}-\mathbf{G}^{\prime } \right ) \delta _{1}\left ( \sqrt{\left \vert \mathbf{g}\right \vert ^{2}- \frac{4}{m}\Delta I_{ij}^{kl}}-\left \vert \mathbf{g}^{\prime } \right \vert \right ) \\ =&\varphi _{i}\varphi _{j}\sigma _{ij}^{kl} \frac{\left \vert \mathbf{g}\right \vert }{\left \vert \mathbf{g}^{\prime }\right \vert ^{2}} \mathbf{1}_{m\left \vert \mathbf{g}\right \vert ^{2}>4\Delta I_{ij}^{kl}} \delta _{3}\left ( \mathbf{G}-\mathbf{G}^{\prime }\right ) \delta _{1} \left ( \sqrt{\left \vert \mathbf{g}\right \vert ^{2}-\frac{4}{m}\Delta I_{ij}^{kl}}-\left \vert \mathbf{g}^{\prime }\right \vert \right ) \text{,} \\ \text{ } &\text{with }\mathbf{G}= \frac{\boldsymbol{\xi }+\boldsymbol{\xi }_{\ast }}{2}\text{ and }\mathbf{G}^{\prime }= \frac{\boldsymbol{\xi }^{\prime }+\boldsymbol{\xi }_{\ast }^{\prime }}{2} \text{.} \end{aligned}$$

Remark 1

Note that, cf. [21],

$$ \delta _{1}\left ( \frac{m}{4}\left ( \left \vert \mathbf{g}\right \vert ^{2}-\left \vert \mathbf{g}^{\prime }\right \vert ^{2}\right ) - \Delta I_{ij}^{kl}\right ) =\delta _{1}\left ( E_{ij}-E_{kl}\right ) , $$

for $E_{ij}=\dfrac{m}{4}\left \vert \mathbf{g}\right \vert ^{2}+I_{i}+I_{j}$ and $E_{kl}=\dfrac{m}{4}\left \vert \mathbf{g}^{\prime }\right \vert ^{2}+I_{k}+I_{l}$.

By a change of variables $\left \{ \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime }\right \} \rightarrow \left \{ \mathbf{g}^{ \prime }=\boldsymbol{\xi }^{\prime }-\boldsymbol{\xi }_{\ast }^{\prime }, \mathbf{G}^{\prime }= \dfrac{\boldsymbol{\xi }^{\prime }+\boldsymbol{\xi }_{\ast }^{\prime }}{2} \right \}$, followed by that to spherical coordinates $\left \{ \mathbf{g}^{\prime }\right \} \rightarrow \left \{ \left \vert \mathbf{g}^{\prime }\right \vert ,\boldsymbol{\omega }= \dfrac{\mathbf{g}^{\prime }}{\left \vert \mathbf{g}^{\prime }\right \vert }\right \}$, noting that

$$ d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime }=d \mathbf{G}^{\prime }d\mathbf{g}^{\prime }=\left \vert \mathbf{g}^{\prime } \right \vert ^{2}d\mathbf{G}^{\prime }d\left \vert \mathbf{g}^{ \prime }\right \vert d\boldsymbol{\omega }\text{,} $$

(9)

the observation that

$$\begin{aligned} &Q_{i}(f,f) \\ =&\sum _{j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{2} \times \mathbb{R}_{+}\mathbb{\times S}^{2}}W(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime }, \boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l}\right . ) \\ &\times \left ( \frac{f_{k}^{\prime }f_{l\ast }^{\prime }}{\varphi _{k}\varphi _{l}}- \frac{f_{i}f_{j\ast }}{\varphi _{i}\varphi _{j}}\right ) \,\left \vert \mathbf{g}^{\prime }\right \vert ^{2}\,d\boldsymbol{\xi }_{ \ast }d\mathbf{G}^{\prime }d\left \vert \mathbf{g}^{\prime }\right \vert d\boldsymbol{\omega } \\ =&\sum _{j,k,l=1}^{r}\int _{\mathbb{R}^{3} \mathbb{\times S}^{2}}\left \vert \mathbf{g}\right \vert \sigma _{ij}^{kl}\left ( \left \vert \mathbf{g}\right \vert ,\cos \theta \right ) \left ( f_{k}^{ \prime }f_{l\ast }^{\prime } \frac{\varphi _{i}\varphi _{j}}{\varphi _{k}\varphi _{l}}-f_{i}f_{j\ast }\right ) \mathbf{1}_{m\left \vert \mathbf{g}\right \vert ^{2}>4\Delta I_{ij}^{kl}}\,d\boldsymbol{\xi }_{\ast }d \boldsymbol{\omega ,} \end{aligned}$$

where

$$ \left \{ \textstyle\begin{array}{l} \boldsymbol{\xi }^{\prime }= \dfrac{\boldsymbol{\xi }+\boldsymbol{\xi }_{\ast }}{2}+ \dfrac{\sqrt{\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }\right \vert ^{2}-\dfrac{4}{m}\Delta I_{ij}^{kl}}}{2} \omega =\mathbf{G}+\dfrac{\sqrt{\left \vert \mathbf{g}\right \vert ^{2}-\dfrac{4}{m}\Delta I_{ij}^{kl}}}{2} \omega \\ \boldsymbol{\xi }_{\ast }^{\prime }= \dfrac{\boldsymbol{\xi }+\boldsymbol{\xi }_{\ast }}{2}- \dfrac{\sqrt{\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }\right \vert ^{2}-\dfrac{4}{m}\Delta I_{ij}^{kl}}}{2}\omega = \mathbf{G}- \dfrac{\sqrt{\left \vert \mathbf{g}\right \vert ^{2}-\dfrac{4}{m}\Delta I_{ij}^{kl}}}{2} \omega \end{array}\displaystyle \right . \text{, }\omega \in S^{2}\text{,} $$

can be made, resulting in a more familiar form of the Boltzmann collision operator for polyatomic molecules modeled with a discrete energy variable, cf. e.g. [17, 20].

Remark 2

Note that, when considering spherical coordinates, we, maybe unconventionally, often represent the direction by a vector in $\mathbb{S}^{2}$, rather than with azimuthal and polar angels, still referring to it as spherical coordinates. By representing the direction by a unit vector, the sine of the polar angle will not appear as a factor in the Jacobian, resulting in the Jacobian to be the square of the radial length.

2.1.2 Collision Invariants and Maxwellian Distributions

The following lemma follows directly by the relations (8).

Lemma 1

For any $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots ,r\right \}$ the measure

$$ dA_{ij}^{kl}=W(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j} \left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{ \prime },I_{k},I_{l}\right . )\,d\boldsymbol{\xi }\,d \boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{ \prime } $$

is invariant under the interchanges of variables

$$\begin{aligned} \left ( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j} \right ) \leftrightarrow &\left ( \boldsymbol{\xi }^{\prime }, \boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l}\right ) \textit{,} \\ \left ( \boldsymbol{\xi },I_{i}\right ) \leftrightarrow &\left ( \boldsymbol{\xi }_{\ast },I_{j}\right ) \textit{, and} \\ \left ( \boldsymbol{\xi }^{\prime },I_{k}\right ) \leftrightarrow & \left ( \boldsymbol{\xi }_{\ast }^{\prime },I_{l}\right ) \textit{,} \end{aligned}$$

(10)

respectively.

The weak form of the collision operator $Q(f,f)$ reads

$$\begin{aligned} \left ( Q(f,f),g\right ) =&\sum _{i,j,k,l=1}^{r}\int _{ \left ( \mathbb{R}^{3}\right ) ^{4}}\left ( \frac{f_{k}^{\prime }f_{l\ast }^{\prime }}{\varphi _{k}\varphi _{l}}- \frac{f_{i}f_{j\ast }}{\varphi _{i}\varphi _{j}}\right ) g_{i}\,dA_{ij}^{kl} \\ =&\sum _{i,j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{4}} \left ( \frac{f_{k}^{\prime }f_{l\ast }^{\prime }}{\varphi _{k}\varphi _{l}}- \frac{f_{i}f_{j\ast }}{\varphi _{i}\varphi _{j}}\right ) g_{j\ast }\,dA_{ij}^{kl} \\ =&-\sum _{i,j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{4}}\left ( \frac{f_{k}^{\prime }f_{l\ast }^{\prime }}{\varphi _{k}\varphi _{l}}- \frac{f_{i}f_{j\ast }}{\varphi _{i}\varphi _{j}}\right ) g_{k}^{ \prime }\,dA_{ij}^{kl} \\ =&-\sum _{i,j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{4}}\left ( \frac{f_{k}^{\prime }f_{l\ast }^{\prime }}{\varphi _{k}\varphi _{l}}- \frac{f_{i}f_{j\ast }}{\varphi _{i}\varphi _{j}}\right ) g_{l\ast }^{ \prime }\,dA_{ij}^{kl} \end{aligned}$$

for any function $g=(g_{1},\ldots,g_{r})$, such that the first integrals are defined for all indices $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots ,r\right \}$, while the following equalities are obtained by applying Lemma 1.

We have the following proposition.

Proposition 1

Let $g=(g_{1},\ldots,g_{r})$ be such that

$$ \int _{\left ( \mathbb{R}^{3}\right ) ^{4}}\left ( \frac{f_{k}^{\prime }f_{l\ast }^{\prime }}{\varphi _{k}\varphi _{l}}- \frac{f_{i}f_{j\ast }}{\varphi _{i}\varphi _{j}}\right ) g_{i}\,dA_{ij}^{kl} $$

is defined for all $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots ,r\right \}$. Then

$$ \left ( Q(f,f),g\right ) =\frac{1}{4}\sum _{i,j,k,l=1}^{r} \int _{\left ( \mathbb{R}^{3}\right ) ^{4}}\left ( \frac{f_{k}^{\prime }f_{l\ast }^{\prime }}{\varphi _{k}\varphi _{l}}- \frac{f_{i}f_{j\ast }}{\varphi _{i}\varphi _{j}}\right ) \left ( g_{i}+g_{j\ast }-g_{k}^{\prime }-g_{l\ast }^{\prime } \right ) \,dA_{ij}^{kl}. $$

Definition 1

A function $g=(g_{1},\ldots,g_{r})$ is a collision invariant if

$$ \left ( g_{i}+g_{j\ast }-g_{k}^{\prime }-g_{l\ast }^{\prime }\right ) W(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l} \right . )=0\text{ a.e.} $$

for all $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots ,r\right \}$.

Clearly, $1_{r}$, $\xi _{x}1_{r}$, $\xi _{y}1_{r}$, $\xi _{z}1_{r}$, and $m\left \vert \boldsymbol{\xi }\right \vert ^{2}1_{r}+2I$, with $1_{r}=(1,\ldots,1)\in \mathbb{R}^{r}$ and the vector internal energy $I=(I_{1},\ldots,I_{r})$, are collision invariants - corresponding to conservation of mass, momentum, and total energy.

In fact, we have the following proposition, cf. [15, 20].

Proposition 2

The vector space of collision invariants is generated by

$$ \left \{ 1_{r},\xi _{x}1_{r},\xi _{y}1_{r},\xi _{z}1_{r},m\left \vert \boldsymbol{\xi }\right \vert ^{2}1_{r}+2I\right \} , $$

where $1_{r}=(1,\ldots,1)\in \mathbb{R}^{r}$ and $I=(I_{1},\ldots,I_{r})$.

Define

$$ \mathcal{W}\left [ f\right ] :=\left ( Q(f,f),\log \left ( \varphi ^{-1}f \right ) \right ) , $$

where $\varphi =\mathrm{diag}\left ( \varphi _{1},\ldots,\varphi _{r}\right )$. It follows by Proposition 1 that

$$ \mathcal{W}\left [ f\right ] =-\frac{1}{4}\sum _{i,j,k,l=1}^{r} \int _{\left ( \mathbb{R}^{3}\right ) ^{4}} \frac{f_{i}f_{j\ast }}{\varphi _{i}\varphi _{j}}\left ( \frac{\varphi _{i}\varphi _{j}f_{k}^{\prime }f_{l\ast }^{\prime }}{f_{i}f_{j\ast }\varphi _{k}\varphi _{l}}-1 \right ) \log \left ( \frac{\varphi _{i}\varphi _{j}f_{k}^{\prime }f_{l\ast }^{\prime }}{f_{i}f_{j\ast }\varphi _{k}\varphi _{l}}\right ) \,dA_{ij}^{kl} \text{.} $$

Since $\left ( x-1\right ) \mathrm{log}\left ( x\right ) \geq 0$ for all $x>0$, with equality if and only if $x=1$,

$$ \mathcal{W}\left [ f\right ] \leq 0\text{,} $$

with equality if and only if for all $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots ,r\right \}$

$$ \left ( \frac{f_{i}f_{j\ast }}{\varphi _{i}\varphi _{j}}- \frac{f_{k}^{\prime }f_{l\ast }^{\prime }}{\varphi _{k}\varphi _{l}} \right ) W(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{ \prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l}\right . )=0 \text{ a.e.,} $$

(11)

or, equivalently, if and only if

$$ Q(f,f)\equiv 0\text{.} $$

For any equilibrium, or Maxwellian, distribution $M=(M_{1},\ldots,M_{r})$, follows by equation (11), since $Q(M,M)\equiv 0$, that for all $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots ,r\right \}$

$$ \left ( \log \frac{M_{i}}{\varphi _{i}}+\log \frac{M_{j\ast }}{\varphi _{j}}-\log \frac{M_{k}^{\prime }}{\varphi _{k}}-\log \frac{M_{l\ast }^{\prime }}{\varphi _{l}}\right ) W(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j} \left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{ \prime },I_{k},I_{l}\right . )=0\text{ a.e. .} $$

Hence, $\log \left ( \varphi ^{-1}M\right ) =\left ( \log \dfrac{M_{1}}{\varphi _{1}},\ldots,\log \dfrac{M_{r}}{\varphi _{r}} \right )$ is a collision invariant, and the components of the Maxwellian distributions $M=(M_{1},\ldots,M_{r})$ are of the form

$$ M_{i}=\frac{n\varphi _{i}m^{3/2}}{\left ( 2\pi T\right ) ^{3/2}q}e^{-m\left \vert \boldsymbol{\xi }-\mathbf{u}\right \vert ^{2}/2T}e^{-I_{i}/T}\text{.} $$

Here $n=\left ( M,1_{r}\right )$, $\mathbf{u}=\dfrac{1}{n}\left ( M,\boldsymbol{\xi }1_{r}\right )$, while $q=\sum \limits _{i=1}^{r}\varphi _{i}e^{-I_{i}/T}$, with $T=\dfrac{m}{3n}\left ( M,\left \vert \boldsymbol{\xi }-\mathbf{u} \right \vert ^{2}1_{r}\right )$, where $1_{r}=(1,\ldots,1)\in \mathbb{R}^{r}$.

Note that by equation (11) any Maxwellian distribution $M=(M_{1},\ldots,M_{r})$ satisfies the relations

$$ \left ( \frac{M_{k}^{\prime }M_{l\ast }^{\prime }}{\varphi _{k}\varphi _{l}}-\frac{M_{i}M_{j\ast }}{\varphi _{i}\varphi _{j}}\right ) W( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{ \prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l}\right . )=0 \text{ a.e. } $$

(12)

for all $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots ,r\right \}$.

Remark 3

Introducing the ℋ-functional

$$ \mathcal{H}\left [ f\right ] =\left ( f,\log f\right ) \text{,} $$

an ℋ-theorem can be obtained.

2.1.3 Linearized Collision Operator

Consider a deviation of a Maxwellian distribution $M=(M_{1},\ldots,M_{r})$, with components $M_{i}=\dfrac{\varphi _{i}m^{3/2}}{\left ( 2\pi \right ) ^{3/2}}e^{-m\left \vert \boldsymbol{\xi }\right \vert ^{2}/2}e^{-I_{i}}$, of the form

$$ f=M+M^{1/2}h. $$

(13)

Insertion in the Boltzmann equation (1) results in the system

$$ \frac{\partial h}{\partial t}+\left ( \boldsymbol{\xi }\cdot \nabla _{ \mathbf{x}}\right ) h+\mathcal{L}h=\Gamma \left ( h,h\right ) \text{.} $$

(14)

The components of the linearized collision operator $\mathcal{L}=\left ( \mathcal{L}_{1},\ldots,\mathcal{L}_{r}\right )$ are given by

$$\begin{aligned} \mathcal{L}_{i}h =&-M_{i}^{-1/2}\left ( Q_{i}(M,\mathcal{M}^{1/2}h)+Q_{i}(\mathcal{M}^{1/2}h,M)\right ) \\ =&M_{i}^{-1/2}\sum _{j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3} \right ) ^{3}}\left ( \frac{M_{i}M_{j\ast }M_{k}^{\prime }M_{l\ast }^{\prime }}{\varphi _{i}\varphi _{j}\varphi _{k}\varphi _{l}}\right ) ^{1/2}W( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l}\right . ) \\ &\times \left ( \frac{h_{i}}{M_{i}^{1/2}}+ \frac{h_{j\ast }}{M_{j\ast }^{1/2}}-\frac{h_{k}^{\prime }}{\left ( M_{k}^{\prime }\right ) ^{1/2}}- \frac{h_{l\ast }^{\prime }}{\left ( M_{l\ast }^{\prime }\right ) ^{1/2}} \right ) \,d\boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d \boldsymbol{\xi }_{\ast }^{\prime } \\ =&\nu _{i}h_{i}-K_{i}\left ( h\right ) \text{, where }\mathcal{M}= \mathrm{diag}\left ( M_{1},\ldots,M_{r}\right ) , \end{aligned}$$

(15)

with

$$\begin{aligned} \nu _{i} =&\sum _{j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3} \right ) ^{3}}\frac{M_{j\ast }}{\varphi _{i}\varphi _{j}}W(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime }, \boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l}\right . )\,d\boldsymbol{\xi }_{ \ast }d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime } \text{, and} \\ K_{i}\left ( h\right ) =&M_{i}^{-1/2}\!\!\sum _{j,k,l=1}^{r} \int _{\left ( \mathbb{R}^{3}\right ) ^{3}}\!\left ( \frac{M_{i}M_{j\ast }M_{k}^{\prime }M_{l\ast }^{\prime }}{\varphi _{i}\varphi _{j}\varphi _{k}\varphi _{l}} \right ) ^{1/2}\!\!W(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j} \left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{ \prime },I_{k},I_{l}\right . ) \\ &\times \left ( \frac{h_{k}^{\prime }}{\left ( M_{k}^{\prime }\right ) ^{1/2}}+\frac{h_{l\ast }^{\prime }}{\left ( M_{l\ast }^{\prime }\right ) ^{1/2}}- \frac{h_{j\ast }}{M_{j\ast }^{1/2}}\right ) \,d\boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime } \text{,} \end{aligned}$$

(16)

for all $i\in \left \{ 1,\ldots ,r\right \}$. The components of the nonlinear (quadratic) term $\Gamma =\left ( \Gamma _{1},\ldots,\Gamma _{r}\right )$ are given by

$$ \Gamma _{i}\left ( h,h\right ) =M_{i}^{-1/2}Q_{i}(\mathcal{M}^{1/2}h, \mathcal{M}^{1/2}h)\text{.} $$

(17)

The multiplication operator $\Lambda$ defined by

$$ \Lambda (f)=\nu f\text{, where }\nu =\mathrm{diag}\left ( \nu _{1},\ldots, \nu _{r}\right ) \text{,} $$

is a closed, densely defined, self-adjoint operator on $\left ( L^{2}\left ( d\boldsymbol{\xi }\right ) \right ) ^{r}$. It is Fredholm, as well, if and only if $\Lambda$ is coercive.

The following lemma follows immediately by Lemma 1.

Lemma 2

For any $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots ,r\right \}$ the measure

$$ d\widetilde{A}_{ij}^{kl}=\left ( \frac{M_{i}M_{j\ast }M_{k}^{\prime }M_{l\ast }^{\prime }}{\varphi _{i}\varphi _{j}\varphi _{k}\varphi _{l}} \right ) ^{1/2}dA_{ij}^{kl} $$

is invariant under the interchanges (10) of variables respectively.

The weak form of the linearized collision operator ℒ reads

$$\begin{aligned} &\left ( \mathcal{L}h,g\right ) \\ =&\sum _{i,j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{4}} \left ( \frac{h_{i}}{M_{i}^{1/2}}+\frac{h_{j\ast }}{M_{j\ast }^{1/2}}- \frac{h_{k}^{\prime }}{\left ( M_{k}^{\prime }\right ) ^{1/2}}- \frac{h_{l\ast }^{\prime }}{\left ( M_{l\ast }^{\prime }\right ) ^{1/2}} \right ) \frac{g_{i}}{M_{i}^{1/2}}\,d\widetilde{A}_{ij}^{kl} \\ =&\sum _{i,j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{4}} \left ( \frac{h_{i}}{M_{i}^{1/2}}+\frac{h_{j\ast }}{M_{j\ast }^{1/2}}- \frac{h_{k}^{\prime }}{\left ( M_{k}^{\prime }\right ) ^{1/2}}- \frac{h_{l\ast }^{\prime }}{\left ( M_{l\ast }^{\prime }\right ) ^{1/2}} \right ) \frac{g_{j\ast }}{M_{j\ast }^{1/2}}\,d\widetilde{A}_{ij}^{kl} \\ =&-\sum _{i,j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{4}}\left ( \frac{h_{i}}{M_{i}^{1/2}}+ \frac{h_{j\ast }}{M_{j\ast }^{1/2}}-\frac{h_{k}^{\prime }}{\left ( M_{k}^{\prime }\right ) ^{1/2}}- \frac{h_{l\ast }^{\prime }}{\left ( M_{l\ast }^{\prime }\right ) ^{1/2}} \right ) \frac{g_{k}^{\prime }}{\left ( M_{k}^{\prime }\right ) ^{1/2}}\,d \widetilde{A}_{ij}^{kl} \\ =&-\sum _{i,j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{4}}\left ( \frac{h_{i}}{M_{i}^{1/2}}+ \frac{h_{j\ast }}{M_{j\ast }^{1/2}}-\frac{h_{k}^{\prime }}{\left ( M_{k}^{\prime }\right ) ^{1/2}}- \frac{h_{l\ast }^{\prime }}{\left ( M_{l\ast }^{\prime }\right ) ^{1/2}} \right ) \frac{g_{l\ast }^{\prime }}{\left ( M_{l\ast }^{\prime }\right ) ^{1/2}}\,d\widetilde{A}_{ij}^{kl}, \end{aligned}$$

for any function $g=(g_{1},\ldots,g_{r})$, such that the first integrals are defined for all indices $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots ,r\right \}$, while the following equalities are obtained by applying Lemma 2.

We have the following lemma.

Lemma 3

Let $g=(g_{1},\ldots,g_{r})$ be such that

$$ \int _{\left ( \mathbb{R}^{3}\right ) ^{4}}\left ( \frac{h_{i}}{M_{i}^{1/2}}+\frac{h_{j\ast }}{M_{j\ast }^{1/2}}- \frac{h_{k}^{\prime }}{\left ( M_{k}^{\prime }\right ) ^{1/2}}- \frac{h_{l\ast }^{\prime }}{\left ( M_{l\ast }^{\prime }\right ) ^{1/2}} \right ) \frac{g_{i}}{M_{i}^{1/2}}\,d\widetilde{A}_{ij}^{kl} $$

is defined for all $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots ,r\right \}$. Then

$$\begin{aligned} \left ( \mathcal{L}h,g\right ) =&\frac{1}{4}\sum _{i,j,k,l=1}^{r} \int _{\left ( \mathbb{R}^{3}\right ) ^{4}}\left ( \frac{h_{i}}{M_{i}^{1/2}}+ \frac{h_{j\ast }}{M_{j\ast }^{1/2}}- \frac{h_{k}^{\prime }}{\left ( M_{k}^{\prime }\right ) ^{1/2}}- \frac{h_{l\ast }^{\prime }}{\left ( M_{l\ast }^{\prime }\right ) ^{1/2}} \right ) \\ &\times \left ( \frac{g_{i}}{M_{i}^{1/2}}+ \frac{g_{j\ast }}{M_{j\ast }^{1/2}}-\frac{g_{k}^{\prime }}{\left ( M_{k}^{\prime }\right ) ^{1/2}}- \frac{g_{l\ast }^{\prime }}{\left ( M_{l\ast }^{\prime }\right ) ^{1/2}} \right ) d\widetilde{A}_{ij}^{kl}. \end{aligned}$$

Proposition 3

The linearized collision operator is symmetric and nonnegative,

$$ \left ( \mathcal{L}h,g\right ) =\left ( h,\mathcal{L}g\right ) \textit{ and }\left ( \mathcal{L}h,h\right ) \geq 0\textit{,} $$

and the kernel of ℒ, $\ker \mathcal{L}$, is generated by

$$ \left \{ M^{1/2},\xi _{x}M^{1/2},\xi _{y}M^{1/2},\xi _{z}M^{1/2},m \left \vert \boldsymbol{\xi }\right \vert ^{2}M^{1/2}+2\mathcal{M}^{1/2}I \right \} , $$

where $I=\left ( I_{1},\ldots,I_{r}\right )$ and $\mathcal{M}=\mathrm{diag}\left ( M_{1},\ldots,M_{r}\right )$.

Proof

By Lemma 3, it is immediate that $\left ( \mathcal{L}h,g\right ) =\left ( h,\mathcal{L}g\right )$, and

$$ \left ( \mathcal{L}h,h\right ) =\frac{1}{4}\sum _{i,j,k,l=1}^{r} \int _{\left ( \mathbb{R}^{3}\right ) ^{4}}\left ( \frac{h_{i}}{M_{i}^{1/2}}+ \frac{h_{j\ast }}{M_{j\ast }^{1/2}}- \frac{h_{k}^{\prime }}{\left ( M_{k}^{\prime }\right ) ^{1/2}}- \frac{h_{l\ast }^{\prime }}{\left ( M_{l\ast }^{\prime }\right ) ^{1/2}} \right ) ^{2}d\widetilde{A}_{ij}^{kl}\geq 0. $$

Furthermore, $h\in \ker \mathcal{L}$ if and only if $\left ( \mathcal{L}h,h\right ) =0$, which will be fulfilled if and only if for all $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots ,r\right \}$

$$ \left ( \frac{h_{i}}{M_{i}^{1/2}}+\frac{h_{j\ast }}{M_{j\ast }^{1/2}}- \frac{h_{k}^{\prime }}{\left ( M_{k}^{\prime }\right ) ^{1/2}}- \frac{h_{l\ast }^{\prime }}{\left ( M_{l\ast }^{\prime }\right ) ^{1/2}} \right ) W(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l}\right . ) \,=0\text{ a.e.,} $$

i.e., if and only if $\mathcal{M}^{-1/2}h$ is a collision invariant. The last part of the lemma now follows by Proposition 2. □

Remark 4

A property of the nonlinear term - although of no relevance to the studies here, still mentioned due to its importance - is that the nonlinear term is orthogonal to the kernel of ℒ, i.e. $\Gamma \left ( h,h\right ) \in \left ( \ker \mathcal{L}\right ) ^{ \perp _{\mathcal{\mathfrak{h}}^{(r)}}}$.

This follows, since any element in $\ker \mathcal{L}$ is of the form $\mathcal{M}^{1/2}g$ for some collision invariant $g$, while for any collision invariant $g$

$$\begin{aligned} \left ( \Gamma \left ( h,h\right ) ,\mathcal{M}^{1/2}g\right ) =& \left ( \mathcal{M}^{-1/2}Q(\mathcal{M}^{1/2}h,\mathcal{M}^{1/2}h), \mathcal{M}^{1/2}g\right ) \\ =&\left ( Q(\mathcal{M}^{1/2}h,\mathcal{M}^{1/2}h),g\right ) =0 \text{.} \end{aligned}$$

2.2 Main Results

This section is devoted to the main results, concerning a compactness property in Theorem 1 and bounds of collision frequencies in Theorem 2.

Assume that for some positive number $\gamma$, such that $0<\gamma <1$, there is a positive constant $C$ such that

$$ 0\leq \sigma _{ij}^{kl}\left ( \left \vert \mathbf{g}\right \vert , \left \vert \cos \theta \right \vert \right ) \leq \frac{C}{\left \vert \mathbf{g}\right \vert ^{2}}\left ( \Psi _{ij}^{kl}+\left ( \Psi _{ij}^{kl} \right ) ^{\gamma /2}\right ) \text{, with }\Psi _{ij}^{kl}=\left \vert \mathbf{g}\right \vert \sqrt{\left \vert \mathbf{g}\right \vert ^{2}- \frac{4}{m}\Delta I_{ij}^{kl}}\text{,} $$

(18)

for $\left \vert \mathbf{g}\right \vert ^{2}>4\Delta I_{ij}^{kl}/m$ on the scattering cross sections $\sigma _{ij}^{kl}$, $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots,r\right \} \!$. Then the following result may be obtained.

Theorem 1

Assume that the scattering cross sections $\sigma _{ij}^{kl}$ for all $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots,r\right \}$, satisfy the bound (18) for some positive number $\gamma$, such that $0<\gamma <1$.

Then the operator $K=\left ( K_{1},\ldots,K_{r}\right )$, with the components $K_{i}$ given by (16) is a self-adjoint compact operator on $\left ( L^{2}\left ( d\boldsymbol{\xi }\right ) \right ) ^{r}$.

Theorem 1 will be proven in Sect. 2.3.1, based on ideas from the corresponding proof for monatomic single species by Grad [19], especially, as presented by Glassey [18]. However, by starting from the probabilistic formulation, there is no initial parametrization of the velocities based on the momentum and total (kinetic plus internal) energy conservation. Then the parametrization can be chosen from scratch to be more suitable from case to case. From our point of view, this really simplifies to find appropriate substitutions, by geometrical motivations and manipulations of the delta-functions, for the non-trivial cases.

Corollary 1

The linearized collision operator ℒ, with scattering cross sections satisfying (18), is a closed, densely defined, self-adjoint operator on $\left ( L^{2}\left ( d\boldsymbol{\xi }\right ) \right ) ^{r}$.

Proof

By Theorem 1, the linear operator $\mathcal{L}=\Lambda -K$, with the multiplication operator given by $\Lambda f=\nu f$ for $\nu =\mathrm{diag}\left ( \nu _{1},\ldots,\nu _{s}\right )$, is closed as the sum of a closed and a bounded operator, and densely defined, since the domains of the linear operators ℒ and $\Lambda$ are equal; $D(\mathcal{L})=D(\Lambda )$. Furthermore, it is a self-adjoint operator, since the set of self-adjoint operators is closed under addition of bounded self-adjoint operators, see Theorem 4.3 of Chapter V in [22]. □

Now consider the scattering cross sections - cf. hard sphere models -

$$ \sigma _{ij}^{kl}=C \dfrac{\sqrt{\left \vert \mathbf{g}\right \vert ^{2}-\frac{4}{m}\Delta I_{ij}^{kl}}}{\left \vert \mathbf{g}\right \vert \varphi _{i}\varphi _{j}} \text{ if }\left \vert \mathbf{g}\right \vert ^{2}>\frac{4}{m}\Delta I_{ij}^{kl}\text{,} $$

(19)

for some positive constant $C>0$ and all $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots,r\right \}$.

In fact, it would be enough with the bounds

$$ C_{-} \dfrac{\sqrt{\left \vert \mathbf{g}\right \vert ^{2}-\frac{4}{m}\Delta I_{ij}^{kl}}}{\left \vert \mathbf{g}\right \vert \varphi _{i}\varphi _{j}} \leq \sigma _{ij}^{kl}\leq C_{+} \dfrac{\sqrt{\left \vert \mathbf{g}\right \vert ^{2}-\frac{4}{m}\Delta I_{ij}^{kl}}}{\left \vert \mathbf{g}\right \vert \varphi _{i}\varphi _{j}} \text{ if }\left \vert \mathbf{g}\right \vert ^{2}>\frac{4}{m}\Delta I_{ij}^{kl}\text{,} $$

(20)

for some positive constants $C_{\pm }>0$ and all $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots,r\right \}$, on the scattering cross sections.

Theorem 2

The linearized collision operator ℒ, with scattering cross sections (19) (or (20)), can be decomposed as a positive multiplication operator $\Lambda$, defined by $\Lambda f=\nu f$, where $\nu =\nu (\left \vert \boldsymbol{\xi }\right \vert )=\mathrm{diag}\left ( \nu _{1},\ldots,\nu _{r}\right )$, minus a compact operator $K$ on $\left ( L^{2}\left ( d\boldsymbol{\xi }\right ) \right ) ^{r}$

$$ \mathcal{L}=\Lambda -K, $$

(21)

where there exist positive numbers $\nu _{-}$ and $\nu _{+}$, $0<\nu _{-}<\nu _{+}$, such that for any $i\in \left \{ 1,\ldots,r\right \}$

$$ \nu _{-}\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right ) \leq \nu _{i}(\left \vert \boldsymbol{\xi }\right \vert )\leq \nu _{+} \left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right ) \textit{ for all }\boldsymbol{\xi }\in \mathbb{R}^{3}\textit{.} $$

(22)

The decomposition (21) follows by the decomposition (15), (16) and Theorem 1, while the bounds (22) are proven in Sect. 2.3.2.

Corollary 2

The linearized collision operator ℒ, with scattering cross sections (19) (or (20)), is a Fredholm operator, with domain

$$ D(\mathcal{L})=\left ( L^{2}\left ( \left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right ) d\boldsymbol{\xi }\right ) \right ) ^{r} \textit{.} $$

Proof

By Theorem 2 the multiplication operator $\Lambda$ is coercive and, hence, a Fredholm operator. The set of Fredholm operators is closed under addition of compact operators, see Theorem 5.26 of Chapter IV in [22] and its proof, so, by Theorem 2, ℒ is a Fredholm operator. □

Corollary 3

For the linearized collision operator ℒ, with scattering cross sections (19) (or (20)), there exists a positive number $\lambda$, $0<\lambda <1$, such that

$$ \left ( h,\mathcal{L}h\right ) \geq \lambda \left ( h,\nu (\left \vert \boldsymbol{\xi }\right \vert )h\right ) \geq \lambda \nu _{-} \left ( h,\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right ) h\right ) $$

for any $h\in \left ( L^{2}(\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right ) d\boldsymbol{\xi })\right ) ^{r}\cap \mathrm{Im} \mathcal{L}$.

Proof

Let $h\in \left ( L^{2}(\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right ) d\boldsymbol{\xi })\right ) ^{r}\cap \left ( \mathrm{ker}\mathcal{L}\right ) ^{\perp }=\left ( L^{2}(\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right ) d\boldsymbol{\xi })\right ) ^{r}\cap \mathrm{Im}\mathcal{L}$. As a Fredholm operator, ℒ is closed with a closed range, and as a compact operator, $K$ is bounded, and so there are positive constants $\nu _{0}>0$ and $c_{K}>0$, such that

$$ (h,\mathcal{L}h)\geq \nu _{0}(h,h)\text{ and }(h,Kh)\leq c_{K}(h,h). $$

Let $\lambda =\dfrac{\nu _{0}}{\nu _{0}+c_{K}}$. Then

$$\begin{aligned} (h,\mathcal{L}h) =&(1-\lambda )(h,\mathcal{L}h)+\lambda (h,(\nu ( \left \vert \boldsymbol{\xi }\right \vert )-K)h) \\ \geq &(1-\lambda )\nu _{0}(h,h)+\lambda (h,\nu (\left \vert \boldsymbol{\xi }\right \vert )h)-\lambda c_{K}(h,h) \\ =&(\nu _{0}-\lambda (\nu _{0}+c_{K}))(h,h)+\lambda (h,\nu (\left \vert \boldsymbol{\xi }\right \vert )h)=\lambda (h,\nu (\left \vert \boldsymbol{\xi }\right \vert )h)\text{.} \end{aligned}$$

 □

Remark 5

By Proposition 3 and Corollary 1–3 the linearized operator ℒ fulfills the properties assumed on the linear operators in [4], and hence, the results therein can be applied to hard sphere like models.

2.3 Compactness and Bounds on the Collision Frequency

This section is devoted to the proofs of the compactness property in Theorem 1 and the bounds on the collision frequency in Theorem 2 of the linearized collision operator for polyatomic molecules modeled with a discrete number of internal energies. Note that throughout this section $C$ will denote a generic positive constant.

To show the compactness property we will apply the following result.

Denote, for any (non-zero) natural number $N$,

$$ \mathfrak{h}_{N}:=\left \{ (\boldsymbol{\xi },\boldsymbol{\xi }_{ \ast })\in \left ( \mathbb{R}^{3}\right ) ^{2}:\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }\right \vert \geq \frac{1}{N}\text{; }\left \vert \boldsymbol{\xi }\right \vert \leq N\right \} $$

and

$$ b^{(N)}=b^{(N)}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }):=b( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\mathbf{1}_{\mathfrak{h}_{N}}\text{.} $$

Then we have the following lemma by Glassey [18], that will be of practical use for us to obtain compactness.

Lemma 4

(Glassey [18, Lemma 3.5.1], Drange [16])

Assume that $b(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\geq 0$ and let $Tf\left ( \boldsymbol{\xi }\right ) =\int _{\mathbb{R}^{3}}b( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast })f\left ( \boldsymbol{\xi }_{\ast }\right ) \,d \boldsymbol{\xi }_{\ast }$.

Then $T$ is compact on $L^{2}\left ( d\boldsymbol{\xi \,}\right )$ if

(i) $\int _{\mathbb{R}^{3}}b(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }) \,d \boldsymbol{\xi }$ is bounded in $\boldsymbol{\xi }_{\ast }$;

(ii) $b^{(N)}\in L^{2}\left ( d\boldsymbol{\xi \,}d\boldsymbol{\xi }_{ \ast }\right )$ for any (non-zero) natural number $N$;

(iii) $\underset{\boldsymbol{\xi }\in \mathbb{R}^{3}}{\sup }\int _{ \mathbb{R}^{3}}b(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })-b^{(N)}( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\,d\boldsymbol{\xi }_{\ast }\rightarrow 0$ as $N\rightarrow \infty$.

Then the operator $T$ is the uniform limit of Hilbert-Schmidt integral operators [18] and we say that the kernel $b(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })$ is approximately Hilbert-Schmidt, while $T$ is an approximately Hilbert-Schmidt integral operator. The reader is referred to Lemma 3.5.1 in [18] by Glassey for a proof of Lemma 4.

2.3.1 Compactness

This section concerns the proof of Theorem 1. Note that in the proof the kernels are rewritten in such a way that $\boldsymbol{\xi }_{\ast }$ - and not $\boldsymbol{\xi }^{\prime }$ and $\boldsymbol{\xi }_{\ast }^{\prime }$ - always will be the argument of the distribution functions. Then there will be essentially two different types of kernels; either $\boldsymbol{\xi }_{\ast }$ is an argument in the loss term (like $\boldsymbol{\xi }$) or in the gain term (unlike $\boldsymbol{\xi }$) of the collision operator. The kernels of the terms from the loss part of the collision operator will be shown to be Hilbert-Schmidt in a quite direct way, while the kernels of the terms from the gain parts of the collision operators will be shown to be the uniform limit of Hilbert-Schmidt integral operators, i.e. approximately Hilbert-Schmidt in the sense of Lemma 4.

Proof

For $i\in \left \{ 1,\ldots,r\right \}$, rewrite expression (16) as

$$\begin{aligned} K_{i}h =&M_{i}^{-1/2}\sum _{j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{3}}w(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j} \left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{ \prime },I_{k},I_{l}\right . ) \\ &\times \left ( \frac{h_{k}^{\prime }}{\left ( M_{k}^{\prime }\right ) ^{1/2}}+\frac{h_{l\ast }^{\prime }}{\left ( M_{l\ast }^{\prime }\right ) ^{1/2}}- \frac{h_{j\ast }}{M_{j\ast }^{1/2}}\right ) \,d\boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime } \text{,} \end{aligned}$$

with

$$ w(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l} \right . )=\left ( \frac{M_{i}M_{j\ast }M_{k}^{\prime }M_{l\ast }^{\prime }}{\varphi _{i}\varphi _{j}\varphi _{k}\varphi _{l}} \right ) ^{1/2}W(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j} \left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{ \prime },I_{k},I_{l}\right . ) $$

for all $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots,r\right \}$. Due to relations (8), the relations

$$\begin{aligned} w(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l} \right . ) =&w(\boldsymbol{\xi }_{\ast },\boldsymbol{\xi },I_{j},I_{i}\left \vert \boldsymbol{\xi }_{\ast }^{\prime }, \boldsymbol{\xi }^{\prime },I_{l},I_{k}\right . ) \\ w(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l} \right . ) =&w(\boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l}\left \vert \boldsymbol{\xi }, \boldsymbol{\xi }_{\ast },I_{i},I_{j}\right . ) \\ w(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l} \right . ) =&w(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }\boldsymbol{,}I_{i},I_{j}\left \vert \boldsymbol{\xi }_{\ast }^{ \prime },\boldsymbol{\xi }^{\prime },I_{l},I_{k}\right . ) \end{aligned}$$

(23)

are satisfied for all $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots,r\right \}$. Hence, for any $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots,r\right \}$, by first renaming $\left \{ \boldsymbol{\xi }_{\ast },I_{j}\right \} \leftrightarrows \left \{ \boldsymbol{\xi }^{\prime },I_{k}\right \}$ and then renaming $\left \{ \boldsymbol{\xi }_{\ast },I_{j}\right \} \leftrightarrows \left \{ \boldsymbol{\xi }_{\ast }^{\prime },I_{l}\right \}$, followed by applying the last relation in (23),

$$\begin{aligned} &\sum _{j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{3}}w(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l} \right . )\, \frac{h_{l\ast }^{\prime }}{\left ( M_{l\ast }^{\prime }\right ) ^{1/2}} \,d\boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime } \\ =&\sum _{j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{3}}w(\boldsymbol{\xi },\boldsymbol{\xi }^{\prime },I_{i},I_{k}\left \vert \boldsymbol{\xi }_{\ast },\boldsymbol{\xi }_{\ast }^{\prime },I_{j},I_{l} \right . )\, \frac{h_{l\ast }^{\prime }}{\left ( M_{l\ast }^{\prime }\right ) ^{1/2}} \,d\boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime } \\ =&\sum _{j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{3}}w(\boldsymbol{\xi },\boldsymbol{\xi }^{\prime },I_{i},I_{k}\left \vert \boldsymbol{\xi }_{\ast }^{\prime },\boldsymbol{\xi }_{\ast },I_{l},I_{j} \right . )\,\frac{h_{j\ast }}{M_{j\ast }^{1/2}}\,d\boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{ \prime } \\ =&\sum _{j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{3}}w(\boldsymbol{\xi },\boldsymbol{\xi }^{\prime },I_{i},I_{k}\left \vert \boldsymbol{\xi }_{\ast },\boldsymbol{\xi }_{\ast }^{\prime },I_{j},I_{l} \right . )\,\frac{h_{j\ast }}{M_{j\ast }^{1/2}}\,d\boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{ \prime }\text{.} \end{aligned}$$

As well, for any $\left \{ i,j,k,l\right \} \subseteq \left \{ 1,\ldots,r\right \}$, by renaming $\left \{ \boldsymbol{\xi }_{\ast },I_{j}\right \} \leftrightarrows \left \{ \boldsymbol{\xi }^{\prime },I_{k}\right \}$,

$$\begin{aligned} &\sum _{j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{3}}w(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l} \right . )\, \frac{h_{k}^{\prime }}{\left ( M_{k}^{\prime }\right ) ^{1/2}}\,d\boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d \boldsymbol{\xi }_{\ast }^{\prime } \\ =&\sum _{j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{3}}w(\boldsymbol{\xi },\boldsymbol{\xi }^{\prime },I_{i},I_{k}\left \vert \boldsymbol{\xi }_{\ast },\boldsymbol{\xi }_{\ast }^{\prime },I_{j},I_{l} \right . )\,\frac{h_{j\ast }}{M_{j\ast }^{1/2}}\,d\boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{ \prime }\text{.} \end{aligned}$$

It follows that for any $i\in \left \{ 1,\ldots,r\right \}$

$$\begin{aligned} K_{i}\left ( h\right ) =&\sum _{j=1}^{r}\int _{\mathbb{R}^{3}}k_{ij}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\,h_{j\ast }\,d \boldsymbol{\xi }_{\ast }\text{, where } \\ k_{ij}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }) =&k_{ij2}( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast })-k_{ij1}(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast })\text{, with} \\ k_{ij1}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }) =&\left ( M_{i}M_{j \ast }\right ) ^{-1/2}\sum _{k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{2}}w(\boldsymbol{\xi },\boldsymbol{\xi }_{ \ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime }, \boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l}\right . )\,d \boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime } \text{ and} \\ k_{ij2}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }) =&2\left ( M_{i}M_{j \ast }\right ) ^{-1/2}\sum _{k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{2}}w(\boldsymbol{\xi },\boldsymbol{\xi }^{\prime },I_{i},I_{k} \left \vert \boldsymbol{\xi }_{\ast },\boldsymbol{\xi }_{\ast }^{ \prime },I_{j},I_{l}\right . )\,d\boldsymbol{\xi }^{\prime }d \boldsymbol{\xi }_{\ast }^{\prime }\text{.} \end{aligned}$$

(24)

Next we obtain some symmetry relations that will help to yield self-adjointness of the operator $K$ below. Indeed, for any $\left \{ i,j\right \} \subseteq \left \{ 1,\ldots,r\right \}$,

$$ k_{ij}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })=k_{ji2}( \boldsymbol{\xi }_{\ast },\boldsymbol{\xi })-k_{ji1}(\boldsymbol{\xi }_{\ast }, \boldsymbol{\xi })=k_{ji}(\boldsymbol{\xi }_{\ast },\boldsymbol{\xi }), $$

since, by applying the first and the last relation in (23),

$$\begin{aligned} k_{ij1}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }) =&\left ( M_{i}M_{j \ast }\right ) ^{-1/2}\sum _{k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{2}}w(\boldsymbol{\xi }_{\ast }, \boldsymbol{\xi ,}I_{j},I_{i}\left \vert \boldsymbol{\xi }_{\ast }^{ \prime },\boldsymbol{\xi }^{\prime },I_{l},I_{k}\right . )\,d \boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime } \\ =&\left ( M_{i}M_{j\ast }\right ) ^{-1/2}\sum _{k,l=1}^{r} \int _{\left ( \mathbb{R}^{3}\right ) ^{2}}w(\boldsymbol{\xi }_{\ast }, \boldsymbol{\xi ,}I_{j},I_{i}\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{ \ast }^{\prime },I_{k},I_{l}\right . )\,d\boldsymbol{\xi }^{\prime }d \boldsymbol{\xi }_{\ast }^{\prime } \\ =&k_{ji1}(\boldsymbol{\xi }_{\ast },\boldsymbol{\xi }) \end{aligned}$$

(25)

and, by applying the second relation in (23) and renaming $\left \{ \boldsymbol{\xi }^{\prime },I_{k}\right \} \leftrightarrows \left \{ \boldsymbol{\xi }_{\ast }^{\prime },I_{l} \right \}$,

$$\begin{aligned} k_{ij2}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }) =&2\left ( M_{i}M_{j \ast }\right ) ^{-1/2}\sum _{k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{2}}w(\boldsymbol{\xi }_{\ast },\boldsymbol{\xi }_{ \ast }^{\prime },I_{j},I_{l}\left \vert \boldsymbol{\xi }, \boldsymbol{\xi }^{\prime },I_{i},I_{k}\right . )\,d\boldsymbol{\xi }^{\prime }d \boldsymbol{\xi }_{\ast }^{\prime } \\ =&2\left ( M_{i}M_{j\ast }\right ) ^{-1/2}\sum _{k,l=1}^{r} \int _{\left ( \mathbb{R}^{3}\right ) ^{2}}w(\boldsymbol{\xi }_{\ast }, \boldsymbol{\xi }^{\prime },I_{j},I_{k}\left \vert \boldsymbol{\xi },\boldsymbol{\xi }_{ \ast }^{\prime },I_{i},I_{l}\right . )\,d\boldsymbol{\xi }^{\prime }d \boldsymbol{\xi }_{\ast }^{\prime } \\ =&k_{ji2}(\boldsymbol{\xi }_{\ast },\boldsymbol{\xi })\text{.} \end{aligned}$$

(26)

We now continue by proving the compactness for the two different types of collision kernel separately.

I. Compactness of $K_{ij}^{\left ( 1\right ) }=\int _{ \mathbb{R}^{3}}k_{ij1}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\,h_{j\ast } \,d\boldsymbol{\xi }_{\ast }$ for $\left \{ i,j\right \} \subseteq \left \{ 1,\ldots,r\right \}$.

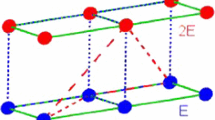
Assume the internal energy gap $\Delta I_{ij}^{kl}=I_{k}+I_{l}-I_{i}-I_{j}$ as well as the velocities $\boldsymbol{\xi }$ and $\boldsymbol{\xi }_{\ast }$, to be given. Then a collision will be uniquely defined by the unit vector $\boldsymbol{\omega }=\mathbf{g}^{\prime }/\left \vert \mathbf{g}^{ \prime }\right \vert$, with $\mathbf{g}^{\prime }=\boldsymbol{\xi }^{\prime }-\boldsymbol{\xi }_{ \ast }^{\prime }$. This follows, since, by conservation of momentum and total energy (2), $\left \vert \mathbf{g}^{\prime }\right \vert$ can be obtained, while also $\boldsymbol{\xi }-\boldsymbol{\xi }^{\prime }=\boldsymbol{\xi }_{ \ast}^{\prime }-\boldsymbol{\xi }_{\ast}$, cf. Fig. 1. Indeed, by a change of variables $\left \{ \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime }\right \} \rightarrow \left \{ \left \vert \mathbf{g}^{\prime }\right \vert ,\boldsymbol{\omega }= \dfrac{\mathbf{g}^{\prime }}{\left \vert \mathbf{g}^{\prime }\right \vert }, \mathbf{G}^{\prime }= \dfrac{\boldsymbol{\xi }^{\prime }+\boldsymbol{\xi }_{\ast }^{\prime }}{2}\right \}$, noting the invariance (9), and using relation (12), expression (24) of $k_{ij1}$ may be transformed to the following form

$$\begin{aligned} &k_{ij1}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }) \\ =&\sum _{k,l=1}^{r}\int _{\mathbb{R}^{3}\times \mathbb{R}_{+}\times \mathbb{S}^{2}}\left ( \frac{M_{k}^{\prime }M_{l\ast }^{\prime }}{\varphi _{i}\varphi _{j}\varphi _{k}\varphi _{l}}\right ) ^{1/2} \left \vert \mathbf{g}^{\prime }\right \vert ^{2}W(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{\prime }, \boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l}\right . )d\mathbf{G}^{\prime }d\left \vert \mathbf{g}^{\prime }\right \vert d\boldsymbol{\omega } \\ =&\left ( M_{i}M_{j\ast }\right ) ^{1/2}\left \vert \mathbf{g} \right \vert \sum _{k,l=1}^{r}\int _{\mathbb{S}^{2}}\sigma _{ij}^{kl} \left ( \left \vert \mathbf{g}\right \vert ,\cos \theta \right ) \mathbf{1}_{m\left \vert \mathbf{g}\right \vert >4\Delta I_{ij}^{kl}}\,d \boldsymbol{\omega }\text{.} \end{aligned}$$

By assumption (18) and the following relation for the exponent of the product $M_{i}M_{j\ast }$

$$ m\frac{\left \vert \boldsymbol{\xi }\right \vert ^{2}}{2}+m \frac{\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}}{2}+I_{i}+I_{j}=m \left \vert \mathbf{G}\right \vert ^{2}+m \frac{\left \vert \mathbf{g}\right \vert ^{2}}{4}+I_{i}+I_{j}=m\left \vert \mathbf{G}\right \vert ^{2}+E_{ij}\text{,} $$

the bound

$$\begin{aligned} &k_{ij1}^{2}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }) \\ \leq &\frac{C}{\left \vert \mathbf{g}\right \vert ^{2}}M_{i}M_{j \ast }\left ( \int _{\mathbb{S}^{2}}\,d\boldsymbol{\omega }\right ) ^{2} \left ( \sum _{k,l=1}^{r}\left ( \Psi _{ij}^{kl}+\left ( \Psi _{ij}^{kl}\right ) ^{\gamma /2}\right ) \mathbf{1}_{m\left \vert \mathbf{g}\right \vert >4\Delta I_{ij}^{kl}}\right ) ^{2} \\ \leq &\frac{C}{\left \vert \mathbf{g}\right \vert ^{2}}e^{-m\left \vert \mathbf{G}\right \vert ^{2}-E_{ij}}\left ( \sum _{k,l=1}^{r} \left ( 1+\left \vert \mathbf{g}\right \vert ^{2}\right ) \right ) ^{2}=C \frac{\left ( 1+\left \vert \mathbf{g}\right \vert ^{2}\right ) ^{2}}{\left \vert \mathbf{g}\right \vert ^{2}}e^{-m\left \vert \mathbf{G}\right \vert ^{2}-E_{ij}} \text{,} \end{aligned}$$

(27)

may be obtained. Then, by applying the bound (27) and first changing variables of integration $\left \{ \boldsymbol{\xi },\boldsymbol{\xi }_{\ast }\right \} \rightarrow \left \{ \mathbf{g}, \mathbf{G}\right \}$, with unitary Jacobian, and then to spherical coordinates,

$$\begin{aligned} \int _{\left ( \mathbb{R}^{3}\right ) ^{2}}k_{ij1}^{2}( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast })d\boldsymbol{\xi }d\boldsymbol{\xi }_{\ast } \leq &C\int _{\left ( \mathbb{R}^{3}\right ) ^{2}} \frac{\left ( 1+\left \vert \mathbf{g}\right \vert ^{4}\right ) }{\left \vert \mathbf{g}\right \vert ^{2}}e^{-m\left \vert \mathbf{G}\right \vert ^{2}-E_{ij}}d\mathbf{g}d \mathbf{G} \\ \leq &C\int _{0}^{\infty }R^{2}e^{-mR^{2}}dR\int _{0}^{\infty }e^{-m \eta ^{2}/4}\left ( 1+\eta ^{4}\right ) d\eta =C\text{.} \end{aligned}$$

Note that, here and below, we will, in general, not indicate an integration over a directional vector in $\mathbb{S}^{2}$ of the form

$$ \int _{\mathbb{S}^{2}}\,d\boldsymbol{\omega }=4\pi \text{,} $$

but just integrate it in the generic constant $C$.

Fig. 1

Typical collision of $K_{ij}^{(1)}$. Classical representation of an inelastic collision

Concluding,

$$ K_{ij}^{\left ( 1\right ) }=\int _{\mathbb{R}^{3}}k_{ij1}( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\,h_{j\ast }\,d\boldsymbol{\xi }_{\ast } $$

are Hilbert-Schmidt integral operators and as such compact on $L^{2}\left ( d\boldsymbol{\xi }\right )$, see e.g., Theorem 7.83 in [25], for all $\left \{ i,j\right \} \subseteq \left \{ 1,\ldots,r\right \}$.

II. Compactness of $K_{ij}^{\left ( 2\right ) }=\int _{ \mathbb{R}^{3}}k_{ij2}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\,h_{j\ast } \,d\boldsymbol{\xi }_{\ast }$ for $\left \{ i,j\right \} \subseteq \left \{ 1,\ldots,r\right \}$.

Assume the internal energy gap $\Delta I_{ik}^{jl}=I_{j}+I_{l}-I_{i}-I_{k}$, as well as the velocities $\boldsymbol{\xi }$ and $\boldsymbol{\xi }_{\ast }$, to be given. Then a collision will be uniquely defined by a vector $\mathbf{w}$ orthogonal to $\mathbf{g}=\boldsymbol{\xi }-\boldsymbol{\xi }\mathbf{_{\ast }}$. This follows, since, by conservation of momentum and total energy (2) (reminding that we relabeled the velocities and internal energies), the relation between $\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }^{\prime }\right \vert$ and $\left \vert \boldsymbol{\xi }_{\ast}^{\prime }-\boldsymbol{\xi }_{ \ast}\right \vert$ can be obtained, while also $\mathbf{g}^{\prime }=\boldsymbol{\xi }_{\ast }^{\prime }- \boldsymbol{\xi }^{\prime }=\mathbf{g}$, cf. Fig. 2. Indeed, noting that - with the aim to obtain expressions for $\mathbf{g}^{\prime }$ and $\chi =\left ( \boldsymbol{\xi }_{\ast }-\boldsymbol{\xi }^{\prime } \right ) \cdot \mathbf{g/}\left \vert \mathbf{g}\right \vert$ in the arguments of the delta-functions - cf. Fig. 2,

$$\begin{aligned} &W(\boldsymbol{\xi },\boldsymbol{\xi }^{\prime },I_{i},I_{k}\left \vert \boldsymbol{\xi }_{\ast },\boldsymbol{\xi }_{\ast }^{\prime },I_{j},I_{l} \right . ) \\ =&4m\varphi _{i}\varphi _{k}\sigma _{ik}^{jl} \frac{\left \vert \widetilde{\mathbf{g}}\right \vert }{\left \vert \mathbf{g}_{\ast }\right \vert } \delta _{3}\left ( \boldsymbol{\xi }^{\prime }-\boldsymbol{\xi }_{ \ast }^{\prime }+\boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }\right ) \delta _{1}\left ( \frac{m}{2}\left ( \left \vert \boldsymbol{\xi }\right \vert ^{2}-\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}+\left \vert \boldsymbol{\xi }^{\prime }\right \vert ^{2}-\left \vert \boldsymbol{\xi }_{\ast }^{ \prime }\right \vert ^{2}\right ) -\Delta I_{ik}^{jl}\right ) \\ =&4m\varphi _{i}\varphi _{k}\sigma _{ik}^{jl} \frac{\left \vert \widetilde{\mathbf{g}}\right \vert }{\left \vert \mathbf{g}_{\ast }\right \vert } \delta _{3}\left ( \mathbf{g}^{\prime }+\mathbf{g}\right ) \delta _{1} \left ( m\left ( \boldsymbol{\xi }_{\ast }-\boldsymbol{\xi }^{\prime } \right ) \cdot \mathbf{g}-\Delta I_{ik}^{jl}\right ) \\ =&4\varphi _{i}\varphi _{k}\sigma _{ik}^{jl} \frac{\left \vert \widetilde{\mathbf{g}}\right \vert }{\left \vert \mathbf{g}_{\ast }\right \vert \left \vert \mathbf{g}\right \vert } \delta _{3}\left ( \mathbf{g}^{\prime }+\mathbf{g}\right ) \delta _{1}\left ( \chi - \frac{\Delta I_{ik}^{jl}}{m\left \vert \mathbf{g}\right \vert } \right ) \text{, with }\chi =\left ( \boldsymbol{\xi }_{\ast }-\boldsymbol{\xi }^{\prime }\right ) \cdot \frac{\mathbf{g}}{\left \vert \mathbf{g}\right \vert }\text{,} \\ &\mathbf{g}=\boldsymbol{\xi }-\boldsymbol{\xi }\mathbf{_{\ast }} \text{, }\mathbf{g}^{\prime }=\boldsymbol{\xi }^{\prime }-\boldsymbol{\xi }_{ \ast }^{\prime }\text{, }\widetilde{\mathbf{g}}=\boldsymbol{\xi }- \boldsymbol{\xi }^{\prime }\text{, }\mathbf{g}_{\ast }=\boldsymbol{\xi }_{\ast }-\boldsymbol{\xi }_{\ast }^{\prime }\!\text{, }\Delta I_{ik}^{jl}=I_{j}+I_{l}-I_{i}-I_{k} \text{,} \end{aligned}$$

by the change of variables $\left \{ \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime }\right \} \rightarrow \left \{ \mathbf{g}^{ \prime }= \boldsymbol{\xi }^{\prime }-\boldsymbol{\xi }_{\ast }^{\prime }, \mathbf{h}= \boldsymbol{\xi }^{\prime }-\boldsymbol{\xi }_{\ast }\right \}$, where

$$ d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime }=d \mathbf{g}^{\prime }d\mathbf{h}=d\mathbf{g}^{\prime }d\chi d\mathbf{w} \text{, with }\mathbf{w}=\boldsymbol{\xi }^{\prime }-\boldsymbol{\xi }_{\ast }+ \chi \mathbf{n}\text{ and }\mathbf{n}= \frac{\mathbf{g}}{\left \vert \mathbf{g}\right \vert }\text{,} $$

the expression (24) of $k_{ij2}$ may be transformed to

$$\begin{aligned} &k_{ij2}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }) \\ =&\sum _{k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{2}}2 \left ( \frac{M_{k}^{\prime }M_{l\ast }^{\prime }}{\varphi _{i}\varphi _{j}\varphi _{k}\varphi _{l}} \right ) ^{1/2}W(\boldsymbol{\xi },\boldsymbol{\xi }^{\prime },I_{i},I_{k} \left \vert \boldsymbol{\xi }_{\ast },\boldsymbol{\xi }_{\ast }^{ \prime },I_{j},I_{l}\right . )\,d\mathbf{g}^{\prime }d\mathbf{h} \\ =&\frac{8\varphi _{i}^{1/2}}{\varphi _{j}^{1/2}}\sum _{k,l=1}^{r} \int _{\left ( \mathbb{R}^{3}\right ) ^{\perp _{\mathbf{n}}}} \frac{\varphi _{k}\left \vert \widetilde{\mathbf{g}}\right \vert }{\left \vert \mathbf{g}_{\ast }\right \vert \left \vert \mathbf{g}\right \vert }\mathbf{1}_{m\left \vert \widetilde{\mathbf{g}}\right \vert ^{2}>4\Delta I_{ik}^{jl}} \left ( \frac{M_{k}^{\prime }M_{l\ast }^{\prime }}{\varphi _{k}\varphi _{l}} \right ) ^{1/2}\sigma _{ik}^{jl}\left ( \left \vert \widetilde{\mathbf{g}}\right \vert , \frac{\widetilde{\mathbf{g}}\cdot \mathbf{g}_{\ast }}{\left \vert \widetilde{\mathbf{g}}\right \vert \left \vert \mathbf{g}_{\ast }\right \vert } \right ) d\mathbf{w}\text{,} \end{aligned}$$

(28)

where

$$ \left ( \mathbb{R}^{3}\right ) ^{\perp _{\mathbf{n}}}=\left \{ \mathbf{w}\in \mathbb{R}^{3}: \mathbf{w}\perp \mathbf{n}\right \}. $$

Here, for any $\left \{ k,l\right \} \subseteq \left \{ 1,\ldots,r\right \}$, see Fig. 2,

$$ \left \{ \textstyle\begin{array}{c} \boldsymbol{\xi }^{\prime }=\boldsymbol{\xi }_{\ast }+\mathbf{w}- \chi \mathbf{n} \\ \boldsymbol{\xi }_{\ast }^{\prime }=\boldsymbol{\xi }+\mathbf{w}- \chi \mathbf{n}\end{array}\displaystyle \right . \text{, with }\chi =\chi _{ik}^{jl}=\chi _{ik}^{jl}\left ( \left \vert \mathbf{g}\right \vert \right ) = \frac{\Delta I_{ik}^{jl}}{m\left \vert \mathbf{g}\right \vert }\text{,} $$

implying the following relation for an expression appearing in the exponent of the product $M_{k}^{\prime }M_{l\ast }^{\prime }$ in expression (28)

$$\begin{aligned} \frac{\left \vert \boldsymbol{\xi }^{\prime }\right \vert ^{2}}{2}+ \frac{\left \vert \boldsymbol{\xi }_{\ast }^{\prime }\right \vert ^{2}}{2} =& \left \vert \frac{\boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }}{2}-\chi _{ik}^{jl}\mathbf{n}+\mathbf{w}\right \vert ^{2}+ \frac{\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }\right \vert ^{2}}{4} \\ =&\left \vert \frac{\left ( \boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }\right ) _{\perp _{\boldsymbol{n}}}}{2}+\mathbf{w}\right \vert ^{2}+\left ( \frac{\left ( \boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }\right ) _{\mathbf{n}}}{2}-\chi _{ik}^{jl} \right ) ^{2}+\frac{\left \vert \mathbf{g}\right \vert ^{2}}{4} \\ =&\left \vert \frac{\left ( \boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }\right ) _{\perp _{\boldsymbol{n}}}}{2}+\mathbf{w}\right \vert ^{2}\!+ \frac{\left ( \left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}-\left \vert \boldsymbol{\xi }\right \vert ^{2}+2\chi _{ik}^{jl}\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }\right \vert \right ) ^{2}}{4\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }\right \vert ^{2}}+ \frac{\left \vert \mathbf{g}\right \vert ^{2}}{4}\text{,} \end{aligned}$$

where

$$ \left ( \boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }\right ) _{\mathbf{n}}=\left ( \boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }\right ) \cdot \mathbf{n}= \frac{\left \vert \boldsymbol{\xi }\right \vert ^{2}-\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}}{\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }\right \vert } \text{ and }\left ( \boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }\right ) _{\perp _{ \boldsymbol{n}}}=\boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }-\left ( \boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }\right ) _{\mathbf{n}}\mathbf{n}. $$

(29)

Hence, by assumption (18),

$$\begin{aligned} &k_{ij2}^{2}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }) \\ \leq &\frac{C}{\left \vert \mathbf{g}\right \vert ^{2}}\exp \left ( - \frac{m}{4}\left \vert \mathbf{g}\right \vert ^{2}\right ) \left ( \sum _{k,l=1}^{r}\frac{1}{e^{\left ( I_{k}+I_{l}\right ) /2}}\exp \left ( -m \frac{\left ( \left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}-\left \vert \boldsymbol{\xi }\right \vert ^{2}+2\chi _{ik}^{jl}\left \vert \mathbf{g}\right \vert \right ) ^{2}}{8\left \vert \mathbf{g}\right \vert ^{2}} \right ) \right . \\ &\times \left . \int _{\left ( \mathbb{R}^{3}\right ) ^{ \perp _{\mathbf{n}}}}\mathbf{1}_{m\left \vert \widetilde{\mathbf{g}}\right \vert ^{2}>4 \Delta I_{ik}^{jl}}\left ( 1+ \frac{1}{\left ( \widetilde{\Psi }_{ik}^{jl}\right ) ^{1-\gamma /2}}\right ) \exp \left ( -\frac{m}{2}\left \vert \frac{\left ( \boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }\right ) _{\perp _{\boldsymbol{n}}}}{2}+ \mathbf{w}\right \vert ^{2}\right ) d\mathbf{w}\right ) ^{2} \\ \leq &\frac{C}{\left \vert \mathbf{g}\right \vert ^{2}}\exp \left ( - \frac{m}{4}\left \vert \mathbf{g}\right \vert ^{2}\right ) \left ( \sum _{k,l=1}^{r}\exp \left ( -m \frac{\left ( \left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}-\left \vert \boldsymbol{\xi }\right \vert ^{2}+2\chi _{ik}^{jl}\left \vert \mathbf{g}\right \vert \right ) ^{2}}{8\left \vert \mathbf{g}\right \vert ^{2}}\right ) \right ) ^{2} \\ =&\frac{C}{\left \vert \mathbf{g}\right \vert ^{2}}\left ( \sum _{k,l=1}^{r}\exp \left ( -\frac{m}{8}\left ( \left \vert \mathbf{g}\right \vert +2\left \vert \boldsymbol{\xi }\right \vert \cos \varphi +2\chi _{ik}^{jl}\right ) ^{2}-\frac{m}{8}\left \vert \mathbf{g}\right \vert ^{2}\right ) \right ) ^{2} \\ \leq &\frac{C}{\left \vert \mathbf{g}\right \vert ^{2}}\sum _{k,l=1}^{r}\exp \left ( -m\left ( \dfrac{\left \vert \mathbf{g}\right \vert }{2}+\left \vert \boldsymbol{\xi }\right \vert \cos \varphi +\chi _{ik}^{jl}\right ) ^{2}-\frac{m}{4}\left \vert \mathbf{g}\right \vert ^{2}\right ) \text{,} \end{aligned}$$

(30)

with $\cos \varphi =\mathbf{n}\cdot \dfrac{\boldsymbol{\xi }}{\left \vert \boldsymbol{\xi }\right \vert }$, $\widetilde{\Psi }_{ik}^{jl}=\left \vert \widetilde{\mathbf{g}} \right \vert \left \vert \mathbf{g}_{\ast }\right \vert$, and $\left \vert \mathbf{g}_{\ast }\right \vert ^{2}=\left \vert \widetilde{\mathbf{g}}\right \vert ^{2}-\dfrac{4}{m}\Delta I_{ik}^{jl}$.

Fig. 2

Typical collision of $K_{ij}^{(2)}$

Here, the second inequality, follows by the following bound, which can be obtained by noting that here $\min \left ( \left \vert \widetilde{\mathbf{g}}\right \vert , \left \vert \mathbf{g}_{\ast }\right \vert \right ) \geq \left \vert \mathbf{w}\right \vert$, cf. Fig. 2, while firstly making a change of variables $\mathbf{w} \rightarrow \widetilde{\mathbf{w}}=\left ( \boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }\right ) _{\perp _{\boldsymbol{n}}}/2+ \mathbf{w}$ followed by one to polar coordinates,

$$\begin{aligned} &\int _{\left ( \mathbb{R}^{3}\right ) ^{\perp _{\mathbf{n}}}} \mathbf{1}_{m\left \vert \widetilde{\mathbf{g}}\right \vert ^{2}>4\Delta I_{ik}^{jl}} \left ( 1+ \frac{1}{\left ( \widetilde{\Psi }_{ik}^{jl}\right ) ^{1-\gamma /2}}\right ) \exp \left ( -\frac{m}{2}\left \vert \frac{\left ( \boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }\right ) _{\perp _{\boldsymbol{n}}}}{2}+ \mathbf{w}\right \vert ^{2}\right ) \,d\mathbf{w} \\ \leq &\int _{\left \vert \mathbf{w}\right \vert \leq 1}1+\left \vert \mathbf{w}\right \vert ^{\gamma -2}\,d\mathbf{w}+2\int _{\left \vert \mathbf{w}\right \vert \geq 1}\exp \left ( -\frac{m}{2}\left \vert \frac{\left ( \boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }\right ) _{\perp _{\boldsymbol{n}}}}{2}+ \mathbf{w}\right \vert ^{2}\right ) \,d\mathbf{w} \\ \leq &\int _{\left \vert \mathbf{w}\right \vert \leq 1}1+\left \vert \mathbf{w}\right \vert ^{\gamma -2}\,d\mathbf{w}+2\int _{\left ( \mathbb{R}^{3} \right ) ^{\perp _{\mathbf{n}}}}e^{-m\left \vert \widetilde{\mathbf{w}}\right \vert ^{2}/2}\,d\widetilde{\mathbf{w}} \\ =&2\pi \left ( \int _{0}^{1}R+R^{\gamma -1}\,dR+\int _{0}^{\infty }Re^{-mR^{2}/2} \,dR\right ) =C\text{.} \end{aligned}$$

The integral of $k_{ij2}^{2}$ over the truncated domain $\mathfrak{h}_{N}$ will be bounded, since, by changing variables $\boldsymbol{\xi }\mathbf{_{\ast }}\rightarrow \mathbf{g}=\boldsymbol{\xi }-\boldsymbol{\xi } \mathbf{_{\ast }}$, and then to spherical coordinates,

$$\begin{aligned} \int _{\mathfrak{h}_{N}}k_{ij2}^{2}(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast })\,d\boldsymbol{\xi }d\boldsymbol{\xi }_{ \ast } \leq &\int _{\mathfrak{h}_{N}}\frac{C}{\left \vert \mathbf{g}\right \vert ^{2}}e^{-m\left \vert \mathbf{g}\right \vert ^{2}/4}d\mathbf{g\,}d\boldsymbol{\xi } \\ \leq &C\int _{0}^{\infty }e^{-R^{2}/4}dR\int _{0}^{N}\eta ^{2}d\eta =CN^{3}. \end{aligned}$$

Next we aim for proving that the integral of $k_{ij2}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })$ with respect to $\boldsymbol{\xi }$ over $\mathbb{R}^{3}$ is bounded in $\boldsymbol{\xi }_{\ast }$. Indeed, directly by the bound (30) on $k_{ij2}^{2}$

$$ 0\leq k_{ij2}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\leq \frac{C}{\left \vert \mathbf{g}\right \vert }\sum _{k,l=1}^{r}\exp \left ( -\frac{m}{8}\left ( \left \vert \mathbf{g}\right \vert +2\left \vert \boldsymbol{\xi }\right \vert \cos \varphi +2\chi _{ik}^{jl}\right ) ^{2}-\frac{m}{8} \left \vert \mathbf{g}\right \vert ^{2}\right ) \text{.} $$

(31)

Hence, by applying the symmetry $k_{ij2}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })=k_{ji2}(\boldsymbol{\xi }_{\ast },\boldsymbol{\xi })$ (26), firstly changing variables $\boldsymbol{\xi }\rightarrow \mathbf{g}=\boldsymbol{\xi }-\boldsymbol{\xi }\mathbf{_{\ast }}$, and then to spherical coordinates,

$$\begin{aligned} &\int _{\mathbb{R}^{3}}k_{ij2}(\boldsymbol{\xi },\boldsymbol{\xi }_{ \ast })\,d\boldsymbol{\xi }=\int _{\mathbb{R}^{3}}k_{ji2}( \boldsymbol{\xi }_{\ast },\boldsymbol{\xi })\,d\boldsymbol{\xi } \\ \leq &\int _{\mathbb{R}^{3}} \frac{C}{\left \vert \mathbf{g}\right \vert }\sum _{k,l=1}^{r}\exp \left ( -\frac{m}{8}\left \vert \mathbf{g}\right \vert ^{2}\right ) \,d\mathbf{g}=C\int _{0}^{\infty }Re^{-mR^{2}/8}dR=C\text{.} \end{aligned}$$

Finally, heading for proving the uniform convergence of the integral of $k_{ij2}$ with respect to $\boldsymbol{\xi }_{\ast }$ over the truncated domain $\mathfrak{h}_{N}$ to the one over all of $\mathbb{R}^{3}$, the following bound on the integral over $\mathbb{R}^{3}$ can be obtained for $\left \vert \boldsymbol{\xi }\right \vert \neq 0$ by bound (31), by changing to (conventional) spherical coordinates, with $\boldsymbol{\xi }$ as zenithal direction, and hence, $\varphi$ as polar angle, followed by the change of variables $\varphi \rightarrow \eta =R+2\left \vert \boldsymbol{\xi }\right \vert \cos \varphi +2\chi _{ik}^{jl}\left ( R\right )$, with $d\eta =-2\left \vert \boldsymbol{\xi }\right \vert \sin \varphi \,d\varphi$,

$$\begin{aligned} &\int _{\mathbb{R}^{3}}k_{ij2}(\boldsymbol{\xi },\boldsymbol{\xi }_{ \ast })\,d\boldsymbol{\xi }_{\ast } \\ \leq &\int _{\mathbb{R}^{3}} \frac{C}{\left \vert \mathbf{g}\right \vert }\sum _{k,l=1}^{r}\exp \left ( -\frac{m}{8}\left ( \left \vert \mathbf{g}\right \vert +2\left \vert \boldsymbol{\xi }\right \vert \cos \varphi +2\chi _{ik}^{jl}\left ( \left \vert \mathbf{g}\right \vert \right ) \right ) ^{2}-\frac{m}{8}\left \vert \mathbf{g}\right \vert ^{2}\right ) d\mathbf{g} \\ =&C\sum _{k,l=1}^{r}\int _{0}^{\infty }\int _{0}^{\pi }R \exp \left ( -\frac{m}{8}\left ( R+2\left \vert \boldsymbol{\xi }\right \vert \cos \varphi +2\chi _{ik}^{jl}\left ( R\right ) \right ) ^{2}-\frac{m}{8}R^{2} \right ) \sin \varphi \,d\varphi dR \\ =&\frac{C}{\left \vert \boldsymbol{\xi }\right \vert }\sum _{k,l=1}^{r}\int _{0}^{\infty }\int _{R+2\chi _{ik}^{jl}\left ( R\right ) -2 \left \vert \boldsymbol{\xi }\right \vert }^{R+2\chi _{ik}^{jl}\left ( R\right ) +2\left \vert \boldsymbol{\xi }\right \vert }Re^{-m\eta ^{2}/8}e^{-mR^{2}/8}d \eta dR \\ \leq &\frac{C}{\left \vert \boldsymbol{\xi }\right \vert }\int _{0}^{ \infty }Re^{-mR^{2}/8}\,dR\int _{-\infty }^{\infty }e^{-m\eta ^{2}/8}d \eta =\frac{C}{\left \vert \boldsymbol{\xi }\right \vert }\text{.} \end{aligned}$$

(32)

Then, by the bounds (31) and (32),

$$\begin{aligned} &\sup _{\boldsymbol{\xi }\in \mathbb{R}^{3}}\int _{\mathbb{R}^{3}}k_{ij2}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })-k_{ij2}( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\mathbf{1}_{\mathfrak{h}_{N}}\,d \boldsymbol{\xi }_{\ast } \\ \leq &\sup _{\boldsymbol{\xi }\in \mathbb{R}^{3}}\int _{\left \vert \mathbf{g}\right \vert \leq \frac{1}{N}}k_{ij2}(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast })\,d\boldsymbol{\xi }_{\ast }+\sup _{\left \vert \boldsymbol{\xi }\right \vert \geq N}\int _{\mathbb{R}^{3}}k_{ij2}(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\,d\boldsymbol{\xi }_{\ast } \\ \leq &\int _{\left \vert \mathbf{g}\right \vert \leq \frac{1}{N}} \frac{C}{\left \vert \mathbf{g}\right \vert }\,d\mathbf{g}+\frac{C}{N}\leq C \left ( \int _{0}^{\frac{1}{N}}R\,dR+\frac{1}{N}\right ) =C\left ( \frac{1}{2N^{2}}+\frac{1}{N}\right ) \rightarrow 0\text{ as }N\rightarrow \infty \text{.} \end{aligned}$$

Hence, by Lemma 4, the operators

$$ K_{ij}^{(2)}=\int _{\mathbb{R}^{3}}k_{ij2}(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast })\,h_{j\ast }\,d\boldsymbol{\xi }_{\ast } $$

are compact on $L^{2}\left ( d\boldsymbol{\xi }\right )$ as uniform limits of Hilbert-Schmidt integral operators for all $\left \{ i,j\right \} \subseteq \left \{ 1,\ldots,r\right \}$.

Concluding, the operator

$$ K=(K_{1},\ldots,K_{r})=\sum _{j=1}^{r}\left ( (K_{1j}^{(2)},\ldots,K_{rj}^{(2)})-(K_{1j}^{(1)},\ldots,K_{rj}^{(1)})\right ) $$

is a compact self-adjoint operator on $\left ( L^{2}\left ( d\boldsymbol{\xi }\right ) \right ) ^{r}$. The self-adjointness is due to the symmetry relations (25), (26), cf. [27, p. 198]. □

2.3.2 Bounds on the Collision Frequency

This section concerns the proof of Theorem 2 and is an extension of that for monatomic single species.

Proof

Under assumption (19) each collision frequency $\nu _{1},\ldots,\nu _{r}$, by a change of variables $\left \{ \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime }\right \} \rightarrow \left \{ \left \vert \mathbf{g}^{\prime }\right \vert ,\boldsymbol{\omega }= \dfrac{\mathbf{g}^{\prime }}{\left \vert \mathbf{g}^{\prime }\right \vert }, \mathbf{G}^{\prime }= \dfrac{\boldsymbol{\xi }^{\prime }+\boldsymbol{\xi }_{\ast }^{\prime }}{2}\right \}$, can be rewritten as

$$\begin{aligned} \nu _{i} =&\sum _{j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3} \right ) ^{3}}\frac{M_{j\ast }}{\varphi _{i}\varphi _{j}}W( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast },I_{i},I_{j}\left \vert \boldsymbol{\xi }^{ \prime },\boldsymbol{\xi }_{\ast }^{\prime },I_{k},I_{l}\right . )\,d \boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{ \prime } \\ =&\sum _{j,k,l=1}^{r}\int _{\left ( \mathbb{R}^{3}\right ) ^{2} \times \mathbb{R}_{+}\times \mathbb{S}^{2}}M_{j\ast }\sigma _{ij}^{kl} \left \vert \mathbf{g}\right \vert \delta _{3}\left ( \mathbf{G}-\mathbf{G}^{\prime }\right ) \mathbf{1}_{m\left \vert \mathbf{g}\right \vert >4 \Delta I_{ij}^{kl}} \\ &\times \delta _{1}\left ( \sqrt{\left \vert \mathbf{g}\right \vert ^{2}- \frac{4}{m}\Delta I_{ij}^{kl}}-\left \vert \mathbf{g}^{\prime }\right \vert \right ) \,d\boldsymbol{\xi }_{\ast }d\mathbf{G}^{\prime }d \left \vert \mathbf{g}^{\prime }\right \vert d\boldsymbol{\omega } \\ =&\frac{C}{\varphi _{i}}\sum _{j,k,l=1}^{r}e^{-I_{j}}\int _{ \mathbb{R}^{3}}e^{-m\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}/2} \sigma _{ij}^{kl}\left \vert \mathbf{g}\right \vert \mathbf{1}_{m \left \vert \mathbf{g}\right \vert >4\Delta I_{ij}^{kl}}\,d\boldsymbol{\xi }_{\ast } \\ =&\frac{C}{\varphi _{i}}\sum _{j,k,l=1}^{r}e^{-I_{j}}\int _{ \mathbb{R}^{3}}e^{-m\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}/2} \sqrt{\left \vert \mathbf{g}\right \vert ^{2}-\frac{4}{m}\Delta I_{ij}^{kl}} \mathbf{1}_{m\left \vert \mathbf{g}\right \vert >4\Delta I_{ij}^{kl}}\,d \boldsymbol{\xi }_{\ast }\text{.} \end{aligned}$$

Given $i\in \left \{ 1,\ldots,r\right \}$, there are $\left \{ j,k,l\right \} \subset \left \{ 1,\ldots,r\right \}$, such that $\Delta I_{ij}^{kl}\leq 0$. Assuming that $\Delta I_{ij}^{kl}\leq 0$ for some fixed $\left \{ j,k,l\right \} \subset \left \{ 1,\ldots,r\right \}$, imply the inequality

$$\begin{aligned} \nu _{i} \geq &\frac{Ce^{-I_{j}}}{\varphi _{i}}\int _{ \mathbb{R}^{3}}e^{-m\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}/2} \sqrt{\left \vert \mathbf{g}\right \vert ^{2}-\frac{4}{m}\Delta I_{ij}^{kl}} \,d\boldsymbol{\xi }_{\ast } \\ \geq &C\int _{\mathbb{R}^{3}}\left \vert \mathbf{g}\right \vert e^{-m \left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}/2}\,d \boldsymbol{\xi }_{\ast }\text{.} \end{aligned}$$

First, aiming to prove the lower bound of Theorem 2, consider the two different cases $\left \vert \boldsymbol{\xi }\right \vert \leq 1$ and $\left \vert \boldsymbol{\xi }\right \vert \geq 1$ separately. If $\left \vert \boldsymbol{\xi }\right \vert \leq 1$, then, by trivial estimates and a change to spherical coordinates,

$$\begin{aligned} \nu _{i} \geq &C\int _{\mathbb{R}^{3}}\left ( \left \vert \left \vert \boldsymbol{\xi }\right \vert -\left \vert \boldsymbol{\xi }_{\ast }\right \vert \right \vert \right ) e^{-m \left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}/2}\,d \boldsymbol{\xi }_{\ast } \\ \geq &C\int _{\left \vert \boldsymbol{\xi }_{\ast }\right \vert \geq 2}\left ( \left \vert \boldsymbol{\xi }_{\ast }\right \vert - \left \vert \boldsymbol{\xi }\right \vert \right ) e^{-m\left \vert \boldsymbol{\xi }_{\ast } \right \vert ^{2}/2}\,d\boldsymbol{\xi }_{\ast } \\ \geq &C\int _{\left \vert \boldsymbol{\xi }_{\ast }\right \vert \geq 2}\frac{1}{2}\left \vert \boldsymbol{\xi }_{\ast }\right \vert e^{-m\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}/2}\,d \boldsymbol{\xi }_{\ast } \\ =&C\int _{1}^{\infty }R^{3}e^{-2mR^{2}}\,dR=C\geq C\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right ) \text{,} \end{aligned}$$

while, if $\left \vert \boldsymbol{\xi }\right \vert \geq 1$, then, by a trivial estimate,

$$\begin{aligned} \nu _{i} \geq &C\int _{\mathbb{R}^{3}}\left ( \left \vert \left \vert \boldsymbol{\xi }\right \vert -\left \vert \boldsymbol{\xi }_{\ast }\right \vert \right \vert \right ) e^{-m \left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}/2}\,d \boldsymbol{\xi }_{\ast } \\ \geq &C\int _{\left \vert \boldsymbol{\xi }_{\ast }\right \vert \leq 1/2}\left ( \left \vert \boldsymbol{\xi }\right \vert -\left \vert \boldsymbol{\xi }_{\ast }\right \vert \right ) e^{-m\left \vert \boldsymbol{\xi }_{ \ast }\right \vert ^{2}/2}\,d\boldsymbol{\xi }_{\ast } \\ \geq &C\frac{1}{2}\left \vert \boldsymbol{\xi }\right \vert e^{-m/8} \int _{\left \vert \boldsymbol{\xi }_{\ast }\right \vert \leq 1/2}\,d\boldsymbol{\xi }_{\ast }=C\left \vert \boldsymbol{\xi }\right \vert \geq C\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right ) \text{.} \end{aligned}$$

Hence, there is a positive constant $\nu _{-}>0$, such that $\nu _{i}\geq \nu _{-}\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right )$ for all $i\in \left \{ 1,\ldots,r\right \}$ and $\boldsymbol{\xi }\in \mathbb{R}^{3}$.

On the other hand, regarding the upper bound, by an estimate and a change to spherical coordinates,

$$\begin{aligned} \nu _{i} \leq &C\sum _{j,k,l=1}^{r}\int _{\mathbb{R}^{3}} \sqrt{\left \vert \mathbf{g}\right \vert ^{2}-\frac{4}{m}\Delta I_{ij}^{kl}}\,e^{-m\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}/2} \mathbf{1}_{m\left \vert \mathbf{g}\right \vert >4\Delta I_{ij}^{kl}}\,d \boldsymbol{\xi }_{\ast } \\ \leq &C\sum _{j,k,l=1}^{r}\int _{\mathbb{R}^{3}}\left ( 1+ \left \vert \boldsymbol{\xi }\right \vert +\left \vert \boldsymbol{\xi }_{\ast }\right \vert \right ) e^{-m\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}/2}\,d\boldsymbol{\xi }_{ \ast } \\ \leq &C\int _{\mathbb{R}^{3}}\left \vert \boldsymbol{\xi }\right \vert R^{2}e^{-2mR^{2}}+\left ( R^{2}+R^{3}\right ) e^{-2mR^{2}}\,dR \leq C\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right ) \text{.} \end{aligned}$$

Hence, there is a positive constant $\nu _{+}>0$, such that $\nu _{i}\leq \nu _{+}\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right )$ for all $i\in \left \{ 1,\ldots,r\right \}$ and $\boldsymbol{\xi }\in \mathbb{R}^{3}$. □

3 Multicomponent Mixtures of Monatomic Species

This section concerns mixtures of $s$ monatomic species for any positive integer $s$ with (possibly) disparate masses, cf. e.g. [10, 12]. The case $s=1$, formally corresponds to a single species, and no mixture, but can still be included.

3.1 Model

This section concerns the considered model for multicomponent mixtures. A probabilistic formulation of the collision operator is considered, whose relation to a more classical formulation is accounted for. Known properties of the model and a corresponding linearized collision operator are also reviewed.

Consider a mixture of $s$, $s\geq 1$, monatomic species $a_{1},\ldots,a_{s}$, with masses $m_{1},\ldots,m_{s}$, respectively ($s=1$ corresponds to the case of a single species). The distribution functions are of the form $f=\left ( f_{1},\ldots,f_{s}\right )$, where $f_{\alpha }=f_{\alpha }\left ( t,\mathbf{x},\boldsymbol{\xi }\right )$, with $t\in \mathbb{R}_{+}$, $\mathbf{x}=\left ( x,y,z\right ) \in \mathbb{R}^{3}$, and $\boldsymbol{\xi }=\left ( \xi _{x},\xi _{y},\xi _{z}\right ) \in \mathbb{R}^{3}$, is the distribution function for species $a_{\alpha }$.

Moreover, consider the real Hilbert space $\mathcal{\mathfrak{h}}^{(s)}:=\left ( L^{2}\left ( d\boldsymbol{\xi }\right ) \right ) ^{s}$, with inner product

$$ \left ( \left . f\right \vert g\right ) =\sum _{\alpha =1}^{s}\int _{ \mathbb{R}^{3}}f_{\alpha }g_{\alpha }\,d\boldsymbol{\xi }\text{ for }f,g\in \left ( L^{2}\left ( d\boldsymbol{\xi }\right ) \right ) ^{s}\text{.} $$

The evolution of the distribution functions is (in the absence of external forces) described by the (vector) Boltzmann equation (1), where the (vector) collision operator $Q=\left ( Q_{1},\ldots,Q_{s}\right )$ is a quadratic bilinear operator that accounts for the change of velocities of particles due to binary collisions (assuming that the gas is rarefied, such that other collisions are negligible), where the component $Q_{\alpha }$ is the collision operator for species $a_{\alpha }$.

A collision can, given two species $a_{\alpha }$ and $a_{\beta }$, $\left \{ \alpha ,\beta \right \} \subset \left \{ 1,\ldots,s\right \}$, be represented by two pairs of microscopic velocities, one pair of pre-collisional velocities $\boldsymbol{\xi }$ and $\boldsymbol{\xi }_{\ast }$ of particles of species $a_{\alpha }$ and $a_{\beta }$, respectively, and one pair of post-collisional velocities $\boldsymbol{\xi }^{\prime }$ and $\boldsymbol{\xi }_{\ast }^{\prime }$ of particles of species $a_{\alpha }$ and $a_{\beta }$, respectively. The notation for pre- and post-collisional pairs may, of course, be interchanged as well. Due to momentum and total energy conservation, the following relations have to be satisfied by the pairs

$$\begin{aligned} m_{\alpha }\boldsymbol{\xi }+m_{\beta }\boldsymbol{\xi }_{\ast } =&m_{ \alpha }\boldsymbol{\xi }^{\prime }+m_{\beta }\boldsymbol{\xi }_{ \ast }^{\prime } \\ m_{\alpha }\left \vert \boldsymbol{\xi }\right \vert ^{2}+m_{\beta } \left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2} =&m_{\alpha } \left \vert \boldsymbol{\xi }^{\prime }\right \vert ^{2}+m_{\beta } \left \vert \boldsymbol{\xi }_{\ast }^{\prime }\right \vert ^{2}\text{.} \end{aligned}$$

(33)

3.1.1 Collision Operator

The (vector) collision operator $Q=\left ( Q_{1},\ldots,Q_{s}\right )$ has components that can be written in the following form - reminding the abbreviations (4),

$$ Q_{\alpha }(f,f)=\sum _{\beta =1}^{s}\int _{\left ( \mathbb{R}^{3}\right ) ^{3}}W_{\alpha \beta }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime }, \boldsymbol{\xi }_{\ast }^{\prime }\right . )\,\left ( f_{\alpha }^{ \prime }f_{\beta \ast }^{\prime }-f_{\alpha }f_{\beta \ast }\right ) \,d\boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime }\text{.} $$

The transition probability $W:\left ( \left ( \mathbb{R}^{3}\right ) ^{2}\times \left \{ a_{1},\ldots,a_{s} \right \} \right ) ^{2}\rightarrow \mathbb{R}_{+}:=[0,\infty )$ is of the form cf. [15, p. 65]

$$\begin{aligned} &W(\boldsymbol{\xi },\boldsymbol{\xi }^{\prime },a_{\alpha }\left \vert \boldsymbol{\xi }_{\ast },\boldsymbol{\xi }_{\ast }^{\prime },a_{ \beta }\right . )=:W_{\alpha \beta }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime }, \boldsymbol{\xi }_{\ast }^{\prime }\right . ) \\ =&2\left ( m_{\alpha }+m_{\beta }\right ) ^{2}m_{\alpha }m_{\beta } \sigma _{\alpha \beta }\left ( \left \vert \mathbf{g}\right \vert , \cos \theta \right ) \delta _{3}\left ( m_{\alpha }\boldsymbol{\xi }+m_{ \beta }\boldsymbol{\xi }_{\ast }-m_{\alpha }\boldsymbol{\xi }^{\prime }-m_{ \beta }\boldsymbol{\xi }_{\ast }^{\prime }\right ) \\ &\times \delta _{1}\left ( m_{\alpha }\left \vert \boldsymbol{\xi }\right \vert ^{2}+m_{\beta }\left \vert \boldsymbol{\xi }_{\ast } \right \vert ^{2}-m_{\alpha }\left \vert \boldsymbol{\xi }^{\prime } \right \vert ^{2}-m_{\beta }\left \vert \boldsymbol{\xi }_{\ast }^{ \prime }\right \vert ^{2}\right ) \text{, with }\cos \theta = \frac{\mathbf{g}\cdot \mathbf{g}^{\prime }}{\left \vert \mathbf{g}\right \vert \left \vert \mathbf{g}^{\prime }\right \vert }\text{, } \\ &\mathbf{g}=\boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }\text{, } \mathbf{g}^{\prime }=\boldsymbol{\xi }^{\prime }-\boldsymbol{\xi }_{ \ast }^{\prime } \text{, and } \sigma _{\alpha \beta }=\sigma _{ \alpha \beta }\left ( \left \vert \mathbf{g}\right \vert ,\cos \theta \right ) >0\text{ a.e. ,} \end{aligned}$$

(34)

where we remind that $\delta _{3}$ and $\delta _{1}$ denote the Dirac’s delta functions in $\mathbb{R}^{3}$ and ℝ, respectively; taking the conservation of momentum and kinetic energy (33) into account. Here and below we use the shorthanded expressions

$$ \sigma _{\alpha \beta }=\sigma (\boldsymbol{\xi },a_{\alpha }, \boldsymbol{\xi }_{\ast },a_{\beta })=\widetilde{\sigma }(\left \vert \mathbf{g}\right \vert ,\cos \theta ,a_{\alpha },a_{\beta }) $$

for a given scattering cross-section $\sigma :\left ( \mathbb{R}^{3}\times \left \{ a_{1},\ldots,a_{s}\right \} \right ) ^{2}\rightarrow \mathbb{R}_{+}$, or in the alternative form $\widetilde{\sigma }:\mathbb{R}_{+}\times \left [ -1,1\right ] \times \left \{ a_{1},\ldots,a_{s}\right \} ^{2}\rightarrow \mathbb{R}_{+}$.

The scattering cross sections $\sigma _{\alpha \beta }$, $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots,s\right \}$, satisfy the symmetry relation

$$ \sigma _{\alpha \beta }=\sigma _{\beta \alpha }\text{,} $$

(35)

while also for any $\alpha \in \left \{ 1,\ldots,s\right \}$

$$ \text{ }\sigma _{\alpha \alpha }\left ( \left \vert \mathbf{g}\right \vert ,-\cos \theta \right ) =\sigma _{\alpha \alpha }\left ( \left \vert \mathbf{g}\right \vert ,\cos \theta \right ) \text{.} $$

(36)

Applying known properties of Dirac’s delta function, the transition probabilities may, with the aim to obtain expressions for $\mathbf{G}_{\alpha \beta }^{\prime } =(m_{\alpha }\boldsymbol{\xi }^{\prime }+m_{\beta }\boldsymbol{\xi }_{\ast }^{\prime })/(m_{\alpha }+m_{\beta })$ and $\left \vert \mathbf{g}^{ \prime }\right \vert$ in the arguments of the Dirac’s delta functions, be transformed to

$$\begin{aligned} &W_{\alpha \beta }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime } \right . ) \\ =&2\left ( m_{\alpha }+m_{\beta }\right ) ^{3}\mu _{\alpha \beta } \delta _{3}\left ( \left ( m_{\alpha }+m_{\beta }\right ) \left ( \mathbf{G}_{\alpha \beta }-\mathbf{G}_{\alpha \beta }^{\prime } \right ) \right ) \delta _{1}\left ( \mu _{\alpha \beta }\left ( \left \vert \mathbf{g}\right \vert ^{2}-\left \vert \mathbf{g}^{ \prime }\right \vert ^{2}\right ) \right ) \\ =& \frac{\sigma _{\alpha \beta }}{\left \vert \mathbf{g}\right \vert } \delta _{3}\left ( \mathbf{G}_{\alpha \beta }-\mathbf{G}_{\alpha \beta }^{\prime }\right ) \delta _{1}\left ( \left \vert \mathbf{g} \right \vert -\left \vert \mathbf{g}^{\prime }\right \vert \right ) \text{, where }\mu _{\alpha \beta }=\frac{m_{\alpha }m_{\beta }}{m_{\alpha }+m_{\beta }}\text{, } \\ &\mathbf{G}_{\alpha \beta }= \frac{m_{\alpha }\boldsymbol{\xi }+m_{\beta }\boldsymbol{\xi }_{\ast }}{m_{\alpha }+m_{\beta }}\text{, and } \mathbf{G}_{\alpha \beta }^{\prime }= \frac{m_{\alpha }\boldsymbol{\xi }^{\prime }+m_{\beta }\boldsymbol{\xi }_{\ast }^{\prime }}{m_{\alpha }+m_{\beta }}\text{.} \end{aligned}$$

(37)

Due to invariance under change of particles in a collision and microreversibility of the collisions, which follows directly by the definition of the transition probability (34), (37), and the symmetry relations (35) and (36) for the collision frequency, the transition probabilities (34) satisfy the relations

$$\begin{aligned} W_{\alpha \beta }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime } \right . ) =&W_{\beta \alpha }(\boldsymbol{\xi }_{\ast }, \boldsymbol{\xi }\left \vert \boldsymbol{\xi }_{\ast }^{\prime }, \boldsymbol{\xi }^{\prime }\right . ) \\ W_{\alpha \beta }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime } \right . ) =&W_{\alpha \beta }(\boldsymbol{\xi }^{\prime }, \boldsymbol{\xi }_{\ast }^{\prime }\left \vert \boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }\right . ) \\ W_{\alpha \alpha }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime } \right . ) =&W_{\alpha \alpha }(\boldsymbol{\xi },\boldsymbol{\xi }_{ \ast }\left \vert \boldsymbol{\xi }_{\ast }^{\prime }, \boldsymbol{\xi }^{\prime }\right . )\text{.} \end{aligned}$$

(38)

By changing variables $\left \{ \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime }\right \} \rightarrow \left \{ \mathbf{g}^{\prime }=\boldsymbol{\xi }^{\prime }-\boldsymbol{\xi }_{\ast }^{\prime }, \mathbf{G}_{\alpha \beta }^{\prime }= \dfrac{m_{\alpha }\boldsymbol{\xi }^{\prime }+m_{\beta }\boldsymbol{\xi }_{\ast }^{\prime }}{m_{\alpha }+m_{\beta }}\right \}$, followed by a change to spherical coordinates, noting that

$$ d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime }=d \mathbf{G}_{\alpha \beta }^{\prime }d\mathbf{g}^{\prime }=\left \vert \mathbf{g}^{\prime }\right \vert ^{2}d\mathbf{G}_{\alpha \beta }^{\prime }d \left \vert \mathbf{g}^{\prime }\right \vert d\boldsymbol{\omega } \text{, with }\boldsymbol{\omega }= \frac{\mathbf{g}^{\prime }}{\left \vert \mathbf{g}^{\prime }\right \vert }\text{,} $$

(39)

the observation that

$$\begin{aligned} &Q_{\alpha }(f,f) \\ =&\sum _{\beta =1}^{s}\int _{\left ( \mathbb{R}^{3}\right ) ^{2} \times \mathbb{R}_{+}\times \mathbb{S}^{2}}\!\!W_{\alpha \beta }( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime }, \boldsymbol{\xi }_{\ast }^{\prime }\right . )\left ( f_{\alpha }^{\prime }f_{ \beta \ast }^{\prime }-f_{\alpha }f_{\beta \ast }\right ) \left \vert \mathbf{g}^{\prime }\right \vert ^{2}d\boldsymbol{\xi }_{\ast }d \mathbf{G}_{\alpha \beta }^{\prime }d\left \vert \mathbf{g}^{\prime } \right \vert d\boldsymbol{\omega } \\ =&\sum _{\beta =1}^{s}\int _{\mathbb{R}\times \mathbb{S}^{2}}\left \vert \mathbf{g}\right \vert \,\sigma _{\alpha \beta } \left ( f_{\alpha }^{\prime }f_{\beta \ast }^{\prime }-f_{\alpha }f_{ \beta \ast }\right ) \,d\boldsymbol{\xi }_{\ast }d \boldsymbol{\omega }\text{,} \end{aligned}$$

where

$$ \left \{ \textstyle\begin{array}{c} \boldsymbol{\xi }^{\prime }= \dfrac{m_{\alpha }\boldsymbol{\xi }+m_{\beta }\boldsymbol{\xi }_{\ast }}{m_{\alpha }+m_{\alpha _{j}}}+ \dfrac{m_{\beta }}{m_{\alpha }+m_{\beta }}\left \vert \boldsymbol{\xi }- \boldsymbol{\xi }_{\ast }\right \vert \omega =\mathbf{G}_{\alpha \beta }+\dfrac{m_{\beta }}{m_{\alpha }+m_{\beta }}\left \vert \mathbf{g}\right \vert \omega \\ \boldsymbol{\xi }_{\ast }^{\prime }= \dfrac{m_{\alpha }\boldsymbol{\xi }+m_{\beta }\boldsymbol{\xi }_{\ast }}{m_{\alpha }+m_{\beta }}- \dfrac{m_{\alpha }}{m_{\alpha }+m_{\beta }}\left \vert \boldsymbol{\xi }- \boldsymbol{\xi }_{\ast }\right \vert \omega =\mathbf{G}_{\alpha \beta }- \dfrac{m_{\alpha }}{m_{\alpha }+m_{\beta }}\left \vert \mathbf{g} \right \vert \omega \end{array}\displaystyle \right . \text{, }\omega \in S^{2}\text{,} $$

can be made, resulting in a more familiar form of the Boltzmann collision operator for mixtures.

3.1.2 Collision Invariants and Maxwellian Distributions

The following lemma follows directly by the relations (38).

Lemma 5

The measures

$$ dA_{\alpha \beta }=W_{\alpha \beta }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{ \ast }^{\prime }\right . )\,d\boldsymbol{\xi }\,d\boldsymbol{\xi }_{ \ast }d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime } \textit{, }\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots ,s \right \} \textit{,} $$

are invariant under the (ordered) interchange

$$ \left ( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast }\right ) \leftrightarrow \left ( \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{ \ast }^{\prime }\right ) $$

(40)

of variables, while

$$ dA_{\alpha \beta }+dA_{\beta \alpha }\textit{, }\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots ,s\right \} \textit{,} $$

are invariant under the (ordered) interchange of variables

$$ \left ( \boldsymbol{\xi },\boldsymbol{\xi }^{\prime }\right ) \leftrightarrow \left ( \boldsymbol{\xi }_{\ast },\boldsymbol{\xi }_{ \ast }^{\prime }\right ) \textit{.} $$

(41)

The weak form of the collision operator $Q(f,f)$ reads

$$\begin{aligned} \left ( Q(f,f),g\right ) =&\sum _{\alpha ,\beta =1}^{s}\int _{ \left ( \mathbb{R}^{3}\right ) ^{4}}\left ( f_{\alpha }^{\prime }f_{ \beta \ast }^{\prime }-f_{\alpha }f_{\beta \ast }\right ) g_{\alpha } \,dA_{\alpha \beta } \\ =&\sum _{\alpha ,\beta =1}^{s}\int _{\left ( \mathbb{R}^{3} \right ) ^{4}}\left ( f_{\alpha }^{\prime }f_{\beta \ast }^{\prime }-f_{ \alpha }f_{\beta \ast }\right ) g_{\beta \ast }\,dA_{\alpha \beta } \\ =&-\sum _{\alpha ,\beta =1}^{s}\int _{\left ( \mathbb{R}^{3} \right ) ^{4}}\left ( f_{\alpha }^{\prime }f_{\beta \ast }^{\prime }-f_{ \alpha }f_{\beta \ast }\right ) g_{\alpha }^{\prime }\,dA_{\alpha \beta } \\ =&-\sum _{\alpha ,\beta =1}^{s}\int _{\left ( \mathbb{R}^{3} \right ) ^{4}}\left ( f_{\alpha }^{\prime }f_{\beta \ast }^{\prime }-f_{ \alpha }f_{\beta \ast }\right ) g_{\beta \ast }^{\prime }\,dA_{ \alpha \beta } \end{aligned}$$

for any function $g=\left ( g_{1},\ldots,g_{s}\right )$, such that the first integrals are defined for all indices $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots ,s \right \}$, while the following equalities are obtained by applying Lemma 5.

We have the following proposition.

Proposition 4

Let $g=\left ( g_{1},\ldots,g_{s}\right )$ be such that

$$ \int _{\left ( \mathbb{R}^{3}\right ) ^{4}}\left ( f_{\alpha }^{ \prime }f_{\beta \ast }^{\prime }-f_{\alpha }f_{\beta \ast }\right ) g_{ \alpha }\,dA_{\alpha \beta } $$

is defined for any $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots ,s \right \}$. Then

$$ \left ( Q(f,f),g\right ) =\frac{1}{4}\sum _{\alpha ,\beta =1}^{s} \int _{\left ( \mathbb{R}^{3}\right ) ^{4}}\left ( f_{\alpha }^{ \prime }f_{\beta \ast }^{\prime }-f_{\alpha }f_{\beta \ast }\right ) \left ( g_{\alpha }+g_{\beta \ast }-g_{\alpha }^{\prime }-g_{\beta \ast }^{\prime }\right ) \,dA_{\alpha \beta }. $$

Definition 2

A function $g=\left ( g_{1},\ldots,g_{s}\right )$ is a collision invariant if

$$ \left ( g_{\alpha }+g_{\beta \ast }-g_{\alpha }^{\prime }-g_{\beta \ast }^{\prime }\right ) W_{\alpha \beta }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{ \ast }^{\prime }\right . )=0\text{ a.e.} $$

for all $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots ,s \right \}$.

It is clear that $e_{1}$, ..., $e_{s}$, $m\xi _{x}$, $m\xi _{y}$, $m\xi _{z}$, and $m\left \vert \boldsymbol{\xi }\right \vert ^{2}$, where $\left \{ e_{1},\ldots,e_{s}\right \}$ is the standard basis of $\mathbb{R}^{s}$ and $m=\left ( m_{1},\ldots,m_{s}\right )$, are collision invariants - corresponding to conservation of mass(es), momentum, and kinetic energy.

In fact, we have the following proposition, cf. [15, 20].

Proposition 5

The vector space of collision invariants is generated by

$$ \left \{ e_{1},\ldots,e_{s},m\xi _{x},m\xi _{y},m\xi _{z},m\left \vert \boldsymbol{\xi }\right \vert ^{2}\right \} \textit{,} $$

where $m=\left ( m_{1},\ldots,m_{s}\right )$ and $\left \{ e_{1},\ldots,e_{s}\right \}$ is the standard basis of $\mathbb{R}^{s}$.

Define

$$ \mathcal{W}\left [ f\right ] :=\left ( Q(f,f),\log f\right ) . $$

It follows by Proposition 4 that

$$ \mathcal{W}\left [ f\right ] =-\frac{1}{4}\sum _{\alpha , \beta =1}^{s}\int _{\left ( \mathbb{R}^{3}\right ) ^{4}}f_{\alpha }f_{ \beta \ast }\left ( \frac{f_{\alpha }^{\prime }f_{\beta \ast }^{\prime }}{f_{\alpha }f_{\beta \ast }}-1 \right ) \log \left ( \frac{f_{\alpha }^{\prime }f_{\beta \ast }^{\prime }}{f_{\alpha }f_{\beta \ast }} \right ) \,dA_{\alpha \beta }\leq 0\text{,} $$

with equality if and only if for all $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots ,s \right \}$

$$ \left ( f_{\alpha }f_{\beta \ast }-f_{\alpha }^{\prime }f_{\beta \ast }^{\prime }\right ) W_{\alpha \beta }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{ \ast }^{\prime }\right . )=0\text{ a.e.,} $$

(42)

or, equivalently, if and only if

$$ Q(f,f)\equiv 0\text{.} $$

For any equilibrium, or Maxwellian, distribution $M=\left ( M_{1},\ldots,M_{s}\right )$, it follows, by equation (42), since $Q(M,M)\equiv 0$, that for all $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots ,s \right \}$

$$ \left ( \log M_{\alpha }+\log M_{\beta \ast }-\log M_{\alpha }^{ \prime }-\log M_{\beta \ast }^{\prime }\right ) W_{\alpha \beta }( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime } \right . )=0\text{ a.e. .} $$

Hence, $\log M=\left ( \log M_{1},\ldots,\log M_{s}\right )$ is a collision invariant, and the components of the Maxwellian distributions $M=\left ( M_{1},\ldots,M_{s}\right )$ are Gaussians

$$ M_{\alpha }=n_{\alpha }\left ( \frac{m_{\alpha }}{2\pi T}\right ) ^{3/2}e^{-m_{ \alpha }\left \vert \boldsymbol{\xi }-\mathbf{u}\right \vert ^{2}/ \left ( 2T\right ) }\text{,} $$

where $n_{\alpha }=\left ( M,e_{\alpha }\right )$, $\mathbf{u}=\dfrac{1}{\rho }\left ( M,m\boldsymbol{\xi }\right )$, and $T=\dfrac{1}{3n}\left ( M,m\left \vert \boldsymbol{\xi }-\mathbf{u} \right \vert ^{2}\right )$, with the mass vector $m=\left ( m_{1},\ldots,m_{s}\right )$, while $n=\sum \limits _{\alpha =1}^{s}n_{\alpha }$ and $\rho =\sum \limits _{\alpha =1}^{s}m_{\alpha }n_{\alpha }$.

Note that by equation (42) any Maxwellian distribution $M=(M_{1},\ldots,M_{s})$ for all $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots ,s \right \}$ satisfies the relations

$$ \left ( M_{\alpha }^{\prime }M_{\beta \ast }^{\prime }-M_{\alpha }M_{ \beta \ast }\right ) W_{\alpha \beta }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime }, \boldsymbol{\xi }_{\ast }^{\prime }\right . )=0 \text{ a.e. .} $$

(43)

Remark 6

Introducing the ℋ-functional

$$ \mathcal{H}\left [ f\right ] =\left ( f,\log f\right ) \text{,} $$

an ℋ-theorem can be obtained.

3.1.3 Linearized Collision Operator

Consider a deviation of a Maxwellian distribution $M=(M_{1},\ldots,M_{s})$, where the components are given by $M_{\alpha }=n_{\alpha }\left ( \dfrac{m_{\alpha }}{2\pi }\right ) ^{3/2}e^{-m_{\alpha }\left \vert \boldsymbol{\xi }\right \vert ^{2}/2}$, of the form (13). Insertion in the Boltzmann equation (1) results in a system (14), where the components of the linearized collision operator $\mathcal{L}=\left ( \mathcal{L}_{1},\ldots,\mathcal{L}_{s}\right )$ are given by

$$\begin{aligned} \mathcal{L}_{\alpha }h =&-\sum _{\beta =1}^{s}M_{\alpha }^{-1/2}\left ( Q_{\alpha \beta }(M_{\alpha },M_{\beta }^{1/2}h_{\beta })+Q_{\alpha \beta }(M_{\alpha }^{1/2}h_{\alpha },M_{\beta })\right ) \\ =&\sum _{\beta =1}^{s}\int _{\left ( \mathbb{R}^{3}\right ) ^{3}} \left ( M_{\beta \ast }M_{\alpha }^{\prime }M_{\beta \ast }^{\prime } \right ) ^{1/2}W_{\alpha \beta }(\boldsymbol{\xi },\boldsymbol{\xi }_{ \ast }\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{ \ast }^{\prime }\right . )\, \\ &\times \left ( \frac{h_{\alpha }}{M_{\alpha }^{1/2}}+ \frac{h_{\beta \ast }}{M_{\beta \ast }^{1/2}}- \frac{h_{\alpha }^{\prime }}{\left ( M_{\alpha }^{\prime }\right ) ^{1/2}}- \frac{h_{\beta \ast }^{\prime }}{\left ( M_{\beta \ast }^{\prime }\right ) ^{1/2}} \right ) \,d\boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime } \\ =&\nu _{\alpha }h_{\alpha }-K_{\alpha }\left ( h\right ) \text{, } \end{aligned}$$

(44)

where

$$\begin{aligned} \nu _{\alpha } =&\sum _{\beta =1}^{s}\int _{\left ( \mathbb{R}^{3}\right ) ^{3}}M_{\beta \ast }W_{\alpha \beta }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime }, \boldsymbol{\xi }_{\ast }^{\prime }\right . )\,d\boldsymbol{\xi }_{\ast }d \boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime }, \\ K_{\alpha }\left ( h\right ) =&\sum _{\beta =1}^{s}\int _{ \left ( \mathbb{R}^{3}\right ) ^{3}}\left ( M_{\beta \ast }M_{\alpha }^{\prime }M_{ \beta \ast }^{\prime }\right ) ^{1/2}W_{\alpha \beta }( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime } \right . )\, \\ &\times \left ( \frac{h_{\alpha }^{\prime }}{\left ( M_{\alpha }^{\prime }\right ) ^{1/2}}+ \frac{h_{\beta \ast }^{\prime }}{\left ( M_{\beta \ast }^{\prime }\right ) ^{1/2}}- \frac{h_{\beta \ast }}{M_{\beta \ast }^{1/2}}\right ) \,d\boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d \boldsymbol{\xi }_{\ast }^{\prime }\text{,} \end{aligned}$$

(45)

while the components of the quadratic term $\Gamma =\left ( \Gamma _{1},\ldots,\Gamma _{s}\right )$ are of the form (17).

The multiplication operator $\Lambda$ defined by

$$ \Lambda (f)=\nu f\text{, where }\nu =\mathrm{diag}\left ( \nu _{1},\ldots, \nu _{s}\right ) , $$

is a closed, densely defined, self-adjoint operator on $\left ( L^{2}\left ( d\boldsymbol{\xi }\right ) \right ) ^{s}$. It is Fredholm, as well, if and only if $\Lambda$ is coercive.

The following lemma follows immediately by Lemma 5.

Lemma 6

For any $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots ,s \right \}$, the measure

$$ d\widetilde{A}_{\alpha \beta }=\left ( M_{\alpha }M_{\beta \ast }M_{ \alpha }^{\prime }M_{\beta \ast }^{\prime }\right ) ^{1/2}dA_{\alpha \beta } $$

is invariant under the (ordered) interchange (40) of variables, while

$$ d\widetilde{A}_{\alpha \beta }+d\widetilde{A}_{\beta \alpha } $$

is invariant under the (ordered) interchange (41) of variables.

The weak form of the linearized collision operator ℒ reads

$$\begin{aligned} &\left ( \mathcal{L}h,g\right ) \\ =&\sum _{\alpha ,\beta =1}^{s}\int _{\left ( \mathbb{R}^{3} \right ) ^{4}}\left ( \frac{h_{\alpha }}{M_{\alpha }^{1/2}}+ \frac{h_{\beta \ast }}{M_{\beta \ast }^{1/2}}- \frac{h_{\alpha }^{\prime }}{\left ( M_{\alpha }^{\prime }\right ) ^{1/2}}- \frac{h_{\beta \ast }^{\prime }}{\left ( M_{\beta \ast }^{\prime }\right ) ^{1/2}} \right ) \frac{g_{\alpha }}{M_{\alpha }^{1/2}}\,d\widetilde{A}_{\alpha \beta } \\ =&\sum _{\alpha ,\beta =1}^{s}\int _{\left ( \mathbb{R}^{3} \right ) ^{4}}\left ( \frac{h_{\alpha }}{M_{\alpha }^{1/2}}+ \frac{h_{\beta \ast }}{M_{\beta \ast }^{1/2}}- \frac{h_{\alpha }^{\prime }}{\left ( M_{\alpha }^{\prime }\right ) ^{1/2}}- \frac{h_{\beta \ast }^{\prime }}{\left ( M_{\beta \ast }^{\prime }\right ) ^{1/2}} \right ) \frac{g_{\beta \ast }}{M_{\beta \ast }^{1/2}}\,d \widetilde{A}_{\alpha \beta } \\ =&-\sum _{\alpha ,\beta =1}^{s}\int _{\left ( \mathbb{R}^{3} \right ) ^{4}}\left ( \frac{h_{\alpha }}{M_{\alpha }^{1/2}}+ \frac{h_{\beta \ast }}{M_{\beta \ast }^{1/2}}- \frac{h_{\alpha }^{\prime }}{\left ( M_{\alpha }^{\prime }\right ) ^{1/2}}- \frac{h_{\beta \ast }^{\prime }}{\left ( M_{\beta \ast }^{\prime }\right ) ^{1/2}} \right ) \frac{g_{\alpha }^{\prime }}{\left ( M_{\alpha }^{\prime }\right ) ^{1/2}}\,d\widetilde{A}_{\alpha \beta } \\ =&-\sum _{\alpha ,\beta =1}^{s}\int _{\left ( \mathbb{R}^{3} \right ) ^{4}}\!\left ( \!\frac{h_{\alpha }}{M_{\alpha }^{1/2}}+ \frac{h_{\beta \ast }}{M_{\beta \ast }^{1/2}}- \frac{h_{\alpha }^{\prime }}{\left ( M_{\alpha }^{\prime }\right ) ^{1/2}}- \frac{h_{\beta \ast }^{\prime }}{\left ( M_{\beta \ast }^{\prime }\right ) ^{1/2}} \!\right ) \! \frac{g_{\beta \ast }^{\prime }}{\left ( M_{\beta \ast }^{\prime }\right ) ^{1/2}}d\widetilde{A}_{ \alpha \beta }\!, \end{aligned}$$

for any function $g=\left ( g_{1},\ldots,g_{s}\right )$, such that the first integrals are defined for all indices $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots ,s \right \}$, while the following equalities are obtained by applying Lemma 6.

We have the following lemma.

Lemma 7

Let $g=\left ( g_{1},\ldots,g_{s}\right )$ be such that for any $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots ,s \right \}$

$$ \int _{\left ( \mathbb{R}^{3}\right ) ^{4}}\left ( \frac{h_{\alpha }}{M_{\alpha }^{1/2}}+ \frac{h_{\beta \ast }}{M_{\beta \ast }^{1/2}}- \frac{h_{\alpha }^{\prime }}{\left ( M_{\alpha }^{\prime }\right ) ^{1/2}}- \frac{h_{\beta \ast }^{\prime }}{\left ( M_{\beta \ast }^{\prime }\right ) ^{1/2}} \right ) \frac{g_{\alpha }}{M_{\alpha }^{1/2}}\,d\widetilde{A}_{\alpha \beta } $$

is defined. Then

$$\begin{aligned} \left ( \mathcal{L}h,g\right ) =&\frac{1}{4}\sum _{\alpha , \beta =1}^{s}\int _{\left ( \mathbb{R}^{3}\right ) ^{4}}\left ( \frac{h_{\alpha }}{M_{\alpha }^{1/2}}+\frac{h_{\beta \ast }}{M_{\beta \ast }^{1/2}}- \frac{h_{\alpha }^{\prime }}{\left ( M_{\alpha }^{\prime }\right ) ^{1/2}}- \frac{h_{\beta \ast }^{\prime }}{\left ( M_{\beta \ast }^{\prime }\right ) ^{1/2}}\right ) \\ &\times \left ( \frac{g_{\alpha }}{M_{\alpha }^{1/2}}+ \frac{g_{\beta \ast }}{M_{\beta \ast }^{1/2}}- \frac{g_{\alpha }^{\prime }}{\left ( M_{\alpha }^{\prime }\right ) ^{1/2}}- \frac{g_{\beta \ast }^{\prime }}{\left ( M_{\beta \ast }^{\prime }\right ) ^{1/2}} \right ) d\widetilde{A}_{\alpha \beta }. \end{aligned}$$

Proposition 6

The linearized collision operator is symmetric and nonnegative,

$$ \left ( \mathcal{L}h,g\right ) =\left ( h,\mathcal{L}g\right ) \textit{ and }\left ( \mathcal{L}h,h\right ) \geq 0\textit{,} $$

and the kernel of ℒ, $\ker \mathcal{L}$, is generated by

$$ \left \{ \sqrt{M_{1}}e_{1},\ldots,\sqrt{M_{s}}e_{s},\mathcal{M}^{1/2}mv_{x},\mathcal{M}^{1/2}mv_{y},\mathcal{M}^{1/2}mv_{z},\mathcal{M}^{1/2}m \left \vert \mathbf{v}\right \vert ^{2}\right \} , $$

where $\mathcal{M}=\mathrm{diag}\left ( M_{1},\ldots,M_{s}\right )$, $m=\left ( m_{1},\ldots,m_{s}\right )$, and $\left \{ e_{1},\ldots,e_{s}\right \}$ is the standard basis of $\mathbb{R}^{s}$.

Proof

By Lemma 7, it is immediate that $\left ( \mathcal{L}h,g\right ) =\left ( h,\mathcal{L}g\right )$, and

$$ \left ( \mathcal{L}h,h\right ) =\frac{1}{4}\sum _{\alpha , \beta =1}^{s}\int _{\left ( \mathbb{R}^{3}\right ) ^{4}}\left ( \frac{h_{\alpha }}{M_{\alpha }^{1/2}}+\frac{h_{\beta \ast }}{M_{\beta \ast }^{1/2}}- \frac{h_{\alpha }^{\prime }}{\left ( M_{\alpha }^{\prime }\right ) ^{1/2}}- \frac{h_{\beta \ast }^{\prime }}{\left ( M_{\beta \ast }^{\prime }\right ) ^{1/2}}\right ) ^{2}d\widetilde{A}_{\alpha \beta }\geq 0. $$

Furthermore, $h\in \ker \mathcal{L}$ if and only if $\left ( \mathcal{L}h,h\right ) =0$, which will be fulfilled if and only if for all $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots ,s \right \}$

$$ \left ( \frac{h_{\alpha }}{M_{\alpha }^{1/2}}+ \frac{h_{\beta \ast }}{M_{\beta \ast }^{1/2}}- \frac{h_{\alpha }^{\prime }}{\left ( M_{\alpha }^{\prime }\right ) ^{1/2}}- \frac{h_{\beta \ast }^{\prime }}{\left ( M_{\beta \ast }^{\prime }\right ) ^{1/2}} \right ) W_{\alpha \beta }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime }, \boldsymbol{\xi }_{\ast }^{\prime }\right . )=0\text{ a.e.,} $$

i.e. if and only if $\mathcal{M}^{-1/2}h$ is a collision invariant. The last part of the lemma now follows by Proposition 5. □

Remark 7

Again, with exactly the same arguments as in Remark 4, although without relevance for the studies here still of great importance, the nonlinear term is orthogonal to the kernel of ℒ, i.e. $\Gamma \left ( h,h\right ) \in \left ( \ker \mathcal{L}\right ) ^{ \perp _{\mathcal{\mathfrak{h}}^{(s)}}}$.

3.2 Focused Properties

This section is devoted to results concerning the compactness property in Theorem 3 and bounds of collision frequencies in Theorem 4.

Assume that for some positive number $\gamma$, such that $0<\gamma <1$, there is a positive constant $C$ such that, cf. [10],

$$ 0\leq \sigma _{\alpha \beta }\left ( \left \vert \mathbf{g}\right \vert ,\cos \theta \right ) \leq C\left ( 1+ \frac{1}{\left \vert \mathbf{g}\right \vert ^{2-\gamma }}\right ) \text{, }0< \gamma < 1\text{,} $$

(46)

on the scattering cross sections $\sigma _{\alpha \beta }$ for any $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots,s\right \}$. This includes, but are not limited to inverse power law potentials under Grad’s cut-off [19], implying scattering cross sections of the form, cf. [10],

$$ \sigma _{\alpha \beta }=b_{\alpha \beta }^{\mu _{\alpha \beta }} \left ( \cos \theta \right ) \left \vert \mathbf{g}\right \vert ^{ \mu _{\alpha \beta }-1}\text{, with }\mu _{\alpha \beta }= \frac{\varsigma _{\alpha \beta }-5}{\varsigma _{\alpha \beta }-1} \text{, where }\varsigma _{\alpha \beta }>3\text{,} $$

(47)

for bounded positive functions $b_{\alpha \beta }^{\mu }\left ( \cos \theta \right )$, including the limiting case of hard sphere gases for which $\sigma _{\alpha \beta }=C_{\alpha \beta }$ for some positive constant $C_{\alpha \beta }$ (i.e. with $\mu _{\alpha \beta } =1$ and $b_{\alpha \beta }^{\mu }\left ( \cos \theta \right ) =C_{\alpha \beta }$ in expression (47)) for any $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots,s\right \}$.

Remark 8

Note that due to a different choice of velocity parametrization Grad’s assumption on the collision kernel in [19] also has a factor $\cos \widetilde{\theta }$, where $\widetilde{\theta }= \dfrac{\mathbf{g}}{\left \vert \mathbf{g}\right \vert }\cdot \dfrac{\widetilde{\mathbf{g}}}{\left \vert \widetilde{\mathbf{g}}\right \vert }$, with $\mathbf{g}=\boldsymbol{\xi }-\boldsymbol{\xi }$ and $\widetilde{\mathbf{g}}=\boldsymbol{\xi }-\boldsymbol{\xi }^{\prime }$, added in the upper bound (46), as well as, since having parametrized in azimuthal and polar angles as integration variables in the collision integral, a factor $\sin \widetilde{\theta }$, cf. Remark 2, also adds. It appears in the literature, see e.g. [10, 12], that a factor $\sin \widetilde{\theta }$ is added in the upper bound although that the collision integral is expressed by a non-parametrized vector in $\mathbb{S}^{2}$, cf. Remark 2 rending in (seemingly) more restrictive conditions, excluding e.g., the important case of hard spheres. However, this might be of technical nature, rather than affecting the validity of the posed results and their proofs.

The following result may be obtained.

Theorem 3

Assume that the scattering cross sections $\sigma _{\alpha \beta }$ for $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots,s\right \}$ satisfy the bound (46) for some positive number $\gamma$, such that $0<\gamma <1$. Then the operator $K=\left ( K_{1},\ldots,K_{s}\right )$, with the components $K_{\alpha }$ given by (45) is a self-adjoint compact operator on $\left ( L^{2}\left ( d\boldsymbol{\xi }\right ) \right ) ^{s}$.

Theorem 3 will be proven in Sect. 3.3.1, based on ideas from the corresponding proof for monatomic single species by Grad [19], especially, as presented by Glassey [18], combined by an crucial lemma by Boudin et al. [10], where a complete proof based on the same lemma and the proof of Grad appeared first. The main innovation in this work is that by starting from the probabilistic formulation, there is no initial parametrization of the velocities based on the momentum and kinetic energy conservation. Then suitable parametrizations can be chosen from scratch from case to case. From our point of view, this really helps to find appropriate substitutions, by geometrical motivations and manipulations of the delta-functions, for the non-trivial cases. The substitutions were developed independently, but are related to the ones in [10, 19]. Based on ideas by [10, 18, 19] the proof is completed. Restricted to equal masses the proof is essentially similar to the one for monatomic single species.

Corollary 4

The linearized collision operator ℒ, with scattering cross sections satisfying (46), is a closed, densely defined, self-adjoint operator on $\left ( L^{2}\left ( d\boldsymbol{\xi }\right ) \right ) ^{s}$.

Now consider a hard sphere model, i.e. such that

$$ \sigma _{\alpha \beta }=C_{\alpha \beta } $$

(48)

for some positive constant $C_{\alpha \beta }>0$ for all $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots,s\right \}$.

In fact, it would be enough with the bounds

$$ C_{-}\leq \sigma _{\alpha \beta }\leq C_{+} $$

(49)

for some positive constants $C_{\pm }>0$ and all $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots,s\right \}$, on the scattering cross sections.

Theorem 4

[10] The linearized collision operator ℒ, for a hard sphere model (48) (or (49)), can be decomposed as a positive multiplication operator $\Lambda$, where $\Lambda f=\nu f$ for $\nu =\nu (\left \vert \boldsymbol{\xi }\right \vert )=\mathrm{diag} \left ( \nu _{1},\ldots,\nu _{s}\right )$, minus a compact operator $K$ on $\left ( L^{2}\left ( d\boldsymbol{\xi }\right ) \right ) ^{s}$

$$ \mathcal{L}=\Lambda -K, $$

(50)

where there exist positive numbers $\nu _{-}$ and $\nu _{+}$, $0<\nu _{-}<\nu _{+}$, such that for any $\alpha \in \left \{ 1,\ldots,s\right \}$

$$ \nu _{-}\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right ) \leq \nu _{\alpha }(\left \vert \boldsymbol{\xi }\right \vert )\leq \nu _{+}\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right ) \textit{ for all }\boldsymbol{\xi }\in \mathbb{R}^{3}\textit{.} $$

(51)

The decomposition (50) follows by the decomposition (44), (45) and Theorem 3, while the bounds (51) are proven in Sect. 3.3.2, essentially mimicking the proof for monatomic single species.

Corollary 5

The linearized collision operator ℒ, for a hard-sphere model (48) (or (49)), is a Fredholm operator, with domain

$$ D(\mathcal{L})=\left ( L^{2}\left ( \left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right ) d\boldsymbol{\xi }\right ) \right ) ^{s} \textit{.} $$

Corollary 6

For the linearized collision operator ℒ, for a hard sphere model (48) (or (49)), there exists a positive number $\lambda$, $0<\lambda <1$, such that

$$ \left ( h,\mathcal{L}h\right ) \geq \lambda \left ( h,\nu (\left \vert \boldsymbol{\xi }\right \vert )h\right ) \geq \lambda \nu _{-} \left ( h,\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right ) h\right ) $$

for any $h\in \left ( L^{2}(\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right ) d\boldsymbol{\xi })\right ) ^{s}\cap \mathrm{Im} \mathcal{L}$.

Remark 9

By Proposition 6 and Corollary 4–6 the linearized operator ℒ fulfills the properties assumed on the linear operators in [4], and hence, the results therein can be applied to hard sphere models, see [3].

3.3 Compactness and Bounds on the Collision Frequency

This section is devoted to the proofs of the compactness property in Theorem 3, cf. the proof by Boudin et al. in [10] and the variant by Glassey [18] of the one by Grad [19] for monatomic single species, and the bounds on the collision frequency in Theorem 4, cf. corresponding proof for monatomic single species, of the linearized collision operator for (monatomic) multicomponent mixtures.

3.3.1 Compactness

This section concerns the proof of Theorem 3. Note that in the proof the kernels are rewritten in such a way that $\boldsymbol{\xi }_{\ast }$ - and not $\boldsymbol{\xi }^{\prime }$ and $\boldsymbol{\xi }_{\ast }^{\prime }$ - always will be argument of the distribution functions. As for single species, either $\boldsymbol{\xi }_{\ast }$ is an argument in the loss term (like $\boldsymbol{\xi }$) or in the gain term (unlike $\boldsymbol{\xi }$) of the collision operator. However, in the latter case, unlike for single species, for mixtures we have to differ between two different cases; either $\boldsymbol{\xi }_{\ast }$ is associated to the same species as $\boldsymbol{\xi }$, or not. The kernels of the terms from the loss part of the collision operator will be shown to be Hilbert-Schmidt in a quite direct way. The kernels of - some of - the terms - for which $\boldsymbol{\xi }_{\ast }$ is associated to the same species as $\boldsymbol{\xi }$ - from the gain parts of the collision operators will be shown to be approximately Hilbert-Schmidt in the sense of Lemma 4. By applying the following lemma, Lemma 8, (for disparate masses) by Boudin et al. in [10], it will be shown that the kernels of the remaining terms - i.e. for which $\boldsymbol{\xi }_{\ast }$ is associated to the opposite species to $\boldsymbol{\xi }$ - from the gain parts of the collision operators, are Hilbert-Schmidt.

Lemma 8

[10] Assume that $m_{\alpha }\neq m_{\beta }$,

$$ \left \{ \textstyle\begin{array}{l} \boldsymbol{\xi }^{\prime }=\boldsymbol{\xi }_{\ast }- \dfrac{m_{\alpha }}{m_{\beta }}\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }^{ \prime }\right \vert \boldsymbol{\eta } \\ \boldsymbol{\xi }_{\ast }^{\prime }=\boldsymbol{\xi }-\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }^{\prime }\right \vert \boldsymbol{\eta }\end{array}\displaystyle \right . \textit{, where }\boldsymbol{\eta }\in \mathbb{S}^{2}\textit{,} $$

(52)

and

$$ m_{\alpha }\left \vert \boldsymbol{\xi }\right \vert ^{2}+m_{\beta } \left \vert \boldsymbol{\xi }^{\prime }\right \vert ^{2}=m_{\alpha } \left \vert \boldsymbol{\xi }_{\ast }^{\prime }\right \vert ^{2}+m_{ \beta }\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}\textit{.} $$

(53)

Then there exists a positive number $\rho$, $0<\rho <1$, such that

$$ m_{\beta }\left \vert \boldsymbol{\xi }^{\prime }\right \vert ^{2}+m_{ \alpha }\left \vert \boldsymbol{\xi }_{\ast }^{\prime }\right \vert ^{2} \geq \rho \left ( m_{\alpha }\left \vert \boldsymbol{\xi }\right \vert ^{2}+m_{\beta }\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}\right ) \textit{.} $$

An alternative - and more basic, in the sense that only very basic calculations are used - to the proof of Lemma 8 in [10] is accounted for in the Appendix. The proof is constructive, in the way that an explicit - not necessarily optimal - value of such a number $\rho$, namely

$$ \rho =\left ( \frac{\sqrt{m_{\alpha }}-\sqrt{m_{\beta }}}{\sqrt{m_{\alpha }}+\sqrt{m_{\beta }}}\right ) ^{2}\text{,} $$

is obtained in the proof.

Now we turn to the proof of Theorem 3.

Proof

For any $\left \{ \alpha ,\beta \right \} \subset \left \{ 1,\ldots,s\right \}$, by firstly renaming $\left \{ \boldsymbol{\xi }_{\ast }\right \} \leftrightarrows \left \{ \boldsymbol{\xi }^{\prime }\right \}$ and then secondly $\left \{ \boldsymbol{\xi }_{\ast }\right \} \leftrightarrows \left \{ \boldsymbol{\xi }_{\ast }^{\prime }\right \}$,

$$\begin{aligned} &\int _{\left ( \mathbb{R}^{3}\right ) ^{3}}\left ( M_{\beta \ast }M_{ \alpha }^{\prime }\right ) ^{1/2}W_{\alpha \beta }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime }, \boldsymbol{\xi }_{\ast }^{\prime }\right . )\,\,h_{\beta \ast }^{ \prime }\,d\boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d \boldsymbol{\xi }_{\ast }^{\prime } \\ =&\int _{\left ( \mathbb{R}^{3}\right ) ^{3}}\left ( M_{\beta }^{ \prime }M_{\alpha \ast }\right ) ^{1/2}W_{\alpha \beta }( \boldsymbol{\xi },\boldsymbol{\xi }^{\prime }\left \vert \boldsymbol{\xi }_{\ast }, \boldsymbol{\xi }_{\ast }^{\prime }\right . )\,h_{\beta \ast }^{\prime }\,d \boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{ \prime } \\ =&\int _{\left ( \mathbb{R}^{3}\right ) ^{3}}\left ( M_{\beta }^{ \prime }M_{\alpha \ast }^{\prime }\right ) ^{1/2}W_{\alpha \beta }( \boldsymbol{\xi },\boldsymbol{\xi }^{\prime }\left \vert \boldsymbol{\xi }_{\ast }^{ \prime },\boldsymbol{\xi }_{\ast }\right . )\,h_{\beta \ast }\,d \boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{ \prime }\text{.} \end{aligned}$$

Moreover, for any $\left \{ \alpha ,\beta \right \} \subset \left \{ 1,\ldots,s\right \}$, by renaming $\left \{ \boldsymbol{\xi }_{\ast }\right \} \leftrightarrows \left \{ \boldsymbol{\xi }^{\prime }\right \}$,

$$\begin{aligned} &\int _{\left ( \mathbb{R}^{3}\right ) ^{3}}\left ( M_{\beta \ast }M_{ \beta \ast }^{\prime }\right ) ^{1/2}W_{\alpha \beta }( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime }, \boldsymbol{\xi }_{\ast }^{\prime }\right . )\,h_{\alpha }^{\prime }\,d \boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{ \prime } \\ =&\int _{\left ( \mathbb{R}^{3}\right ) ^{3}}\left ( M_{\beta }^{ \prime }M_{\beta \ast }^{\prime }\right ) ^{1/2}W_{\alpha \beta }( \boldsymbol{\xi },\boldsymbol{\xi }^{\prime }\left \vert \boldsymbol{\xi }_{\ast }, \boldsymbol{\xi }_{\ast }^{\prime }\right . )\,h_{\alpha \ast }\,d \boldsymbol{\xi }_{\ast }d\boldsymbol{\xi }^{\prime }d \boldsymbol{\xi }_{\ast }^{\prime }\text{.} \end{aligned}$$

It follows that for any $\alpha \in \left \{ 1,\ldots,s\right \}$

$$\begin{aligned} K_{\alpha }\left ( h\right ) =&\sum _{\beta =1}^{s}\int _{ \mathbb{R}^{3}}k_{\alpha \beta }\left ( \boldsymbol{\xi },\boldsymbol{\xi }_{ \ast }\right ) \,h_{\ast }\,d\boldsymbol{\xi }_{\ast }\text{, where } \\ k_{\alpha \beta }h_{\ast } =&k_{\alpha \beta }^{\left ( \alpha \right ) }h_{\alpha \ast }+k_{\alpha \beta }^{\left ( \beta \right ) }h_{ \beta \ast }=k_{\alpha \beta }^{\left ( \alpha \right ) }h_{\alpha \ast }-k_{\alpha \beta 1}^{\left ( \beta \right ) }h_{\beta \ast }+k_{ \alpha \beta 2}^{\left ( \beta \right ) }h_{\beta \ast }\text{, with} \\ k_{\alpha \beta }^{\left ( \alpha \right ) }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }) =&\int _{\left ( \mathbb{R}^{3}\right ) ^{2}} \left ( M_{\beta }^{\prime }M_{\beta \ast }^{\prime }\right ) ^{1/2}W_{ \alpha \beta }(\boldsymbol{\xi },\boldsymbol{\xi }^{\prime }\left \vert \boldsymbol{\xi }_{\ast },\boldsymbol{\xi }_{\ast }^{\prime }\right . )\,d \boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime }\text{,} \\ k_{\alpha \beta 1}^{\left ( \beta \right ) }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }) =&\int _{\left ( \mathbb{R}^{3}\right ) ^{2}} \left ( M_{\alpha }^{\prime }M_{\beta \ast }^{\prime }\right ) ^{1/2}W_{ \alpha \beta }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime }\right . )\,d \boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime }\text{, and} \\ k_{\alpha \beta 2}^{\left ( \beta \right ) }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }) =&\int _{\left ( \mathbb{R}^{3}\right ) ^{2}} \left ( M_{\alpha \ast }^{\prime }M_{\beta }^{\prime }\right ) ^{1/2}W_{ \alpha \beta }\left ( \boldsymbol{\xi },\boldsymbol{\xi }^{\prime } \left \vert \boldsymbol{\xi }_{\ast }^{\prime },\boldsymbol{\xi }_{\ast }\right . \right ) \,d \boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime }\text{.} \end{aligned}$$

(54)

Next we obtain some symmetry relations that will help to yield self-adjointness of the operator $K$ below. By applying the second relation in (38) and renaming $\left \{ \boldsymbol{\xi }^{\prime }\right \} \leftrightarrows \left \{ \boldsymbol{\xi }_{\ast }^{\prime }\right \}$,

$$\begin{aligned} k_{\alpha \beta }^{\left ( \alpha \right ) }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }) =&\int _{\left ( \mathbb{R}^{3}\right ) ^{2}} \left ( M_{\beta }^{\prime }M_{\beta \ast }^{\prime }\right ) ^{1/2}W_{ \alpha \beta }(\boldsymbol{\xi }_{\ast },\boldsymbol{\xi }_{\ast }^{\prime }\left \vert \boldsymbol{\xi },\boldsymbol{\xi }^{\prime }\right . )\,d \boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime } \\ =&\int _{\left ( \mathbb{R}^{3}\right ) ^{2}}\left ( M_{\beta }^{ \prime }M_{\beta \ast }^{\prime }\right ) ^{1/2}W_{\alpha \beta }( \boldsymbol{\xi }_{\ast },\boldsymbol{\xi }^{\prime }\left \vert \boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }^{\prime }\right . )\,d\boldsymbol{\xi }^{\prime }d \boldsymbol{\xi }_{\ast }^{\prime } \\ =&k_{\alpha \beta }^{\left ( \alpha \right ) }(\boldsymbol{\xi }_{ \ast },\boldsymbol{\xi }) \end{aligned}$$

(55)

for all $\left \{ \alpha ,\beta \right \} \subset \left \{ 1,\ldots,s\right \}$. Moreover, for all $\left \{ \alpha ,\beta \right \} \subset \left \{ 1,\ldots,s\right \}$

$$ k_{\alpha \beta }^{\left ( \beta \right ) }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast })=k_{\beta \alpha 1}^{\left ( \alpha \right ) }( \boldsymbol{\xi }_{\ast },\boldsymbol{\xi })-k_{\beta \alpha 2}^{\left ( \alpha \right ) }(\boldsymbol{\xi }_{\ast },\boldsymbol{\xi })=k_{\beta \alpha }^{ \left ( \alpha \right ) }(\boldsymbol{\xi }_{\ast },\boldsymbol{\xi }), $$

(56)

since, by applying the first relation in (38) and renaming $\left \{ \boldsymbol{\xi }^{\prime }\right \} \leftrightarrows \left \{ \boldsymbol{\xi }_{\ast }^{\prime }\right \}$,

$$\begin{aligned} k_{\alpha \beta 1}^{\left ( \beta \right ) }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }) =&\int _{\left ( \mathbb{R}^{3}\right ) ^{2}} \left ( M_{\alpha }^{\prime }M_{\beta \ast }^{\prime }\right ) ^{1/2}W_{ \beta \alpha }(\boldsymbol{\xi }_{\ast },\boldsymbol{\xi }\left \vert \boldsymbol{\xi }_{\ast }^{\prime },\boldsymbol{\xi }^{\prime }\right . )\,d \boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime } \\ =&\int _{\left ( \mathbb{R}^{3}\right ) ^{2}}\left ( M_{\alpha \ast }^{ \prime }M_{\beta }^{\prime }\right ) ^{1/2}W_{\beta \alpha }( \boldsymbol{\xi }_{\ast },\boldsymbol{\xi }\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime }\right . )\,d\boldsymbol{\xi }^{\prime }d \boldsymbol{\xi }_{\ast }^{\prime } \\ =&k_{\beta \alpha 1}^{\left ( \alpha \right ) }(\boldsymbol{\xi }_{ \ast },\boldsymbol{\xi })\text{,} \end{aligned}$$

while, by applying the two first relations in (38) and renaming $\left \{ \boldsymbol{\xi }^{\prime }\right \} \leftrightarrows \left \{ \boldsymbol{\xi }_{\ast }^{\prime }\right \}$,

$$\begin{aligned} k_{\alpha \beta 2}^{\left ( \beta \right ) }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }) =&\int _{\left ( \mathbb{R}^{3}\right ) ^{2}} \left ( M_{\alpha \ast }^{\prime }M_{\beta }^{\prime }\right ) ^{1/2}W_{ \beta \alpha }\left ( \boldsymbol{\xi }^{\prime },\boldsymbol{\xi } \left \vert \boldsymbol{\xi }_{\ast },\boldsymbol{\xi }_{\ast }^{\prime }\right . \right ) \,d \boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime } \\ =&\int _{\left ( \mathbb{R}^{3}\right ) ^{2}}\left ( M_{\alpha \ast }^{ \prime }M_{\beta }^{\prime }\right ) ^{1/2}W_{\beta \alpha }\left ( \boldsymbol{\xi }_{\ast },\boldsymbol{\xi }_{\ast }^{\prime }\left \vert \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }\right . \right ) \,d\boldsymbol{\xi }^{ \prime }d\boldsymbol{\xi }_{\ast }^{\prime } \\ =&\int _{\left ( \mathbb{R}^{3}\right ) ^{2}}\left ( M_{\alpha }^{ \prime }M_{\beta \ast }^{\prime }\right ) ^{1/2}W_{\beta \alpha } \left ( \boldsymbol{\xi }_{\ast },\boldsymbol{\xi }^{\prime }\left \vert \boldsymbol{\xi }_{\ast }^{\prime },\boldsymbol{\xi }\right . \right ) \,d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime } \\ =&k_{\beta \alpha 2}^{\left ( \alpha \right ) }(\boldsymbol{\xi }_{ \ast },\boldsymbol{\xi })\text{.} \end{aligned}$$

We now continue by proving the compactness for the three different types of collision kernel separately. Note that, by applying the last relation in (38), $k_{\alpha \beta 2}^{\left ( \beta \right ) }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })=k_{\alpha \beta }^{ \left ( \alpha \right ) }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })$ if $\alpha =\beta$, and we will remain with only two cases - the first two below. Even if $m_{\alpha }=m_{\beta }$, the kernels $k_{\alpha \beta }^{\left ( \alpha \right ) }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast })$ and $k_{\alpha \beta 2}^{\left ( \beta \right ) }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast })$ are structurally equal, why we (in principle) remain with (first) two cases (the second one twice).

I. Compactness of $K_{\alpha \beta }^{(1)}=\int _{ \mathbb{R}^{3}}k_{\alpha \beta 1}^{\left ( \beta \right ) }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\,h_{\beta \ast }\,d\boldsymbol{\xi }_{ \ast }$ for $\left \{ \alpha ,\beta \right \} \subset \left \{ 1,\ldots,s\right \}$.

Assume the velocities $\boldsymbol{\xi }$ and $\boldsymbol{\xi }_{\ast }$ to be given. Then a collision will be uniquely defined by the unit vector $\boldsymbol{\omega }=\mathbf{g}^{\prime }/\left \vert \mathbf{g}^{ \prime }\right \vert$, with $\mathbf{g}^{\prime }=\boldsymbol{\xi }^{\prime }-\boldsymbol{\xi }_{ \ast }^{\prime }$. This follows, since, by conservation of momentum and kinetic energy (2), $\left \vert \mathbf{g}^{\prime }\right \vert =\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }^{\prime }\right \vert = \left \vert \mathbf{g}\right \vert$, while also $m_{\alpha }\left (\boldsymbol{\xi }-\boldsymbol{\xi }^{\prime } \right )=m_{\beta }\left (\boldsymbol{\xi }_{\ast}^{\prime }- \boldsymbol{\xi }_{\ast}\right )$, cf. Fig. 3. Indeed, by applying the change of variables $\left \{ \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{ \prime }\right \} \rightarrow \left \{ \left \vert \mathbf{g}^{ \prime }\right \vert ,\boldsymbol{\omega }= \dfrac{\mathbf{g}^{\prime }}{\left \vert \mathbf{g}^{\prime }\right \vert }, \mathbf{G}_{\alpha \beta }^{\prime }=\dfrac{m_{\alpha }\boldsymbol{\xi }^{\prime }+m_{\beta }\boldsymbol{\xi }_{\ast }^{\prime }}{m_{\alpha }+m_{\beta }}\right \}$, noting that (39), and using relation (43), expression (54) of $k_{\alpha \beta 1}^{\left ( \beta \right ) }$ may be transformed to

$$\begin{aligned} &k_{\alpha \beta 1}^{\left ( \beta \right ) }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }) \\ =&\left ( M_{\alpha }M_{\beta \ast }\right ) ^{1/2}\int _{ \mathbb{R}^{3}\times \mathbb{R}_{+}\times \mathbb{S}^{2}} \frac{\sigma _{\alpha \beta }\left \vert \mathbf{g}^{\prime }\right \vert ^{2}}{\left \vert \mathbf{g}\right \vert }\delta _{3}\left ( \mathbf{G}_{\alpha \beta }- \mathbf{G}_{\alpha \beta }^{\prime }\right ) \delta _{1}\left ( \left \vert \mathbf{g}\right \vert -\left \vert \mathbf{g}^{\prime }\right \vert \right ) \,d\mathbf{G}_{\alpha \beta }^{\prime }d\left \vert \mathbf{g}^{\prime }\right \vert d\boldsymbol{\omega } \\ =&\left ( M_{\alpha }M_{\beta \ast }\right ) ^{1/2}\left \vert \mathbf{g}\right \vert \int _{\mathbb{S}^{2}}\sigma _{\alpha \beta }\left ( \left \vert \mathbf{g}\right \vert ,\cos \theta \right ) \,d \boldsymbol{\omega }\text{, with }\cos \theta =\boldsymbol{\omega } \cdot \frac{\mathbf{g}}{\left \vert \mathbf{g}\right \vert }\text{.} \end{aligned}$$

By assumption (46) and the relation

$$ m_{\alpha }\left \vert \boldsymbol{\xi }\right \vert ^{2}+m_{\beta } \left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}=\left ( m_{ \alpha }+m_{\beta }\right ) \left \vert \mathbf{G}_{\alpha \beta } \right \vert ^{2}+\mu _{\alpha \beta }\left \vert \mathbf{g}\right \vert ^{2}\text{, with }\mu _{\alpha \beta }= \frac{m_{\alpha }m_{\beta }}{m_{\alpha }+m_{\beta }}\text{,} $$

for the exponent of the product $\left (M_{\alpha }M_{\beta \ast }\right )^{2}$, the bound

$$\begin{aligned} \left ( k_{\alpha \beta 1}^{\left ( \beta \right ) }( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\right ) ^{2} \leq &CM_{\alpha }M_{\beta \ast }\left ( 1+ \frac{1}{\left \vert \mathbf{g}\right \vert ^{2-\gamma }}\right ) ^{2} \left \vert \mathbf{g}\right \vert ^{2} \\ =&Ce^{-\left ( m_{\alpha }+m_{\beta }\right ) \left \vert \mathbf{G}_{ \alpha \beta }\right \vert ^{2}/2-\mu _{\alpha \beta }\left \vert \mathbf{g}\right \vert ^{2}/2}\left ( \left \vert \mathbf{g}\right \vert + \frac{1}{\left \vert \mathbf{g}\right \vert ^{1-\gamma }}\right ) ^{2} \end{aligned}$$

(57)

may be obtained. Then, by applying the bound (57) and first changing variables of integration $\left \{ \boldsymbol{\xi },\boldsymbol{\xi }_{\ast }\right \} \rightarrow \left \{ \mathbf{g}, \mathbf{G}_{\alpha \beta }\right \}$, with unitary Jacobian, and then to spherical coordinates,

$$\begin{aligned} &\int _{\left ( \mathbb{R}^{3}\right ) ^{2}}\left ( k_{\alpha \beta 1}^{ \left ( \beta \right ) }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }) \right ) ^{2}d\boldsymbol{\xi }d\boldsymbol{\xi }_{\ast } \\ \leq &C\int _{\left ( \mathbb{R}^{3}\right ) ^{2}}e^{-\left ( m_{ \alpha }+m_{\beta }\right ) \left \vert \mathbf{G}_{\alpha \beta } \right \vert ^{2}/2-\mu _{\alpha \beta }\left \vert \mathbf{g}\right \vert ^{2}/2}\left ( \left \vert \mathbf{g}\right \vert + \frac{1}{\left \vert \mathbf{g}\right \vert ^{1-\gamma }}\right ) ^{2}d \mathbf{g}\boldsymbol{\,}d\mathbf{G}_{\alpha \beta } \\ \leq &C\int _{0}^{\infty }R^{2}e^{-R^{2}}dR\int _{0}^{\infty }\left ( \eta ^{2}+\eta ^{\gamma }\right ) ^{2}e^{-\eta ^{2}/4}d\eta \leq C \int _{0}^{\infty }\left ( 1+\eta ^{4}\right ) e^{-\eta ^{2}/4}d\eta =C \text{.} \end{aligned}$$

Hence,

$$ K_{\alpha \beta }^{(1)}=\int _{\mathbb{R}^{3}}k_{\alpha \beta 1}^{ \left ( \beta \right ) }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }) \,h_{\beta \ast }\,d\boldsymbol{\xi }_{\ast } $$

are Hilbert-Schmidt integral operators and as such continuous and compact on $L^{2}\left ( d\boldsymbol{\xi }\right )$, see e.g., Theorem 7.83 in [25], for all $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots,s\right \}$.

Fig. 3

Typical collision of $K_{\alpha \beta }^{(1)}$. Classical representation of a collision between particles of different species

II. Compactness of $K_{\alpha \beta }^{(3)}=\int _{ \mathbb{R}^{3}}k_{\alpha \beta }^{\left ( \alpha \right ) }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\,h_{\alpha \ast }\,d\boldsymbol{\xi }_{ \ast }$ for $\left \{ \alpha ,\beta \right \} \subset \left \{ 1,\ldots,s\right \}$.

Assume that the velocities $\boldsymbol{\xi }$ and $\boldsymbol{\xi }_{\ast }$ are given. Then a collision will be uniquely defined by a vector $\mathbf{w}$ orthogonal to $\mathbf{g}=\boldsymbol{\xi }-\boldsymbol{\xi }\mathbf{_{\ast }}$. This follows, since, by conservation of momentum and kinetic energy (33) (reminding that we here relabeled the velocities), we obtain that $m_{\beta }\mathbf{g}^{\prime }=m_{\beta }\left (\boldsymbol{\xi }_{ \ast}^{\prime }-\boldsymbol{\xi }^{\prime }\right )=m_{\alpha } \mathbf{g}$, while also the equality $\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }^{\prime }\right \vert =\left \vert \boldsymbol{\xi }_{\ast}^{\prime }- \boldsymbol{\xi }_{\ast}\right \vert$ can be obtained, cf. Fig. 4. Indeed, note that - aiming to obtain expressions for $\mathbf{g}^{\prime }$ and $\chi =\left ( \boldsymbol{\xi }_{\ast }^{\prime }-\boldsymbol{\xi } \right ) \cdot \mathbf{n}$ in the arguments of the delta-functions - cf. Fig. 4,

$$\begin{aligned} &W_{\alpha \beta }(\boldsymbol{\xi },\boldsymbol{\xi }^{\prime } \left \vert \boldsymbol{\xi }_{\ast },\boldsymbol{\xi }_{\ast }^{ \prime }\right . ) \\ =&2\left ( m_{\alpha }+m_{\beta }\right ) ^{2}m_{\alpha }m_{\beta } \sigma _{\alpha \beta }\delta _{3}\left ( m_{\alpha }\mathbf{g}+m_{ \beta }\mathbf{g}^{\prime }\right ) \delta _{1}\left ( 2\left \vert \mathbf{g}\right \vert m_{\alpha }\left ( \chi - \frac{m_{\alpha }-m_{\beta }}{2m_{\beta }}\left \vert \mathbf{g} \right \vert \right ) \right ) \\ =& \frac{\left ( m_{\alpha }+m_{\beta }\right ) ^{2}}{\left \vert \mathbf{g}\right \vert m_{\beta }^{2}}\sigma _{\alpha \beta }\,\delta _{3}\! \left ( \frac{m_{\alpha }}{m_{\beta }}\mathbf{g}+\mathbf{g}^{\prime } \right ) \delta _{1}\!\left ( \chi - \frac{m_{\alpha }-m_{\beta }}{2m_{\beta }}\left \vert \mathbf{g} \right \vert \!\right ) \text{,} \end{aligned}$$

where $\mathbf{g}=\boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }$, $\mathbf{g}^{\prime }=\boldsymbol{\xi }^{\prime }-\boldsymbol{\xi }_{\ast }^{ \prime }$, $\chi =\left ( \boldsymbol{\xi }_{\ast }^{\prime }-\boldsymbol{\xi } \right ) \cdot \mathbf{n}$, and $\mathbf{n}= \dfrac{\boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }}{\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }\right \vert }$. Then, by a change of variables $\left \{ \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime }\right \} \rightarrow \left \{ \mathbf{g}^{\prime }=\boldsymbol{\xi }^{\prime }-\boldsymbol{\xi }_{\ast }^{ \prime }, \widetilde{\mathbf{h}}=\boldsymbol{\xi }_{\ast }^{\prime }- \boldsymbol{\xi }\right \}$, noting that

$$ d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime }=d \mathbf{g}^{\prime }d\widetilde{\mathbf{h}}=d\mathbf{g}^{\prime }d\chi d \mathbf{w}\text{, with }\mathbf{w}=\boldsymbol{\xi }_{\ast }^{\prime }- \boldsymbol{\xi }-\chi \mathbf{n}\text{,} $$

the expression (54) of $k_{\alpha \beta }^{\left ( \alpha \right ) }$ may be rewritten in the following way

$$\begin{aligned} k_{\alpha \beta }^{\left ( \alpha \right ) }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }) =&\int _{\left ( \mathbb{R}^{3}\right ) ^{2}} \left ( M_{\beta }^{\prime }M_{\beta \ast }^{\prime }\right ) ^{1/2}W_{ \alpha \beta }(\boldsymbol{\xi },\boldsymbol{\xi }^{\prime }\left \vert \boldsymbol{\xi }_{\ast },\boldsymbol{\xi }_{\ast }^{\prime }\right . )\,d\mathbf{g}^{ \prime }d\widetilde{\mathbf{h}} \\ =&\int _{\left ( \mathbb{R}^{3}\right ) ^{\perp _{\mathbf{n}}}} \frac{\left ( m_{\alpha }+m_{\beta }\right ) ^{2}}{m_{\beta }^{2}} \frac{\left ( M_{\beta }^{\prime }M_{\beta \ast }^{\prime }\right ) ^{1/2}}{\left \vert \mathbf{g}\right \vert }\sigma _{\alpha \beta }\,\left ( \left \vert \widetilde{\mathbf{g}}\right \vert , \frac{\widetilde{\mathbf{g}}\cdot \mathbf{g}_{\ast }}{\left \vert \widetilde{\mathbf{g}}\right \vert \left \vert \mathbf{g}_{\ast }\right \vert } \right ) d\mathbf{w} \end{aligned}$$

where $\left ( \mathbb{R}^{3}\right ) ^{\perp _{\mathbf{n}}}=\left \{ \mathbf{w}\in \mathbb{R}^{3}: \mathbf{w}\perp \mathbf{n}\right \}$, $\widetilde{\mathbf{g}}=\boldsymbol{\xi }-\boldsymbol{\xi }^{\prime }$, and $\mathbf{g}_{\ast }=\boldsymbol{\xi }_{\ast }-\boldsymbol{\xi }_{ \ast }^{\prime }$.

Fig. 4

Typical collision of $K_{\alpha \beta }^{(3)}$

Here, see Fig. 4,

$$ \left \{ \textstyle\begin{array}{l} \boldsymbol{\xi }^{\prime }=\boldsymbol{\xi }_{\ast }+\mathbf{w}- \chi \mathbf{n} \\ \boldsymbol{\xi }_{\ast }^{\prime }=\boldsymbol{\xi }+\mathbf{w}+ \chi \mathbf{n}\end{array}\displaystyle \right . \text{, with }\chi = \frac{m_{\alpha }-m_{\beta }}{2m_{\beta }}\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }\right \vert \text{,} $$

implying that, reminding the notations (29), the following relation for the exponent of the product $M_{\alpha }^{\prime }M_{\beta \ast }^{\prime }$

$$\begin{aligned} \frac{\left \vert \boldsymbol{\xi }^{\prime }\right \vert ^{2}}{2}+ \frac{\left \vert \boldsymbol{\xi }_{\ast }^{\prime }\right \vert ^{2}}{2} =& \left \vert \frac{\boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }}{2}+\mathbf{w} \right \vert ^{2}+ \frac{\left ( \left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }\right \vert +2\chi \right ) ^{2}}{4} \\ =&\left \vert \frac{\left ( \boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }\right ) _{\perp _{\boldsymbol{n}}}}{2}+\mathbf{w}\right \vert ^{2}+\left ( \frac{\left ( \boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }\right ) _{\mathbf{n}}}{2}\right ) ^{2}+ \frac{m_{\alpha }^{2}}{4m_{\beta }^{2}}\left \vert \boldsymbol{\xi }- \boldsymbol{\xi }_{\ast }\right \vert ^{2} \\ =&\left \vert \frac{\left ( \boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }\right ) _{\perp _{\boldsymbol{n}}}}{2}+\mathbf{w}\right \vert ^{2}+ \frac{\left ( \left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}-\left \vert \boldsymbol{\xi }\right \vert ^{2}\right ) ^{2}}{4\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }\right \vert ^{2}}+\frac{m_{\alpha }^{2}}{4m_{\beta }^{2}} \left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }\right \vert ^{2}. \end{aligned}$$

Hence, by assumption (46)

$$\begin{aligned} &\left ( k_{\alpha \beta }^{\left ( \alpha \right ) }( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\right ) ^{2} \\ \leq &\frac{C}{\left \vert \mathbf{g}\right \vert ^{2}}\exp \left ( - \frac{m_{\beta }}{4} \frac{\left ( \left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}-\left \vert \boldsymbol{\xi }\right \vert ^{2}\right ) ^{2}}{\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }\right \vert ^{2}}- \frac{m_{\alpha }^{2}}{4m_{\beta }}\left \vert \boldsymbol{\xi }- \boldsymbol{\xi }_{\ast }\right \vert ^{2}\right ) \\ &\times \left ( \int _{\left ( \mathbb{R}^{3}\right ) ^{\perp _{ \mathbf{n}}}}\left ( 1+ \frac{1}{\left \vert \widetilde{\mathbf{g}}\right \vert ^{2-\gamma }} \right ) \exp \left ( -\frac{m_{\beta }}{2}\left \vert \frac{\left ( \boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }\right ) _{\perp _{\boldsymbol{n}}}}{2}+ \mathbf{w}\right \vert ^{2}\right ) \,d\mathbf{w}\right ) ^{2} \\ \leq &\frac{C}{\left \vert \mathbf{g}\right \vert ^{2}}\exp \left ( - \frac{m_{\beta }}{4} \frac{\left ( \left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}-\left \vert \boldsymbol{\xi }\right \vert ^{2}\right ) ^{2}}{\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }\right \vert ^{2}}- \frac{m_{\alpha }^{2}}{4m_{\beta }}\left \vert \boldsymbol{\xi }- \boldsymbol{\xi }_{\ast }\right \vert ^{2}\right ) \\ =&\frac{C}{\left \vert \mathbf{g}\right \vert ^{2}}\exp \left ( - \frac{m_{\beta }}{4} \frac{\left ( \left \vert \mathbf{g}\right \vert ^{2}+2\mathbf{g}\cdot \boldsymbol{\xi }\right ) ^{2}}{\left \vert \mathbf{g}\right \vert ^{2}}-\frac{m_{\alpha }^{2}}{4m_{\beta }}\left \vert \mathbf{g}\right \vert ^{2}\right ) \\ =&\frac{C}{\left \vert \mathbf{g}\right \vert ^{2}}\exp \left ( -m_{ \beta }\left ( \dfrac{\left \vert \mathbf{g}\right \vert }{2}+\left \vert \boldsymbol{\xi }\right \vert \cos \varphi \right ) ^{2}- \frac{m_{\alpha }^{2}}{4m_{\beta }}\left \vert \mathbf{g}\right \vert ^{2}\right ) \text{, } \end{aligned}$$

(58)

with $\cos \varphi = \dfrac{\mathbf{g}\cdot \boldsymbol{\xi }}{\left \vert \mathbf{g}\right \vert \left \vert \boldsymbol{\xi }\right \vert }$. Here, the second inequality, follows by the following bound, which can be obtained by noting that $\left \vert \widetilde{\mathbf{g}}\right \vert \geq \left \vert \mathbf{w}\right \vert$, cf. Fig. 4, while firstly making a change of variables $\mathbf{w} \rightarrow \widetilde{\mathbf{w}}=\left ( \boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }\right ) _{\perp _{\boldsymbol{n}}}/2+ \mathbf{w}$ followed by one to polar coordinates,

$$\begin{aligned} &\int _{\left ( \mathbb{R}^{3}\right ) ^{\perp _{\mathbf{n}}}}\left ( 1+ \frac{1}{\left \vert \widetilde{\mathbf{g}}\right \vert ^{2-\gamma }}\right ) \exp \left ( -\frac{m_{\beta }}{2}\left \vert \frac{\left ( \boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }\right ) _{\perp _{\boldsymbol{n}}}}{2}+\mathbf{w}\right \vert ^{2}\right ) \,d\mathbf{w} \\ \leq &\int _{\left \vert \mathbf{w}\right \vert \leq 1}1+\left \vert \mathbf{w}\right \vert ^{\gamma -2}\,d\mathbf{w}+2\int _{\left \vert \mathbf{w}\right \vert \geq 1}\exp \left ( -\frac{m_{\beta }}{2}\left \vert \frac{\left ( \boldsymbol{\xi} +\boldsymbol{\xi}_{\ast }\right ) _{\perp _{\boldsymbol{n}}}}{2}+ \mathbf{w}\right \vert ^{2}\right ) \,d\mathbf{w} \\ \leq &\int _{\left \vert \mathbf{w}\right \vert \leq 1}1+\left \vert \mathbf{w}\right \vert ^{\gamma -2}\,d\mathbf{w}+2\int _{\left ( \mathbb{R}^{3} \right ) ^{\perp _{\mathbf{n}}}}e^{-m_{\beta }\left \vert \widetilde{\mathbf{w}}\right \vert ^{2}/2}\,d\widetilde{\mathbf{w}} \\ =&2\pi \left ( \int _{0}^{1}R+R^{\gamma -1}\,dR+2\int _{0}^{\infty }Re^{-m_{ \beta }R^{2}/2}\,dR\right ) =C\text{.} \end{aligned}$$

We obtain that the integral of $\left ( k_{\alpha \beta }^{\left ( \alpha \right ) }\right ) ^{2}$ over the truncated domain $\mathfrak{h}_{N}$ is bounded, by first changing variables $\boldsymbol{\xi }_{\ast }\rightarrow \mathbf{g}=\boldsymbol{\xi }- \boldsymbol{\xi }_{\ast }$, and then to spherical coordinates,

$$\begin{aligned} &\int _{\mathfrak{h}_{N}}\left ( k_{\alpha \beta }^{\left ( \alpha \right ) }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\right ) ^{2}\,d \boldsymbol{\xi \,}d\boldsymbol{\xi }_{\ast }\leq \int _{\mathfrak{h}_{N}} \frac{C}{\left \vert \mathbf{g}\right \vert ^{2}}e^{-m_{\alpha }^{2} \left \vert \mathbf{g}\right \vert ^{2}/\left ( 4m_{\beta }\right ) }d\mathbf{g} \boldsymbol{\,}d\boldsymbol{\xi } \\ =&C\int _{0}^{\infty }e^{-m_{\alpha }^{2}R^{2}/\left ( 4m_{\beta } \right ) }dR\int _{0}^{N}\boldsymbol{\,}\eta ^{2}d\eta =CN^{3} \text{.} \end{aligned}$$

Next we are heading for proving that the integral of $k_{\alpha \beta }^{\left ( \alpha \right ) }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast })$ with respect to $\boldsymbol{\xi }$ over $\mathbb{R}^{3}$ is bounded in $\boldsymbol{\xi }_{\ast }$. Indeed, directly by the bound (58) on $\left ( k_{\alpha \beta }^{\left ( \alpha \right ) }\right ) ^{2}$,

$$ 0\leq k_{\alpha \beta }^{\left ( \alpha \right ) }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\leq \frac{C}{\left \vert \mathbf{g}\right \vert }\exp \left ( -\frac{m_{\beta }}{8}\left ( \left \vert \mathbf{g} \right \vert +2\left \vert \boldsymbol{\xi }\right \vert \cos \varphi \right ) ^{2}-\frac{m_{\alpha }^{2}}{8m_{\beta }}\left \vert \mathbf{g}\right \vert ^{2} \right ) \text{.} $$

(59)

Due to the symmetry $k_{\alpha \beta }^{\left ( \alpha \right ) }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })=k_{\alpha \beta }^{\left ( \alpha \right ) }(\boldsymbol{\xi }_{\ast },\boldsymbol{\xi })$ (55) and bound (59), by changing variables $\boldsymbol{\xi }\rightarrow \mathbf{g}=\boldsymbol{\xi }-\boldsymbol{\xi } \mathbf{_{\ast }}$, and then to spherical coordinates,

$$\begin{aligned} &\int _{\mathbb{R}^{3}}k_{\alpha \beta }^{\left ( \alpha \right ) }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\,d\boldsymbol{\xi }= \int _{\mathbb{R}^{3}}k_{\alpha \beta }^{\left ( \alpha \right ) }( \boldsymbol{\xi }_{\ast },\boldsymbol{\xi })\,d\boldsymbol{\xi } \\ \leq &\int _{\mathbb{R}^{3}} \frac{C}{\left \vert \mathbf{g}\right \vert }\exp \left ( - \frac{m_{\alpha }^{2}}{8m_{\beta }}\left \vert \mathbf{g}\right \vert ^{2}\right ) \,d\mathbf{g}=\int _{0}^{\infty }Re^{-m_{\alpha }^{2}R^{2}/ \left ( 8m_{\beta }\right ) }dR=C\text{.} \end{aligned}$$

Furthermore, heading for proving the uniform convergence of the integral of $k_{\alpha \beta }^{\left ( \alpha \right ) }$ with respect to $\boldsymbol{\xi }_{\ast }$ over the truncated domain $\mathfrak{h}_{N}$ to the one over all of $\mathbb{R}^{3}$, the following bound on the integral over $\mathbb{R}^{3}$ can be obtained for $\left \vert \boldsymbol{\xi }\right \vert \neq 0$ by bound (59), by changing to (conventional) spherical coordinates, with $\boldsymbol{\xi }$ as zenithal direction, and hence, $\varphi$ as polar angle, followed by the change of variables $\varphi \rightarrow \eta =R+2\left \vert \boldsymbol{\xi }\right \vert \cos \varphi$, with $d\eta =-2\left \vert \boldsymbol{\xi }\right \vert \sin \varphi \,d \varphi$,

$$\begin{aligned} &\int _{\mathbb{R}^{3}}k_{\alpha \beta }^{\left ( \alpha \right ) }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\,d\boldsymbol{\xi }_{ \ast } \\ \leq &\int _{\mathbb{R}^{3}} \frac{C}{\left \vert \mathbf{g}\right \vert }\exp \left ( - \frac{m_{\beta }}{8}\left ( \left \vert \mathbf{g}\right \vert +2 \left \vert \boldsymbol{\xi }\right \vert \cos \varphi \right ) ^{2}- \frac{m_{\alpha }^{2}}{8m_{\beta }}\left \vert \mathbf{g}\right \vert ^{2} \right ) \,d\mathbf{g} \\ =&C\int _{0}^{\infty }\int _{0}^{\pi }R\exp \left ( - \frac{m_{\beta }}{8}\left ( R+2\left \vert \boldsymbol{\xi }\right \vert \cos \varphi \right ) ^{2}-\frac{m_{\alpha }^{2}}{8m_{\beta }}R^{2}\right ) \sin \varphi \,d \varphi \boldsymbol{\,}dR \\ =&\frac{C}{\left \vert \boldsymbol{\xi }\right \vert }\int _{0}^{ \infty }\int _{R-2\left \vert \boldsymbol{\xi }\right \vert }^{R+2 \left \vert \boldsymbol{\xi }\right \vert }Re^{-m_{\beta }\eta ^{2}/8}e^{-m_{ \alpha }^{2}R^{2}/\left ( 8m_{\beta }\right ) }d\eta \boldsymbol{\,}dR \\ \leq &\frac{C}{\left \vert \boldsymbol{\xi }\right \vert }\int _{0}^{ \infty }Re^{-m_{\alpha }^{2}R^{2}/\left ( 8m_{\beta }\right ) }\,dR \int _{-\infty }^{\infty }e^{-m_{\beta }\eta ^{2}/8}d\eta = \frac{C}{\left \vert \boldsymbol{\xi }\right \vert }\text{.} \end{aligned}$$

(60)

By bounds (59) and (60), and a change to spherical coordinates

$$\begin{aligned} &\sup _{\boldsymbol{\xi }\in \mathbb{R}^{3}}\int _{\mathbb{R}^{3}}k_{ \alpha \beta }^{\left ( \alpha \right ) }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast })-k_{\alpha \beta }^{\left ( \alpha \right ) }( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\mathbf{1}_{\mathfrak{h}_{N}}\,d\boldsymbol{\xi }_{ \ast } \\ \leq &\sup _{\boldsymbol{\xi }\in \mathbb{R}^{3}}\int _{\left \vert \mathbf{g}\right \vert \leq \frac{1}{N}}k_{\alpha \beta }^{\left ( \alpha \right ) }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\,d\boldsymbol{\xi }_{ \ast }+\sup _{\left \vert \boldsymbol{\xi }\right \vert \geq N}\int _{ \mathbb{R}^{3}}k_{\alpha \beta }^{\left ( \alpha \right ) }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\,d\boldsymbol{\xi }_{\ast } \\ \leq &\int _{\left \vert \mathbf{g}\right \vert \leq \frac{1}{N}} \frac{C}{\left \vert \mathbf{g}\right \vert }\,d\mathbf{g}+\frac{C}{N}\leq C \left ( \int _{0}^{\frac{1}{N}}R\,dR+\frac{1}{N}\right ) \\ =&C\left ( \frac{1}{N^{2}}+\frac{1}{N}\right ) \rightarrow 0 \text{ as }N\rightarrow \infty \text{.} \end{aligned}$$

Hence, by Lemma 4 the operators

$$ K_{\alpha \beta }^{(3)}=\int _{\mathbb{R}^{3}}k_{\alpha \beta }^{ \left ( \alpha \right ) }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }) \,h_{\alpha \ast }\,d\boldsymbol{\xi }_{\ast } $$

are compact on $L^{2}\left ( d\boldsymbol{\xi }\right )$ for all $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots,s\right \}$.

III. Compactness of $K_{\alpha \beta }^{(2)}=\int _{ \mathbb{R}^{3}}k_{\alpha \beta 2}^{\left ( \beta \right ) }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\,h_{\beta \ast }\,d\boldsymbol{\xi }_{ \ast }$ for $\left \{ \alpha ,\beta \right \} \subset \left \{ 1,\ldots,s\right \}$.

First assume that $m_{\alpha }\neq m_{\beta }$.

Assume that the velocities $\boldsymbol{\xi }$ and $\boldsymbol{\xi }_{\ast }$ are given. Then a collision will be uniquely defined by a unit vector $\boldsymbol{\eta }=\left (\boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }^{ \prime }\right )/\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{ \ast }^{\prime }\right \vert$, or, $\boldsymbol{\omega }=\left (\boldsymbol{\xi }^{\prime }- \boldsymbol{\xi }_{\ast }^{\prime }\right )/\left \vert \boldsymbol{\xi }^{\prime }-\boldsymbol{\xi }_{\ast }^{\prime } \right \vert$. This follows, since, by conservation of momentum and kinetic energy (33) (reminding that we relabeled the velocities), the equality $\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }^{\prime }\right \vert =\left \vert \boldsymbol{\xi }_{\ast}^{\prime }- \boldsymbol{\xi }_{\ast}\right \vert$ (or, equivalently, $\left \vert \boldsymbol{\xi }^{\prime }-\boldsymbol{\xi }_{\ast}^{ \prime }\right \vert =\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{ \ast}\right \vert$) can be obtained, while also $m_{\beta }\left (\boldsymbol{\xi }_{\ast}-\boldsymbol{\xi }^{\prime } \right )=m_{\alpha }\left (\boldsymbol{\xi }-\boldsymbol{\xi }_{\ast}^{ \prime }\right )$, cf. Fig. 5. Note that, knowing $\boldsymbol{\eta }$ will give us $\mathbf{b}=\left (m_{\alpha }-m_{\beta }\right )\left ( \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast}^{\prime }\right )/\left (2m_{ \beta }\right )$, cf. Fig. 5. Indeed, noting that - with the aim to obtain expressions for $\left \vert \mathbf{g}^{\prime }\right \vert$ and $\mathbf{g}_{\alpha \beta }^{\prime }= \dfrac{m_{\alpha }\boldsymbol{\xi }_{\ast }^{\prime }-m_{\beta }\boldsymbol{\xi }^{\prime }}{m_{\alpha }-m_{\beta }}$ in the arguments of the delta-functions,

$$\begin{aligned} W_{\alpha \beta }\left ( \boldsymbol{\xi },\boldsymbol{\xi }^{\prime } \left \vert \boldsymbol{\xi }_{\ast }^{\prime },\boldsymbol{\xi }_{ \ast }\right . \right ) =&2\left ( m_{\alpha }+m_{\beta }\right ) ^{2}m_{ \alpha }m_{\beta }\sigma _{\alpha \beta }\delta _{1}\left ( \frac{m_{\alpha }m_{\beta }}{m_{\alpha }-m_{\beta }}\left ( \left \vert \mathbf{g}\right \vert ^{2}-\left \vert \mathbf{g}^{\prime } \right \vert ^{2}\right ) \right ) \\ &\times \delta _{3}\left ( \left ( m_{\alpha }-m_{\beta }\right ) \left ( \mathbf{g}_{\alpha \beta }-\mathbf{g}_{\alpha \beta }^{ \prime }\right ) \right ) \\ =& \frac{\left ( m_{\alpha }+m_{\beta }\right ) ^{2}}{\left ( m_{\alpha }-m_{\beta }\right ) ^{2}} \frac{\sigma _{\alpha \beta }}{\left \vert \mathbf{g}\right \vert }\delta _{1}\left ( \left \vert \mathbf{g}\right \vert - \left \vert \mathbf{g}^{\prime }\right \vert \right ) \delta _{3} \left ( \mathbf{g}_{\alpha \beta }-\mathbf{g}_{\alpha \beta }^{ \prime }\right ) \text{, with} \\ &\mathbf{g}_{\alpha \beta }= \dfrac{m_{\alpha }\boldsymbol{\xi }-m_{\beta }\boldsymbol{\xi }_{\ast }}{m_{\alpha }-m_{\beta }}\text{ and } \mathbf{g}_{\alpha \beta }^{\prime }= \dfrac{m_{\alpha }\boldsymbol{\xi }_{\ast }^{\prime }-m_{\beta }\boldsymbol{\xi }^{\prime }}{m_{\alpha }-m_{\beta }}\text{,} \end{aligned}$$

by first changing variables $\left \{ \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{\prime }\right \} \rightarrow \left \{ \!\mathbf{g}^{ \prime }=\boldsymbol{\xi }^{\prime }-\boldsymbol{\xi }_{\ast }^{\prime }, \mathbf{g}_{\alpha \beta }^{\prime }= \dfrac{m_{\alpha }\boldsymbol{\xi }_{\ast }^{\prime }-m_{\beta }\boldsymbol{\xi }^{\prime }}{m_{\alpha }-m_{\beta }}\!\right \}$, and then to spherical coordinates, where

$$ d\boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime }=d \mathbf{g}^{\prime }d\mathbf{g}_{\alpha \beta }^{\prime }=\left \vert \mathbf{g}^{\prime }\right \vert ^{2}d\left \vert \mathbf{g}^{\prime }\right \vert d\mathbf{g}_{\alpha \beta }^{\prime }d\boldsymbol{\omega }, \text{ with } \boldsymbol{\omega }= \frac{\mathbf{g}^{\prime }}{\left \vert \mathbf{g}^{\prime }\right \vert }\text{,} $$

the expression (54) of $k_{\alpha \beta 2}^{\left ( \beta \right ) }$ may be transformed to

$$\begin{aligned} k_{\alpha \beta 2}^{\left ( \beta \right ) }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }) =&\int _{\mathbb{R}^{3}\times \mathbb{R}_{+} \times \mathbb{S}^{2}}\left ( M_{\beta }^{\prime }M_{\alpha \ast }^{ \prime }\right ) ^{1/2}W_{\alpha \beta }\left ( \boldsymbol{\xi }, \boldsymbol{\xi }^{\prime }\left \vert \boldsymbol{\xi }_{\ast }^{\prime },\boldsymbol{\xi }_{\ast }\right . \right )\left \vert \mathbf{g}^{\prime }\right \vert ^{2} \,d \mathbf{g}_{\alpha \beta }^{\prime }d\left \vert \mathbf{g}^{\prime }\right \vert d\boldsymbol{\omega } \\ =& \frac{\left ( m_{\alpha }+m_{\beta }\right ) ^{2}}{\left ( m_{\alpha }-m_{\beta }\right ) ^{2}} \int _{\mathbb{S}^{2}}\left ( M_{\beta }^{\prime }M_{\alpha \ast }^{ \prime }\right ) ^{1/2}\left \vert \mathbf{g}\right \vert \sigma _{ \alpha \beta }\,\left ( \left \vert \widetilde{\mathbf{g}}\right \vert ,- \frac{\widetilde{\mathbf{g}}\cdot \mathbf{g}_{\ast }}{\left \vert \widetilde{\mathbf{g}}\right \vert \left \vert \mathbf{g}_{\ast }\right \vert }\right ) \,d\boldsymbol{\omega }\text{,} \\ \text{with} &\mathbf{g}=\boldsymbol{\xi }-\boldsymbol{\xi }_{\ast } \text{, }\mathbf{g}^{\prime }=\boldsymbol{\xi }^{\prime }-\boldsymbol{\xi }_{ \ast }^{\prime }\text{, }\widetilde{\mathbf{g}}=\boldsymbol{\xi }- \boldsymbol{\xi }^{\prime }\text{ and }\mathbf{g}_{\ast }= \boldsymbol{\xi }_{\ast }-\boldsymbol{\xi }_{\ast }^{\prime }\text{.} \end{aligned}$$

Here, see Fig. 5,

$$ \left \{ \textstyle\begin{array}{l} \boldsymbol{\xi }^{\prime }=\boldsymbol{\xi }_{\ast }- \dfrac{m_{\alpha }}{m_{\beta }}\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }^{ \prime }\right \vert \boldsymbol{\eta } \\ \boldsymbol{\xi }_{\ast }^{\prime }=\boldsymbol{\xi }-\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }^{\prime }\right \vert \boldsymbol{\eta }\end{array}\displaystyle \right . \text{, }\boldsymbol{\eta }= \frac{\boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }^{\prime }}{\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }^{\prime }\right \vert } \in \mathbb{S}^{2}\text{.} $$

Then, by Lemma 3, since relation (53) follows by energy conservation, we have the following relation between the exponents of the products $\left (M_{\alpha }^{\prime }M_{\beta \ast }^{\prime }\right )^{2}$ and $\left (M_{\alpha }M_{\beta \ast }\right )^{2}$, respectively,

$$ m_{\beta }\left \vert \boldsymbol{\xi }^{\prime }\right \vert ^{2}+m_{ \alpha }\left \vert \boldsymbol{\xi }_{\ast }^{\prime }\right \vert ^{2} \geq \rho \left ( m_{\alpha }\left \vert \boldsymbol{\xi }\right \vert ^{2}+m_{\beta }\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}\right ) , $$

for some positive number $\rho$, $0<\rho <1$. Hence, by assumption (46), noticing that $\left \vert \mathbf{g}\right \vert \leq \left \vert \widetilde{\mathbf{g}}\right \vert$, cf. Fig. 5, the bound

$$\begin{aligned} \left ( k_{\alpha \beta 2}^{\left ( \beta \right ) }( \boldsymbol{\xi },\boldsymbol{\xi }_{\ast })\right ) ^{2} \leq &C\left ( M_{\alpha }M_{ \beta \ast }\right ) ^{\rho }\left ( \int _{\mathbb{S}^{2}}\left ( 1+ \frac{1}{\left \vert \widetilde{\mathbf{g}}\right \vert ^{2-\gamma }}\right ) \left \vert \mathbf{g}\right \vert \,d\boldsymbol{\omega }\right ) ^{2} \\ \leq &CM_{\alpha }^{\rho }M_{\beta \ast }^{\rho }\left ( 1+ \frac{1}{\left \vert \mathbf{g}\right \vert ^{2-\gamma }}\right ) ^{2}\left \vert \mathbf{g}\right \vert ^{2}\left ( \int _{\mathbb{S}^{2}}\,d \boldsymbol{\omega }\right ) ^{2} \\ =&CM_{\alpha }^{\rho }M_{\beta \ast }^{\rho }\left ( \left \vert \mathbf{g}\right \vert + \frac{1}{\left \vert \mathbf{g}\right \vert ^{1-\gamma }}\right ) ^{2} \end{aligned}$$

(61)

may be obtained. Then, by applying bound (61) combined with firstly changing variables $\left \{ \boldsymbol{\xi },\boldsymbol{\xi }_{\ast }\right \} \rightarrow \left \{ \mathbf{g},\mathbf{G}_{\alpha \beta }\right \}$, with unitary Jacobian, and then to spherical coordinates,

$$\begin{aligned} &\int _{\left ( \mathbb{R}^{3}\right ) ^{2}}\left ( k_{\alpha \beta 2}^{ \left ( \beta \right ) }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }) \right ) ^{2}d\boldsymbol{\xi \,}d\boldsymbol{\xi }_{\ast } \\ \leq &C\int _{\left ( \mathbb{R}^{3}\right ) ^{2}}e^{-\rho \left ( m_{ \alpha }+m_{\beta }\right ) \left \vert \mathbf{G}_{\alpha \beta } \right \vert ^{2}/2-\mu _{\alpha \beta }\rho \left \vert \mathbf{g} \right \vert ^{2}/2}\left ( \left \vert \mathbf{g}\right \vert + \frac{1}{\left \vert \mathbf{g}\right \vert ^{1-\gamma }}\right ) ^{2}d\mathbf{g}\boldsymbol{\,}d \mathbf{G}_{\alpha \beta } \\ \leq &C\int _{0}^{\infty }R^{2}e^{-R^{2}}dR\int _{0}^{\infty }\left ( r^{2}+r^{\gamma }\right ) ^{2}e^{-r^{2}/4}dr \\ \leq &C\int _{0}^{\infty }R^{2}e^{-R^{2}}dR\int _{0}^{\infty }\left ( 1+r^{4}\right ) e^{-r^{2}/4}dr=C\text{.} \end{aligned}$$

Hence,

$$ K_{\alpha \beta }^{(2)}=\int _{\mathbb{R}^{3}}k_{\alpha \beta 2}^{ \left ( \beta \right ) }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }) \,h_{\beta \ast }\,d\boldsymbol{\xi }_{\ast } $$

are Hilbert-Schmidt integral operators and as such also continuous and compact on $L^{2}\left ( d\boldsymbol{\xi }\right )$ [25, Theorem 7.83] for all $\left \{ \alpha ,\beta \right \} \subseteq \left \{ 1,\ldots,s\right \}$.

Fig. 5

Typical collision of $K_{\alpha \beta }^{(2)}$

On the other hand, if $m_{\alpha }=m_{\beta }$, then

$$\begin{aligned} k_{\alpha \beta 2}^{\left ( \beta \right ) }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast }) =&\int _{\left ( \mathbb{R}^{3}\right ) ^{ \perp _{\mathbf{n}}}}4\left ( M_{\beta }^{\prime }M_{\alpha \ast }^{\prime }\right ) ^{1/2} \frac{\sigma _{\alpha \beta }}{\left \vert \mathbf{g}\right \vert }\,\left ( \left \vert \widetilde{\mathbf{g}}\right \vert ,- \frac{\widetilde{\mathbf{g}}\cdot \mathbf{g}_{\ast }}{\left \vert \widetilde{\mathbf{g}}\right \vert \left \vert \mathbf{g}_{\ast }\right \vert } \right ) \boldsymbol{\,}d\mathbf{w}\text{,} \\ &\text{with }\widetilde{\mathbf{g}}=\boldsymbol{\xi }- \boldsymbol{\xi }^{\prime }\text{ and }\mathbf{g}_{\ast }=\boldsymbol{\xi }_{\ast }-\boldsymbol{\xi }_{\ast }^{\prime }\text{.} \end{aligned}$$

Here

$$ \left \{ \textstyle\begin{array}{l} \boldsymbol{\xi }^{\prime }=\boldsymbol{\xi }_{\ast }+\mathbf{w} \\ \boldsymbol{\xi }_{\ast }^{\prime }=\boldsymbol{\xi }+\mathbf{w}\end{array}\displaystyle \right . \text{, with }\mathbf{w\perp g}\text{ and }\mathbf{g}= \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }\text{.} $$

Then similar arguments to the ones for $k_{\alpha \beta }^{\left ( \alpha \right ) }(\boldsymbol{\xi }, \boldsymbol{\xi }_{\ast })$ (with $m_{\alpha }=m_{\beta }$) above, can be applied.

Concluding, the operator

$$ K=(K_{1},\ldots,K_{s})=\sum _{\beta =1}^{s}\left ( (K_{1\beta }^{(3)},\ldots,K_{s \beta }^{(3)})-(K_{1\beta }^{(1)},\ldots,K_{s\beta }^{(1)})+(K_{1\beta }^{(2)},\ldots,K_{s \beta }^{(2)})\right ) $$

is a compact self-adjoint operator on $\left ( L^{2}\left ( d\boldsymbol{\xi }\right ) \right ) ^{s}$. Self-adjointness is due to the symmetry relations (55), (56), cf. [27, p. 198]. □

3.3.2 Bounds on the Collision Frequency

This section concerns the proof of Theorem 4, which is essentially a mimicking of the corresponding proof for monatomic single species.

Proof

For a hard sphere model $\sigma _{\alpha \beta }=C_{\alpha \beta }$ for some positive constant $C_{\alpha \beta }$ for any indices $\left \{ \alpha ,\beta \right \} \subset \left \{ 1,\ldots,s\right \}$. Then each collision frequency $\nu _{\alpha }$, with $\alpha \in \left \{ 1,\ldots,s\right \}$, by the change of variables $\left \{ \boldsymbol{\xi }^{\prime },\boldsymbol{\xi }_{\ast }^{ \prime }\right \} \rightarrow \left \{ \left \vert \mathbf{g}^{ \prime }\right \vert ,\boldsymbol{\omega }= \dfrac{\mathbf{g}^{\prime }}{\left \vert \mathbf{g}^{\prime }\right \vert }, \mathbf{G}_{\alpha \beta }^{\prime }=\dfrac{m_{\alpha }\boldsymbol{\xi }^{\prime }+m_{\beta }\boldsymbol{\xi }_{\ast }^{\prime }}{m_{\alpha }+m_{\beta }}\right \}$, can be rewritten as

$$\begin{aligned} \nu _{\alpha } =&\sum _{\beta =1}^{s}\int _{\left ( \mathbb{R}^{3}\right ) ^{3}}M_{\beta \ast }W_{\alpha \beta }(\boldsymbol{\xi },\boldsymbol{\xi }_{\ast }\left \vert \boldsymbol{\xi }^{\prime }, \boldsymbol{\xi }_{\ast }^{\prime }\right . )\,d\boldsymbol{\xi }_{\ast }d \boldsymbol{\xi }^{\prime }d\boldsymbol{\xi }_{\ast }^{\prime } \\ =&\sum _{\beta =1}^{s}\int _{\left ( \mathbb{R}^{3}\right ) ^{2} \times \mathbb{R}_{+}\times \mathbb{S}^{2}}M_{\beta \ast } \frac{C_{\alpha \beta }}{\left \vert \mathbf{g}\right \vert }\left \vert \mathbf{g}^{\prime }\right \vert ^{2}\delta _{3}\left ( \mathbf{G}_{\alpha \beta }- \mathbf{G}_{\alpha \beta }^{\prime }\right ) \delta _{1}\left ( \left \vert \mathbf{g}\right \vert -\left \vert \mathbf{g}^{\prime } \right \vert \right ) \,d\boldsymbol{\xi }_{\ast }d\mathbf{G}^{\prime }d\left \vert \mathbf{g}^{\prime }\right \vert \,d\boldsymbol{\omega } \\ =&4\pi \sum _{\beta =1}^{s}C_{\alpha \beta }\int _{ \mathbb{R}^{3}}M_{\beta \ast }\left \vert \mathbf{g}\right \vert \,d \boldsymbol{\xi }_{\ast } \\ =&\sum _{\beta =1}^{s}n_{\beta }m_{\beta }\sqrt{ \frac{2m_{\beta }}{\pi }}C_{\alpha \beta }\int _{\mathbb{R}^{3}}e^{-m_{\beta }\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}/2}\left \vert \boldsymbol{\xi }_{\ast }-\boldsymbol{\xi }\right \vert \,d\boldsymbol{\xi }_{\ast } \text{.} \end{aligned}$$

First, aiming to prove the lower bound of Theorem 4, consider the two different cases $\left \vert \boldsymbol{\xi }\right \vert \leq 1$ and $\left \vert \boldsymbol{\xi }\right \vert \geq 1$ separately. For any $\alpha \in \left \{ 1,\ldots,s\right \}$, if $\left \vert \boldsymbol{\xi }\right \vert \geq 1$, then by a trivial estimate

$$\begin{aligned} \nu _{\alpha } \geq &C\sum _{\beta =1}^{s}\int _{ \mathbb{R}^{3}}e^{-m_{\beta }\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}} \left \vert \left \vert \boldsymbol{\xi }\right \vert -\left \vert \boldsymbol{\xi }_{\ast }\right \vert \right \vert \,d \boldsymbol{\xi }_{\ast } \\ \geq &C\sum _{\beta =1}^{s}\int _{\left \vert \boldsymbol{\xi }_{\ast }\right \vert \leq 1/2}e^{-m_{\beta }\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}}\left ( \left \vert \boldsymbol{\xi }\right \vert -\left \vert \boldsymbol{\xi }_{\ast } \right \vert \right ) \,d\boldsymbol{\xi }_{\ast } \\ \geq &C\sum _{\beta =1}^{s}e^{-m_{\beta }/4} \frac{\left \vert \boldsymbol{\xi }\right \vert }{2}\int _{\left \vert \boldsymbol{\xi }_{\ast }\right \vert \leq 1/2}\,d \boldsymbol{\xi }_{\ast }\geq C\left \vert \boldsymbol{\xi }\right \vert \geq C\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right ) \text{,} \end{aligned}$$

while, if $\left \vert \boldsymbol{\xi }\right \vert \leq 1$, then, by trivial estimates and a change to spherical coordinates,

$$\begin{aligned} \nu _{\alpha } \geq &C\sum _{\beta =1}^{s}\int _{ \mathbb{R}^{3}}e^{-m_{\beta }\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}} \left \vert \left \vert \boldsymbol{\xi }\right \vert -\left \vert \boldsymbol{\xi }_{\ast }\right \vert \right \vert \,d \boldsymbol{\xi }_{\ast } \\ \geq &C\sum _{\beta =1}^{s}\int _{\left \vert \boldsymbol{\xi }_{\ast }\right \vert \geq 2}e^{-m_{\beta }\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}}\left ( \left \vert \boldsymbol{\xi }_{\ast }\right \vert -\left \vert \boldsymbol{\xi } \right \vert \right ) \,d\boldsymbol{\xi }_{\ast } \\ \geq &C\sum _{\beta =1}^{s}\int _{\left \vert \boldsymbol{\xi }_{\ast }\right \vert \geq 2}e^{-m_{\beta }\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}} \frac{\left \vert \boldsymbol{\xi }_{\ast }\right \vert }{2}\,d\boldsymbol{\xi }_{\ast } \\ =&C\sum _{\beta =1}^{s}\int _{2}^{\infty }R^{3}e^{-m_{\beta }R^{2}} \,dR=C\geq C\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right ) \text{.} \end{aligned}$$

Hence, there is a positive constant $\nu _{-}>0$, such that $\nu _{\alpha }\geq \nu _{-}\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right )$ for all $\alpha \in \left \{ 1,\ldots,s\right \}$ and $\boldsymbol{\xi }\in \mathbb{R}^{3}$.

On the other hand, regarding the upper bound, for any $\alpha \in \left \{ 1,\ldots,s\right \}$, by a change to spherical coordinates,

$$\begin{aligned} \nu _{\alpha } \leq &C\sum _{\beta =1}^{s}\int _{ \mathbb{R}^{3}}e^{-m_{\beta }\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}} \left ( \left \vert \boldsymbol{\xi }\right \vert +\left \vert \boldsymbol{\xi }_{\ast }\right \vert \right ) \,d\boldsymbol{\xi }_{\ast } \\ =&C\sum _{\beta =1}^{s}\int _{0}^{\infty }\left \vert \boldsymbol{\xi }\right \vert R^{2}e^{-m_{\beta }R^{2}}+R^{3}e^{-m_{\beta }R^{2}}\,dR \leq C\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right ) \text{.} \end{aligned}$$

Hence, there is a positive constant $\nu _{+}>0$, such that $\nu _{\alpha }\leq \nu _{+}\left ( 1+\left \vert \boldsymbol{\xi }\right \vert \right )$ for all $\alpha \in \left \{ 1,\ldots,s\right \}$ and $\boldsymbol{\xi }\in \mathbb{R}^{3}$, and the theorem follows. □

Appendix: Proof of Lemma 8

This appendix concerns an alternative, to the one presented in [10], proof of Lemma 8.

Proof

Denote $q:=\left \vert \boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }^{\prime } \right \vert$. For $\boldsymbol{\eta }=\left (\boldsymbol{\xi }-\boldsymbol{\xi }_{\ast }^{ \prime }\right ) /q$ the following (unique) decompositions can be made, by the relations (52), cf. Fig. 5,

$$ \left \{ \textstyle\begin{array}{l} \boldsymbol{\xi }=\mathbf{w}+r\boldsymbol{\eta } \\ \boldsymbol{\xi }_{\ast }^{\prime }=\mathbf{w}+\left ( r-q\right ) \boldsymbol{\eta }\end{array}\displaystyle \right . \text{, with }\mathbf{w}\perp \boldsymbol{\eta }, $$

while

$$ \left \{ \textstyle\begin{array}{l} \boldsymbol{\xi }^{\prime }=\widetilde{\mathbf{w}}+\left ( r_{\ast }- \dfrac{m_{\alpha }}{m_{\beta }}q\right ) \boldsymbol{\eta } \\ \boldsymbol{\xi }_{\ast }=\widetilde{\mathbf{w}}+r_{\ast } \boldsymbol{\eta }\end{array}\displaystyle \right . \text{, with }\widetilde{\mathbf{w}}\perp \boldsymbol{\eta } \text{.} $$

It follows by relation (53) that

$$ r_{\ast }=r+\frac{m_{\alpha }-m_{\beta }}{2m_{\beta }}q\text{,} $$

and hence,

$$ \left \{ \textstyle\begin{array}{l} \boldsymbol{\xi }^{\prime }=\widetilde{\mathbf{w}}+\left ( r- \dfrac{m_{\alpha }+m_{\beta }}{2m_{\beta }}q\right ) \boldsymbol{\eta } \\ \boldsymbol{\xi }_{\ast }=\widetilde{\mathbf{w}}+\left ( r+ \dfrac{m_{\alpha }-m_{\beta }}{2m_{\beta }}q\right ) \boldsymbol{\eta }\end{array}\displaystyle \right . \text{, with }\widetilde{\mathbf{w}}\perp \boldsymbol{\eta } \text{. } $$

Then

$$\begin{aligned} &m_{\beta }\left \vert \boldsymbol{\xi }^{\prime }\right \vert ^{2}+m_{ \alpha }\left \vert \boldsymbol{\xi }_{\ast }^{\prime }\right \vert ^{2} \\ =&m_{\alpha }\left \vert \mathbf{w}\right \vert ^{2}+m_{\beta } \left \vert \widetilde{\mathbf{w}}\right \vert ^{2}+\left ( m_{ \alpha }+m_{\beta }\right ) r^{2}-\left ( 3m_{\alpha }+m_{\beta } \right ) qr + \frac{m_{\alpha }^{2}+6m_{\alpha }m_{\beta }+m_{\beta }^{2}}{4m_{\beta }}q^{2} \\ =&m_{\alpha }\left \vert \mathbf{w}\right \vert ^{2}+m_{\beta } \left \vert \widetilde{\mathbf{w}}\right \vert ^{2}+\left ( m_{ \alpha }+m_{\beta }\right ) \left ( r- \frac{3m_{\alpha }+m_{\beta }}{2\left ( m_{\alpha }+m_{\beta }\right ) }q \right ) ^{2} + \frac{\left ( m_{\alpha }-m_{\beta }\right ) ^{2}}{m_{\alpha }+m_{\beta }}\frac{m_{\alpha }}{4m_{\beta }}q^{2}\text{,} \end{aligned}$$

while

$$\begin{aligned} &m_{\alpha }\left \vert \boldsymbol{\xi }\right \vert ^{2}+m_{\beta } \left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2} \\ =&m_{\alpha }\left \vert \mathbf{w}\right \vert ^{2}+m_{\beta } \left \vert \widetilde{\mathbf{w}}\right \vert ^{2}+\left ( m_{ \alpha }+m_{\beta }\right ) r^{2}+\left ( m_{\alpha }-m_{\beta } \right ) qr+ \frac{\left ( m_{\alpha }-m_{\beta }\right ) ^{2}}{4m_{\beta }}q^{2} \\ =&m_{\alpha }\left \vert \mathbf{w}\right \vert ^{2}+m_{\beta } \left \vert \widetilde{\mathbf{w}}\right \vert ^{2}+\left ( m_{ \alpha }+m_{\beta }\right ) \left ( r+ \frac{m_{\alpha }-m_{\beta }}{2\left ( m_{\alpha }+m_{\beta }\right ) }q\right ) ^{2} + \frac{\left ( m_{\alpha }-m_{\beta }\right ) ^{2}}{m_{\alpha }+m_{\beta }}\frac{m_{\alpha }}{4m_{\beta }}q^{2}\text{.} \end{aligned}$$

Denote

$$ a:=- \dfrac{3m_{\alpha }+m_{\beta }}{2\left ( m_{\alpha }+m_{\beta }\right ) }q\text{, }b:= \dfrac{m_{\alpha }-m_{\beta }}{2\left ( m_{\alpha }+m_{\beta }\right ) }q \text{, and }c^{2}:= \dfrac{\left ( m_{\alpha }-m_{\beta }\right ) ^{2}}{4\left ( m_{\alpha }+m_{\beta }\right ) ^{2}} \dfrac{m_{\alpha }}{m_{\beta }}q^{2}. $$

Then

$$\begin{aligned} & \frac{m_{\beta }\left \vert \boldsymbol{\xi }^{\prime }\right \vert ^{2}+m_{\alpha }\left \vert \boldsymbol{\xi }_{\ast }^{\prime }\right \vert ^{2}-\rho \left ( m_{\alpha }\left \vert \boldsymbol{\xi }\right \vert ^{2}+m_{\beta }\left \vert \boldsymbol{\xi }_{\ast }\right \vert ^{2}\right ) }{m_{\alpha }+m_{\beta }} \\ \geq & \left ( r+a\right ) ^{2}-\rho \left ( r+b\right ) ^{2}+\left ( 1- \rho \right ) c^{2} \\ =& \left ( 1-\rho \right ) \left ( r^{2}+2\frac{a-\rho b}{1-\rho }r+ \frac{a^{2}-\rho b^{2}}{1-\rho }+c^{2}\right ) \\ =& \left ( 1-\rho \right ) \left ( r+\frac{a-\rho b}{1-\rho }\right ) ^{2}+ \frac{c^{2}}{1-\rho }\left ( \rho ^{2}-2\rho \left ( 1+ \frac{\left ( a-b\right ) ^{2}}{2c^{2}}\right ) +1\right ) \\ \geq & \frac{c^{2}}{1-\rho }\left ( \rho ^{2}-2\rho \left ( 1+ \frac{\left ( a-b\right ) ^{2}}{2c^{2}}\right ) +1\right ) \geq 0 \\ & \text{if }0\leq \rho \leq 1+\frac{\left ( a-b\right ) ^{2}}{2c^{2}}- \frac{1}{2c^{2}}\sqrt{\left ( a-b\right ) ^{4}+4c^{2}\left ( a-b\right ) ^{2}}< 1 \text{.} \end{aligned}$$

Let

$$\begin{aligned} \rho =&1+\frac{\left ( a-b\right ) ^{2}}{2d^{2}}-\frac{1}{2c^{2}} \sqrt{\left ( a-b\right ) ^{4}+4d^{2}\left ( a-b\right ) ^{2}} \\ =&1+ \frac{1-\sqrt{1+\dfrac{4d^{2}}{\left ( a-b\right ) ^{2}}}}{\dfrac{2d^{2}}{\left ( a-b\right ) ^{2}}}=1- \frac{2}{1+\sqrt{1+\dfrac{4d^{2}}{\left ( a-b\right ) ^{2}}}} \\ =&1- \frac{2}{1+\sqrt{1+\dfrac{\left ( m_{\alpha }-m_{\beta }\right ) ^{2}}{4m_{\alpha }m_{\beta }}}}=\left ( \frac{\sqrt{m_{\alpha }}-\sqrt{m_{\beta }}}{\sqrt{m_{\alpha }}+\sqrt{m_{\beta }}}\right ) ^{2}>0\text{ if }m_{ \alpha }\neq m_{\beta }\text{.} \end{aligned}$$

 □