Stretch and stress distributions in the human artery based on two-layer model considering residual stresses

Abstract

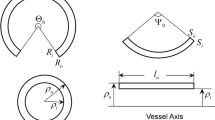
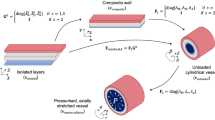
The objective is to know the stress distributions in the arterial walls under residual stresses based on two-layer model. Human common carotid arteries were analysed to show stress distributions at physiological and supraphysiological intraluminal pressures. The analyses for the loaded states were performed with stretch ratios with reference to a Riemannian stress-free configuration which is a 3D non-Euclidean manifold due to the nonzero Riemann curvature tensor. The experimental data obtained by other literature were used for the common carotid arteries to analyse the stretch and stress distributions in the arterial wall although kinematics is different from the literature. The stretches and stresses were calculated for the unloaded state, i.e. the residual stretches and stresses. And those at the axial stretch ratio 1.1 with reference to the unloaded state were calculated at the intraluminal pressures 16, 50, and 100 kPa. The stresses increased from the inner surface to the outer surface at all pressures analysed. These results suggest that in the human arteries the mechanical loads are mainly supported with the adventitia even though the media and intima play an important role to control of physiological functions.

Appendix 1

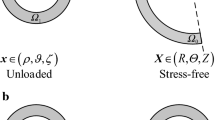
If the Riemannian stress-free configuration is non-Euclidean, the Riemann curvature tensor is not zero and the stress-free configuration cannot be embedded in 3D Euclidean space. The covariant components of Riemann curvature tensor are defined as follows (Sokolnikoff 1964):

$$\begin{gathered} R_{{{\text{abcd}}}} = \frac{1}{2}\left( {\frac{{\partial^{2} \eta_{{{\text{ad}}}} }}{{\partial \xi^{{\text{b}}} \partial \xi^{{\text{c}}} }} + \frac{{\partial^{2} \eta_{{{\text{bc}}}} }}{{\partial \xi^{{\text{a}}} \partial \xi^{{\text{d}}} }} - \frac{{\partial^{2} \eta_{{{\text{ac}}}} }}{{\partial \xi^{{\text{b}}} \partial \xi^{{\text{d}}} }} - \frac{{\partial^{2} \eta_{{{\text{bd}}}} }}{{\partial \xi^{{\text{a}}} \partial \xi^{{\text{c}}} }}} \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, + \eta^{{{\text{ef}}}} (\Gamma_{{{\text{ead}}}} \Gamma_{{{\text{fbc}}}} - \Gamma_{{{\text{eac}}}} \Gamma_{{{\text{fbd}}}} ) \hfill \\ \end{gathered}$$

(31)

where a, b, c, d, e, f are variable sub- and super-scripts taking values of 1, 2, 3, and $\eta^{{{\text{ef}}}}$ denote the reciprocal components of the metric tensor and $\Gamma_{{{\text{ead}}}}$ the Christoffel symbols of the first kind, respectively. We omit the subscript of X which denotes adventitia or media and take $\xi^{1} = \vartheta$, $\xi^{2} = \zeta$, $\xi^{3} = \rho$.

In general, the number of distinct components of Riemann curvature tensor that may not vanish is six for 3D manifold, because a n-dimensional Riemannian manifold has $n^{2} (n^{2} - 1)/12$ of distinct components (Sokolnikoff 1964).

The Christoffel symbols of the first kind (Sokolnikoff 1964) of the Riemannian stress-free configuration are:

$$\Gamma_{{{\text{cab}}}} = \frac{1}{2}\left( {\frac{{\partial \eta_{{{\text{ac}}}} }}{{\partial \xi^{{\text{b}}} }} + \frac{{\partial \eta_{{{\text{bc}}}} }}{{\partial \xi^{{\text{a}}} }} - \frac{{\partial \eta_{{{\text{ab}}}} }}{{\partial \xi^{{\text{c}}} }}} \right)$$

(32)

where the components of the metric tensor in the present study are as follows (Eq. 7):

$$\eta_{\vartheta \vartheta } = \left( {\frac{{\Theta_{0} R}}{2\pi }} \right)^{2} ,\,\,\,\,\eta_{\zeta \zeta } = \left( {\frac{{\Psi_{0} S}}{{l_{{\text{u}}} }}} \right)^{2} ,\,\,\,\,\eta_{\rho \rho } = \left( {\frac{dR}{{d\rho }}} \right)^{2} = \left( {\frac{dS}{{d\rho }}} \right)^{2}$$

$$\eta_{{{\text{ab}}}} = 0\quad (a \ne b)$$

The Christoffel symbols of the first kind of 3D manifold in the present study are as follows:

• (1)
  $$a = b:$$

$$\Gamma_{{{\text{caa}}}} = \frac{1}{2}\left( {\frac{{\partial \eta_{{{\text{ac}}}} }}{{\partial \xi^{{\text{a}}} }} + \frac{{\partial \eta_{{{\text{ac}}}} }}{{\partial \xi^{{\text{a}}} }} - \frac{{\partial \eta_{{{\text{aa}}}} }}{{\partial \xi^{{\text{c}}} }}} \right)\,\,\,\,\left( {{\text{not sum with}}\;a} \right)$$

• (i)
  $$a = c:\;\Gamma_{{{\text{caa}}}} = \frac{1}{2}\frac{{\partial \eta_{{{\text{aa}}}} }}{{\partial \xi^{{\text{a}}} }}\;\;\left( {{\text{not sum with}}\;a} \right)$$

Nonvanishing case:

$$\Gamma_{\rho \rho \rho } = \frac{1}{2}\frac{{d\eta_{\rho \rho } }}{d\rho }$$

• (ii)
  $$a \ne c:\;\Gamma_{{{\text{caa}}}} = - \frac{1}{2}\frac{{\partial \eta_{{{\text{aa}}}} }}{{\partial \xi^{{\text{c}}} }}\;\;\;\left( {{\text{not sum with}}\;a} \right)$$

Nonvanishing case:

$$\Gamma_{\rho \vartheta \vartheta } = - \frac{1}{2}\frac{{d\eta_{\vartheta \vartheta } }}{d\rho },\,\,\,\,\Gamma_{\rho \zeta \zeta } = - \frac{1}{2}\frac{{d\eta_{\zeta \zeta } }}{d\rho }$$

• (2)
  $$a \ne b:$$

$$\Gamma_{{{\text{cab}}}} = \frac{1}{2}\left( {\frac{{\partial \eta_{{{\text{ac}}}} }}{{\partial \xi^{{\text{b}}} }} + \frac{{\partial \eta_{{{\text{bc}}}} }}{{\partial \xi^{{\text{a}}} }}} \right)$$

• (i)
  $$a = c:\;\Gamma_{{{\text{cab}}}} = \frac{1}{2}\frac{{\partial \eta_{{{\text{aa}}}} }}{{\partial \xi^{{\text{b}}} }}\;\;\;\left( {{\text{not sum with}}\,a} \right)$$

Nonvanishing case:

$$\Gamma_{aa\rho } = \frac{1}{2}\frac{{d\eta_{{{\text{aa}}}} }}{d\rho }\quad (a \ne \rho )\,\;\;\,\left( {{\text{not sum with}}\;a} \right)$$

$$\Gamma_{\vartheta \vartheta \rho } = \frac{1}{2}\frac{{d\eta_{\vartheta \vartheta } }}{d\rho },\;\;\;\Gamma_{\zeta \zeta \rho } = \frac{1}{2}\frac{{d\eta_{\zeta \zeta } }}{d\rho }$$

• (ii)
  $$ii)\;b = c:\;\Gamma_{{{\text{cab}}}} = \frac{1}{2}\frac{{\partial \eta_{{{\text{bb}}}} }}{{\partial \xi^{{\text{a}}} }}\;\;\;\left( {{\text{not sum with}}\;b} \right)$$

Nonvanishing case:

$$\Gamma_{\vartheta \rho \vartheta } = \frac{1}{2}\frac{{d\eta_{\vartheta \vartheta } }}{d\rho },\;\;\;\Gamma_{\zeta \rho \zeta } = \frac{1}{2}\frac{{d\eta_{\zeta \zeta } }}{d\rho }$$

Therefore, the nonvanishing components of the Christoffel symbols of the first kind are as follows:

$$\Gamma_{\vartheta \vartheta \rho } = \Gamma_{\vartheta \rho \vartheta } = \frac{1}{2}\frac{{d\eta_{\vartheta \vartheta } }}{d\rho } = \left( {\frac{{\Theta_{0} }}{2\pi }} \right)^{2} R\frac{dR}{{d\rho }}$$

$$\Gamma_{\zeta \zeta \rho } = \Gamma_{\zeta \rho \zeta } = \frac{1}{2}\frac{{d\eta_{\zeta \zeta } }}{d\rho } = \left( {\frac{{\Psi_{0} }}{{l_{u} }}} \right)^{2} S\frac{dS}{{d\rho }}$$

$$\Gamma_{\rho \vartheta \vartheta } = - \frac{1}{2}\frac{{d\eta_{\vartheta \vartheta } }}{d\rho } = - \left( {\frac{{\Theta_{0} }}{2\pi }} \right)^{2} R\frac{dR}{{d\rho }}$$

(33)

$$\Gamma_{\rho \zeta \zeta } = - \frac{1}{2}\frac{{d\eta_{\zeta \zeta } }}{d\rho } = - \left( {\frac{{\Psi_{0} }}{{l_{u} }}} \right)^{2} S\frac{dS}{{d\rho }}$$

$$\Gamma_{\rho \rho \rho } = \frac{1}{2}\frac{{d\eta_{\rho \rho } }}{d\rho } = \frac{dR}{{d\rho }}\frac{{d^{2} R}}{{d\rho^{2} }} = \frac{dS}{{d\rho }}\frac{{d^{2} S}}{{d\rho^{2} }}$$

The independent covariant components of Riemann curvature tensor in 3D Riemannian manifold are $R_{\vartheta \zeta \vartheta \zeta }$, $R_{\vartheta \rho \vartheta \rho }$, $R_{\zeta \rho \zeta \rho }$, $R_{\vartheta \zeta \vartheta \rho }$, $R_{\zeta \vartheta \zeta \rho }$, $R_{\rho \vartheta \rho \zeta }$:

$$\begin{gathered} R_{\vartheta \zeta \vartheta \zeta } = \frac{1}{{\eta_{\vartheta \vartheta } }}\left( {\Gamma_{\vartheta \vartheta \zeta } \Gamma_{\vartheta \zeta \vartheta } - \Gamma_{\vartheta \vartheta \vartheta } \Gamma_{\vartheta \zeta \zeta } } \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, + \frac{1}{{\eta_{\zeta \zeta } }}\left( {\Gamma_{\zeta \vartheta \zeta } \Gamma_{\zeta \zeta \vartheta } - \Gamma_{\zeta \vartheta \vartheta } \Gamma_{\zeta \zeta \zeta } } \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, + \frac{1}{{\eta_{\rho \rho } }}\left( {\Gamma_{\rho \vartheta \zeta } \Gamma_{\rho \rho \vartheta } - \Gamma_{\rho \vartheta \vartheta } \Gamma_{\rho \zeta \zeta } } \right) \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\, = - \frac{1}{{\eta_{\rho \rho } }}\Gamma_{\rho \vartheta \vartheta } \Gamma_{\rho \zeta \zeta } = \left( {\frac{{\Theta_{0} \Psi_{0} }}{{2\pi l_{u} }}} \right)^{2} {\text{RS}} \hfill \\ \end{gathered}$$

(34)

By the similar calculations we can obtain the following results:

$$R_{\vartheta \rho \vartheta \rho } = R_{\zeta \rho \zeta \rho } = R_{\vartheta \zeta \vartheta \rho } = R_{\zeta \vartheta \zeta \rho } = R_{\rho \vartheta \rho \zeta } = 0$$

(35)