Skip to main content
SpringerLink
Log in

Behavioral premium principles

  • Published:
Decisions in Economics and Finance Aims and scope Submit manuscript

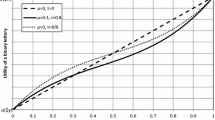
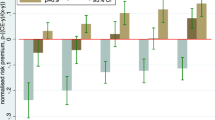
Abstract

We define a premium principle under the continuous cumulative prospect theory which extends the equivalent utility principle. In prospect theory, risk attitude and loss aversion are shaped via a value function, whereas a transformation of objective probabilities, which is commonly referred as probability weighting, models probabilistic risk perception. In cumulative prospect theory, probabilities of individual outcomes are replaced by decision weights, which are differences in transformed, through the weighting function, counter-cumulative probabilities of gains and cumulative probabilities of losses, with outcomes ordered from worst to best. Empirical evidence suggests a typical inverse-S shaped function: decision makers tend to overweight small probabilities, and underweight medium and high probabilities; moreover, the probability weighting function is initially concave and then convex. We study some properties of the behavioral premium principle. We also assume an alternative framing of the outcomes; then, we discuss several applications to the pricing of insurance contracts, considering different value functions and probability weighting functions proposed in the literature, and an alternative mental accounting. Finally, we focus on the shape of the probability weighting function.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

Notes

  1. The book of Wakker (2010) provides a thorough treatment on prospect theory.

  2. See Quiggin (1993), p. 56.

  3. See also Rieger and Wang (2008), Wakker (2010), and Kothiyal et al. (2011).

  4. When there is no ambiguity, we simply use the notation \(F(x) = {\mathbb {P}}(X\le x)\) and \(S(x) = 1- F(x) = {\mathbb {P}}(X > x)\), and f for the probability density function of the random loss X.

  5. The premium principle defined in Wang (1996) assumes an increasing and concave distortion function and maintains the second-order stochastic dominance.

  6. See Thaler (1985), p. 202.

  7. Time-value of money is normally disregarded when dealing with non-life insurance contracts, but may become important on a multi-year horizon.

  8. Note that \({\mathbb {E}}_{w^+w^-} (cX) = c {\mathbb {E}}_{w^+w^-} (X)\), for \(c\ge 0\).

  9. In general, linearity does not hold for the generalized Choquet integral. In Sect. 3.1, we discussed the case with \(w^+=w^-\). When \(w^+\ne w^-\), and \(c\in {\mathbb {R}}\), we apply the following result

    $$\begin{aligned} {\mathbb {E}}_{w^+w^-}(X+c) = {\mathbb {E}}_{w^+w^-}(X) + c + \int _0^c [w^-({\mathbb {P}}(-X>s))-\overline{w}^+({\mathbb {P}}(-X>s))]\,\mathrm{{d}}s, \end{aligned}$$

    where \(\overline{w}\) is the dual probability weighting function. See Kaluszka and Krzeszowiec (2012) for the proof and discussion of further properties of the generalized Choquet integral.

  10. We have \(u(0)=0\), \(u^\prime >0\), \(u^\prime (0)=b/a\), \(u^{\prime \prime }<0\). Heilpern (2003) considers the normalized case \(a=b\).

  11. The same result arises also when \(a=b\) and with \(W=0\).

  12. We have \(u(0)=0\), \(u^\prime >0\), \(u^\prime (0)=b/a\), and \(u^{\prime \prime }>0\), which may be useful to model the value function in the domain of losses.

  13. Observe that \(\int _0^c \psi (F(x))f(x)\mathrm{{d}}x = w(F(c))\).

  14. In the literature discontinuous (neo-additive) weighting functions are also considered.

  15. See e.g., Prelec (1998), Abdellaoui (2000), Bleichrodt and Pinto (2000), Bleichrodt et al. (2001), and Abdellaoui et al. (2007).

  16. In the same paper, Prelec derives two other probability weighting functions: the conditionally-invariantexponential-power and the projection-invarianthyperbolic-logarithm function.

  17. This is not the case for weighting function (25); when \(a \ne b\), both parameters controls for curvature and all parameters may influence elevation.

References

  • Abdellaoui, M.: Parameter-free elicitation of utility and probability weighting functions. Manag. Sci. 46, 1497–1512 (2000)

    Article  Google Scholar 

  • Abdellaoui, M., Barrios, C., Wakker, P.P.: Reconciling introspective utility with revealed preference: Experimental arguments based on prospect theory. J. Econom. 138, 336–378 (2007)

    Article  Google Scholar 

  • Abdellaoui, M., L’Haridon, O., Zank, H.: Separating curvature and elevation: a parametric probability weighting function. J. Risk Uncertain. 41, 39–65 (2010)

    Article  Google Scholar 

  • Allais, M.: Le comportement de l’homme rationnel devant le risque: Critique des postulats et axiomes de l’école Américaine. Econometrica 21(4), 503–546 (1953)

    Article  Google Scholar 

  • Allais, M.: The general theory of random choices in relation to the invariant cardinal utility function and the specific probability function. The \((U, \theta )-\)Model: a general overview. In: Munier, B.R. (ed.) Risk, Decision and Rationality, pp. 231–289. D. Reidel Publishing Company, Dordrecht, Holland (1988)

    Chapter  Google Scholar 

  • Balbás, A., Garrido, J., Mayoral, S.: Properties of distortion risk measures. Methodol. Comput. Appl. Probab. 11, 385–399 (2009)

    Article  Google Scholar 

  • Bell, D.E.: Disappointment in decision making under uncertainty. Oper. Res. 33, 1–27 (1985)

    Article  Google Scholar 

  • Belles-Sampera, J., Merigó, J.M., Guillén, M., Santolino, M.: The connection between distortion risk measures and ordered weighted averaging operators. Insur. Math. Econ. 52(2), 411–420 (2013)

    Article  Google Scholar 

  • Belles-Sampera, J., Guillén, M., Santolino, M.: The use of flexible quantile-based measures in risk assessment. Commun. Stat. Theory Methods 45(6), 1670–1681 (2016)

    Article  Google Scholar 

  • Birnbaum, M.H., McIntosh, W.R.: Violations of branch independence in choices between gambles. Organ. Behav. Hum. Decis. Process. 67, 91–110 (1996)

    Article  Google Scholar 

  • Bleichrodt, H., Pinto, J.L.: A parameter-free elicitation of the probability weighting function in medical decision analysis. Manag. Sci. 46, 1485–1496 (2000)

    Article  Google Scholar 

  • Bleichrodt, H., Pinto, J.L., Wakker, P.P.: Making descriptive use of prospect theory to improve the prescriptive use of expected utility. Manag. Sci. 47, 1498–1514 (2001)

    Article  Google Scholar 

  • Currim, I.S., Sarin, R.K.: Prospect versus utility. Manag. Sci. 35(1), 22–41 (1989)

    Article  Google Scholar 

  • Davies, G.B., Satchell, S.E.: The behavioural components of risk aversion. J. Math. Psychol. 51, 1–13 (2007)

    Article  Google Scholar 

  • Diecidue, E., Schmidt, U., Zank, H.: Parametric weighting functions. J. Econ. Theory 144(3), 1102–1118 (2009)

    Article  Google Scholar 

  • Gerber, H.U.: An Introduction to Mathematical Risk Theory. S.S. Huebner Foundation for Insurance, University of Pennsylvania, Philadelphia (1979)

    Google Scholar 

  • Gerber, H.U.: On additive principles of zero utility. Insur. Math. Econ. 4, 249–251 (1985)

    Article  Google Scholar 

  • Goldstein, W.M., Einhorn, H.J.: Expression theory and the preference reversal phenomena. Psychol. Rev. 94(2), 236–254 (1987)

    Article  Google Scholar 

  • Gonzalez, R., Wu, G.: On the shape of the probability weighting function. Cognit. Psychol. 38, 129–166 (1999)

    Article  Google Scholar 

  • Goovaerts, M.J., Kaas, R., Laeven, R.J.A.: A note on additive risk measures in rank-dependent utility. Insur. Math. Econ. 47(2), 187–189 (2010)

    Article  Google Scholar 

  • Goovaerts, M.J., Kaas, R., Laeven, R.J.A., Tang, Q.: A comonotonic image of independence for additive risk measures. Insur. Math. Econ. 35(3), 581–594 (2004)

    Article  Google Scholar 

  • Hamada, M., Sherris, M.: Contingent claim pricing using probability distortion operators: methods from insurance risk pricing and their relationship to financial theory. Appl. Math. Finance 10, 19–47 (2003)

    Article  Google Scholar 

  • Heilpern, S.: A rank-dependent generalization of zero utility principle. Insur. Math. Econ. 33(1), 67–73 (2003)

    Article  Google Scholar 

  • Hey, J.D., Orme, C.: Investigating generalizations of expected utility theory using experimental data. Econometrica 62(6), 1291–1326 (1994)

    Article  Google Scholar 

  • Kahneman, D., Tversky, A.: Prospect theory: an analysis of decision under risk. Econometrica 47(2), 263–291 (1979)

    Article  Google Scholar 

  • Kaluszka, M., Krzeszowiec, M.: Pricing insurance contracts under cumulative prospect theory. Insur. Math. Econ. 50(1), 159–166 (2012)

    Article  Google Scholar 

  • Kaluszka, M., Krzeszowiec, M.: On iterative premium calculation principles under cumulative prospect theory. Insur. Math. Econ. 52(3), 435–440 (2013)

    Article  Google Scholar 

  • Kaluszka, M., Okolewski, A.: An extension of Arrow’s result on optimal reinsurance contract. J. Risk Insur. 75(2), 275–288 (2008)

    Article  Google Scholar 

  • Karmarkar, U.S.: Subjectively weighted utility: a descriptive extension of the expected utility model. Organ. Behav. Hum. Perform. 21, 61–72 (1978)

    Article  Google Scholar 

  • Karmarkar, U.S.: Subjectively weighted utility and the Allais paradox. Organ. Behav. Hum. Perform. 24, 67–72 (1979)

    Article  Google Scholar 

  • Kilka, M., Weber, M.: What determines the shape of the probability weighting function under uncertainty? Manag. Sci. 47(12), 1712–1726 (2001)

    Article  Google Scholar 

  • Kothiyal, A., Spinu, V., Wakker, P.P.: Prospect theory for continuous distributions: a preference foundation. J. Risk Uncertain. 42, 195–210 (2011)

    Article  Google Scholar 

  • Lattimore, P.K., Baker, J.R., Witte, A.D.: The influence of probability on risky choice: a parametric examination. J. Econ. Behav. Organ. 17(3), 377–400 (1992)

    Article  Google Scholar 

  • Loomes, G., Moffatt, P.G., Sugden, R.: A microeconometric test of alternative stochastic theories of risk choice. J. Risk. Uncertain. 24, 103–130 (2002)

    Article  Google Scholar 

  • Luce, D.R.: Utility of Gains and Losses: Measurement-Theoretical and Experimental Approaches. Lawrence Erlbaum Publishers, London (2000)

    Google Scholar 

  • Luce, D.R.: Reduction invariance and Prelec’s weighting functions. J. Math. Psychol. 45, 167–179 (2001)

    Article  Google Scholar 

  • Luce, D.R., Mellers, B.A., Chang, S.J.: Is choice the correct primitive? On using certainty equivalents and reference levels to predict choices among gambles. J. Risk Uncertain. 6, 115–143 (1993)

    Article  Google Scholar 

  • Nardon, M., Pianca, P.: European option pricing under cumulative prospect theory with constant relative sensitivity probability weighting functions. Comput. Manag. Sci. 16, 249–274 (2018). https://doi.org/10.1007/s10287-018-0324-y

    Article  Google Scholar 

  • Pfiffelmann, M.: Solving the St. Petersburg paradox in cumulative prospect theory: the right amount of probability weighting. Theory Decis. 75, 325–341 (2011)

    Article  Google Scholar 

  • Prelec, D.: The probability weighting function. Econometrica 66, 497–527 (1998)

    Article  Google Scholar 

  • Quiggin, J.: A theory of anticipated utility. J. Econ. Behav. Organ. 3, 323–343 (1982)

    Article  Google Scholar 

  • Quiggin, J.: Generalized Expected Utility Theory: The Rank-Dependent Model. Springer, Netherlands (1993)

    Book  Google Scholar 

  • Rieger, M.O., Wang, M.: Cumulative prospect theory and the St Petrsburg paradox. J. Econ. Theory. 28, 665–679 (2006)

    Article  Google Scholar 

  • Rieger, M.O., Wang, M.: Prospect theory for continuous distributions. J. Risk Uncertain. 36, 83–102 (2008)

    Article  Google Scholar 

  • Röell, A.: Risk aversion in Quiggin and Yaari’s rank-order model of choice under uncertainty. Econ. J. 97, 143–159 (1987)

    Article  Google Scholar 

  • Safra, Z., Segal, U.: Constant risk aversion. J. Econ. Theory 83, 19–42 (1998)

    Article  Google Scholar 

  • Schmeidler, D.: Subjective probability and expected utility without additivity. Econometrica 57, 571–587 (1989)

    Article  Google Scholar 

  • Shiller, R.J.: Human behavior and the efficiency of the financial system. In: Taylor, J.B., Woodford, M. (eds.) Handbook of Macroeconomics, vol. 1C, pp. 1305–1340. Elsevier, Amsterdam (1999)

    Chapter  Google Scholar 

  • Sung, K.C.J., Yam, S.C.P., Yung, S.P., Zhou, J.H.: Behavioral optimal insurance. Insur. Math. Econ. 49, 418–428 (2011)

    Article  Google Scholar 

  • Thaler, R.H.: Mental accounting and consumer choice. Mark. Sci. 4, 199–214 (1985)

    Article  Google Scholar 

  • Tsanakas, A.: To split or not to split: capital allocation with convex risk measures. Insur. Math. Econ. 44, 268–277 (2009)

    Article  Google Scholar 

  • Tversky, A., Fox, C.R.: Weighting risk and uncertainty. Psychol. Rev. 102, 269–283 (1995)

    Article  Google Scholar 

  • Tversky, A., Kahneman, D.: Advances in prospect theory: cumulative representation of the uncertainty. J. Risk Uncertain. 5, 297–323 (1992)

    Article  Google Scholar 

  • van der Hoek, J., Sherris, M.: A class of non-expected utility risk measures and implications for asset allocations. Insur. Math. Econ. 28(1), 69–82 (2001)

    Article  Google Scholar 

  • Wakker, P.P.: Prospect Theory: For Risk and Ambiguity. Cambridge University Press, Cambridge (2010)

    Book  Google Scholar 

  • Walther, H.: Normal randomness expected utility, time preferences and emotional distortions. J. Econ. Behav. Organ. 52, 253–266 (2003)

    Article  Google Scholar 

  • Wang, S.S.: Premium calculation by transforming the layer premium density. ASTIN Bull. 26(1), 71–92 (1996)

    Article  Google Scholar 

  • Wang, S.S.: A class of distortion operators for pricing financial and insurance risks. J. Risk Insur. 67(1), 15–36 (2000)

    Article  Google Scholar 

  • Werner, K.M., Zank, H.: A revealed reference point for prospect theory. J. Econ. Theory (2018). https://doi.org/10.1007/s00199-017-1096-2

    Article  Google Scholar 

  • Wu, G., Gonzalez, R.: Curvature of the probability weighting function. Manag. Sci. 42(12), 1676–1690 (1996)

    Article  Google Scholar 

  • Wu, G., Gonzalez, R.: Nonlinear decision weights in choice under uncertainty. Manag. Sci. 45(1), 74–85 (1999)

    Article  Google Scholar 

  • Yaari, M.: The dual theory of choice under risk. Econometrica 55(1), 95–115 (1987)

    Article  Google Scholar 

Download references

Acknowledgements

The authors thank two anonymous referees for useful comments and suggestions of references.

Author information

Authors and Affiliations

  1. Department of Economics, Ca’ Foscari University of Venice, San Giobbe - Cannaregio 873, 30121, Venezia, Italy

    Martina Nardon & Paolo Pianca

Authors
  1. Martina Nardon
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Paolo Pianca
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Martina Nardon.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Nardon, M., Pianca, P. Behavioral premium principles. Decisions Econ Finan 42, 229–257 (2019). https://doi.org/10.1007/s10203-019-00246-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10203-019-00246-x

Keywords

JEL Classification

Search

Navigation