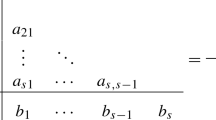Skip to main content
SpringerLink
Log in

Embedded (4, 5) pairs of explicit 7-stage Runge–Kutta methods with FSAL property

  • Published:
Calcolo Aims and scope Submit manuscript

Abstract

The general case of embedded (4, 5) pairs of explicit 7-stage Runge–Kutta methods with FSAL property (\(a_{7 j} = b_{ j}\), \(1 \le j \le 7\), \(c_7 = 1\)) is considered. Besides exceptional cases, the pairs form five 4-dimensional families. The pairs within two (already known) families satisfy the simplifying assumption \(\sum _j a_{ij}c_{j} = c_i^2 / 2\), \(i \ge 3\).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

Notes

  1. It is natural and will be assumed that \(\sum _{j = 1}^{i - 1} a_{ij}= c_{ i}\). For \(i = 1\) the sum is empty, so \(c_1 = 0\) and \({{{\varvec{F}}}}_1 = {{{\varvec{f}}}} \bigl ( t, {{{\varvec{x}}}}(t) \bigr )\).

  2. Usually the \({4}{\text {th}}\) order method vector of weights \({{{\varvec{b}}}} + {{{\varvec{d}}}}\) is written in place of \({{{\varvec{d}}}}\).

  3. The FSAL property \(a_{7 j} = b_{ j}\), \(1 \le j \le 7\) and the order conditions \({{{\varvec{b}}}}^{\text {T}} {{{\varvec{c}}}} = 1 / 2\), \({{{\varvec{b}}}}^{\text {T}} {{{\varvec{c}}}}' = 1 / 6\), \({{{\varvec{b}}}}^{\text {T}} {{{\varvec{c}}}}'' = 1 / 24\) result in \(c'_7 = 1 / 2\), \(c''_7 = 1 / 6\), and \(c'''_7 = 1 / 24\).

  4. The full analysis of \(a_{65} a_{54} a_{43} a_{32} = 0\) case is tedious and is not expected to result in an embedded pair of practical interest. For instance, if \(a_{32} = 0\), then \(c_3 = 3 c_2 / (8 c_2 - 3)\) and \(c_4 = 0\).

  5. Further derivation was done in interaction with computer algebra system Wolfram Mathematica 8.0, mainly using commands Solve to symbolically solve linear equations, Simplify, and (in Sect. 3) Factor.

  6. Also \(b_4 \mu _{4, *} + b_5 \mu _{5, *} + b_6 \mu _{6, *} = 0\), as \(b_4 = (1 / 24 - b_5 c''_5 - b_6 c''_6) / c''_4\).

  7. In the case of an embedded pair of 6-stage Runge–Kutta methods, i.e., \(d_7 = 0\), the rank of the matrix \({{{\varvec{M}}}}\) without the \({5}{\text {th}}\) row should be equal to 1.

  8. Compare with [11, Eq. (3.2)], and [19, Eq. (16)] (the latter contains a sign error), where \(c'_3 = c_3^2 / 2\).

  9. They are also satisfied when \((c_3 - c_2) (c_3^2 - 2 c'_3) = 0\), \(c_3^2 (c_3 - c_2) - c'_3 c_2 = 0\), and \(c_3^2 (c_3 - c_2) + 2 c'_3 c_2 = 0\), respectively. Not satisfying any of Eq. (6) and Eq. (7) would imply \(c'_3 c_2 = 0\) then.

  10. Compare with [12, Eq. (20)], [11, Eq. (3.3)], [19, p. 1173, Corollary 1].

  11. If instead of \(c'_3\) the variable \(g'_3 = c'_3 / c_3 (c_3 - c_2)\) is used, then the degrees are 6, 2, 3, 2, and 8.

  12. Pairs form a set of codimension 2 in the 6-dimensional space \(({{{\varvec{c}}}}, c'_3)\). In Fig. 2 the curves in the cut have codimension 1, as at least one of the eqs. (6) and (7) (in fact, both) is satisfied.

References

  1. Ascher, U.M., Petzold, L.R.: Computer methods for ordinary differential equations and differential-algebraic equations. SIAM (1998). https://doi.org/10.1137/1.9781611971392

    Article  MATH  Google Scholar 

  2. Bogacki, P., Shampine, L.F.: An efficient Runge–Kutta \((4, 5)\) pair. Comput. Math. Appl. 32(6), 15–28 (1996). https://doi.org/10.1016/0898-1221(96)00141-1

    Article  MathSciNet  MATH  Google Scholar 

  3. Butcher, J.C.: On Runge–Kutta processes of high order. J. Aust. Math. Soc. 4(2), 179–194 (1964). https://doi.org/10.1017/S1446788700023387

    Article  MathSciNet  MATH  Google Scholar 

  4. Butcher, J.C.: Numerical Methods for Ordinary Differential Equations, 3rd edn. John Wiley & Sons Ltd, New York (2016). https://doi.org/10.1002/9781119121534

    Book  MATH  Google Scholar 

  5. Butcher, J.C.: B-series: Algebraic Analysis of Numerical Methods. Springer, Berlin (2021). https://doi.org/10.1007/978-3-030-70956-3

    Book  MATH  Google Scholar 

  6. Butcher, J.C., Wanner, G.: Runge–Kutta methods: some historical notes. Appl. Numer. Math. 22(1–3), 113–151 (1996). https://doi.org/10.1016/S0168-9274(96)00048-7

    Article  MathSciNet  MATH  Google Scholar 

  7. Cash, J.R., Karp, A.H.: A variable order Runge–Kutta method for initial value problems with rapidly varying right-hand sides. ACM Trans. Math. Softw. 16(3), 201–222 (1990). https://doi.org/10.1145/79505.79507

    Article  MathSciNet  MATH  Google Scholar 

  8. Cassity, C.R.: Solution of the fifth-order Runge–Kutta equations. SIAM J. Numer. Anal. 3(4), 598–606 (1966). https://doi.org/10.1137/0703052

    Article  MathSciNet  MATH  Google Scholar 

  9. Cassity, C.R.: The complete solution of the fifth order Runge–Kutta equations. SIAM J. Numer. Anal. 6(3), 432–436 (1969). https://doi.org/10.1137/0706038

    Article  MathSciNet  MATH  Google Scholar 

  10. Dormand, J.R., Prince, P.J.: New Runge–Kutta algorithms for numerical simulation in dynamical astronomy. Celest. Mech. 18(3), 223–232 (1978). https://doi.org/10.1007/BF01230162

    Article  MathSciNet  MATH  Google Scholar 

  11. Dormand, J.R., Prince, P.J.: A family of embedded Runge–Kutta formulae. J. Comput. Appl. Math. 6(1), 19–26 (1980). https://doi.org/10.1016/0771-050X(80)90013-3

    Article  MathSciNet  MATH  Google Scholar 

  12. Fehlberg, E.: Low-order classical Runge–Kutta formulas with stepsize control and their application to some heat transfer problems, NASA Technical Report R-315 (1969)

  13. Fehlberg, E.: Klassische Runge–Kutta–Formeln vierter und niedrigerer Ordnung mit Schrittweiten-Kontrolle und ihre Anwendung auf Wärmeleitungsprobleme. Computing 6, 61–71 (1970). https://doi.org/10.1007/BF02241732

    Article  MathSciNet  MATH  Google Scholar 

  14. Hairer, E., Nørsett, S.P.: Solving Ordinary Differential Equations I: Nonstiff Problems, 2nd edn. Springer, Berlin (1993). https://doi.org/10.1007/978-3-540-78862-1

    Book  MATH  Google Scholar 

  15. Hull, T.E., Enright, W.H., Fellen, B.M., Sedgwick, A.E.: Comparing numerical methods for ordinary differential equations. SIAM J. Numer. Anal. 9(4), 603–637 (1972). https://doi.org/10.1137/0709052

    Article  MathSciNet  MATH  Google Scholar 

  16. Iserles, A.: A First Course in the Numerical Analysis of Differential Equations, 2nd edn. Cambridge University Press, Cambridge (2008). https://doi.org/10.1017/CBO9780511995569

    Book  MATH  Google Scholar 

  17. Lawson, J.D.: An order five Runge–Kutta process with extended region of stability. SIAM J. Numer. Anal. 3(4), 593–597 (1966). https://doi.org/10.1137/0703051

    Article  MathSciNet  MATH  Google Scholar 

  18. Owren, B., Zennaro, M.: Derivation of efficient, continuous, explicit Runge–Kutta methods. SIAM J. Sci. Stat. Comput. 13(6), 1488–1501 (1992). https://doi.org/10.1137/0913084

    Article  MathSciNet  MATH  Google Scholar 

  19. Papakostas, S.N., Papageorgiou, G.: A family of fifth-order Runge–Kutta pairs. Math. Comput. 65(215), 1165–1181 (1996). https://doi.org/10.1090/S0025-5718-96-00718-1

    Article  MathSciNet  MATH  Google Scholar 

  20. Sharp, P.W., Smart, E.: Explicit Runge–Kutta pairs with one more derivative evaluation than the minimum. SIAM J. Sci. Comput. 14(2), 338–348 (1993). https://doi.org/10.1137/0914021

    Article  MathSciNet  MATH  Google Scholar 

  21. Tsitouras, Ch.: Runge–Kutta pairs of order 5(4) satisfying only the first column simplifying assumption. Comput. Math. Appl. 62(2), 770–775 (2011). https://doi.org/10.1016/j.camwa.2011.06.002

    Article  MathSciNet  MATH  Google Scholar 

  22. Verner, J.H.: Explicit Runge–Kutta methods with estimates of the local truncation error. SIAM J. Numer. Anal. 15(4), 772–790 (1978). https://doi.org/10.1137/0715051

    Article  MathSciNet  MATH  Google Scholar 

  23. Verner, J.H.: Explicit Runge–Kutta pairs with lower stage-order. Numer. Algorithms 65(3), 555–577 (2014). https://doi.org/10.1007/s11075-013-9783-y

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The author is grateful to anonymous reviewers for helpful comments and suggestions.

Author information

Authors and Affiliations

  1. Department of Mathematics and Program in Applied Mathematics, University of Arizona, Tucson, AZ, 85721, USA

    Misha Stepanov

Authors
  1. Misha Stepanov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Misha Stepanov.

Ethics declarations

Conflict of interest

Not applicable.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendices

Formulas for pairs of type A

$$\begin{aligned} c_4&= c_3 \mathop {\big /} 2 (1 - 4 c_3 + 5 c_3^2) \\ c'_m&= c_m^2 / 2 , \qquad m = 3, 4, 5, 6 \\ c''_m&= c_m (c_m - c_3) (c_3 + c_m - 4 c_3 c_m) \mathop {\big /} 2 \bigl ( 3 - 12 c_3 + 10 c_3^2 \bigr ) , \qquad m = 4, 5, 6 \\ c'''_5&= c_3 c_5 (c_5 - c_3) (c_5 - c_4) \mathop {\big /} 4 (3 - 12 c_3 + 10 c_3^2) \\ g&= 8 c_3 - 15 c_3^2 - 4 c_5 (1 - 4 c_3 + 5 c_3^2) + 2 c_6 (2 - 13 c_3 + 20 c_3^2) \\ c'''_6&= \frac{g \, c_6 (c_6 - c_3) (c_6 - c_4)}{4 (3 - 12 c_3 + 10 c_3^2) (8 - 15 c_3 - 10 c_5 + 20 c_3 c_5)} \\ b_6 a_{65} c'''_5&= c_4 (2 - 5 c_3) / 240 \\ b_6 c'''_6&= g / 480 (c_6 - c_5) \bigl ( 1 - 4 c_3 + 5 c_3^2 \bigr ) \\ b_2&= d_2 = d_7 = 0 \\ d_5 c_5 (c_5&- c_3) (c_5 - c_4) + d_6 c_6 (c_6 - c_3) (c_6 - c_4) = 0 \end{aligned}$$

Note that \({{{\varvec{c}}}}'\), \({{{\varvec{c}}}}''\), \({{{\varvec{c}}}}'''\), and \(b_6\) do not depend on \(c_2\). As \(b_2 = d_2 = 0\), the whole vectors \({{{\varvec{b}}}}\) and \({{{\varvec{d}}}}\) do not depend on \(c_2\). The coefficients \(a_{ij}\) and the weights \(b_j\), \(d_j\) are obtained using formulas in the beginning of Section 1, e.g., \(b_5 = (1 / 120 - b_6 c'''_6) / c'''_5\).

Formulas for pairs of type B

$$\begin{aligned} g&= (3 - 12 c_2 + 10 c_2^2) (3 - 12 c_3 + 10 c_3^2) + 15 (c_2 + c_3 - 4 c_2 c_3)^2 \\ c_4&= 3 (3 - 10 c_2 c_3) (c_2 + c_3 - 4 c_2 c_3) \mathop {\big /} 2 g \\ c'_m&= 3 (c_m - c_2) (c_2 + c_m - 4 c_2 c_m) \mathop {\big /} 2 \bigl ( 3 - 12 c_2 + 10 c_2^2 \bigr ) , \qquad m = 3, 4, 5, 6 \\ h_m&= 3 c_2 + 3 c_3 + 3 c_m - 12 c_2 c_3 - 12 c_2 c_m - 12 c_3 c_m + 38 c_2 c_3 c_m \\ c''_m&= \frac{(c_m - c_2) (c_m - c_3) \, h_m}{2 \bigl ( 3 - 12 c_2 + 10 c_2^2 \bigr ) \bigl ( 3 - 12 c_3 + 10 c_3^2 \bigr )} , \qquad m = 4, 5, 6 \\ c'''_5&= \frac{3 (c_5 - c_2) (c_5 - c_3) (c_5 - c_4) (c_2 + c_3 - 4 c_2 c_3)}{4 \bigl ( 3 - 12 c_2 + 10 c_2^2 \bigr ) \bigl ( 3 - 12 c_3 + 10 c_3^2 \bigr )} \\ p&= 24 - 45 c_2 - 45 c_3 + 100 c_2 c_3 - 10 \bigl [ 3 - 6 c_2 - 6 c_3 + 14 c_2 c_3 \bigr ] c_5 \\ q&= 3 (c_2 + c_3 - 4 c_2 c_3) (24 - 45 c_2 - 45 c_3 + 100 c_2 c_3) {} \\&- \bigl [ 4 \bigl ( 3 - 12 c_2 + 10 c_2^2 \bigr ) \bigl ( 3 - 12 c_3 + 10 c_3^2 \bigr ) + 60 (c_2 + c_3 - 4 c_2 c_3)^2 \bigr ] c_5 {} \\&+ \bigl [ 4 \bigl ( 3 - 12 c_2 + 10 c_2^2 \bigr ) \bigl ( 3 - 12 c_3 + 10 c_3^2 \bigr ) {} \\&{}\qquad \quad {} - 30 (c_2 + c_3 - 4 c_2 c_3) (3 - 8 c_2 - 8 c_3 + 22 c_2 c_3) \bigr ] c_6 \\ c'''_6&= \frac{(c_6 - c_2) (c_6 - c_3) (c_6 - c_4) q}{4 \bigl ( 3 - 12 c_2 + 10 c_2^2 \bigr ) \bigl ( 3 - 12 c_3 + 10 c_3^2 \bigr ) p} \\ b_6 a_{65} c'''_5&= (c_2 + c_3 - 4 c_2 c_3) (6 - 15 c_2 - 15 c_3 + 40 c_2 c_3) \mathop {\big /} 160 g \\ b_6 c'''_6&= q \mathop {\big /} 480 (c_6 - c_5) g \\ b_1&= 1 / 9 \\ d_1&= d_7 = 0 \\ d_5 (c_5&- c_2) (c_5 - c_3) (c_5 - c_4) + d_6 (c_6 - c_2) (c_6 - c_3) (c_6 - c_4) = 0 \end{aligned}$$

Formulas for pairs of type B\({}'\), \(c_3 = 0\)

$$\begin{aligned} c_3&= 0 \\ c_6&= 1 \\&\begin{array}{|c|cc|cc|cc|cc|} \hline {\alpha _{l m n}} &{} { l = 0} &{}&{} { l = 1} &{}&{} { l = 2} &{}&{} { l = 3} &{}\\ &{} { m = 0} &{} { m = 1} &{} { m = 0} &{} { m = 1} &{} { m = 0} &{} { m = 1} &{} { m = 0} &{} { m = 1} \\ \hline { n = 0} &{} 144 &{} 180 &{} 180 &{} 228 &{} 72 &{} 93 &{} 9 &{} 12 \\ { n = 1} &{} 360 &{} 940 &{} 512 &{} 940 &{} 222 &{} 366 &{} 30 &{} 48 \\ { n = 2} &{} 200 &{} 1100 &{} 340 &{} 960 &{} 162 &{} 360 &{} 24 &{} 48 \\ \hline \end{array} \\ g&= 5 (c_2^2 + 4 c_4^2) c_5 (3 - 5 c_5) - c_2 c_4 \sum _{l = 0}^3 \sum _{m = 0}^1 \sum _{n = 0}^2 (-1)^{ l + m + n} \alpha _{l m n} (5 c_2)^l c_4^m c_5^n \\ p&= 3 - 5 c_2 - 5 c_4 + 10 c_2 c_4 \\ q&= 12 - 15 c_2 - 15 c_4 - 15 c_5 + 20 c_2 c_4 + 20 c_2 c_5 + 20 c_4 c_5 - 30 c_2 c_4 c_5 \\ c'_3&= 3 g \mathop {\big /} 2 (6 - 15 c_2 - 10 c_5 + 30 c_2 c_5) p q \\ c'_4&= 3 c_4 (c_4 - c_2) / 2 \\ c'_5&= 3 (c_5 - c_2) \bigl ( c_5 + c_4 (2 - 5 c_2 - 5 c_5 + 10 c_2 c_5) \bigr ) \mathop {\big /} 2 p \\ c'_6&= 3 (1 - c_2) \bigl ( 4 - 7 c_4 - 5 c_5 + 5 c_2 c_4 + 10 (1 - c_2) c_4 c_5 \bigr ) \mathop {\big /} 2 q \\ c''_m&= c'_m c_m / 3 , \qquad m = 4, 5, 6 \\ c'''_5&= c_4 c_5 (c_5 - c_2) (c_5 - c_4) (2 - 5 c_2) / 4 p \\ c'''_6&= (1 - c_2) (1 - c_4) (2 - 2 c_4 - 2 c_5 + 5 c_2 c_4) / 4 q \\ b_6 a_{65} c'''_5&= c_4 (2 - 5 c_2) / 240 \\ b_6 c'''_6&= (2 - 2 c_4 - 2 c_5 + 5 c_2 c_4) / 240 (1 - c_5) \\ d_5&= p \bigl ( c_2 c_4 + (c_2 - 2 c_4) (3 - 5 c_5) + 15 c_2 (1 - c_2) c_4 (1 - 2 c_5) \bigr ) \\ d_6&= q c_5 (c_5 - c_2) (c_5 - c_4) \bigl ( 4 c_4 - 2 c_2 - 14 c_2 c_4 + 15 c_2^2 c_4 \bigr ) \mathop {\big /} (1 - c_2) (1 - c_4) \\ d_7&= 15 c_5 (c_5 - c_2) (c_5 - c_4) (1 - c_5) \bigl (c_2 - 2 c_4 + 8 c_2 c_4 - 10 c_2^2 c_4 \bigr ) \end{aligned}$$

Formulas for pairs of type B\({}'\), \(c_3 = c_2\)

$$\begin{aligned} c_3&= c_2 \\ c_4&= (3 - 5 c_5) \mathop {\big /} 5 (1 - 2 c_5) \\ c_6&= 1 \\ c'_m&= c_m^2 / 2, \qquad m = 4, 5, 6 \\ c''_4&= c_4^2 (c_4 - c_2) / 2 \\ c''_5&= c_5 (c_5 - c_2) \bigl ( c_5 + c_4 (2 - 5 c_2 - 5 c_5 + 10 c_2 c_5) \bigr ) \mathop {\big /} 2 p \\ c''_6&= (1 - c_2) \bigl ( 4 - 7 c_4 - 5 c_5 + 5 c_2 c_4 + 10 (1 - c_2) c_4 c_5 \bigr ) \mathop {\big /} 2 q \\ b_1&= (1 - 8 c_5 + 10 c_5^2) / 12 c_5 (5 c_5 - 3) \\ b_2&= b_3 = 0 \\ b_4&= 125 (2 c_5 - 1)^4 / 12 (5 c_5 - 2) (5 c_5 - 3) (3 - 10 c_5 + 10 c_5^2) \\ b_5&= 1 / 12 c_5 (1 - c_5) (3 - 10 c_5 + 10 c_5^2) \\ b_6&= -(3 - 12 c_5 + 10 c_5^2) / 12 (1 - c_5) (5 c_5 - 2) \\ d_5&= -(1 - c_2) (5 c_5 - 3) (6 - 15 c_2 - 10 c_5 + 30 c_2 c_5) \mathop {\big /} 3 c_5 (3 - 10 c_5 + 10 c_5^2) \\ d_6&= \bigl ( 12 - 52 c_2 + 45 c_2^2 - 5 c_5 (4 - 18 c_2 + 15 c_2^2) \bigr ) (3 - 12 c_5 + 10 c_5^2) \mathop {\big /} 3 (5 c_5 - 2) \\ d_7&= (1 - c_5) \bigl ( 6 - 29 c_2 + 30 c_2^2 - 10 c_5 (1 - 5 c_2 + 5 c_2^2) \bigr ) \end{aligned}$$

See Appendix C for the expressions for p, q, \(c'''_5\), \(c'''_6\), and \(b_6 a_{65} c'''_5\). The whole vector \({{{\varvec{b}}}}\) depends on \(c_5\) only.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Stepanov, M. Embedded (4, 5) pairs of explicit 7-stage Runge–Kutta methods with FSAL property. Calcolo 59, 41 (2022). https://doi.org/10.1007/s10092-022-00486-1

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10092-022-00486-1

Keywords

Mathematics Subject Classification

Search

Navigation