Abstract
We propose a political theory for the slow adoption of technology in sports and other contests. We investigate players’ preferences for new technology that improves contest accuracy. Modeling accuracy as the elasticity of “production” in a standard Tullock contest, we show that players may dislike accuracy if heterogeneity among them is: (1) sufficiently low; (2) moderate when the initial accuracy is low; or (3) high when the initial accuracy is high. We apply our results to the recent adoption of goal-line technology by major European soccer leagues.


Similar content being viewed by others
Notes
According to Deloitte Consulting, in the midst of economic pressures, the European soccer market reached €19.4 billion in revenue terms in 2012, which implies an 11 % growth in 2011/2012. More details can be found at http://www.deloitte.com/ie/en/pages/about-deloitte/articles/european-football-market.html.
Perhaps the most memorable ones are England’s second goal against Germany in the 1966 World Cup Final, Argentina’s first goal against England in the 1986 World Cup, and England’s second goal against Germany in the 2010 World Cup.
More details can be found at http://www.eku.edu/academics/facultysenate/minutes/2003-04/11-03-03/plus_minus_report/final.pdf
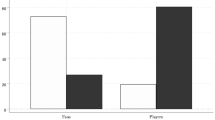
In Fig. 1, the competitive balance measures the dispersion of wins across teams between 1994 and 2010 seasons. Formally, it is the standard deviation of wins. Thus, while 0 implies that the number of wins is the same for all teams, any non-zero number shows the degree of heterogeneity among teams’ number of wins with respect to the mean number of wins. More details can be found at http://www.soccerbythenumbers.com/2011/06/comparing-competitiveness-of-european.html.
If efforts are interpreted as rent-seeking, then the design aims to minimize total effort.
For \(r=1\), it is possible that the high-cost players may drop out of the contest, especially when the number of the low-cost ones is sufficiently large. We ignore this corner case here both because our investigation is about the interaction between the two types of players and because one can take the limit \(r\rightarrow 1\).
As mentioned in the Introduction, Wang (2010) also investigates the impact of contest accuracy in a two-player setting, but his sole focus is on total efforts.
That is, \(\frac{d\pi _{F}^{*}}{dr}\rightarrow 0\) and \(\frac{d\pi _{F}^{*}}{dr}\rightarrow 0~\hbox {as}~N \rightarrow \infty \), which is obvious from the proof of Proposition 5.
In the US, charter schools admit students by lottery. Our result suggests that depending on applicant pools, this may be aligned with parents’ preferences.
“Established in 1887, the Boston Athletic Association is a non-profit organization with a mission of promoting a healthy lifestyle through sports, especially running.” http://www.baa.org/about.aspx.
Formally, replicating the population of players by \(k>1\), note that \(\frac{ kn-1}{km}> \frac{n-1}{m}\), which implies a lower cost threshold in part (a).
See Footnote 4.
For more information, see http://www.independent.co.uk/sport/tennis/djokovic-resists-hawkeye-calls-1990418.html.
A parallel argument can also be made between a contest and a signalling environment where players exert effort to signal their abilities. Focusing on separating equilibrium, a favorite player better differentiates himself under a higher accuracy, \(r\).
See Footnote 15.
References
Alcalde Pérez J, Dahm M (2007) All-pay auction equilibria in contests. Working paper
Amegashie JA (1999) The number of rent-seekers and aggregate rent-seeking expenditures: an unpleasant result. Public Choice 99(1–2):57–62
Amegashie JA (2000) Some results on rent-seeking contests with shortlisting. Public Choice 105(3–4):245–253
Baye MR, Kovenock D, De Vries CG (1993) Rigging the lobbying process: an application of the all-pay auction. Am Econ Rev 83(1):289–294
Che YK, Gale I (1997) Rent dissipation when rent seekers are budget constrained. Public Choice 92(1–2):109–126
Corchón LC (2007) The theory of contests: a survey. Rev Econ Des 11(2):69–100
Dasgupta A, Nti KO (1998) Designing an optimal contest. Eur J Political Econ 14(4):587–603
Dixit A (1987) Strategic behavior in contests. Am Econ Rev 77(5):891–898
Dixon C (2004) Plus/minus grading: if given a choice. Coll Stud J 38(2):280
Glazer A, Hassin R (1988) Optimal contests. Econ Inq 26(1):133–143
Gradstein M (1998) Optimal contest design: volume and timing of rent-seeking in contests. Eur J Political Econ 14(4):575–585
Gradstein M, Konrad KA (1999) Orchestrating rent-seeking contests. Econ J 109(458):536–545
Konrad KA (2009) Strategy and dynamics in contests. Oxford University Press, Oxford
Michaels R (1988) The design of rent-seeking competitions. Public Choice 56(1):17–29
Moldovanu B, Sela A (2001) The optimal allocation of prizes in contests. Am Econ Rev 91(3):542–558
Nti KO (1999) Rent-seeking with asymmetric valuations. Public Choice 98(3–4):415–430
Nti KO (2004) Maximum efforts in contests with asymmetric valuations. Eur J Political Econ 20(4):1059–1066
Szidarovszky F, Okuguchi K (1997) On the existence and uniqueness of pure Nash equilibrium in rent-seeking games. Games Econ Behav 18(1):135–140
Tullock G (1980) Efficient rent seeking. In: Buchanan J, Tollison R, Tullock G (eds) Toward a theory of the rent seeking society. Texas A&M Univ. Press, College Station, pp 97–112
Wang Z (2010) The optimal accuracy level in asymmetric contests. B.E. J Theor Econ 10(1):1–13
Wärneryd K (2003) Information in conflicts. J Econ Theory 110(1):121–136
Wärneryd K (2012) Multi-player contests with asymmetric information. Econ Theory 51(2):277–287
Yildirim H (2005) Contests with multiple rounds. Games Econ Behav 51(1):213–227
Author information
Authors and Affiliations
Corresponding author
Additional information
This paper is based on my dissertation submitted to the Stockholm School of Economics. I am indebted to Karl Wärneryd for his continuous supervision. I thank Tore Ellingsen, Dan Kovenock, Stergios Skaperdas, Huseyin Yildirim, and especially Atila Abdulkadiroglu (the Editor) for comments. I also thank seminar participants at the Istanbul Technical University, the Stockholm School of Economics, and the University of California-Irvine. All errors are mine.
Appendix
Appendix
Proof of Proposition 3
The derivative of \(\theta ^{*}\) with respect to \(r\) is given by
where \(g(.)\) is defined as in (6). Routine algebra yields
Note that (6) implies
Inserting (9) in the numerator and observing that (6) also implies \(mc\theta ^{*}+(n-1)c(\theta ^{*})^{1-r}=(m-1)(\theta ^{*})^{r}+n\), (8) can be rewritten as
Clearly, the numerator has a positive sign because \(\theta ^{*}<1\) by Proposition 1. In contrast, the denominator has a negative sign because \( 0<r<1\) implies
or, employing (9),
Therefore, \(\frac{\partial \theta ^{*}}{\partial r}<0\) by (7), proving part (a). To prove part (b), note that the derivative of \(\pi _{U}^{*}\) with respect to \(r\) is given by
where simple calculations yield
Because, \(0<\theta ^{*}\) if \(0<r<1\) by Proposition 1 and \(1\le m\) by assumption, \(\frac{\partial \pi _{U}^{*}}{\partial r}<0\) and \(\frac{\partial \pi _{U}^{*}}{\partial \theta }>0\). Moreover, \(\frac{\partial \theta ^{*}}{\partial r}<0\) by part (a). Thus, both the first and second terms in (10) have negative signs, proving part (b). \(\square \)
Lemma 2
\(\lim _{r\rightarrow 0}\theta ^{*}=\frac{1}{c}\) and
Proof of Lemma 2
Let \(\theta _{0}^{*}\equiv \lim _{r\rightarrow 0}\theta ^{*}\) and \(\theta _{1}^{*}\equiv \lim _{r\rightarrow 1}\theta ^{*}\). As \(r\rightarrow 0\), (6) reduces to
which gives \(\theta _{0}^{*}=\frac{1}{c}\), as claimed. Likewise, as \(r\rightarrow 1\), (6) simplifies to
Clearly, for \(c\ge \frac{n}{n-1}\), we have \(\theta _{1}^{*}=0\). Suppose that \(c<\frac{n}{n-1}\) and by way of contradiction \(\theta _{1}^{*}=0\). Then, as \(r\rightarrow 1\), \(x_{U}^{*}\rightarrow 0\) and the first-order conditions in (4) reduce to
and
which, together, imply \(c=\frac{n}{n-1}\nless \frac{n}{n-1}\), which, in turn, implies \(\theta ^{*}_{1}\ne 0\). Thus, \(\theta ^{*}_{1}=\frac{n-(n-1)c}{mc-(m-1)}\) for \(c<\frac{ n}{n-1}\), as desired. \(\square \)
Proof of Proposition 4
Using Proposition 1(c), the derivative of \(\pi _{F}^{*}\) with respect to \(r\) can be written as
where \(p_{F}^{*}=\frac{1}{n+m\times (\theta ^{*})^{r}}\) by Proposition 1(a). Noting that \(\frac{dp_{F}^{*}}{dr}=\frac{\partial p_{F}^{*}}{\partial r}+\frac{\partial p_{F}^{*}}{\partial \theta ^{*}}\frac{\partial \theta ^{*}}{\partial r}\) and employing (8), routine algebra yields
Observe that \(\lim _{r\rightarrow 0}p_{F}^{*}=\frac{1}{m+n}\) and \( \lim _{r\rightarrow 0}\frac{dp_{F}^{*}}{dr}=\frac{m\ln c}{(m+n)^{2}}\) because \(\lim _{r\rightarrow 0}\theta ^{*}=\frac{1}{c}\) by Lemma 2. Because, by (11), we have
it follows
This proves part (a) because it readily implies that \(\lim \nolimits _{r \rightarrow 0}\frac{d\pi _{F}^{*}}{dr}<0\) if \(c<e^{1+(n-1)/m}\) and \( \lim \nolimits _{r\rightarrow 0}\frac{d\pi _{F}^{*}}{dr}>0\) if \( c>e^{1+(n-1)/m}\). The same steps in part (a) along with Lemma 2 yield
and
Obviously, \(\lim \nolimits _{r\rightarrow 1}\frac{d\pi _{F}^{*}}{dr}<0\) if\( \ c>\frac{n}{n-1}\). To examine the sign of \(\frac{d\pi _{F}^{*}}{dr}\) for \(c<\frac{n}{n-1}\), let \(f(c)\equiv 2mc\ln (\frac{n-(n-1)c}{mc-(m-1)} )+mc+n\). Observe that \(f(1)=m+n>0\) and \(\lim _{c\rightarrow \frac{n}{n-1} }f(c)=-\infty <0\), which, together with the continuity, implies that there exists \(\widetilde{c}(n,m)\in (1,\frac{n}{n-1})\) such that \(f(\widetilde{c} (n,m))=0\). To show uniqueness, it is enough to show that \(f(c)\) is monotonic over \((1,\frac{n}{n-1})\). To this end, calculate the second derivative to obtain
Observe that \(\tfrac{\partial ^{2}f(c)}{\partial c^{2}}>0\) for \(\frac{ 2n\left( m -1\right) }{1 + m(n - 1) + n(m - 1)}>c\) and \(\tfrac{\partial ^{2}f(c)}{ \partial c^{2}}<0\) for \(\frac{2n\left( m-1\right) }{1+m(n-1)+n(m-1)}<c\). Also, observe that
Therefore, if \(m-1<n\), \(\tfrac{\partial f(c)}{\partial c}\) is monotonically decreasing over \((1,\frac{n}{n-1})\), it thus takes its supremum at \(c=1\). Because
it implies that, if \(m-1<n\), \(\frac{\partial f(c)}{\partial c}<0\) for all \(c\in \) \((1,\frac{n}{n-1})\). If \(m-1>n\), however, \(\frac{\partial f(c)}{ \partial c}\) takes its maximum at \(c=\frac{2n\left( m-1\right) }{ 1+m(n-1)+n(m-1)}\) over \((1,\frac{n}{n-1})\). Because
for \(m-1>n,\) it implies that, if \(m-1>n\), \(\frac{\partial f(c)}{\partial c}<0\) for all \(c\in (1,\frac{n}{n-1})\). As a result, \(f(c)\) is monotonically decreasing over \((1, \frac{n}{n-1})\), which shows that \(\widetilde{c}(n,m)\) is unique. Moreover, as \(f(c)\) is decreasing, referring to (13), this shows
which completes the proof. \(\square \)
Proof of Proposition 5
By (11),
And by definition of total payoff, \(\frac{d(\alpha N\times \pi _{F}^{*})}{dr}=\alpha N\frac{d\pi _{F}^{*}}{dr}\). As \(N\rightarrow \infty \), we therefore have
As \(p_{F}^{*}=\frac{1}{N(\alpha +(\theta ^{*})^{r})}\) from Proposition 1(a), the second limit is
By (12), we have
From here, it follows
Thus,
Next, for \(n=\alpha N\) and \(m=N\), (6) reduces to
From here, as \(\theta ^{*}>0\) for \(r\in (0,1)\), it readily follows that \( \lim _{N\rightarrow \infty }\theta ^{*}=c^{-1/(1-r)}\). Using this, (14) becomes
Using Proposition 1(c) and replicating the same lines of algebra, we also find
It is easy to see that \(\lim _{N\rightarrow \infty }\frac{d(N\times \pi _{U}^{*})}{dr}<0\). To examine the sign of \(\lim _{N\rightarrow \infty } \frac{d(\alpha N\times \pi _{F}^{*})}{dr}\), let \(f(c)\equiv \alpha +c^{- \frac{r}{1-r}}(1-\frac{\ln c}{1-r})\). Obviously, \(\lim _{N\rightarrow \infty } \frac{d(\alpha N\times \pi _{F}^{*})}{dr}\) and \(f(c)\) have the opposite signs. Now, observe that \(f(c)\) takes its unique minimum at \( c=e^{(1-r^{2})/r}\) because
where \(f(e^{\frac{1-r^{2}}{r}})=\alpha -\frac{1}{re^{1+r}}<0\) if \(\alpha < \frac{1}{re^{1+r}}\) and \(f(e^{\frac{1-r^{2}}{r}})>0\) if \(\alpha >\frac{1}{ re^{1+r}}\). Because \(f(1)=\alpha +1>0\), \(\lim _{c\rightarrow \infty }f(c)=\alpha >0\), and \(\lim _{N\rightarrow \infty }\frac{d(\alpha N\times \pi _{F}^{*})}{dr}\) and \(f(c)\) have the opposite signs, it follows
where \(\underline{c}(\alpha ,r)\) and \(\overline{c}(\alpha ,r)\) solve \(f(c)=0\), which completes the proof. \(\square \)
Proof of Proposition 6
As there are \(n\) favorites and \(m\) underdogs, total effort (\(TE\)) is given by \(TE=nx_{F}^{*}+mx_{U}^{*}\). Employing \(\theta ^{*}=\frac{ x_{U}^{*}}{x_{F}^{*}}\) and Proposition 1(a) and 1(b), it can be written explicitly as
or, more conveniently, as
where we have employed that \(\theta ^{*}=\frac{\left( (m-1)(\theta ^{*})^{r}+n\right) (\theta ^{*})^{r}}{(m(\theta ^{*})^{r}+n-1)c}\) by (6) and \(c=\frac{c_{U}}{c_{F}}\) by definition.
As the derivative of \(TE\) with respect to \(r\) is given by
routine algebra, along with Lemma 2, yields
From (15), it is straightforward to see that \( \lim _{r\rightarrow 0}\frac{d(TE)}{dr}>0\), proving part (a). Likewise, if \( c\ge \frac{n}{n-1}\), it is easy to see from (16) that \(\lim _{r\rightarrow 1}\frac{d(TE)}{dr}>0\). Suppose now \(c<\frac{n}{n-1}\) and let \(f(c)\equiv mc+n+mn\left( c-1\right) \ln (\frac{n-(n-1)c}{mc-(m-1)})\) . As all other terms are always positive, the sign of \(\lim \limits _{r \rightarrow 1}\frac{d(TE)}{dr}\) is the same as that of \(f(c)\). Note that \( f(1)=m\) and \(\lim \limits _{c\rightarrow \frac{n}{n-1}}f(c)=-\infty \), which together with the continuity assures that there exists \(\widehat{c}(n,m)\in (1,\frac{n}{n-1})\) such that \(f(\widehat{c}(n,m))=0\). To show uniqueness, note that the first and second derivatives of \(f(c)\) are given as
Obviously, \(f(c)\) is concave as \(\frac{\partial ^{2}f(c)}{\partial c^{2}}<0\), which together with
assures that there exists a unique \(\widehat{c}(n,m)\in (1,\frac{n}{n-1})\) such that \(f(\widehat{c}(n,m))=0\). Moreover, because \(f(1)=m>0\) and the sign of \(\frac{d(TE)}{dr}\) is the same as that of \(f(c)\), we have
which completes the proof. \(\square \)
Proof of Proposition 8
Let \(\pi _{ij}^{*}\) denote the payoff of type \(i\) in a pairwise contest when his opponent is of type \(j\). Also, let \(E[\pi _{i}^{*}]\) denote the expected payoff of type \(i\). Because the opponent is chosen randomly, the underdog’s expected payoff is given by
Differentiating it with respect to \(r\) gives
Note that \(\frac{d\pi _{UF}^{*}}{dr}<0\) by Proposition 2(b) and employing Proposition 1(a) and (c), it immediately follows that \(\frac{d\pi _{UU}^{*}}{dr}=-\frac{V}{4}<0\). Because \(\frac{dE[\pi _{U}^{*}]}{dr}\) is a convex combination of \(\frac{d\pi _{UU}^{*}}{dr}<0\) and \(\frac{d\pi _{UF}^{*}}{dr}<0\), it must be that \(\frac{dE[\pi _{U}^{*}]}{dr}<0\), which proves part (a). To prove part (b), note that the favorite’s expected payoff is given by
Differentiating it with respect to \(r\) yields
where \(\pi _{FF}^{*}=\dfrac{2-r}{4}V\) and \(\pi _{FU}^{*}=\dfrac{ c^{r}(c^{r}-r+1)}{\left( c^{r}+1\right) ^{2}}V\) are obtained by using Proposition 1(a) and (c). Routine algebra yields
Taking now its limit as \(r\rightarrow 0\) gives
Clearly, \(\frac{dE[\pi _{F}^{*}]}{\partial r}<0\) if \(c<e^{1+(n-1)/m}\) and \(\frac{dE[\pi _{F}^{*}]}{\partial r}>0\) if \(c>e^{1+(n-1)/m}\). Finally, taking the limit as \(r\rightarrow 1\) yields
Similarly, \(\lim \limits _{r\rightarrow 1}\frac{\partial (E[\pi _{F}^{*}]) }{\partial r}>0\) if \(\frac{4c(2c\ln c-c-1)}{(c+1)^{3}}>\frac{n-1}{m}\) and \( \lim \limits _{r\rightarrow 1}\frac{\partial (E[\pi _{F}^{*}])}{\partial r} <0\) if \(\frac{4c(2c\ln c-c-1)}{(c+1)^{3}}<\frac{n-1}{m}\). Because \(\frac{ 4c(2c\ln c-c-1)}{(c+1)^{3}}\) has only one critical point over \((1,\infty )\) that turns out to be its maximizer, \(\frac{4c(2c\ln c-c-1)}{(c+1)^{3}}>\frac{ n-1}{m}\) if and only if \(s>\dfrac{n-1}{m}\) and \(\underline{c}(m,n)<c< \overline{c}(m,n)\), where \(s\approx 1.06\) uniquely solves \(\max \limits _{c\in (1,\infty )}\{\frac{4c(2c\ln c-c-1)}{(c+1)^{3}}\}\) and \(\underline{c}(m,n)< \overline{c}(m,n)\) solve \(\frac{4c(2c\ln c-c-1)}{(c+1)^{3}}-\frac{n-1}{m}=0\) . This completes the proof because these findings clearly imply that \( \lim \limits _{r\rightarrow 1}\frac{\partial (E[\pi _{F}^{*}])}{\partial r} >0\) if \(\frac{n-1}{m}<s\) and \(\underline{c}(m,n)\) \(<c<\overline{c}(m,n)\) and \( \lim \limits _{r\rightarrow 1}\frac{\partial (E[\pi _{F}^{*}])}{\partial r} <0\) if otherwise. \(\square \)
Rights and permissions
About this article
Cite this article
Yildirim, M. Accuracy in contests: players’ perspective. Rev Econ Design 19, 67–90 (2015). https://doi.org/10.1007/s10058-015-0166-9
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10058-015-0166-9