Estimation method of the capsizing probability in irregular beam seas using non-Gaussian probability density function

Abstract

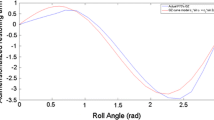
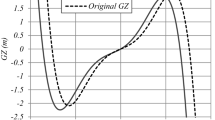
This paper aims to describe a probabilistic assessment technique for ship roll behavior in beam sea conditions. Here, following the work of Kimura and its previous application by the author, probability density function (pdf) of roll response is calculated by combining the moment method with equivalent linearization technique. Results produced using this method are shown to be in good agreement with Monte Carlo simulation results. Moreover, this procedure is extended to the estimation of the capsizing probability. The final results concerning capsizing probability for the linear damping coefficient case are well correlated to the Monte Carlo simulation results. The advantage of this method is that it does not require a significant amount of computation and it enables the direct assessment of capsizing probability for ships with strongly nonlinear restoring terms.

Appendices

Appendix 1

Here, the derivation of Eq. 14 is briefly explained. Consider the following linear differential equation with the excitation term.

$$ \ddot {\phi } + \alpha \dot {\phi } + \omega_{0}^{2} \phi = f(t). $$

(31)

The solution of the above linear differential equation is described using the impulse response function $H_{12}$ and $H_{22}$ as follows [16]:

$$ \begin{aligned} \phi \left( t \right) = \int_{0}^{t} {H_{12} \left( {t - v} \right)\,f\left( v \right)\,{\text{d}}v} + H_{11} \left( t \right)\,\phi \left( 0 \right) + H_{12} \left( t \right)\,\dot {\phi } \left( 0 \right), \hfill \\ \dot {\phi } \left( t \right) = \int_{0}^{t} {H_{22} \left( {t - v} \right)\,f\left( v \right)\,{\text{d}}v} + H_{21} \left( t \right)\,\phi \left( 0 \right) + H_{21} \left( t \right)\,\dot {\phi } \left( 0 \right). \hfill \\ \end{aligned} $$

(32)

Here, putting $\omega_{\text{h}} = \sqrt {\omega_{0}^{2} - \alpha_{\text{e}}^{2} /4}$, the impulse response function and the other terms are described as follows:

$$ \text{H} = \left[ {\begin{array}{*{20}c} {H_{11} } & {H_{12} } \\ {H_{21} } & {H_{22} } \\ \end{array} } \right] = \left[ {\begin{array}{*{20}c} {e^{ - \alpha t/2} \left( {\frac{\alpha }{{2\omega_{\text{h}} }}\sin \omega_{\text{h}} t + \cos \omega_{\text{h}} t} \right)} & {\frac{{e^{ - \alpha t/2} }}{{2\omega_{\text{h}} }}\sin \omega_{\text{h}} t} \\ {\frac{{e^{ - \alpha t/2} \omega_{0}^{2} }}{{2\omega_{\text{h}} }}\sin \omega_{\text{h}} t} & {e^{ - \alpha t/2} \left( {\cos \omega_{\text{h}} t - \frac{\alpha }{{2\omega_{\text{h}} }}\sin \omega_{\text{h}} t} \right)} \\ \end{array} } \right]. $$

(33)

In Eq. 32, integral terms represent the homogeneous solution, whereas the later part represents the particular solution. Now, the stationary solution is necessary for analysis, and in this case, the homogeneous solution does not contribute. Then, the following relation can be obtained:

$$ \begin{aligned} E\left[ {f\left( t \right)\,\phi \left( t \right)} \right] = \mathop {\lim }\limits_{t \to \infty } \int_{0}^{t} {H_{12} \left( {t - v} \right)R\left( {t - v} \right){\text{d}}v} , \\ E\left[ {f\left( t \right)\,\dot {\phi } \left( t \right)} \right] = \mathop {\lim }\limits_{t \to \infty } \int_{0}^{t} {H_{22} \left( {t - v} \right)R\left( {t - v} \right){\text{d}}v} . \\ \end{aligned} $$

(34)

Here, $R\left( {t - v} \right) = E\left[ {f\left( v \right)\,f\left( t \right)} \right]$ is the autocorrelation function of external wave moment. Putting $k \equiv t - v$, then it becomes as follows:

$$ \begin{aligned} \hfill E\left[ {f\left( t \right)\,\phi \left( t \right)} \right] = \int_{0}^{\infty } {H_{12} \left( k \right)R\left( k \right){\text{d}}k} , \\ \hfill E\left[ {f\left( t \right)\,\dot {\phi } \left( t \right)} \right] = \int_{0}^{\infty } {H_{22} \left( k \right)R\left( k \right){\text{d}}k} . \\ \end{aligned} $$

(35)

Appendix 2

Here, the analytical expressions of $E\left[ {f\left( t \right)\,\phi \left( t \right)} \right]$ and $E\left[ {f\left( t \right)\,\dot {\phi } \left( t \right)} \right]$ in Eq. 14 are shown. The functions in integrals are oscillating ones having the following form:

$$ \begin{aligned} \int_{0}^{\infty } {H_{12} \left( k \right)R\left( k \right){\text{d}}k} = \sum\limits_{n} {\int_{0}^{\infty } {a_{n}^{2} \frac{{e^{{ - \alpha_{\text{e}} t/2}} }}{{2\omega_{\text{h}} }}\sin \omega_{\text{h}} t \cdot \cos \omega_{n} t{\text{d}}t\,} } , \\ \int_{0}^{\infty } {H_{22} \left( k \right)R\left( k \right){\text{d}}k} = \sum\limits_{n} {\int_{0}^{\infty } {a_{n}^{2} \frac{{e^{{ - \alpha_{\text{e}} t/2}} }}{{2\omega_{\text{h}} }}\left( {\cos \omega_{\text{h}} t - \frac{{\alpha_{\text{e}} }}{{2\omega_{\text{h}} }}\sin \omega_{\text{h}} t} \right) \cdot \cos \omega_{n} t{\text{d}}t\,} } . \\ \end{aligned} $$

(36)

The accuracy of the value obtained from this equation strongly influences the final results; so, it should be analytically calculated. The results of the integral with respect to time t becomes as follows:

$$ \begin{aligned} \int_{0}^{\infty } {H_{12} \left( k \right)R\left( k \right){\text{d}}k} = \sum\nolimits_{n} {\frac{{a_{n}^{2} \left( {\alpha_{\text{e}}^{ 2} /4 + \omega_{\text{h}}^{2} - \omega^{2} } \right)}}{{\left( {\alpha_{\text{e}}^{ 2} /4 + \omega_{\text{h}}^{2} + \omega^{2} } \right)^{2} - \;4\omega_{\text{h}}^{2} \omega^{2} }}} , \\ \int_{0}^{\infty } {H_{22} \left( k \right)R\left( k \right){\text{d}}k} = \sum\nolimits_{n} {\frac{{\alpha_{\text{e}} a_{n}^{2} \omega^{2} }}{{\left( {\alpha_{\text{e}}^{ 2} /4 + \omega_{\text{h}}^{ 2} + \omega^{2} } \right)^{2} - \;4\omega_{\text{h}}^{ 2} \omega^{2} }}.} \\ \end{aligned} $$

(37)