Skip to main content
SpringerLink
Log in

Force–displacement characteristics of circular-shaped massless elastica

  • Original Paper
  • Published:
Acta Mechanica Aims and scope Submit manuscript

Abstract

The deformation of circular-shaped elasticas, subjected to unilateral constraints due to ground contact, is studied under vertical loading. For C-shaped elasticas, we consider the cases where the elastica rolls without slipping due to the presence of friction and rolls while slipping due to lack of friction. The elastica behaves as a hardening spring in the absence of friction; in the presence of friction, it behaves as a softening or hardening spring depending on boundary conditions. For O-shaped elasticas, we consider symmetric and asymmetric loadings. Under symmetric loading, the stiffness of the elastica decreases with increase in the load while point contact is maintained; thereafter, the stiffness remains nearly constant while the elastica undergoes large deformation and makes line contact. Similar behavior is observed for a symmetric mass-elastica system. Asymmetric loading is studied by assuming that the initial point of contact of the elastica with the ground is fixed; vertical loading results in both vertical and horizontal motion of the point of loading. For the different statics and dynamics problems considered, the deformed shapes of the elasticas are obtained iteratively using an algorithm that solves a series of two-point boundary value problems.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20

Similar content being viewed by others

Notes

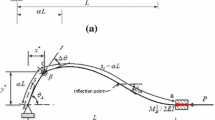
  1. The loading on the line segment may not be uniform; it is implicitly assumed that the line segment does not buckle.

  2. It can be seen from Eq. (3a) that a constant value of \(\theta \) implies \(({\text {d}\theta }/{\text {d}s}) = 0\), which in turn implies that the curvature is constant and equal to zero. It will be seen later that the curvature is constant but not equal to zero for an initially curved elastica.

  3. Note that the horizontal force p (see Fig. 2) is zero due to the absence of friction between the elastica and the ground.

  4. It should be noted that the value of \(q_{\mathrm{cr}}\) is quite sensitive to the tolerance used to distinguish a line contact from a point contact.

  5. Note that the value of \(\alpha \) is determined using the procedure discussed in Sect. 4.1.

  6. Since the bending moments (horizontal forces) at the tip of the right and left VC-LES should be equal and opposite, the bending moments (horizontal forces) at the tip of the right and transformed left VC-LES are equal.

  7. Note that the value of \(\alpha \) has to be determined at each time step using the procedure discussed in Sect. 4.3.

  8. When the natural frequencies are not integer multiples of one another, the trajectory of the mass traces a path that does not repeat itself and is dense in a bounded region of the Cartesian space.

References

  1. Wahl, A.M.: Mechanical Springs. Penton Publishing Company, New York (1944)

    Google Scholar 

  2. Frisch-Fay, R.: Flexible Bars. Butterworths, London (1962)

    MATH  Google Scholar 

  3. Shinohara, A., Hara, M.: Large deflection of a circular C-shaped spring. Int. J. Mech. Sci. 21(3), 179–186 (1979)

    Article  Google Scholar 

  4. Wang, C., Watson, L.: On the large deformations of C-shaped springs. Int. J. Mech. Sci. 22(7), 395–400 (1980)

    Article  Google Scholar 

  5. Srpčič, S., Saje, M.: Large deformations of thin curved plane beam of constant initial curvature. Int. J. Mech. Sci. 28(5), 275–287 (1986)

    Article  Google Scholar 

  6. Wang, C.: Crushing an elastic-perfectly plastic ring between two point loads. Int. J. Non-Linear Mech. 23(3), 205–216 (1988)

    Article  MathSciNet  Google Scholar 

  7. Cannarozzi, M., Molari, L.: Stress-based formulation for non-linear analysis of planar elastic curved beams. Int. J. Non-Linear Mech. 55, 35–47 (2013)

    Article  Google Scholar 

  8. Wu, C.H., Plunkett, R.: On the contact problem of thin circular rings. J. Appl. Mech. 32(1), 11–20 (1965)

    Article  MathSciNet  Google Scholar 

  9. Plaut, R., Klusman, C.: Two-dimensional analysis of stacked geosynthetic tubes on deformable foundations. Thin-Walled Struct. 34(3), 179–194 (1999)

    Article  Google Scholar 

  10. Plaut, R.H., Suherman, S., Dillard, D.A., Williams, B.E., Watson, L.T.: Deflections and buckling of a bent elastica in contact with a flat surface. Int. J. Solids Struct. 36(8), 1209–1229 (1999)

    Article  Google Scholar 

  11. Lu, Z.H., Chen, J.S.: Deformations of a clamped–clamped elastica inside a circular channel with clearance. Int. J. Solids Struct. 45(9), 2470–2492 (2008)

    Article  Google Scholar 

  12. Chau, S., Mukherjee, R.: Kinetic to potential energy transformation using a spring as an intermediary: application to the pole vault problem. J. Appl. Mech. 86(5), 051001 (2019)

    Article  Google Scholar 

  13. Chen, J.S., Ro, W.C.: Deformations and stability of an elastica subjected to an off-axis point constraint. J. Appl. Mech. 77(3), 031006 (2010)

    Article  Google Scholar 

  14. Plaut, R.H., Virgin, L.N.: Deformation and vibration of upright loops on a foundation and of hanging loops. Int. J. Solids Struct. 51(18), 3067–3075 (2014)

    Article  Google Scholar 

  15. Virgin, L.N., Giliberto, J.V., Plaut, R.H.: Deformation and vibration of compressed, nested, elastic rings on rigid base. Thin-Walled Struct. 132, 167–175 (2018)

    Article  Google Scholar 

  16. Schmidt, W.F.: Nonlinear bending of beams using the finite element method. Comput. Struct. 8(1), 153–158 (1978)

    Article  Google Scholar 

  17. Noor, A.K., Peters, J.M.: Penalty finite element formulation for curved elastica. J. Eng. Mech. 110(5), 694–712 (1984)

    Article  Google Scholar 

  18. Golley, B.: The solution of open and closed elasticas using intrinsic coordinate finite elements. Comput. Methods Appl. Mech. Eng. 146(1–2), 127–134 (1997)

    Article  Google Scholar 

  19. Santos, H.: A novel updated Lagrangian complementary energy-based formulation for the elastica problem: force-based finite element model. Acta Mech. 226(4), 1133–1151 (2015)

    Article  MathSciNet  Google Scholar 

  20. Saje, M.: Finite element formulation of finite planar deformation of curved elastic beams. Comput. Struct. 39(3–4), 327–337 (1991)

    Article  Google Scholar 

  21. Fried, I.: Stability and equilibrium of the straight and curved elastica-finite element computation. Comput. Methods Appl. Mech. Eng. 28(1), 49–61 (1981)

    Article  Google Scholar 

  22. Sitar, M., Kosel, F., Brojan, M.: A simple method for determining large deflection states of arbitrarily curved planar elastica. Arch. Appl. Mech. 84(2), 263–275 (2014)

    Article  Google Scholar 

  23. Kierzenka, J., Shampine, L.F.: A BVP solver based on residual control and the Matlab PSE. ACM Trans. Math. Softw. 27(3), 299–316 (2001)

    Article  MathSciNet  Google Scholar 

  24. Press, W.H., Teukolsky, S.A., Vetterling, W.T., Flannery, B.P.: Numerical Recipes 3rd Edition: The Art of Scientific Computing. Cambridge University Press, Cambridge (2007)

    MATH  Google Scholar 

  25. Timoshenko, S.P., Gere, J.M.: Theory of Elastic Stability, 2nd edn. McGraw-Hill, New York (1961)

    Google Scholar 

Download references

Acknowledgements

The first author gratefully acknowledges the support provided by the National Science Foundation graduate research fellowship program, Grant No. DGE-1424871.

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, Michigan State University, East Lansing, MI, USA

    Sheryl Chau & Ranjan Mukherjee

Authors
  1. Sheryl Chau
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ranjan Mukherjee
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Ranjan Mukherjee.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Chau, S., Mukherjee, R. Force–displacement characteristics of circular-shaped massless elastica. Acta Mech 231, 4585–4602 (2020). https://doi.org/10.1007/s00707-020-02766-9

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-020-02766-9

Search

Navigation