Temporal instability of a confined nano-liquid film with the Marangoni convection effect: viscous potential theory

Abstract

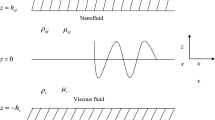
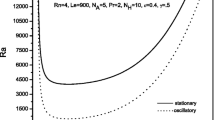
The current paper utilizes the viscous potential theory to analyze the temporal stability of a swirling annulus of two nano gas–liquid layers. The analysis depends mainly on the normal mode technique. The distributions of the heat, volume fraction, and velocity field are achieved. Furthermore, surface tension is considered as a function of the heat and the volume fraction distributions. Therefore, the impact of the Marangoni convection is taken into account. The balance of the normal stress tensor at the interface yields a complicated transcendental dispersion equation. Actually, this equation has no exact solution. Consequently, numerical calculations are established to indicate the relation between the growth rate and the wavenumber of the surface waves. Graphically, the influences of the various physical parameters are depicted. It is found that the Brownian motion accelerates the particles which leads to a destabilization of the interface. Simultaneously, the thermophoresis produces a motion of hot particles from the interface towards the boundaries, which stabilizes the interface. Furthermore, the increase of the surface tension, due to the Marangoni effect, enhances the interface rigidity and stabilizes it.

Appendix

The constants that appearing in Eq. (25) may be listed as follows:

$$ \begin{aligned} & m_{n}^{2} = k^{2} + \left( {\text{Re} \,\Pr } \right)_{j} \left( {\varOmega + i\,k} \right)\,\,,\,\,\,\,\,\,\,\,\,n = 1\,at\,j = \ell ,\,n = 2\,at\,j = g \\ & m_{s}^{2} = k^{2} + \left( {\text{Re} \,\Pr Le} \right)_{j} \left( {\varOmega + i\,k} \right)\,\,\,\,\,\,\,\,\,\,\,\,s = 3\,at\,j = \ell ,\,s = 4\,at\,j = g, \\ \end{aligned} $$

where $\text{Re}_{j} = \frac{{\rho_{j} U_{j} R}}{{\mu_{j} }},\,$ are Reynold’s numbers for liquid and gas phases, and

$\Pr_{j} = \frac{{(c_{p\,} \mu )_{j} }}{{k_{{f_{j} }} }},\,$ are Prandtl numbers for liquid and gas phases.

$Le_{j} = \frac{{k_{{f_{j} }} }}{{(\rho c_{p} )_{j} D_{{B_{j} }} }}$, are Lewis numbers for liquid and gas phases, respectively.

The constants of solutions (28)-(30) are derived by using the boundary conditions (27) as follows:

$$ A_{2} = \frac{i\varOmega }{{kI_{0} (\lambda )}},A_{1} = \frac{i\varOmega }{k}\left( {\frac{{K_{1} (\lambda R_{w} )}}{{I_{0} (\lambda )K_{1} (\lambda R_{w} ) + I_{1} (\lambda R_{w} )K_{0} (\lambda )}}} \right),B_{1} = \frac{i\varOmega }{k}\left( {\frac{{I_{1} (\lambda R_{w} )}}{{I_{0} (\lambda )K_{1} (\lambda R_{w} ) + I_{1} (\lambda R_{w} )K_{0} (\lambda )}}} \right) $$

$$ \begin{aligned} & a_{11} = \,\frac{{T_{w} }}{{I_{0} (m_{1} R_{w} )}} - a_{21} \,\frac{{K_{0} (m_{1} R_{w} )}}{{I_{0} (m_{1} R_{w} )}}\,, \\ & a_{11} \,I_{0} (m_{1} ) + a_{21} \,K_{0} (m_{1} ) = a_{12} \,I_{0} (m_{2} )\,, \\ & k_{{f_{\ell } }} \left( {a_{11} m_{1} I_{1} (m_{1} ) - a_{21} m_{1} \,K_{1} (m_{1} )} \right) = k_{{f_{g} }} \left( {a_{12} m_{2} I_{1} (m_{2} )} \right) \\ \end{aligned} $$

$$ \begin{aligned} & a_{13} I_{0} (m_{3} R_{w} ) + a_{23} \,K_{0} (m_{3} R_{w} ) + F_{1} (R_{w} ) = \,C_{w} \,, \\ & a_{13} I_{0} (m_{3} ) + a_{23} \,K_{0} (m_{3} ) + F_{1} (1) = a_{14} \,I_{0} (m_{4} )\, + F_{2} (1), \\ & D_{{B_{\ell } }} \left( {a_{13} m_{3} I_{1} (m_{1} ) - a_{23} m_{3} \,K_{1} (m_{1} ) + F_{1}^{\backslash } (1)} \right) = D_{{B_{g} }} \left( {a_{14} m_{4} I_{1} (m_{4} ) + F_{2}^{\backslash } (1)} \right) \\ \end{aligned} $$

$$ a_{11} = \frac{{\Delta_{1} }}{{\Delta_{T} }},\quad a_{21} = \frac{{\Delta_{2} }}{{\Delta_{T} }},\quad a_{12} = \frac{{\Delta_{3} }}{{\Delta_{T} }}, $$

$$ \Delta_{T} = \left| {\begin{array}{*{20}c} {I_{0} (m_{1} R_{w} )} & {K_{0} (m_{1} R_{w} )} & 0 \\ {I_{0} (m_{1} )} & {K_{0} (m_{1} )} & { - I_{0} (m_{2} )} \\ {k_{f}^{*} m_{1} I_{1} (m_{1} )} & { - k_{f}^{*} m_{1} K_{1} (m_{1} )} & { - m_{2} I_{1} (m_{2} )} \\ \end{array} } \right|, $$

$$ \begin{aligned} & \Delta_{1} = \left| {\begin{array}{*{20}c} {T_{w} } & {K_{0} (m_{1} R_{w} )} & 0 \\ 0 & {K_{0} (m_{1} )} & { - I_{0} (m_{2} )} \\ 0 & { - k_{f}^{*} m_{1} K_{1} (m_{1} )} & { - m_{2} I_{1} (m_{2} )} \\ \end{array} } \right|,\quad \Delta_{2} = \left| {\begin{array}{*{20}c} {I_{0} (m_{1} R_{w} )} & {T_{w} } & 0 \\ {I_{0} (m_{1} )} & 0 & { - I_{0} (m_{2} )} \\ {k_{f}^{*} m_{1} I_{1} (m_{1} )} & 0 & { - m_{2} I_{1} (m_{2} )} \\ \end{array} } \right| \\ & \Delta_{3} = \left| {\begin{array}{*{20}c} {I_{0} (m_{1} R_{w} )} & {K_{0} (m_{1} R_{w} )} & {T_{w} } \\ {I_{0} (m_{1} )} & {K_{0} (m_{1} )} & 0 \\ {k_{f}^{*} m_{1} I_{1} (m_{1} )} & { - k_{f}^{*} m_{1} K_{1} (m_{1} )} & 0 \\ \end{array} } \right| \\ \end{aligned} $$

$$ a_{13} = \frac{{\Delta_{5} }}{{\Delta_{C} }},\quad a_{23} = \frac{{\Delta_{6} }}{{\Delta_{C} }},\quad a_{14} = \frac{{\Delta_{7} }}{{\Delta_{C} }}, $$

where

$$ \Delta_{C} = \left| {\begin{array}{*{20}c} {I_{0} (m_{3} R_{w} )} & {K_{0} (m_{3} R_{w} )} & 0 \\ {I_{0} (m_{3} )} & {K_{0} (m_{3} )} & { - I_{0} (m_{4} )} \\ {D_{B}^{*} m_{3} I_{1} (m_{3} )} & { - D_{B}^{*} m_{3} K_{1} (m_{3} )} & { - m_{4} I_{1} (m_{4} )} \\ \end{array} } \right|, $$

$$ \begin{aligned} & \Delta_{5} = \left| {\begin{array}{*{20}c} {I_{0} (m_{3} R_{w} )} & {C_{w} - F_{1} (R_{w} )} & 0 \\ {I_{0} (m_{3} )} & 0 & { - I_{0} (m_{4} )} \\ {D_{B}^{*} m_{3} I_{1} (m_{3} )} & 0 & { - m_{4} I_{1} (m_{4} )} \\ \end{array} } \right|,\quad \Delta_{6} = \left| {\begin{array}{*{20}c} {I_{0} (m_{3} R_{w} )} & {K_{0} (m_{1} R_{w} )} & {C_{w} - F_{1} (R_{w} )} \\ {I_{0} (m_{3} )} & {K_{0} (m_{1} )} & 0 \\ {D_{B}^{*} m_{3} I_{1} (m_{3} )} & { - D_{B}^{*} m_{3} K_{1} (m_{3} )} & 0 \\ \end{array} } \right| \\ & \Delta_{7} = \left| {\begin{array}{*{20}c} {C_{w} - F_{1} (R_{w} )} & {K_{0} (m_{3} R_{w} )} & 0 \\ 0 & {K_{0} (m_{3} )} & { - I_{0} (m_{4} )} \\ 0 & { - D_{B}^{*} m_{3} K_{1} (m_{3} )} & { - m_{4} I_{1} (m_{4} )} \\ \end{array} } \right|, \\ \end{aligned} $$