Convergence rates for linear elasticity systems on perforated domains

Abstract

In the present work, we established almost-sharp error estimates for linear elasticity systems in periodically perforated domains. The first result was $L^{\frac{2d}{d-1-\tau }}$-error estimates $O\big (\varepsilon ^{1-\frac{\tau }{2}}\big )$ for all $\tau \in (0,1)$ in a bounded smooth domain, which is new even for homogenization problems on unperforated domains. It followed from weighted Hardy–Sobolev’s inequalities (given by Lehrbäck and Vähäkangas in J Funct Anal 271(2):330–364, 2016) and a suboptimal error estimate for the square function of the first-order approximating corrector (earliest investigated by Kenig et al. in Arch Ration Mech Anal 203(3):1009–1036, 2012) under additional regularity assumption on coefficient). The new approach relied on the weighted quenched Calderón–Zygmund estimate (initially appeared in Gloria et al. work Milan J Math 88(1):99–170, 2020 for a quantitative stochastic homogenization theory). The second effort was $L^2$-error estimates $O\big (\varepsilon ^{\frac{5}{6}} \ln ^{\frac{2}{3}}(1/\varepsilon )\big )$ for a Lipschitz domain, followed from a duality scheme coupled with interpolation inequalities. Also, we developed a new weighted extension theorem and local-type Sobolev–Poincaré inequalities on perforated domains. Throughout the paper, we do not impose any smoothness assumption on the coefficients.

Appendix

Lemma 8.1

(Caccioppoli’s inequality with nonvanishing boundary data) Let $\varOmega$ be a Lipschitz domain and $0<r\le 1$. Suppose ${\mathcal {L}}$ is an elliptic operator with a constant coefficient and satisfies the condition (1.4). Let v be the solution to ${\mathcal {L}}(v) = 0$ in $D_{2r}$ and $v = g$ on $\varDelta _{2r}$ with $g\in H^1(\varDelta _{2r};{\mathbb {R}}^d)$. Then we have

$$\begin{aligned} \Big (\fint _{D_r}|\nabla v|^2dx\Big )^{1/2} \lesssim \frac{1}{r}\bigg \{\Big (\fint _{D_{2r}}|v|^2dx\Big )^{1/2} + \Big (\fint _{\varDelta _{2r}}|g|^2dS\Big )^{1/2} \bigg \} + \Big (\fint _{\varDelta _{2r}} |\nabla _{tan }g|^2dS\Big )^{1/2}, \end{aligned}$$

(8.1)

where the constant depends on $\mu _0,\mu _1,d$ and the boundary character of $\varOmega$.

Proof

By rescaling argument it is fine to assume $r=1$. Let ${\tilde{g}}$ be the extension of g such that ${\tilde{g}} = g$ on $\varDelta _{2}$ with ${\tilde{g}}\in H_0^1(\varDelta _3)$, and $\Vert {\tilde{g}}\Vert _{H^1(\partial D_3)}\lesssim \Vert g\Vert _{H^1(\varDelta _{2})}$. Then we construct an auxiliary function G satisfying

$$\begin{aligned} {\mathcal {L}}(G) = 0 \quad \text {in} \quad D_3, \qquad G={\tilde{g}} \quad \text {on}\quad \partial D_3. \end{aligned}$$

Then there holds

$$\begin{aligned} \begin{aligned}&\Vert G\Vert _{L^2(D_3)} \lesssim \Vert \nabla G\Vert _{L^2(D_3)} \lesssim \Vert {\tilde{g}}\Vert _{H^{1/2}(\partial D_3)} \lesssim \Vert {\tilde{g}}\Vert _{H^1(\partial D_3)} \lesssim \Vert g\Vert _{H^1(\varDelta _2)}, \end{aligned} \end{aligned}$$

(8.2)

where we use the fact that $G = 0$ on $\partial D_3{\setminus }\varDelta _3$ in the first inequality. Let $w = v-G$, and then ${\mathcal {L}}(w) = 0$ in $D_2$ and $w=0$ on $\varDelta _2$, it follows from the estimate similar to (7.1) that

$$\begin{aligned} \Vert \nabla w\Vert _{L^2(D_1)} \lesssim \Vert w\Vert _{L^2(D_2)}, \end{aligned}$$

and therefore we have

$$\begin{aligned} \begin{aligned} \Vert \nabla v\Vert _{L^2(D_1)}&\le \Vert \nabla w\Vert _{L^2(D_1)} + \Vert \nabla G\Vert _{L^2(D_3)} \\&\lesssim \Vert w\Vert _{L^2(D_2)} + \Vert \nabla G\Vert _{L^2(D_3)} \lesssim \Vert v\Vert _{L^2(D_2)} + \Vert g\Vert _{H^1(\varDelta _2)}, \end{aligned} \end{aligned}$$

where we use the estimate (8.2) in the last step. Then, by rescaling back, there holds the desired estimate (8.1) and we have completed the proof. $\square$

Theorem 8.1

(weighted $W^{1,2}$ estimates for mixed boundary conditions) Let $\omega _{\sigma }=\delta _{\varOmega }^{\sigma }$ with $\delta _{\varOmega }(x)=dist (x,\partial \varOmega )$, $\sigma \in (-1,1).$ Assume that $\varOmega$ is a bounded Lipschitz domain, and $\partial \varOmega =(\partial \varOmega )_D\cup (\partial \varOmega )_N$ with $(\partial \varOmega )_D\cap (\partial \varOmega )_N=\emptyset$ satisfies the same geometry conditions as in [12, Theorem 1.5]. Suppose that ${\bar{a}}$ is a constant coefficient matrix satisfying the ellipticity and symmetry conditions (1.4). Let u be associated with f by

$$\begin{aligned} \left\{ \begin{aligned} \nabla \cdot {\bar{a}}\nabla u&= \nabla \cdot f&\quad&in ~~\varOmega ,\\ \partial u/\partial \nu&= -n\cdot f&\quad&on ~~ (\partial \varOmega )_N,\\ u&= 0&\quad&on ~~ (\partial \varOmega )_D, \end{aligned}\right. \end{aligned}$$

(8.3)

in which $\partial u/\partial \nu :=n\cdot {\bar{a}}\nabla u$ is the conormal derivative of u. Then, there holds the weighted estimate

$$\begin{aligned} \Big (\int _{\varOmega }|\nabla u|^2\omega _{\sigma } dx\Big )^{1/2} \lesssim \Big (\int _{\varOmega }|f|^2\omega _{\sigma } dx\Big )^{1/2}, \end{aligned}$$

(8.4)

where the up to constant depends on $\mu _0, \mu _1, p, d$ and the boundary character of $\varOmega$.

Proof

The main idea is actually parallel to that given for Theorem 1.4, and for simplicity, we employ a new scheme of shen’s recent work [44] as we have mentioned in Remark 6.1. We just give the proof in the case of $\sigma \in (-1,0)$, while the other case follows from the duality argument.

Let $B=B(x_0,r)$ with $4B\subset \varOmega$ or $x_0\in \partial \varOmega$, $D_{4r}=4B\cap \varOmega$. For the ease of the statement, we impose the following notations: $\varDelta _{4r}^N:=(\partial \varOmega )_N\cap \partial D_{4r}$, $\varDelta _{4r}^D:=(\partial \varOmega )_D \cap \partial D_{4r}$. The whole proof is divided into two parts.

Step 1. We will show that there exists $p>2$ such that

$$\begin{aligned} \Big (\fint _{D_{r}}|\nabla u|^p\omega _{\sigma }^{p/2}dx\Big )^{1/p} \lesssim \fint _{D_{2r}}|\nabla u|\omega _{\sigma }^{1/2}dx, \end{aligned}$$

(8.5)

provided u satisfies $\nabla \cdot {\bar{a}}\nabla u = 0$ in $D_{4r}$, and $\partial u/\partial \nu = 0$ on $\varDelta _{4r}^N$ with $u = 0$ on $\varDelta _{4r}^D$. Based upon the Rellich’s estimate [12, Theorem 1.5] (we remark that the Rellich’s estimate comes from Rellich’s identity, which is still valid for elliptic systems with symmetry coefficients), for $t\in (1,2)$, one may derive that

$$\begin{aligned} \begin{aligned} \Big (\int _{\partial D_{tr}} |(\nabla u)^{*}|^{2}dS\Big )^{1/2}&\lesssim \Big (\int _{\partial D_{tr}{\setminus }\varDelta _{4r}} |\nabla u|^{2}dS\Big )^{1/2} +\Big (\int _{\varDelta _{tr}^D} |\nabla _{\text {tan}} u|^{2}dS\Big )^{1/2} \\&\quad +\Big (\int _{\varDelta _{tr}^N} \Big |\frac{\partial u}{\partial \nu }\Big |^2 dS\Big )^{1/2}\\&\lesssim \Big (\int _{\partial D_{tr}{\setminus }\varDelta _{4r}} |\nabla u|^{2}dS\Big )^{1/2}, \end{aligned} \end{aligned}$$

(8.6)

where the last inequality is due to the assumptions on the boundary data. Then, by squaring and integrating both sides of (8.6) with respect to $t\in (1,2)$, we obtain

$$\begin{aligned} \int _{\varDelta _{r}}|(\nabla u)^*|^{2}dS \lesssim \frac{1}{r}\int _{D_{2r}} |\nabla u|^{2}dx. \end{aligned}$$

(8.7)

Hence, we have

$$\begin{aligned} \begin{aligned} \fint _{D_r}|\nabla u|^2\omega _{\sigma }dx&\lesssim r^{1+\sigma -d}\int _{\varDelta _r}|(\nabla u)^*|^2dS+r^{\sigma } \fint _{D_{2r}}|\nabla u|^2dx\\&\lesssim ^{(8.7)} r^{\sigma }\fint _{D_{2r}}|\nabla u|^2dx \lesssim \fint _{D_{2r}}|\nabla u|^2dx\Big (\fint _{B}\omega _{\sigma }\Big ). \end{aligned} \end{aligned}$$

(8.8)

According to the fact that $\Big (\fint _{D_{2r}}|\nabla u|^2dx\Big )^{1/2}\lesssim \fint _{D_{2r}}|\nabla u|dx$ which follows from reverse Hölder’s inequality and a convexity argument(see [23, pp.173]), we have

$$\begin{aligned} \Big (\fint _{D_r}|\nabla u|^2\omega _{\sigma }dx\Big )^{1/2}\lesssim \Big (\fint _{D_{2r}}|\nabla u|dx\Big )\Big (\fint _{B}\omega _{\sigma }\Big )^{1/2} \lesssim \fint _{D_{2r}}|\nabla u|\omega _{\sigma }^{1/2}dx, \end{aligned}$$

(8.9)

and in the last inequality we use the following property of $A_1$ weight: for any ball $B\subset {\mathbb {R}}^d$, it holds that

$$\begin{aligned} \fint _{B}\omega _{\sigma }\le C \inf _{B}\omega _{\sigma }. \end{aligned}$$

(8.10)

Due to the self-improvement property of the above inequality (8.9), there exists $p>2$ such that

$$\begin{aligned} \Big (\fint _{D_{r}}|\nabla u|^p\omega _{\sigma }^{p/2}dx\Big )^{1/p} \lesssim \fint _{D_{2r}}|\nabla u|\omega _{\sigma }^{1/2}dx. \end{aligned}$$

Step 2. The weighted real arguments lead to weighted $W^{1,2}$ estimates (8.4). To do so, we decompose the equation (8.3) as follows:

$$\begin{aligned} (\text {i})\left\{ \begin{aligned} \nabla \cdot {\bar{a}}\nabla v&= \nabla \cdot (I_{4B}f)&\quad&\text {in}~~\varOmega ,\\ \partial v/\partial \nu&= -n\cdot (I_{4B}f)&\quad&\text {on}~~ (\partial \varOmega )_N,\\ v&= 0&\quad&\text {on}~~ (\partial \varOmega )_D, \end{aligned}\right. \qquad (\text {ii})\left\{ \begin{aligned} \nabla \cdot {\bar{a}}\nabla w&= \nabla \cdot g&\quad&\text {in}~~\varOmega ,\\ \partial w/\partial \nu&= -n\cdot g&\quad&\text {on}~~ (\partial \varOmega )_N,\\ w&= 0&\quad&\text {on}~~ (\partial \varOmega )_D, \end{aligned}\right. \end{aligned}$$

(8.11)

where $g:=(1-I_{4B})f$. Hence, it is not hard to see that $u=v+w$. On the one hand, from the equation $(\text {i})$ one may have, there exists a $1<p_0<2$ such that

$$\begin{aligned} \Big (\fint _{B\cap \varOmega }|\nabla v|^{p_0}dx\Big )^{1/p_0} \lesssim \Big (\fint _{4B\cap \varOmega }|f|^{p_0}dx\Big )^{1/p_0}, \end{aligned}$$

(8.12)

where we employ the Meyer’s estimate for the solution of $(\text {i})$ and $p_0$ is close to 2. One the other hand, it is known by Step 1 that w in $(\text {ii})$ satisfies the estimate

$$\begin{aligned} \begin{aligned} \Big (\fint _{B\cap \varOmega }|\nabla w|^p\omega _{\sigma }^{p/2}dx\Big )^{1/p}&\lesssim \fint _{2B\cap \varOmega }|\nabla w|\omega _{\sigma }^{1/2}dx\\&\lesssim \Big (\fint _{2B\cap \varOmega }|\nabla w|^{p_0}dx\Big )^{1/p_0} \Big (\fint _{B}\omega _{\sigma }\Big )^{1/2}, \end{aligned} \end{aligned}$$

(8.13)

in the last inequality we employ Hölder’s inequality and the property of $A_p$ weight (6.17). Then it follows that

$$\begin{aligned} \begin{aligned}&\Big (\fint _{B\cap \varOmega }|\nabla w|^p\omega _{\sigma }dx\Big )^{1/p} \Big (\fint _{B}\omega _{\sigma }\Big )^{\frac{1}{2}-\frac{1}{p}} \\&\quad \lesssim ^{(8.10)} \Big (\fint _{B\cap \varOmega }|\nabla w|^p\omega _{\sigma }^{p/2}dx\Big )^{1/p}\\&\quad \lesssim ^{(8.13)} \Big (\fint _{2B\cap \varOmega }|\nabla w|^{p_0}dx\Big )^{1/p_0} \Big (\fint _{B}\omega _{\sigma }\Big )^{1/2} \\&\quad \lesssim \Bigg (\Big (\fint _{2B\cap \varOmega }|\nabla u|^{p_0}dx \Big )^{1/p_0} + \Big (\fint _{2B\cap \varOmega }|\nabla v|^{p_0}dx \Big )^{1/p_0}\Bigg ) \Big (\fint _{B}\omega _{\sigma }\Big )^{1/2}\\&\quad \lesssim ^{(8.12)} \Bigg (\Big (\fint _{2B\cap \varOmega }|\nabla u|^{p_0}dx \Big )^{1/p_0} + \Big (\fint _{4B\cap \varOmega }|f|^{p_0}dx\Big )^{1/p_0}\Bigg ) \Big (\fint _{B}\omega _{\sigma }\Big )^{1/2}. \end{aligned} \end{aligned}$$

Namely, it holds that

$$\begin{aligned} \Big (\fint _{B\cap \varOmega }|\nabla w|^p\omega _{\sigma }dx\Big )^{1/p}\lesssim \Bigg (\Big (\fint _{2B\cap \varOmega }|\nabla u|^{p_0}dx \Big )^{1/p_0} + \Big (\fint _{4B\cap \varOmega }|f|^{p_0}dx\Big )^{1/p_0}\Bigg ) \Big (\fint _{B}\omega _{\sigma }\Big )^{1/p}. \end{aligned}$$

(8.14)

Thus, the estimates (8.12) and (8.14) together with [44, Theorem 4.1] (weighted real methods) lead to

$$\begin{aligned} \int _{\varOmega }|\nabla u|^{2}\omega _{\sigma }dx \lesssim \Big (\int _{\varOmega }|\nabla u|^2dx\Big )\Big (\fint _{\varOmega }\omega _{\sigma }\Big ) + \int _{\varOmega }|f|^2\omega _{\sigma }dx \lesssim \int _{\varOmega }|f|^2\omega _{\sigma }dx, \end{aligned}$$

where the last inequality follows from energy estimate and (8.10). This completes the proof of (8.4). $\square$

Theorem 8.2

(layer & co-layer type estimates) Let $\varOmega \subset {\mathbb {R}}^d$ be a bounded Lipschitz domain and $0<\varepsilon <1$ small. Given $F\in L^2(\varOmega ;{\mathbb {R}}^d)$ and $g\in H^1(\partial \varOmega ; {\mathbb {R}}^d)$, let $u_0$ be associated with F and g by the equation (1.6), where the coefficient ${\widehat{A}}$ satisfies the elasticity condition (2.1). Then there hold the following estimates.

• 1. $L^p$ estimates:

  $$\begin{aligned} \Vert \nabla u_0\Vert _{L^{\frac{2d}{d-1}}(\varOmega )} \lesssim \Big \{\Vert F\Vert _{L^{\frac{2d}{d+1}}(\varOmega )} +\Vert g\Vert _{H^1(\partial \varOmega )}\Big \}. \end{aligned}$$

  (8.15)

• 2. Radial maximal function estimates

  $$\begin{aligned} \Vert \mathrm {M}_{r }(\nabla u_0)\Vert _{L^{2}(\partial \varOmega )} \lesssim \Big \{\Vert F\Vert _{L^{2}(\varOmega )} +\Vert g\Vert _{H^1(\partial \varOmega )}\Big \}, \end{aligned}$$

  (8.16)

  where the operator $\mathrm {M}_{r }$ is defined by (1.31).

• 3. Layer type estimates

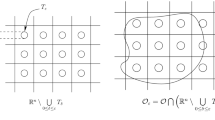
  $$\begin{aligned} \begin{aligned} \Vert \nabla u_{0}\Vert _{L^{2}(O_{4\varepsilon })}&\lesssim \varepsilon ^{\frac{1}{2}}\Big \{\Vert g\Vert _{H^{1}(\partial \varOmega )} +\Vert F\Vert _{L^{2}(\varOmega )}\Big \},\\ \Big (\int _{O_{4\varepsilon }} |\nabla u_{0}|^{2} \delta dx\Big )^{\frac{1}{2}}&\lesssim \varepsilon \Big \{\Vert g\Vert _{H^{1}(\partial \varOmega )} +\Vert F\Vert _{L^{2}(\varOmega )}\Big \}. \end{aligned} \end{aligned}$$

  (8.17)

• 4. Co-layer type estimates

  $$\begin{aligned} \begin{aligned} \Vert \nabla ^2 u_{0}\Vert _{L^{2}(\varOmega \backslash O_{4\varepsilon })}&\lesssim \varepsilon ^{-\frac{1}{2}} \Big \{\Vert g\Vert _{H^{1}(\partial \varOmega )}+ \Vert F\Vert _{L^{2}(\varOmega )}\Big \},\\ \Big (\int _{\varOmega \backslash O_{4\varepsilon }} |\nabla ^{2} u_{0}|^{2} \delta dx\Big )^{\frac{1}{2}}&\lesssim \ln ^{\frac{1}{2}}(1/\varepsilon ) \Big \{\Vert g\Vert _{H^{1}(\partial \varOmega )} +\Vert F\Vert _{L^{2}(\varOmega )}\Big \},\\ \Big (\int _{\varOmega \backslash O_{4\varepsilon }} |\nabla u_{0}|^{2}\delta ^{-1}dx\Big )^{\frac{1}{2}}&\lesssim \ln ^{\frac{1}{2}}(1/\varepsilon ) \Big \{\Vert g\Vert _{H^{1}(\partial \varOmega )} +\Vert F\Vert _{L^{2}(\varOmega )} \Big \}. \end{aligned} \end{aligned}$$

  (8.18)

The up to constants depend only on ${\widehat{\mu }}_{0}$, ${\widehat{\mu }}_{1}$, d and the boundary character of $\varOmega$.

Remark 8.1

To the authors’ best knowledge, the first line of the estimates (8.17) and (8.18) were originally investigated by Shen [43, Theorems 2.6], while the second author generalized his arguments to the weighted type estimates (see [53, Lemma 4.5]).

Proof

Here we merely show the proofs of (8.15) and (8.16) by using a similar idea given for the estimates (8.17) and (8.18), which is inspired by [43, Theorems 2.6] as we have mentioned in Remark 8.1.

Firstly, we decompose $u_{0}$ into two parts: v and w, which satisfy the following systems:

$$\begin{aligned} -\nabla \cdot ({\widehat{A}}\nabla v)={\tilde{F}} \qquad \text {in} \quad {\mathbb {R}}^d, \end{aligned}$$

(8.19)

and

$$\begin{aligned} \left\{ \begin{aligned} -\nabla \cdot ({\widehat{A}}\nabla w)&=0&\qquad&\text {in~} \varOmega ,\\ w&=g-v&\qquad&\text {on~}\partial \varOmega , \end{aligned} \right. \end{aligned}$$

(8.20)

respectively. Let ${\tilde{F}}$ be a zero-extension of F, satisfying ${\tilde{F}}=F$ in $\varOmega$ and ${\tilde{F}}=0$ in ${\mathbb {R}}^d\backslash \varOmega$. In terms of (8.19), by the well-known fractional integral estimates and singular integral estimates for v (see for example [49]), we have that

$$\begin{aligned} \begin{aligned} \Vert \nabla v\Vert _{L^{p'}({\mathbb {R}}^d)}+ \Vert \nabla ^2 v\Vert _{L^p({\mathbb {R}}^d)}\le C\Vert {\tilde{F}}\Vert _{L^p({\mathbb {R}}^d)} \qquad \text {for~} 1<p<d, \quad \frac{1}{p'}=\frac{1}{p}-\frac{1}{d}. \end{aligned} \end{aligned}$$

(8.21)

It follows from the divergence theorem that

$$\begin{aligned} \begin{aligned} \int _{\partial \varOmega }|\nabla v|^2dS&\le C\bigg [ \int _{\varOmega }|\nabla v|^2dx+\int _{\varOmega }|\nabla v||\nabla ^2 v|dx\bigg ]\\&\lesssim \Vert \nabla v\Vert ^{2}_{L^{\frac{2d}{d-1}}(\varOmega )} +\Vert \nabla ^2 v\Vert ^{2}_{L^{\frac{2d}{d+1}}(\varOmega )}, \end{aligned} \end{aligned}$$

(8.22)

where we use Hölder’s inequality. Then we turn to the equation (8.20). It follows from the nontangential maximal function estimates for $L^2$-regular problem in Lipschitz domains (see [18]) that

$$\begin{aligned} \begin{aligned} \Vert (\nabla w)^{*}\Vert _{L^{2}(\partial \varOmega )}&\le C \bigg (\Vert \nabla _{\text {tan}}g\Vert _{L^{2}(\partial \varOmega )} +\Vert \nabla _{\text {tan}}v\Vert _{L^{2}(\partial \varOmega )}\bigg )\\&\lesssim \Vert g\Vert _{H^{1}(\partial \varOmega )} +\Vert \nabla v\Vert _{L^{2}(\partial \varOmega )}, \end{aligned} \end{aligned}$$

where $(\nabla w)^{*}$ denotes the nontangential maximal function of $\nabla w$ (see (1.32) for its definition). On account of (8.22), we have

$$\begin{aligned} \begin{aligned} \Vert (\nabla w)^{*}\Vert _{L^{2}(\partial \varOmega )}&\lesssim \Vert g\Vert _{H^{1}(\partial \varOmega )}+\Vert \nabla v\Vert _{L^{\frac{2d}{d-1}}(\varOmega )} +\Vert \nabla ^2 v\Vert _{L^{\frac{2d}{d+1}}(\varOmega )}\\&\lesssim ^{(8.21)}\Vert g\Vert _{H^{1}(\partial \varOmega )} +\Vert F\Vert _{L^{\frac{2d}{d+1}}(\varOmega )}. \end{aligned} \end{aligned}$$

(8.23)

Secondly, for any $(u)^{*}\in L^2(\partial \varOmega )$, the fractional integral coupled with a duality argument gives the following inequality

$$\begin{aligned} \Vert u\Vert _{L^{\frac{2d}{d-1}}(\varOmega )} \lesssim \Vert (u)^{*}\Vert _{L^2(\partial \varOmega )} \end{aligned}$$

(8.24)

(see for example [34, Remark 9.3]). In terms of the radial maximal function estimates, it follows from [54, Lemma 2.24] that

$$\begin{aligned} \Vert \mathrm {M}_{\text {r}}(u)\Vert _{L^2(\partial \varOmega )} \lesssim \Vert u\Vert _{H^1(\varOmega )}. \end{aligned}$$

(8.25)

Consequently, based upon the decomposition $u_0 = v+w$ above, there hold

$$\begin{aligned} \begin{aligned} \Vert \nabla u_0\Vert _{L^{\frac{2d}{d-1}}(\varOmega )}&\le \Vert \nabla v\Vert _{L^{\frac{2d}{d-1}}(\varOmega )} + \Vert \nabla w\Vert _{L^{\frac{2d}{d-1}}(\varOmega )}\\&\lesssim ^{(8.21),(8.24)} \Vert F\Vert _{L^{\frac{2d}{d+1}}(\varOmega )} + \Vert (\nabla w)^*\Vert _{L^{2}(\partial \varOmega )}\\&\lesssim ^{(8.23)} \Vert F\Vert _{L^{\frac{2d}{d+1}}(\varOmega )} + \Vert g\Vert _{H^1(\partial \varOmega )}, \end{aligned} \end{aligned}$$

and

$$\begin{aligned} \begin{aligned} \Vert \mathrm {M}_{\text {r}}(\nabla u_0)\Vert _{L^{2}(\partial \varOmega )}&\le \Vert \mathrm {M}_{\text {r}}(\nabla v)\Vert _{L^{2}(\partial \varOmega )} + \Vert \mathrm {M}_{\text {r}}(\nabla w)\Vert _{L^{2}(\partial \varOmega )}\\&\lesssim ^{(8.25)} \Vert \nabla v\Vert _{H^{1}(\varOmega )} + \Vert (\nabla w)^*\Vert _{L^{2}(\partial \varOmega )}\\&\lesssim ^{(8.21),(8.23)} \Vert F\Vert _{L^{2}(\varOmega )} + \Vert g\Vert _{H^1(\partial \varOmega )}, \end{aligned} \end{aligned}$$

where we note the fact that $\mathrm {M}_{\text {r}}(\nabla w)(Q) \le (\nabla w)^*(Q)$ for a.e. $Q\in \partial \varOmega$. This ends the proof. $\square$

Remark 8.2

If $\partial \varOmega \in C^1$, then, for any $1<q<\infty$, the solution w to (8.20) owns the nontangential maximal function estimates $\Vert (\nabla w)^*\Vert _{L^q(\partial \varOmega )} \lesssim \Vert \nabla w\Vert _{L^q(\partial \varOmega )}$. Thus, for $2\le p < \frac{2d}{d-2}$, there holds

$$\begin{aligned} \Vert \nabla u_0\Vert _{L^{p}(\varOmega )} \lesssim \Big \{\Vert F\Vert _{L^{2}(\varOmega )} +\Vert g\Vert _{W^{1,{\bar{p}}}(\partial \varOmega )}\Big \}, \end{aligned}$$

(8.26)

where ${\bar{p}}=p-p/d$, and the proof is the same as that given for (8.15), and so is omitted.