Abstract
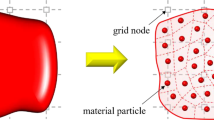
The material point method (MPM) suffers from poor accuracy and suboptimal convergence rates compared to other numerical methods due to the under-integration of the weak form; the locations of material points with respect to the background grid are suboptimal in performing numerical quadrature. Although this approach enables the MPM to model large deformation efficiently, it also results in the loss of Galerkin exactness in the variational equation and possible stress oscillation due to the cell-crossing instability. This paper introduces a novel MPM formulation that employs the reproducing kernel approximation to overcome the cell-crossing instability due to the higher-order continuity employed. The reproducing kernel method also ensures completeness in the approximation. In addition, this paper implements a variationally consistent material point integration scheme into the MPM framework to address the issue of Galerkin exactness, which is shown to recover theoretical convergence and increase the robustness of the formulation. Numerical examples demonstrate that the proposed method recovers optimal accuracy and stability compared to the conventional approaches and removes spurious pressure oscillation. The F-bar stabilization method of overcoming pressure instability is then coupled with the presented formulation to demonstrate its ability to accurately model incompressible materials.




















Similar content being viewed by others
References
Chen J-S, Hillman M, Sheng-Wei C (2017) Meshfree methods: progress made after 20 years. J Eng Mech 143(4):04017001
Wu C, Chen J-S, Chi L, Huck F (2001) Lagrangian meshfree formulation for analysis of geotechnical materials. J Eng Mech 127(5):440–449
Rabczuk T, Samaniego E (2008) Discontinuous modelling of shear bands using adaptive meshfree methods. Comput Methods Appl Mech Eng 197(6):641–658
Duan Q, Li X, Zhang H, Belytschko T (2012) Second-order accurate derivatives and integration schemes for meshfree methods. Int J Numer Meth Eng 92(4):399–424
Wang H-P, Wu C-T, Chen J-S (2014) A reproducing kernel smooth contact formulation for metal forming simulations. Comput Mech 54(1):151–169
Li S, Qian D, Liu WK, Belytschko T (2001) A meshfree contact-detection algorithm. Comput Methods Appl Mech Eng 190(24):3271–3292
Sherburn JA, Roth MJ, Chen J, Hillman M (2015) Meshfree modeling of concrete slab perforation using a reproducing kernel particle impact and penetration formulation. Int J Impact Eng 86:96–110
Rabczuk T, Belytschko T (2004) Cracking particles: a simplified meshfree method for arbitrary evolving cracks. Int J Numer Meth Eng 61(13):2316–2343
Rabczuk T, Zi G, Bordas S, Nguyen-Xuan H (2010) A simple and robust three-dimensional cracking-particle method without enrichment. Comput Methods Appl Mech Eng 199(37):2437–2455
Sulsky D, Chen Z, Schreyer HL (1994) A particle method for history-dependent materials. Comput Methods Appl Mech Eng 118(1–2):179–196
Bardenhagen SG, Brackbill JU, Sulsky D (2000) The material-point method for granular materials. Comput Methods Appl Mech Eng 187(3–4):529–541
Chen Z, Brannon R (2002) an evaluation of the material point method. SAND Report, SAND2002-0482
Andersen L, Andersen S (2010) Modelling of landslides with the material-point method. Comput Geosci 14(1):137–147
Zhang F, Zhang X, Sze K, Lian Y, Liu Y (2017) Incompressible material point method for free surface flow. J Comput Phys 330:92–110
Sulsky D, Schreyer H, Peterson K, Kwok R, Coon M (2007) Using the material-point method to model sea ice dynamics. J Geophys Res Atmos. https://doi.org/10.1029/2005JC003329
Bardenhagen SG, Kober EM (2004) The generalized interpolation material point method. Comput Model Eng Sci 5(6):477–496
Moutsanidis G, Long CC, Bazilevs Y (2020) IGA-MPM: the isogeometric material point method. Comput Methods Appl Mech Eng 372:113346
Steffen M, Kirby R, Berzins M (2008) Analysis and reduction of quadrature errors in the material point method (MPM). Int J Numer Meth Eng 76:922–948
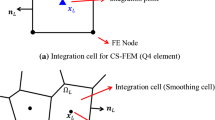
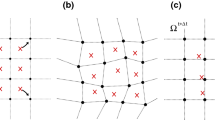
Chen J-S, Hillman M, Rüter M (2013) An arbitrary order variationally consistent integration for Galerkin meshfree methods. Int J Numer Meth Eng 95:387–418
Huang T-H, Wei H, Chen J-S, Hillman M (2019) RKPM2D: an open-source implementation of nodally integrated reproducing kernel particle method for solving partial differential equations. Comput Part Mech 7:393–433
Huang T-H, Chao C-L (2022) A stabilized one-point integrated mixed formulation for finite element and meshfree methods in modeling nearly incompressible materials. Acta Mech 233(3):1147–1172
Sulsky D, Gong M (2016) Improving the material-point method. In: Weinberg K, Pandolfi A (eds) Innovative numerical approaches for multi-field and multi-scale problems. Springer, Cham
de Vaucorbeil A, Nguyen VP, Sinaie S, Wu JY (2020) Material point method after 25 years: theory, implementation and applications. Adv Appl Mech 53:185–398
He L, Gan Y, Chen Z (2019) Preliminary effort in developing the smoothed material point method for impact. Comput Part Mech 6(1):45–53
He L, Chen Z (2019) Study on one-dimensional softening with localization via integrated MPM and SPH. Comput Part Mech 6(4):629–636
Sadeghirad A, Brannon RM, Burghardt J (2011) A convected particle domain interpolation technique to extend applicability of the material point method for problems involving massive deformations. Int J Numer Meth Eng 86(12):1435–1456
Sadeghirad A, Brannon R, Guilkey J (2013) Second-order convected particle domain interpolation (CPDI2) with enrichment for weak discontinuities at material interfaces. Int J Numer Meth Eng 95:928–952
Nguyen VP, Nguyen CT, Rabczuk T, Natarajan S (2017) On a family of convected particle domain interpolations in the material point method. Finite Elem Anal Des 126:50–64
Wang L, Coombs W, Augarde C, Cortis M, Charlton T, Brown M, Knappett J, Brennan A, Davidson C, Richards D, Blake A (2019) On the use of domain-based material point methods for problems involving large distortion. Comput Methods Appl Mech Eng 355:1003–1025
de Vaucorbeil A, Nguyen VP, Hutchinson CR (2020) A Total-Lagrangian Material Point Method for solid mechanics problems involving large deformations. Comput Methods Appl Mech Eng 360:112783
Moutsanidis G, Li W, Bazilevs Y (2021) Reduced quadrature for FEM, IGA and meshfree methods. Comput Methods Appl Mech Eng 373:113521
Hillman M, Chen J-S (2016) An accelerated, convergent, and stable nodal integration in Galerkin meshfree methods for linear and nonlinear mechanics. Int J Numer Meth Eng 107(7):603–630
Chen J-S, Wu C-T, Yoon S, You Y (2001) A stabilized conforming nodal integration for Galerkin mesh-free methods. Int J Numer Meth Eng 50(2):435–466
Huang T-H (2023) Stabilized and variationally consistent integrated meshfree formulation for advection-dominated problems. Comput Methods Appl Mech Eng 403:115698
Chen J, Hu W, Puso M, Wu Y, Zhang X (2007) Strain smoothing for stabilization and regularization of Galerkin Meshfree methods. In: Griebel M, Schweitzer MA (eds) Meshfree methods for partial differential equations III. Springer, Berlin
Chen J-S, Hillman M, Chi S-W (2017) Meshfree methods: progress made after 20 years. J Eng Mech 143(4):04017001
Liu WK, Jun S, Zhang YF (1995) Reproducing kernel particle methods. Int J Numer Meth Fluids 20(8–9):1081–1106
Liu WK, Jun S, Li S, Adee J, Belytschko T (1995) Reproducing kernel particle methods for structural dynamics. Int J Numer Meth Eng 38(10):1655–1679
Zhang L, Gerstenberger A, Wang X, Liu WK (2004) Immersed finite element method. Comput Methods Appl Mech Eng 193(21):2051–2067
Nakamura K, Matsumura S, Mizutani T (2023) Taylor particle-in-cell transfer and kernel correction for material point method. Comput Methods Appl Mech Eng 403:115720
Hu Y, Fang Y, Ge Z, Qu Z, Zhu Y, Pradhana A, Jiang C (2018) A moving least squares material point method with displacement discontinuity and two-way rigid body coupling. ACM Trans Gr 37(4):1–14
Cortis M, Coombs W, Augarde C, Charlton T (2018) Overcoming volumetric locking in material point methods. Comput Methods Appl Mech Eng 333:1–21
Wallstedt PC, Guilkey JE (2007) Improved Velocity Projection for the Material Point Method. Comput Model Eng Sci 19(3):223–232
Jiang C, Schroeder C, Selle A, Teran J, Stomakhin A (2015) The affine particle-in-cell method. ACM Trans Gr 34(4):1–10
Chi S-W, Siriaksorn T, Lin S-P (2017) Von Neumann stability analysis of the u–p reproducing kernel formulation for saturated porous media. Comput Mech 59(2):335–357
Zhang DZ, Ma X, Giguere PT (2011) Material point method enhanced by modified gradient of shape function. J Comput Phys 230(16):6379–6398
Nakamura K, Matsumura S, Mizutani T (2022) Taylor particle-in-cell transfer and kernel correction for material point method. Comput Methods Appl Mech Eng 403:115720
Wang D, Zhang H (2014) A consistently coupled isogeometric–meshfree method. Comput Methods Appl Mech Eng 268:843–870
Zhang H, Wang D (2017) Reproducing kernel formulation of B-spline and NURBS basis functions: a meshfree local refinement strategy for isogeometric analysis. Comput Methods Appl Mech Eng 320:474–508
Wallstedt P, Guilkey J (2008) An evaluation of explicit time integration schemes for use with the generalized interpolation material point method. J Comput Phys 227(22):9628–9642
Nitsche J (1971) Über ein Variationsprinzip zur Lösung von Dirichlet-Problemen bei Verwendung von Teilräumen, die keinen Randbedingungen unterworfen sind. Abh Math Semin Univ Hambg 36:9–15
Fernández-Méndez S, Huerta A (2004) Imposing essential boundary conditions in mesh-free methods. Comput Methods Appl Mech Eng 193(12):1257–1275
Wu J, Wang D (2021) An accuracy analysis of Galerkin meshfree methods accounting for numerical integration. Comput Methods Appl Mech Eng 375:113631
Beuth L, Wieckowski Z, Vermeer P (2010) Solution of quasi-static large-strain problems by the material point method. Int J Numer Anal Meth Geomech 35:1451–1465
Gan Y, Sun Z, Chen Z, Zhang X, Liu Y (2017) Enhancement of the material point method using B-spline basis functions. Int J Numer Meth Eng 113:411–431
Bardenhagen S, Brydon A, Guilkey J (2005) Insight into the physics of foam densification via numerical simulation. J Mech Phys Solids 53(3):597–617
Brydon A, Bardenhagen S, Miller E, Seidler G (2005) Simulation of the densification of real open-celled foam microstructures. J Mech Phys Solids 53(12):2638–2660
Sinaie S, Ngo TD, Kashani A, Whittaker AS (2019) Simulation of cellular structures under large deformations using the material point method. Int J Impact Eng 134:103385
Bardenhagen S, Guilkey J, Roessig K, Brackbill J, Witzel W, Foster J (2001) An improved contact algorithm for the material point method and application to stress propagation in granular material. Comput Model Eng Sci 2(4):509–522
Nairn J, Bardenhagen S, Smith G (2018) Generalized contact and improved frictional heating in the material point method. Comput Part Mech 5(3):285–296
Karabelas E, Haase G, Plank G, Augustin CM (2020) Versatile stabilized finite element formulations for nearly and fully incompressible solid mechanics. Comput Mech 65(1):193–215
Geuzaine C, Remacle J-F (2009) Gmsh: a 3-D finite element mesh generator with built-in pre- and post-processing facilities. Int J Numer Meth Eng 79(11):1309–1331
Zeng Z, Zhang H, Zhang X, Liu Y, Chen Z (2022) An adaptive peridynamics material point method for dynamic fracture problem. Comput Methods Appl Mech Eng 393:114786
Bazilevs Y, Moutsanidis G, Bueno J, Kamran K, Kamensky D, Hillman MC, Gomez H, Chen JS (2017) A new formulation for air-blast fluid–structure interaction using an immersed approach: part II—coupling of IGA and meshfree discretizations. Comput Mech 60(1):101–116
Moutsanidis G, Kamensky D, Chen J, Bazilevs Y (2018) Hyperbolic phase field modeling of brittle fracture: Part II—immersed IGA–RKPM coupling for air-blast–structure interaction. J Mech Phys Solids 121:114–132
Acknowledgements
This work was supported by the National Science and Technology Council (formerly Ministry of Science and Technology, MOST), Taiwan, under project contract number 111-2628-E-007-016. The author would like to appreciate the anonymous reviewers for their comments and suggestions for improving the quality of this work.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The corresponding authors report no conflict of interest.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix
Appendix
The results of the curve fitting problem of Eq. (24) show that the GIMP formulation is able to satisfy the linear reproducing condition when the uniform particle distribution is employed, but not for the non-uniform particle distribution. This can be explained by deriving the constraint necessary for GIMP to satisfy the linear reproducing condition, as follows. We first start by plugging the GIMP shape function into the completeness condition of Eq. (23):
where \({\widetilde{\Psi }}_{I}\left({\varvec{x}}\right)\) represents the GIMP shape function. Within the context of the MPM, and implementing the particle domain averaging approach of Eq. (25), the completeness condition is then defined as:
which, using commutativity and associativity of the summation, is redefined as follows:
GIMP employs finite element shape functions, which satisfy linear completeness. Therefore, if we set \(\alpha =1\) for linear completeness, Eq. (51) is simplified as:
The above constraint to achieve linear completeness can be interpreted as a statement that the weighted average of all smoothing points \({{\varvec{x}}}_{q}\) should be equal to the particle location \({{\varvec{x}}}_{p}\). Therefore, as long as the smoothing points \({{\varvec{x}}}_{q}\) and weights \({w}_{q}\) are chosen carefully such that Eq. (52) is satisfied, the GIMP shape functions can satisfy the linear reproducing condition.
For the curve fitting problem of Sect. 2.2, it can be shown that only the particle domain averaging scheme used for the uniform discretization satisfies the constraint in Eq. (52), whereas the scheme used for the non-uniform discretization does not. Therefore, the same curve fitting problem of Eq. (24) is then repeated, to show that GIMP shape functions can satisfy linear completeness such that additional measures are taken to satisfy the constraint. Here, we recalculate the weights \({w}_{q}\) under the constraint that \(\sum_{q\in {S}_{p}}{w}_{q}={V}_{p}\) in order to satisfy Eq. (52). The particle domains used are also conforming. The results for the linear exact solution of Eq. (30) are shown below in Table
5, where it is seen that the GIMP shape functions are able to reproduce the exact solution to machine precision for each mesh employed.
The convergence test results for the exponential exact solution of Eq. (31) are shown below in Fig.
21, and are again compared with results for the RK approximation as well as cubic B-splines. Results demonstrate that GIMP can recover near optimal convergence rates for both the uniform and non-uniform particle distributions, and its accuracy approaches the RK approximation result.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Rodriguez, C., Huang, TH. A variationally consistent reproducing kernel enhanced material point method and its applications to incompressible materials. Comput Mech 73, 599–618 (2024). https://doi.org/10.1007/s00466-023-02381-0
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00466-023-02381-0