An improved Yuan–Agrawal method with rapid convergence rate for fractional differential equations

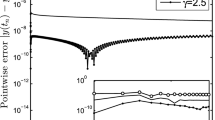
Abstract

Due to the merit of transforming fractional differential equations into ordinary differential equations, the Yuan and Agrawal method has gained a lot of research interests over the past decade. In this paper, this method is improved with major emphasis on enhancing the convergence rate. The key procedure is to transform fractional derivative into an improper integral, which is integrated by Gauss–Laguerre quadrature rule. However, the integration converges slowly due to the singularity and slow decay of the integrand. To solve these problems, we reproduce the integrand to circumvent the singularity and slow decay simultaneously. With the reproduced integrand, the convergence rate is estimated to be no slower than $\, O(n^{ - 2} )$ with $n$ as the number of quadrature nodes. In addition, we utilize a generalized Gauss–Laguerre rule to further improve the accuracy. Numerical examples are presented to validate the rapid convergence rate of the improved method, without causing additional computational burden compared to the original approach.

Appendix: Determination of initial conditions for general system of Eq. (26)

Consider a more general form of Eq. (26)

$$ D^{\lambda } z = Az + f(t),\quad z(0) = z_{0} \quad (0 < \lambda < 1) $$

(A1)

where $z = [z_{1} ,z_{2} , \ldots ,z_{r} ]^{T}$ is the r-dimensional unknown vector and A denotes a matrix of dimension $r \times r$. For simplicity, we assume that $f(t)$ at t = 0 can be expanded as

$$ f(t) = f(0) + \sum\limits_{i = 1}^{s} {c_{i} t^{{\gamma_{i} }} } + o(t) $$

(A2)

herein $0 < \gamma_{1} < \cdots < \gamma_{s} \le 1$, and $o(t)$ denotes higher order terms. To determine initial condition $z^{'} (0)$, we need to estimate the asymptotic behavior of $z$ as $t$ approaches 0. To do so, we approximate the solution of Eq. (A1) as [5]

$$ z(t) = z(0) + \sum\limits_{l = 1}^{L} {d_{l} t^{l\lambda } } + \sum\limits_{i = 1}^{S} {\bar{c}_{i} t^{{\lambda + \gamma_{i} }} } + o(t) $$

(A3)

where the coefficients are $\bar{c}_{i} = \frac{B(\beta ,1 - \alpha )\beta }{\Gamma (1 - \alpha )}c_{i}$ with $B(\beta ,1 - \alpha )$ as the Beta function being defined as $B(\beta ,1 - \alpha ) = \int_{0}^{1} {x^{\beta - 1} (1 - x)^{1 - \alpha - 1} } dx$, and $d_{1} = \lambda \Gamma (\lambda )(Az(0) + f(0))$ and $d_{l + 1} = \frac{(l + 1)\lambda B((l + 1)\lambda ,1 - \lambda )}{\Gamma (1 - \lambda )}d_{l}$ with $l = 1,2, \ldots ,L - 1$.

Note that, there is singularity in $z^{'} (0)$ according to Eq. (A3) as long as there are fractional powers lower than $t$. Before applying the improved method, we should first eliminate the singularity. Assume all the fractional powers lower than $t$ in (A3) are $\sum\limits_{l = 1}^{{L_{1} }} {d_{l} t^{l\lambda } } + \sum\limits_{i = 1}^{{S_{1} }} {\bar{c}_{i} t^{{\lambda + \gamma_{i} }} }$ where $L_{ 1} \lambda < 1\le (L_{ 1} + 1)\lambda$ and $\lambda + \gamma_{{S_{ 1} }} < 1 \le \lambda + \gamma_{{S_{ 1} + 1}}$. Introduce a transformation $y(t) = z(t) - z(0) - \sum\limits_{l = 1}^{{L_{1} }} {d_{l} t^{l\lambda } } - \sum\limits_{i = 1}^{{S_{1} }} {\bar{c}_{i} t^{{\lambda + \gamma_{i} }} }$ to Eq. (A1), we have

$$ D^{\lambda } y = Ay + f_{1} (t),\quad y(0) = 0 $$

(A4)

where $f_{1} (t) = d_{{L_{1} }} t^{{L_{1} \lambda }} + c_{{S_{1} }} t^{{\gamma_{{S_{1} }} }} + {\text{higher terms}}$ which yields a solution being approximated as

$$ y(t) = \bar{d}_{{L_{1} }} t^{{(L_{1} + 1)\lambda }} + \bar{c}_{{S_{1} }} t^{{\gamma_{{S_{1} }} + \lambda }} + o(t) $$

(A5)

Based on Eq. (A5), $y^{{\prime }} (0)$ can be determined as: (1) $y^{{\prime }} (0) = \bar{d}_{{L_{1} }} + \bar{c}_{{S_{1} }}$ if $(L_{ 1} + 1)\lambda = \gamma_{{S_{1} }} + \lambda = 1$; or (2) $y^{{\prime }} (0) = \bar{d}_{{L_{1} }}$ if $(L_{ 1} + 1)\lambda = 1$ and $\gamma_{{S_{1} }} + \lambda > 1$; or (3) $y^{{\prime }} (0) = \bar{c}_{{S_{1} }}$ if $(L_{ 1} + 1)\lambda > 1$ and $\gamma_{{S_{1} }} + \lambda = 1$; otherwise (4) $y^{{\prime }} (0) = 0$ if $(L_{ 1} + 1)\lambda > 1$ and $\gamma_{{S_{1} }} + \lambda > 1$. After determining the initial condition $y^{{\prime }} (0)$, the improved method is employed to solve Eq. (A4) rather than (A1).

As for Eq. (26) with $f(t) = \frac{1}{\Gamma (2 - \lambda )}t^{ 1- \lambda } + t + o(t)$ and $z(0) = 0$, by using the above procedures we obtain the initial condition as $z^{{\prime }} (0) = 1$.