Experimental studies and two-dimensional modelling of a packed bed bioreactor used for production of galacto-oligosaccharides (GOS) from milk whey

Abstract

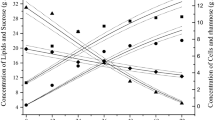
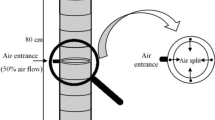
In the present study, extensive experimental investigations and detailed theoretical analysis of a two-dimensional packed bed bioreactor, employed for the production of galacto-oligosaccharides (GOS) from milk whey were performed. Model equations, in one- and two-dimensions, capable of predicting the substrate concentration distribution in the bioreactor were developed by coupling mass balance equation with appropriate velocity distribution equation and solved numerically. Validation of the proposed model equations was done by a set of experimental data obtained from the bioreactor. The effects of reactor to catalyst particle diameter ratio (d _t/d _p), feed flowrate (10⁻⁶–10⁻⁹ m³ s⁻¹), and initial lactose concentration (50–200 kg m⁻³) on substrate concentration distribution were investigated in detail. While, the distribution of substrate concentration in axial direction was independent of d _t/d _p, it was observed that for d _t/d _p <40, significant radial concentration distribution existed. It was further observed that the substrate conversion and product yield obtained experimentally showed an excellent agreement (97 ± 2 %) with the results predicted by the two-dimensional model equation, whereas, the results predicted by the one-dimensional model equation did not lie within the desired confidence level (<90 %). The results were confirmed by both curve fitting and statistical analysis. The prediction of substrate concentration distribution in axial and radial directions using the developed two-dimensional model equation is necessary for computing the bioreactor volume to achieve the desired GOS yield.

Appendix

The governing differential Eq. (15a) along with the boundary conditions given by Eqs. (16)–(19) was used for predicting concentration distribution in the axial and the radial directions. The equation was solved by finite difference method through the method of lines outlined as follows:

One chooses N + 1 equispaced finite-difference grid lines, $r_{1}^{*} ( = 0), \, r_{2}^{*} , \, r_{3}^{*} , \ldots ,r_{N + 1}^{*} ( = R/d_{\text{p}} )$ with the spacing being $\Delta r^{*} = (R/d_{\text{p}} )/N$. Here N = 6 and the value of x _A at the ith grid line are denoted by $x_{A} \left( {r_{i}^{*} ,z} \right) = x_{Ai} \left( z \right)$; $i = 1,2, \ldots N + 1$. The partial derivatives in r may be written as:

$$ \frac{{\partial x_{A} }}{\partial r*}|_{i} = \frac{{x_{A,i + 1} (z) - x_{A,i - 1} (z)}}{{2\Delta r^{*} }} + O[(\Delta r^{*} )^{2} ] $$

(25)

$$ \frac{{\partial^{2} x_{A} }}{{\partial r*^{2} }}|_{i} = \frac{{x_{A,i + 1} (z) - 2x_{A,i} (z) + x_{A,i - 1} (z)}}{{(\Delta r^{*} )^{2} }} + O[(\Delta r^{*} )^{2} ] $$

(26)

It is implied here that the value of z used for all the x _A,i ’s is the same. The residuals can now be equated to zero at the appropriate grid lines for Eq. (15a).

This leads to:

$$ \begin{aligned} \frac{1}{{Pe_{\text{L}} }}\frac{{{\text{d}}^{2} x_{A} }}{{{\text{d}}z^{*2} }} - \frac{{{\text{d}}x_{A} }}{{{\text{d}}z^{*} }} = - \frac{1}{{Pe_{\text{R}} }}\left[ {\frac{{x_{A,i + 1} (z^{*} ) - 2x_{A,i} (z^{*} ) + x_{A,i - 1} (z^{*} )}}{{(\Delta r*)^{2} }} + \frac{1}{{r_{i}^{*} }}\frac{{x_{A,i + 1} (z^{*} ) - x_{A,i - 1} (z^{*} )}}{{2\Delta r^{*} }}} \right] - \hfill \\ {\kern 1pt} {\kern 1pt} \quad \frac{{k_{\text{cat}} C_{E0} (1 - x_{A} )(1 - \varepsilon )d_{p} }}{{u(r_{i} )\varepsilon C_{A0} \left[ {\frac{{K_{\text{m}} }}{{C_{A0} }} + (1 - x_{A} )} \right]}} \hfill \\ \end{aligned} $$

(27)

where, $i = 1,2, \ldots N + 1.$

Here, the velocity profile $u(r_{i}^{*} )$ is given by Eq. (10).

At $r^{*} = r_{1}^{*}$, the boundary condition on x _A gives:

$$ \frac{{x_{A,2} (z) - x_{A,0} (z)}}{{2(\Delta r)}} = 0 $$

(28)

where, $r^{*} = r_{0}^{*}$ is a hypothetical grid line, at which conversion is x _A (z). x _A (z) can be eliminated by making the residual of the PDE at $r^{*} = r_{1}^{*}$ equal to zero, which leads to:

$$ \frac{1}{{Pe_{\text{L}} }}\frac{{\partial^{2} x_{A} }}{{\partial z^{*2} }} - \frac{{\partial x_{A} }}{{\partial z^{*} }} = - \frac{1}{{Pe_{\text{R}} }}\left[ {\frac{{\partial^{2} x_{A} }}{{\partial r^{*2} }} + \frac{1}{{r^{*} }}\frac{{\partial x_{A} }}{{\partial r^{*} }}} \right]_{{r^{*} = r_{1}^{*} }} - \frac{{k_{\text{cat}} C_{E0} (1 - x_{A} )(1 - \varepsilon )d_{\text{p}} }}{{u(r_{i} )\varepsilon C_{A0} \left[ {\frac{{K_{\text{m}} }}{{C_{A0} }} + (1 - x_{A} )} \right]}}|_{{r_{i} = r_{1} }} = 0 $$

(29)

The term $\frac{1}{{r^{*} }}\frac{{\partial x_{A} }}{{\partial r^{*} }}$ gives 0/0 at r = r ₁ = 0. L′Hospital’s rule applied to this term reduces it to $\frac{{\partial^{2} x_{A} }}{{\partial r^{*2} }}$. Thus, the terms in bracket on the right hand side become $2\frac{{\partial^{2} x_{A} }}{{\partial r^{*2} }}$. Using the finite difference form, one gets:

$$ \frac{1}{{Pe_{\text{L}} }}\frac{{{\text{d}}^{2} x_{A,1} }}{{{\text{d}}z^{*2} }} - \frac{{{\text{d}}x_{A,1} }}{{{\text{d}}z^{*} }} = - \frac{1}{{Pe_{\text{R}} }} \left [\frac{{2(x_{A,2} - 2x_{A,1} + x_{A,0} )}}{{(\Delta r^{*2} )}} \right ] - \frac{{k_{\text{cat}} C_{E0} (1 - x_{A,1} )(1 - \varepsilon )d_{\text{p}} }}{{u(r_{i} )\varepsilon C_{A0} \left[ {\frac{{K_{\text{m}} }}{{C_{A0} }} + (1 - x_{A,1} )} \right]}}|_{{r_{i} = r_{1} }} $$

(30)

which upon rearranging becomes:

$$ \frac{1}{{Pe_{\text{L}} }}\frac{{{\text{d}}^{2} x_{A,1} }}{{{\text{d}}z^{*2} }} - \frac{{{\text{d}}x_{A,1} }}{{{\text{d}}z^{*} }} = - \frac{1}{{Pe_{\text{R}} }}\left[ {\frac{{4(x_{A,2} - x_{A,1} )}}{{(\Delta r^{*2} )}}} \right] - \frac{{k_{\text{cat}} C_{E0} (1 - x_{A,1} )(1 - \varepsilon )d_{\text{p}} }}{{u(r_{i} )\varepsilon C_{A0} \left[ {\frac{{K_{\text{m}} }}{{C_{A0} }} + (1 - x_{A,1} )} \right]}}|_{{r_{i} = r_{1} }} $$

(31)

For $i = 2, \ldots N,$ Eq. (27) reduces to:

$$ \frac{1}{{Pe_{\text{L}} }}\frac{{{\text{d}}^{2} x_{A,i} }}{{{\text{d}}z^{*2} }} - \frac{{{\text{d}}x_{A,i} }}{{{\text{d}}z^{*} }} = - \frac{1}{{Pe_{\text{R}} }}\left[ {\frac{{2(x_{A,i + 1} - 2x_{A,i} + x_{A,i - 1} )}}{{(\Delta r^{*2} )}}} \right] - \frac{{k_{\text{cat}} C_{E0} (1 - x_{A,i} )(1 - \varepsilon )d_{\text{p}} }}{{u(r_{i} )\varepsilon C_{A0} \left[ {\frac{{K_{\text{m}} }}{{C_{A0} }} + (1 - x_{A,i} )} \right]}}|_{{r_{i} = 2, \ldots N}}$$

(32)

At $r^{*} = r_{N + 1}^{*} = R/d_{\text{p}}$, Eq. (27) becomes:

$$ \frac{1}{{Pe_{\text{L}} }}\frac{{{\text{d}}^{2} x_{A,N + 1} }}{{{\text{d}}z^{*2} }} - \frac{{{\text{d}}x_{A,N + 1} }}{{{\text{d}}z^{*} }} = - \frac{1}{{Pe_{\text{R}} }}\left[ {\frac{{2(x_{A,N + 2} - 2x_{A,N + 1} + x_{A,N} )}}{{(\Delta r^{*2} )}}} \right] - \frac{{k_{\text{cat}} C_{E0} (1 - x_{A,N + 1} )(1 - \varepsilon )d_{\text{p}} }}{{u(r_{i} )\varepsilon C_{A0} \left[ {\frac{{K_{\text{m}} }}{{C_{A0} }} + (1 - x_{A,N + 1} )} \right]}}|_{{r_{i} = r_{N + 1} }} $$

(33)

Because of the no slip boundary condition at the wall, (r _N+1 = R), a region very close to the wall has been considered to be r _N+1,where the velocity is very small but has a non-zero value.

The set of PDEs represented by Eq. (28) has thus reduced to a set of second order ordinary differential equations under the (ODE-BVPs) in z direction as given by Eqs. (31)–(33). One chooses M + 1 equispaced finite-difference grid points $z_{1}^{*} ( = 0), \, z_{2}^{*} , \, z_{3}^{*} , \ldots ,z_{M + 1}^{*} ( = L/d_{\text{p}} )$ with the spacing being $\Delta z^{*} = \left( {L/d_{\text{p}} } \right)/M$, where M = 19.

For $r^{*} = r_{1}^{*}$, Eq. (31) may be expressed as:

$$ \frac{1}{{Pe_{\text{L}} }}\frac{{{\text{d}}^{2} x_{A,1} }}{{{\text{d}}z^{*2} }} - \frac{{{\text{d}}x_{A,1} }}{{{\text{d}}z^{*} }} + \frac{1}{{Pe_{\text{R}} }}\left[ {\frac{{4(x_{A,2} - x_{A,1} )}}{{(\Delta r^{*2} )}}} \right] = - \frac{{k_{\text{cat}} C_{E0} (1 - x_{A,1} )(1 - \varepsilon )d_{\text{p}} }}{{u(r_{i} )\varepsilon C_{A0} \left[ {\frac{{K_{\text{m}} }}{{C_{A0} }} + (1 - x_{A,1} )} \right]}}|_{{r_{i} = r_{1} = 0}} $$

(34)

At $z^{*} = 0$, the boundary condition represented by Eq. (16) reduces to:

$$ u_{c} x_{A,11} = \frac{{(D_{\text{e}} )_{\text{L}} }}{{d_{\text{p}} }}\frac{{{\text{d}}x_{A,11} }}{{{\text{d}}z^{*} }} = \frac{{(D_{\text{e}} )_{\text{L}} }}{{d_{\text{p}} }}\frac{{(x_{A,12} - x_{A,10} )}}{{2\Delta z^{*} }} $$

(35)

Here, in x _A,11, the first subscript 1 signifies the radial position $r_{1}^{*}$ and the second subscript 1 signifies the axial position $z_{1}^{*}$, corresponding to $r_{1}^{*}$.

Hence, substituting the boundary condition (35) in equation (34) and rearranging, one gets:

$$ \begin{aligned} \left[ { - \frac{2}{{Pe_{\text{L}} (\Delta z^{*} )^{2} }} - \frac{{2u_{c} d_{\text{p}} }}{{Pe_{\text{L}} D_{\text{eL}} (\Delta z^{*} )}} - \frac{{u_{c} d_{\text{p}} }}{{D_{\text{eL}} }} - \frac{4}{{(\Delta r^{*} )^{2} Pe_{\text{R}} }}} \right]x_{A,11} + \frac{2}{{(\Delta z^{*} )^{2} Pe_{\text{L}} }}x_{A,12} + \frac{4}{{(\Delta r^{*} )^{2} Pe_{\text{R}} }}x_{A,21} = \hfill \\ \quad - \frac{{k_{\text{cat}} C_{E0} (1 - \varepsilon )d_{\text{p}} (1 - x_{A,11} )}}{{u(r)\varepsilon C_{A0} \left[ {\frac{{K_{\text{m}} }}{{C_{A0} }} + (1 - x_{A,11} )} \right]}} \hfill \\ \end{aligned} $$

(36)

which may be written as:

$$ \begin{aligned} F(x_{A,11} ) = \left[ - \frac{2}{{Pe_{\text{L}} (\Delta z^{*} )^{2} }} - \frac{{2u_{c} d_{\text{p}} }}{{Pe_{\text{L}} D_{\text{eL}} (\Delta z^{*} )}} - \frac{{u_{c} d_{\text{p}} }}{{D_{\text{eL}} }} - \frac{4}{{(\Delta r^{*} )^{2} Pe_{\text{R}} }}\right]x_{A,11} + \frac{2}{{(\Delta z^{*} )^{2} Pe_{\text{L}} }}x_{A,12} + \frac{4}{{(\Delta r^{*} )^{2} Pe_{\text{R}} }}x_{A,21} \hfill \\ {\kern 1pt} {\kern 1pt} \quad + \frac{{k_{\text{cat}} C_{E0} (1 - \varepsilon )d_{\text{p}} (1 - x_{A,11} )}}{{u(r)\varepsilon C_{A0} \left[ {\frac{{K_{\text{m}} }}{{C_{A0} }} + (1 - x_{A,11} )} \right]}} \hfill \\ \end{aligned} $$

(37)

For $z_{j}^{*} = z_{2}^{*} , \ldots z_{M}^{*}$ Eq. 34 may be written as:

$$ \begin{aligned} \left[ {\frac{1}{{Pe_{L} (\Delta z*)^{2} }} + \frac{1}{2\Delta z*}} \right]x_{A,1j - 1} + \left[ {\frac{ - 2}{{Pe_{L} (\Delta z*)^{2} }} - \frac{4}{{(\Delta r*)^{2} Pe_{R} }}} \right]x_{A,1j} + \left[ {\frac{1}{{Pe_{L} (\Delta z*)^{2} }} - \frac{1}{2\Delta z*}} \right]x_{A,1j + 1} + \frac{4}{{(\Delta r*)^{2} Pe_{R} }}x_{A,2j} \hfill \\ \quad \quad = \frac{{k_{cat} C_{E0} (1 - \varepsilon )d_{p} (1 - x_{A,1j} )}}{{u(r_{i} )\varepsilon C_{A0} \left[ {\frac{{K_{m} }}{{C_{A0} }} + (1 - x_{A,1j} )} \right]}} \hfill \\ \end{aligned} $$

(38)

At $z_{i}^{*} = z_{M + 1}^{*} ( = L/d_{\text{p}} ),$ the boundary condition given by Eq. (17) reduces to:

$$ \frac{{x_{A,M + 2} - x_{A,M} }}{{2\Delta z^{*} }} = 0 $$

(39)

which when substituted in equation (38) yields:

$$ \frac{2}{{Pe_{\text{L}} (\Delta z^{*} )^{2} }}x_{A,1M} + \left [ - \frac{2}{{Pe_{\text{L}} (\Delta z^{*} )^{2} }} - \frac{4}{{(\Delta r^{*} )^{2} Pe_{\text{R}} }}\right ]x_{A,1M + 1} + \frac{4}{{(\Delta r^{*} )^{2} Pe_{\text{R}} }}x_{A,2M + 1} = - \frac{{k_{\text{cat}} C_{E0} (1 - \varepsilon )d_{\text{p}} (1 - x_{A,M + 1} )}}{{u(r_{i} )\varepsilon C_{A0} \left[ {\frac{{K_{\text{m}} }}{{C_{A0} }} + (1 - x_{A,M + 1} )} \right]}}|_{{r_{i} = r_{1} }} $$

(40)

Equations identical to (37) can be written for equation (38) and (40) also. The equations can be rearranged in matrix form and written as:

$$ x_{A,ij}^{k + 1} = x_{A,ij}^{k} - \left[ {A\left[ {x_{A,ij}^{k} } \right]} \right]^{ - 1} (F(x_{A,ij}^{k} )) $$

(41)

Here, $A\left[ {x_{A,ij}^{k} } \right]$ is the Jacobian matrix $\frac{\partial F}{\partial x}$

Similarly, the matrix can be constructed for $i = 2, \ldots N$ and $j = 1, \ldots M + 1$ on rearranging Eq. (27), and solved to obtain the substrate conversion in radial and axial directions.