A three-dimensional computational multiscale micromorphic analysis of porous materials in linear elasticity

Abstract

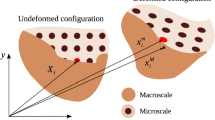
We present an extension of a multiscale micromorphic theory to three-dimensional problems for porous materials, where a clear scale separation is not given. Following the multiscale micromorphic framework of Biswas and Poh (J Mech Phys Solids 102:187–208, 2017), macroscopic governing equations of a micromorphic continuum are derived from a classical continuum on the microscale by means of a kinematic field decomposition. The macro–microenergy equivalence is guaranteed via the Hill–Mandel condition. For linear elasticity problems, generalized elasticity tensors are determined via several RVE computations once and for all, avoiding concurrent RVE computations for an online structural analysis. A three-dimensional implementation is revealed in detail. A comparative study with direct numerical simulations and first-order multiscale computations shows that the computational multiscale micromorphic method has sufficient accuracy and computational efficiency, thus providing a powerful tool for design and practical engineering applications of porous materials.

Appendices

Appendix A: Technical details for three-dimensional periodic boundary conditions

In the following, several details are given for the three-dimensional periodic boundary condition in Sect. 3.1. By means of interpolation of corresponding corner displacements in Eq. (25), Eq. (23) becomes

$$\begin{aligned} \hat{u}_{i}^{F}-\hat{u}_{i}^{Ba} =&\frac{1}{4}(1-h)(1-g)(\hat{u}_{i}^{(4)}-\hat{u}_{i}^{(8)})+\frac{1}{4}(1+h)(1-g)(\hat{u}_{i}^{(3)}-\hat{u}_{i}^{(7)}) \nonumber \\&+\frac{1}{4}(1-h)(1+g)(\hat{u}_{i}^{(1)}-\hat{u}_{i}^{(5)})+\frac{1}{4}(1+h)(1+g)(\hat{u}_{i}^{(2)}-\hat{u}_{i}^{(6)}), \end{aligned}$$

(A.1a)

$$\begin{aligned} \hat{u}_{i}^{T}-\hat{u}_{i}^{Bo}=&\frac{1}{4}(1-h)(1-k)(\hat{u}_{i}^{(5)}-\hat{u}_{i}^{(8)})+\frac{1}{4}(1+h)(1-k)(\hat{u}_{i}^{(6)}-\hat{u}_{i}^{(7)}) \nonumber \\&+\frac{1}{4}(1-h)(1+k)(\hat{u}_{i}^{(3)}-\hat{u}_{i}^{(4)})+\frac{1}{4}(1+h)(1+k)(\hat{u}_{i}^{(2)}-\hat{u}_{i}^{(3)}), \end{aligned}$$

(A.1b)

$$\begin{aligned} \hat{u}_{i}^{R}-\hat{u}_{i}^{L}=&\frac{1}{4}(1-g)(1-k)(\hat{u}_{i}^{(7)}-\hat{u}_{i}^{(8)})+\frac{1}{4}(1+g)(1-k)(\hat{u}_{i}^{(6)}-\hat{u}_{i}^{(5)}) \nonumber \\&+\frac{1}{4}(1-g)(1+k)(\hat{u}_{i}^{(3)}-\hat{u}_{i}^{(4)})+\frac{1}{4}(1+g)(1+k)(\hat{u}_{i}^{(2)}-\hat{u}_{i}^{(1)}). \end{aligned}$$

(A.1c)

Here, $h=\frac{2{\varvec{y}_{1}}}{l}$, $g=\frac{2{\varvec{y}_{2}}}{l}$ and $k=\frac{2{\varvec{y}_{3}}}{l}$ represent the normalized coordinates of the analogous node pairs at the front–back, top–bottom and left–right boundary pairs, respectively. Similarly, by means of linear interpolation of corresponding corner displacements, Eq. (24) becomes

$$\begin{aligned}&\hat{u}_{i}^{L12}-\hat{u}_{i}^{L43}=\frac{1}{2}(1-h)(\hat{u}_{i}^{(1)}-\hat{u}_{i}^{(4)})+\frac{1}{2}(1+h)(\hat{u}_{i}^{(2)}-\hat{u}_{i}^{(3)}), \end{aligned}$$

(A.2a)

$$\begin{aligned}&\hat{u}_{i}^{L12}-\hat{u}_{i}^{L56}=\frac{1}{2}(1-h)(\hat{u}_{i}^{(1)}-\hat{u}_{i}^{(5)})+\frac{1}{2}(1+h)(\hat{u}_{i}^{(2)}-\hat{u}_{i}^{(6)}), \end{aligned}$$

(A.2b)

$$\begin{aligned}&\hat{u}_{i}^{L12}-\hat{u}_{i}^{L87}=\frac{1}{2}(1-h)(\hat{u}_{i}^{(1)}-\hat{u}_{i}^{(8)})+\frac{1}{2}(1+h)(\hat{u}_{i}^{(2)}-\hat{u}_{i}^{(7)}), \end{aligned}$$

(A.2c)

$$\begin{aligned}&\hat{u}_{i}^{L23}-\hat{u}_{i}^{L14}=\frac{1}{2}(1-g)(\hat{u}_{i}^{(3)}-\hat{u}_{i}^{(4)})+\frac{1}{2}(1+g)(\hat{u}_{i}^{(2)}-\hat{u}_{i}^{(1)}), \end{aligned}$$

(A.2d)

$$\begin{aligned}&\hat{u}_{i}^{L23}-\hat{u}_{i}^{L58}=\frac{1}{2}(1-g)(\hat{u}_{i}^{(3)}-\hat{u}_{i}^{(8)})+\frac{1}{2}(1+g)(\hat{u}_{i}^{(2)}-\hat{u}_{i}^{(5)}), \end{aligned}$$

(A.2e)

$$\begin{aligned}&\hat{u}_{i}^{L23}-\hat{u}_{i}^{L67}=\frac{1}{2}(1-g)(\hat{u}_{i}^{(3)}-\hat{u}_{i}^{(7)})+\frac{1}{2}(1+g)(\hat{u}_{i}^{(2)}-\hat{u}_{i}^{(6)}), \end{aligned}$$

(A.2f)

$$\begin{aligned}&\hat{u}_{i}^{L26}-\hat{u}_{i}^{L15}=\frac{1}{2}(1-k)(\hat{u}_{i}^{(6)}-\hat{u}_{i}^{(5)})+\frac{1}{2}(1+k)(\hat{u}_{i}^{(2)}-\hat{u}_{i}^{(1)}), \end{aligned}$$

(A.2g)

$$\begin{aligned}&\hat{u}_{i}^{L26}-\hat{u}_{i}^{L48}=\frac{1}{2}(1-k)(\hat{u}_{i}^{(6)}-\hat{u}_{i}^{(8)})+\frac{1}{2}(1+k)(\hat{u}_{i}^{(2)}-\hat{u}_{i}^{(4)}), \end{aligned}$$

(A.2h)

$$\begin{aligned}&\hat{u}_{i}^{L26}-\hat{u}_{i}^{L37}=\frac{1}{2}(1-k)(\hat{u}_{i}^{(6)}-\hat{u}_{i}^{(7)})+\frac{1}{2}(1+k)(\hat{u}_{i}^{(2)}-\hat{u}_{i}^{(3)}). \end{aligned}$$

(A.2i)

which are similar to the formulas in a two-dimensional implementation in [1].

Appendix B: Numerical determination of the elasticity tensors

The elasticity tensors ${{{\varvec{C}}}^{m}}$, ${{{\varvec{E}}}^{n}}$ and ${\varvec{D}}$ are computed similarly to the work [1]. For our three-dimensional implementation, the macroscopic elasticity operator is obtained by condensing the microscopic finite element equations. In the microscale, the finite element equation system reads

$$\begin{aligned} \underline{\underline{{\hat{K}}}}\underline{{\hat{u}}}=\underline{{\hat{r}}}, \end{aligned}$$

(B.1)

where $\underline{\underline{\hat{K}}}$ is the global stiffness matrix of the RVE and $\underline{{\hat{r}}}$ represents the residual force. Then, the global stiffness matrix is partitioned as follows

$$\begin{aligned} \left[ \begin{matrix} {{\underline{\underline{{\hat{K}}}}}_{ii}} &{} {{\underline{\underline{{\hat{K}}}}}_{id}} \\ {{\underline{\underline{{\hat{K}}}}}_{di}} &{} {{\underline{\underline{{\hat{K}}}}}_{dd}} \\ \end{matrix} \right] \left[ \begin{matrix} {{\underline{{\hat{u}}}}_{i}} \\ {{\underline{{\hat{u}}}}_{d}} \\ \end{matrix} \right] =\left[ \begin{matrix} {{\underline{{\hat{r}}}}_{i}} \\ {{\underline{{\hat{r}}}}_{d}} \\ \end{matrix} \right] , \end{aligned}$$

(B.2)

where the subscript d denotes the dependent degrees of freedom involved in the periodic boundary conditions (23) and (24). There holds ${{\underline{{\hat{u}}}}_{d}}=\underline{\underline{{\hat{C}}}}{{\underline{{\hat{u}}}}_{i}}$ for the independent degrees of freedom ${{\underline{{\hat{u}}}}_{i}}$, with the correlation matrix $\underline{\underline{{\hat{C}}}}$. By means of a static condensation [22], Eq. (B.1) reduces to

$$\begin{aligned} \underline{\underline{{{{\hat{K}}}^{*}}}}{{\underline{{\hat{u}}}}_{i}}=\underline{{{{\hat{r}}}^{*}}}, \end{aligned}$$

(B.3)

where

$$\begin{aligned}&\underline{\underline{{{{\hat{K}}}^{*}}}}={{\underline{\underline{{\hat{K}}}}}_{ii}}+{{\underline{\underline{{\hat{K}}}}}_{id}}\underline{\underline{{\hat{C}}}}+{{\underline{\underline{{\hat{C}}}}}^{T}}{{\underline{\underline{{\hat{K}}}}}_{di}}+{{\underline{\underline{{\hat{C}}}}}^{T}}{{\underline{\underline{{\hat{K}}}}}_{dd}}\underline{\underline{{\hat{C}}}}, \end{aligned}$$

(B.4a)

$$\begin{aligned}&{{\underline{{{{\hat{r}}}^{*}}}}_{i}}={{\underline{{\hat{r}}}}_{i}}+{{\underline{\underline{{\hat{C}}}}}^{T}}{{\underline{{\hat{r}}}}_{d}}. \end{aligned}$$

(B.4b)

In order to impose the constraint (30), dummy degrees of freedom with the subscript $\tilde{\lambda }$ are introduced as

$$\begin{aligned} \underline{\underline{W}}{{\underline{{\hat{u}}}}_{I}}=\underline{{\tilde{H}}}={{\underline{{\hat{u}}}}_{{\tilde{\lambda }}}}, \end{aligned}$$

(B.5)

where $\underline{\underline{W}}$ and ${{\underline{{\hat{u}}}}_{I}}$ represent the coefficient matrix in Eq. (30) and degrees of freedom along the hole surfaces, respectively. Next, we rewrite Eq. (B.5) as

$$\begin{aligned} {{\underline{{\hat{u}}}}_{{{I}_{d}}}}=\left[ \begin{matrix} -\underline{\underline{{\hat{W}}}}_{2}^{-1}{{\underline{\underline{{\hat{W}}}}}_{1}} &{} \underline{\underline{{\hat{W}}}}_{2}^{-1} \\ \end{matrix} \right] \left[ \begin{matrix} {{\underline{{\hat{u}}}}_{a}} \\ {{\underline{{\hat{u}}}}_{{\tilde{\lambda }}}} \\ \end{matrix} \right] =\left[ \begin{matrix} {{\underline{\underline{{\hat{C}}}}}_{1}} &{} {{\underline{\underline{{\hat{C}}}}}_{2}} \\ \end{matrix} \right] \left[ \begin{matrix} {{\underline{{\hat{u}}}}_{a}} \\ {{\underline{{\hat{u}}}}_{{\tilde{\lambda }}}} \\ \end{matrix} \right] , \end{aligned}$$

(B.6)

where ${{\underline{{\hat{u}}}}_{{{I}_{d}}}}$ and ${{\underline{{\hat{u}}}}_{a}}$ denote the dependent degrees of freedom to be eliminated at the hole surface and the remaining degrees of freedom in Eq. (B.3), respectively. As a result, Eq. (B.3) becomes

$$\begin{aligned} \left[ \begin{matrix} {{\underline{\underline{{{{\hat{K}}}^{*}}}}}_{aa}} &{} \underline{\underline{0}} &{} {{\underline{\underline{{{{\hat{K}}}^{*}}}}}_{a{{I}_{d}}}} \\ \underline{\underline{0}} &{} \underline{\underline{I}} &{} \underline{\underline{0}} \\ {{\underline{\underline{{{{\hat{K}}}^{*}}}}}_{{{I}_{d}}a}} &{} \underline{\underline{0}} &{} {{\underline{\underline{{{{\hat{K}}}^{*}}}}}_{{{I}_{d}}{{I}_{d}}}} \\ \end{matrix} \right] \left[ \begin{matrix} {{\underline{{\hat{u}}}}_{a}} \\ {{\underline{{\hat{u}}}}_{{\tilde{\lambda }}}} \\ {{\underline{{\hat{u}}}}_{{{I}_{d}}}} \\ \end{matrix} \right] =\left[ \begin{matrix} {{\underline{{{{\hat{r}}}^{*}}}}_{a}} \\ {{\underline{{{r}^{*}}}}_{{\tilde{\lambda }}}} \\ {{\underline{{{r}^{*}}}}_{{{I}_{d}}}} \\ \end{matrix} \right] , \end{aligned}$$

(B.7)

where $\underline{\underline{I}}$ is the identity matrix. On the basis of Eqs. (B.6), (B.7) is further condensed to

$$\begin{aligned} \left[ \begin{matrix} {{\underline{\underline{{{{\hat{K}}}^{**}}}}}_{aa}} &{} {{\underline{\underline{{{{\hat{K}}}^{**}}}}}_{a\tilde{\lambda }}} \\ {{\underline{\underline{{{{\hat{K}}}^{**}}}}}_{\tilde{\lambda }a}} &{} {{\underline{\underline{{{{\hat{K}}}^{**}}}}}_{\tilde{\lambda }\tilde{\lambda }}} \\ \end{matrix} \right] \left[ \begin{matrix} {{\underline{{\hat{u}}}}_{a}} \\ {{\underline{{\hat{u}}}}_{{\tilde{\lambda }}}} \\ \end{matrix} \right] =\left[ \begin{matrix} {{\underline{{{{\hat{r}}}^{**}}}}_{a}} \\ {{\underline{{{r}^{**}}}}_{{\tilde{\lambda }}}} \\ \end{matrix} \right] , \end{aligned}$$

(B.8)

where

$$\begin{aligned}&{{\underline{\underline{{{{\hat{K}}}^{**}}}}}_{aa}}={{\underline{\underline{{{{\hat{K}}}^{*}}}}}_{aa}}+{{\underline{\underline{{{{\hat{K}}}^{*}}}}}_{a{{I}_{d}}}}{{\underline{\underline{{\hat{C}}}}}_{1}}+{{\underline{\underline{{\hat{C}}}}}_{1}}^{T}{{\underline{\underline{{{{\hat{K}}}^{*}}}}}_{{{I}_{d}}a}}+{{\underline{\underline{{\hat{C}}}}}_{1}}^{T}{{\underline{\underline{{{{\hat{K}}}^{*}}}}}_{{{I}_{d}}{{I}_{d}}}}{{\underline{\underline{{\hat{C}}}}}_{1}}, \end{aligned}$$

(B.9a)

$$\begin{aligned}&{{\underline{\underline{{{{\hat{K}}}^{**}}}}}_{a\tilde{\lambda }}}={{\underline{\underline{{{{\hat{K}}}^{*}}}}}_{a{{I}_{d}}}}{{\underline{\underline{{\hat{C}}}}}_{2}}+{{\underline{\underline{{\hat{C}}}}}_{1}}^{T}{{\underline{\underline{{{{\hat{K}}}^{*}}}}}_{{{I}_{d}}{{I}_{d}}}}{{\underline{\underline{{\hat{C}}}}}_{2}}, \end{aligned}$$

(B.9b)

$$\begin{aligned}&{{\underline{\underline{{{{\hat{K}}}^{**}}}}}_{\tilde{\lambda }a}}={{\underline{\underline{{\hat{C}}}}}_{2}}^{T}{{\underline{\underline{{{{\hat{K}}}^{*}}}}}_{{{I}_{d}}a}}+{{\underline{\underline{{\hat{C}}}}}_{2}}^{T}{{\underline{\underline{{{{\hat{K}}}^{*}}}}}_{{{I}_{d}}{{I}_{d}}}}{{\underline{\underline{{\hat{C}}}}}_{1}}, \end{aligned}$$

(B.9c)

$$\begin{aligned}&{{\underline{\underline{{{{\hat{K}}}^{**}}}}}_{\tilde{\lambda }\tilde{\lambda }}}=\underline{\underline{I}}+{{\underline{\underline{{\hat{C}}}}}_{2}}^{T}{{\underline{\underline{{{{\hat{K}}}^{*}}}}}_{{{I}_{d}}{{I}_{d}}}}{{\underline{\underline{{\hat{C}}}}}_{2}}, \end{aligned}$$

(B.9d)

$$\begin{aligned}&{{\underline{{{{\hat{r}}}^{**}}}}_{a}}={{\underline{{{{\hat{r}}}^{*}}}}_{a}}+{{\underline{\underline{{\hat{C}}}}}_{1}}^{T}{{\underline{{{{\hat{r}}}^{*}}}}_{{{I}_{d}}}}, \end{aligned}$$

(B.9e)

$$\begin{aligned}&{{\underline{{{{\hat{r}}}^{**}}}}_{{\tilde{\lambda }}}}={{\underline{{{{\hat{r}}}^{*}}}}_{{\tilde{\lambda }}}}+{{\underline{\underline{{\hat{C}}}}}_{2}}^{T}{{\underline{{{{\hat{r}}}^{*}}}}_{{{I}_{d}}}}. \end{aligned}$$

(B.9f)

The equation system (B.8) is next partitioned as

(B.10)

with the subscripts p and f denoting the corner and the internal free nodes, respectively. By a further condensation [22], Eq. (B.10) finally reduces to

(B.11)

In the numerical implementation, the displacements of the corner nodes of the RVE are prescribed by Eq. (25) as

$$\begin{aligned} \hat{u}_{i}^{(c)}={{H}_{ij}}y_{j}^{(c)}+\frac{1}{4}\{{{\nabla }_{k}}{{\tilde{H}}_{ij}}+{{\nabla }_{k}}{{\tilde{H}}_{ji}}\}y_{j}^{(c)}y_{k}^{(c)}, \qquad c=1,\cdots ,8. \end{aligned}$$

(B.12)

Due to the relation ${{\underline{{\hat{u}}}}_{{\tilde{\lambda }}}}=\underline{{\tilde{H}}}$ in Eq. (B.5), the RVE problem is solved after Eq. (B.11).

Under the periodic boundary conditions (A.1) and (A.2), only corner nodes contribute to Eq. (14). Accordingly, the macroscopic stress tensors are computed by

$$\begin{aligned}&{{\sigma }_{ij}}=\frac{1}{{\hat{V}}}\underset{c=1}{\overset{8}{\mathop {\sum }}}\,f_{i}^{(c)}y_{j}^{(c)}, \end{aligned}$$

(B.13a)

$$\begin{aligned}&{{\xi }_{ij}}=\frac{{{{\hat{V}}}_{I}}}{{\hat{V}}}{{\tilde{\lambda }}_{ij}}, \end{aligned}$$

(B.13b)

$$\begin{aligned}&{{\zeta }_{ijk}}=\frac{1}{4\hat{V}}\underset{c=1}{\overset{8}{\mathop {\sum }}}\,(f_{i}^{(c)}y_{j}^{(c)}y_{k}^{(c)}+f_{j}^{(c)}y_{i}^{(c)}y_{k}^{(c)}). \end{aligned}$$

(B.13c)

From Eqs. (B.11) and (B.13), the components for the elasticity tensors ${{{\varvec{C}}}^{m}}$, ${{{\varvec{E}}}^{n}}$ and ${\varvec{D}}$ in the macroscopic constitutive relations (20) are computed by

$$\begin{aligned}&{{\underline{\underline{{{C}^{1}}}}}_{ijkl}}=\frac{1}{{\hat{V}}}\sum \limits _{a=1}^{8}{\sum \limits _{b=1}^{8}{\underline{\underline{{\bar{K}}}}_{pp(i,k)}^{(ab)}\underline{y}_{(j)}^{(a)}\underline{y}_{(l)}^{(b)}}}, \end{aligned}$$

(B.14a)

$$\begin{aligned}&{{\underline{\underline{{{C}^{2}}}}}_{ij\underline{\beta }}}=\frac{1}{{\hat{V}}}\sum \limits _{a=1}^{8}{\underline{\underline{{\bar{K}}}}_{p\tilde{\lambda }(i,\underline{\beta })}^{(a\tilde{\lambda })}\underline{y}_{(j)}^{(a)}}, \end{aligned}$$

(B.14b)

$$\begin{aligned}&{{\underline{\underline{{{C}^{3}}}}}_{\underline{\alpha }jk}}=\frac{{{{\hat{V}}}_{I}}}{{\hat{V}}}\sum \limits _{a=1}^{8}{\underline{\underline{{\bar{K}}}}_{\tilde{\lambda }p(\underset{\scriptscriptstyle -}{\alpha },j)}^{(\tilde{\lambda }a)}\underline{y}_{(k)}^{(a)}}, \end{aligned}$$

(B.14c)

$$\begin{aligned}&{{\underline{\underline{{{C}^{4}}}}}_{\underline{\alpha }\underline{\beta }}}=\frac{{{{\hat{V}}}_{I}}}{{\hat{V}}}{{\underline{\underline{{\bar{K}}}}}_{\tilde{\lambda }\tilde{\lambda }(\underline{\alpha },\underline{\beta })}}, \end{aligned}$$

(B.14d)

$$\begin{aligned}&{{\underline{\underline{{{E}^{1}}}}}_{ijklm}}=\frac{1}{4\hat{V}}\sum \limits _{a=1}^{8}{\sum \limits _{b=1}^{8}{\left[ \underline{\underline{{\bar{K}}}}_{pp(i,k)}^{(ab)}\underline{y}_{(j)}^{(a)}\underline{y}_{(l)}^{(b)}\underline{y}_{(m)}^{(b)}+\underline{\underline{{\bar{K}}}}_{pp(i,l)}^{(ab)}\underline{y}_{(j)}^{(a)}\underline{y}_{(k)}^{(b)}\underline{y}_{(m)}^{(b)} \right] }}, \end{aligned}$$

(B.14e)

$$\begin{aligned}&{{\underline{\underline{{{E}^{2}}}}}_{\underline{\alpha }jkl}}=\frac{{{{\hat{V}}}_{I}}}{4\hat{V}}\sum \limits _{a=1}^{8}{\left[ \underline{\underline{{\bar{K}}}}_{\tilde{\lambda }p(\underline{\alpha },j)}^{(\tilde{\lambda }a)}\underline{y}_{(k)}^{(a)}\underline{y}_{(l)}^{(a)}+\underline{\underline{{\bar{K}}}}_{\tilde{\lambda }p(\underline{\alpha },k)}^{(\tilde{\lambda }a)}\underline{y}_{(j)}^{(a)}\underline{y}_{(l)}^{(a)} \right] }, \end{aligned}$$

(B.14f)

$$\begin{aligned}&{{\underline{\underline{{{E}^{3}}}}}_{ijklm}}=\frac{1}{4\hat{V}}\sum \limits _{a=1}^{8}{\sum \limits _{b=1}^{8}{\left[ \underline{\underline{{\bar{K}}}}_{pp(i,l)}^{(ab)}\underline{y}_{(j)}^{(a)}\underline{y}_{(k)}^{(a)}\underline{y}_{(m)}^{(b)}+\underline{\underline{{\bar{K}}}}_{pp(j,l)}^{(ab)}\underline{y}_{(i)}^{(a)}\underline{y}_{(k)}^{(a)}\underline{y}_{(m)}^{(b)} \right] }}, \end{aligned}$$

(B.14g)

$$\begin{aligned}&{{\underline{\underline{{{E}^{4}}}}}_{ijk\underline{\beta }}}=\frac{1}{4\hat{V}}\sum \limits _{a=1}^{8}{\left[ \underline{\underline{{\bar{K}}}}_{p\tilde{\lambda }(i,\underline{\beta })}^{(a\tilde{\lambda })}\underline{y}_{(j)}^{(a)}\underline{y}_{(k)}^{(a)}+\underline{\underline{{\bar{K}}}}_{p\tilde{\lambda }(j,\underline{\beta })}^{(a\tilde{\lambda })}\underline{y}_{(i)}^{(a)}\underline{y}_{(k)}^{(a)} \right] }, \end{aligned}$$

(B.14h)

$$\begin{aligned}&{{\underline{\underline{D}}}_{ijklmn}}=\frac{1}{16\hat{V}}\sum \limits _{a=1}^{8}{\sum \limits _{b=1}^{8}{\left[ \underline{\underline{{\bar{K}}}}_{pp(i,l)}^{(ab)}\underline{y}_{(j)}^{(a)}\underline{y}_{(k)}^{(a)}\underline{y}_{(m)}^{(b)}\underline{y}_{(n)}^{(b)}+\underline{\underline{{\bar{K}}}}_{pp(i,m)}^{(ab)}\underline{y}_{(j)}^{(a)}\underline{y}_{(k)}^{(a)}\underline{y}_{(l)}^{(b)}\underline{y}_{(n)}^{(b)} \right. }} \nonumber \\&\left. +\underline{\underline{{\bar{K}}}}_{pp(j,l)}^{(ab)}\underline{y}_{(i)}^{(a)}\underline{y}_{(k)}^{(a)}\underline{y}_{(m)}^{(b)}\underline{y}_{(n)}^{(b)}+\underline{\underline{{\bar{K}}}}_{pp(j,m)}^{(ab)}\underline{y}_{(i)}^{(a)}\underline{y}_{(k)}^{(a)}\underline{y}_{(l)}^{(b)}\underline{y}_{(n)}^{(b)} \right] . \end{aligned}$$

(B.14i)

with $i,j,k,l,m,n=1,2,3$ and $\underline{\alpha },\underline{\beta }=1,2,\cdots ,9$. Taking $\underline{\underline{{\bar{K}}}}_{pp(i,l)}^{(ab)}$ as an example for notation, the superscripts a and b denote the partition in the matrix ${{\underline{\underline{{\bar{K}}}}}_{pp}}$, whereas the subscripts i and l are indices for components.