Performance analysis of parametrically and directly excited nonlinear piezoelectric energy harvester

Abstract

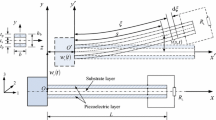
The performance of bimorph cantilever energy harvester subjected to horizontal and vertical excitations is investigated. The energy harvester is simulated as an inextensible piezoelectric beam with the Euler–Bernoulli assumptions. A horizontal base excitation along the axis of the beam is converted into the parametric excitation. The governing equations include geometric, inertia and electromechanical coupling nonlinearities. Using the Galerkin method, the electromechanical coupling Mathieu–Duffing equation is developed. Analytical solutions of the frequency response curves are presented by using the method of multiple scales. Some analytical results are obtained, which reveal the influence of different parameters such as the damping, load resistance and excitation amplitude on the output power of the energy harvester. In the case of parametric excitation, the effect of mechanical damping and load resistance on the initiation excitation threshold is studied. In the case of combination of parametric and direct excitations, the dynamic characteristics and performance of the nonlinear piezoelectric energy harvesters are studied. Our studies revealed that the bending deformation generated by direct excitation pushes the system out of axial deformation and overcomes the limitation of initial threshold of parametric excitation system. The combination of parametric and direct excitations, which compensates and complements each other, can be served as a better solution which enhances performance of energy harvesters.

Appendices

Appendix A

The coefficients defined in Eqs. (9) and (10) are given as:

$$\begin{aligned} a_{1n} \left( {\bar{{s}}} \right)= & {} \int _{\bar{{s}}}^1 {\int _0^{{\bar{{\xi }}}}{{\phi '}_{n}^{2} \left( {\bar{{\eta }}} \right) \mathrm{d}\bar{{\eta }}\mathrm{d}\bar{{\xi }}}} ,\, a_{2n} \left( {\bar{{s}}} \right) =\int _0^{\bar{{s}}}{{\phi '}_{n}^{2} \left( {\bar{{\eta }}} \right) \mathrm{d}\bar{{\eta }}} \nonumber \\ b{ }_{1nn}= & {} \int _0^1 {\phi _n \phi _n \mathrm{d}\bar{{s}}} ,\,b{ }_{2nn}=\int _0^{1} {\left( {\bar{{\beta }}_{n}^{4} {\phi '}_{n}^{2} X_{n} +4{\phi '}_{n} {\phi ''}_{n} {\phi '''}_{n} +{\phi ''}_n ^{3}} \right) \phi _n \mathrm{d}\bar{{s}}} ,b{ }_{3nn}=\int _0^1 {{\phi ''}_{n} \phi _n \mathrm{d}\bar{{s}}}\nonumber \\ b{ }_{4nn}= & {} \int _0^1 {\bar{{s}}{\phi }''_n \phi _n \mathrm{d}\bar{{s}}} , b{ }_{5nn}=\int _0^1 {a_{1n} \left( {\bar{{s}}} \right) {\phi }''_n \phi _n \mathrm{d}\bar{{s}}} ,~b{ }_{6nn}=\int _0^1 {{\phi }'_n \phi _n \mathrm{d}\bar{{s}}} ,\,b{ }_{7nn}=\int _0^1 {a_{2n} \left( {\bar{{s}}} \right) {\phi }'_n \phi _n \mathrm{d}\bar{{s}}}\nonumber \\ b{ }_{8nn}= & {} \int _0^1 {\phi _n \mathrm{d}\bar{{s}}} , \quad b{ }_{9nn}=\left. {\frac{\mathrm{d}\phi _n \left( {\bar{{s}}} \right) }{\mathrm{d}\bar{{s}}}} \right| _{\bar{{s}}=1} ,\, b{ }_{10nn}=\left. {\frac{\mathrm{d}\left[ {\phi _n \left( {\bar{{s}}} \right) {\phi '}_{{n}}^{2} \left( {\bar{{s}}} \right) } \right] }{\mathrm{d}\bar{{s}}}} \right| _{\bar{{s}}=1} ,\,b{ }_{11nn}=\phi _{n} \left( 1 \right) {\phi '}_{n} \left( 1 \right) {\phi ''}_{n} \left( 1 \right) \nonumber \\ b_{12nn}= & {} \int _0^1 {{\phi }''_n } \mathrm{d}\bar{{s}},\,b_{13nn} =\int _0^1 {{\phi '}_{{n}}^{2} {\phi ''}_n \mathrm{d}\bar{{s}}}\nonumber \\ {\tilde{c}}= & {} \frac{1}{2}\bar{{c}},\bar{{\sigma }}_n =\frac{b{ }_{3nn}-b{ }_{4nn}-b{ }_{6nn}}{2b{ }_{1nn}},\beta _n =\frac{b{ }_{2nn}}{b{ }_{1nn}},\kappa _n =\frac{b{ }_{7nn}-b{ }_{5nn}}{b{ }_{1nn}} \nonumber \\ \zeta _n= & {} \frac{b{ }_{9nn}}{b{ }_{1nn}},\gamma _n =\frac{b{ }_{10nn}-2b{ }_{11nn}}{2b{ }_{1nn}},\bar{{\lambda }}_n =\frac{b_{8nn} }{b_{1nn} },\eta _n =b{ }_{12nn},\chi _n =\frac{3}{2}b_{13nn} \nonumber \\ \end{aligned}$$

(A1)

$$\begin{aligned} b_{1nn}= & {} \int _0^1 {\phi _n } \phi _n \mathrm{d}\bar{{s}}=1,b_{12nn} =\int _0^1 {{\phi }''_n \mathrm{d}\bar{{s}}=\left. {\frac{\mathrm{d}\phi _n (\bar{{s}})}{\mathrm{d}\bar{{s}}}} \right| } _{\bar{{s}}=1} =b_{9nn} \nonumber \\ b_{10nn}= & {} \frac{\mathrm{d}\left[ {{\phi '}_{n} (\bar{{s}}){\phi '}_{n} (\bar{{s}})\phi _n (\bar{{s}})} \right] }{\mathrm{d}\bar{{s}}} \big |_{\bar{{s}}=1} =2\phi _n (1){\phi '}_n (1){\phi ''}_{n} (1)+{\phi '}_{n}^{3} (1) \nonumber \\ b_{13nn}= & {} \int _0^1 {{\phi '}_n {\phi '}_n {\phi ''}_{n}} \mathrm{d}\bar{{s}}=\frac{1}{3}{\phi '}_n^3 (1),\eta _n =\zeta _n ,\chi _n =\gamma _n \end{aligned}$$

(A2)

Appendix B

The coefficients defined in Eqs. (26)–(31) are as following:

$$\begin{aligned} c_1= & {} \hat{{c}}+\bar{{\alpha }}^{2}\frac{\hat{{\zeta }}\eta \mu }{2(\mu ^{2}+\bar{{\omega }}^{2})},\,c_2 =\bar{{\alpha }}^{2}\frac{\chi \hat{{\zeta }}\mu }{8(\bar{{\omega }}^{2}+\mu ^{2})}+\bar{{\alpha }}^{2}\frac{\eta \mu \hat{{\gamma }}}{4(\bar{{\omega }}^{2}+\mu ^{2})} \\ c_3= & {} \bar{{\alpha }}^{2}\frac{\mu \hat{{\gamma }}\chi }{16(\bar{{\omega }}^{2}+\mu ^{2})}+\bar{{\alpha }}^{2}\frac{\mu \hat{{\gamma }}\chi }{32(9\bar{{\omega }}^{2}+\mu ^{2})},\,c_4 =\frac{\hat{{\delta }}_y }{2\bar{{\omega }}},c_5 =\bar{{\alpha }}^{2}\frac{\bar{{\omega }}\eta \hat{{\zeta }}}{2(\bar{{\omega }}^{2}+\mu ^{2})} \\ c_6= & {} \frac{3\hat{{\beta }}}{8\bar{{\omega }}}+\bar{{\alpha }}^{2}\frac{\hat{{\zeta }}\bar{{\omega }}\chi }{8(\bar{{\omega }}^{2}+\mu ^{2})}-\frac{1}{4}\bar{{\omega }}\hat{{\kappa }}+\bar{{\alpha }}^{2}\frac{\mu \bar{{\omega }}\eta }{4(\bar{{\omega }}^{2}+\mu ^{2})} \\ c_7= & {} \bar{{\alpha }}^{2}\frac{\mu \bar{{\omega }}\chi }{16(\bar{{\omega }}^{2}+\mu ^{2})}+\alpha _0 ^{2}\frac{3\mu \bar{{\omega }}\chi }{32(9\bar{{\omega }}^{2}+\mu ^{2})},\,c_8 =\frac{\hat{{\delta }}_x }{2\bar{{\omega }}} \end{aligned}$$