A Regularized Model for Wetting/Dewetting Problems: Positivity and Asymptotic Analysis

Abstract

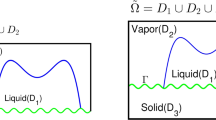
We consider a general regularized variational model for simulating wetting/dewetting phenomena arising from solids or fluids. The regularized model leads to the appearance of a precursor layer which covers the bare substrate, with the precursor height depending on the regularization parameter $\varepsilon$. This model enjoys lots of advantages in analysis and simulations. With the help of the precursor layer, the spatial domain is naturally extended to a larger fixed one in the regularized model, which leads to both analytical and computational eases. There is no need to explicitly track the contact line motion, and difficulties arising from free boundary problems can be avoided. In addition, topological change events can be automatically captured. Under some mild and physically meaningful conditions, we show the positivity-preserving property of the minimizers of the regularized model. By using formal asymptotic analysis and $\Gamma$-limit analysis, we investigate the convergence relations between the regularized model and the classical sharp-interface model. Finally, numerical results are provided to validate our theoretical analysis, as well as the accuracy and efficiency of the regularized model.

Appendices

Appendix A: Functions of Bounded Variation

In this appendix, we briefly introduce some definitions and properties of the functions of bounded variation. For more details, we refer the reader to Refs. (Ambrosio et al. 2000; Evans and Garzepy 2018). In this appendix, we always assume $\Omega \subset {\mathbb {R}}^{n}$.

Definition A.1

(Function of bounded variation) Let $u \in L^{1}(\Omega )$. u is a function of bounded variation in $\Omega$ if the distributional derivative of u is represented by a finite Radon measure, i.e.,

$$\begin{aligned} \int _{\Omega } u \frac{\partial \psi }{\partial x_{i}} \textrm{d}x=-\int _{\Omega } \psi \textrm{d}D_{i} u \quad \forall \psi \in C_{0}^{\infty }(\Omega ), \quad i=1, \ldots , n \end{aligned}$$

for some ${\mathbb {R}}^{n}$-valued Radon measure $D u=\left( D_{1} u, \ldots , D_{n} u\right)$ in $\Omega$. The vector space of all functions of bounded variation is denoted by $BV(\Omega )$.

We can always write $D u=\sigma |D u|$, where |Du| is a positive Radon measure and $\sigma =\left( \sigma _{1}, \ldots , \sigma _{n}\right)$ with $|\sigma (x)|=1$ for |Du|-a.e. $x \in \Omega$.

Moreover, we say that $u\in BV_{\textrm{loc}}(\Omega )$ if $u \in BV(\Omega _0)$ for every $\Omega _0\subset \subset \Omega$, i.e., every open $\Omega _0$ with $\overline{\Omega _0}$ compact and contained in $\Omega$.

Definition A.2

(Sets of finite perimeter) Let U be an ${\mathcal {L}}^n$-measurable subset of ${\textbf{R}}^n$. For any open set $\Omega \subset {\mathbb {R}}^n$, the perimeter of U in $\Omega$, denoted by $P(U,\Omega )$, is the variation of $\chi _U$ in $\Omega$, i.e.,

$$\begin{aligned} P(U, \Omega ):=\sup \left\{ \int _E {\text {div}} \varphi \textrm{d}x: \varphi \in \left[ C_0^1(\Omega )\right] ^n,~\Vert \varphi \Vert _{\infty } \leqslant 1\right\} . \end{aligned}$$

We say that U is a set of finite perimeter in $\Omega$ if $P(U, \Omega )<\infty$.

Definition A.3

(Approximate jump points) Let $u \in L_{\textrm{loc}}^{1}(\Omega )$ and $x \in \Omega$. We say that x is an approximate jump point of u if there exist $a, b \in {\mathbb {R}}$ and an $(n-1)$-dimensional unit vector $\varvec{\nu }$ such that $a \ne b$ and

$$\begin{aligned} \lim _{R \downarrow 0} \int _{B_{R}^{+}(x,\varvec{\nu } )}R^{-n}|u(y)-a| \textrm{d}y=0, \quad \lim _{R \downarrow 0} \int _{B_{R}^{-}(x, \varvec{\nu })}R^{-n}|u(y)-b| \textrm{d}y=0, \end{aligned}$$

where $u^+:=a$ and $u^-:=b$ are called one-side approximate limits and

$$\begin{aligned} \begin{array}{l} B_{R}^{+}(x, \varvec{\nu }):=\left\{ y \in B_{R}(x):\langle y-x, \varvec{\nu }\rangle >0\right\} , \\ B_{R}^{-}(x, \varvec{\nu }):=\left\{ y \in B_{R}(x):\{y-x, \varvec{\nu }\rangle <0\right\} , \end{array} \end{aligned}$$

which means two half balls contained in $B_{R}(x)$ determined by $\varvec{\nu }$. The set of approximate jump points is denoted by $J_u$.

We recall the usual decomposition

$$\begin{aligned} D u=\nabla u {\mathcal {L}}^{n}+\left( u^{+}-u^{-}\right) \otimes \varvec{\nu }_{u} {\mathcal {H}}^{n-1}\mathrm {~\llcorner } J_u+D^{c} u, \end{aligned}$$

where Du is the distribution derivative of u, $\nabla u$ is the Radon–Nikodym derivative of Du with respect to the Lebesgue measure ${\mathcal {L}}^n$, $\varvec{\nu }_{u}$ is unit normal vector, ${\mathcal {H}}^{n-1}\mathrm {~\llcorner } J_u$ means Hausdorff measure restricted to the set $J_u$, and $D^{c} u$ is the Cantor part of Du. For the sake of simplicity, we denote $D^su:= \left( u^{+}-u^{-}\right) \otimes \varvec{\nu }_{u} {\mathcal {H}}^{n-1}\mathrm {~\llcorner } J_u+D^{c} u$, which is called the singular part.

We introduce two important properties by the following two lemmas whose proof can be seen in Ref. Ambrosio et al. (2000).

Lemma A.1

(Property of $D^{a} u, D^{s} u$) Let $u \in B V(\Omega )$, then $D^{a} u=D u\llcorner (\Omega \backslash S)$ and $D^{s} u=D u\llcorner S$, where

$$\begin{aligned} S:=\left\{ x \in \Omega : \lim _{R \downarrow 0} R^{-n}|D u|\left( B_{R}(x)\right) =\infty \right\} . \end{aligned}$$

Lemma A.2

(Property of $D^{c} u$) Let $u \in B V(\Omega )$, and let B be a Borel set with its $(n-1)$-dimensional Hausdorff measure ${\mathcal {H}}^{n-1}(B)<+\infty$. Then $\left| D^{c} u\right| (B)=0$.

Definition A.4

(Area functional) We define the area functional as follows:

$$\begin{aligned}{} & {} \int _{\Omega } \sqrt{1+|D u|^2}\\{} & {} =\sup \left\{ \int _{\Omega }\left( \varphi _{n+1}+u D_i \varphi _i\right) \textrm{d} x\left| \varphi =\left( \varphi _1, \ldots , \varphi _{n+1}\right) \in C_0^1(\Omega ),\right| \varphi \mid \leqslant 1\right\} . \end{aligned}$$

Proposition A.1

The area functional has a special decomposition (Demengel and Temam 1984):

$$\begin{aligned} \int _{\Omega }\sqrt{1+|Du|^2}=\int _{\Omega }\sqrt{1+|\nabla u|^2}\textrm{d}x+|D^{c} u|(\Omega )+\int _{J_u\cap \Omega }|u^+-u^-| \mathrm {~d} {\mathcal {H}}^{n-1}, \end{aligned}$$

(6.1)

whose geometric meaning is the perimeter of the subgraph $U:=\{(x,t)\in \Omega \times {\mathbb {R}}:t<h(x)\}$ of h in $\Omega$ (Giusti 1984). In particular, if h is Lipschitz continuous, it represents the area of the surface $\{(x,h(x)):x\in \Omega \}$.

Next, we introduce the compactness of the functions of bounded variation (Evans and Garzepy 2018).

Lemma A.3

(Compactness of BV functions) Let $\Omega \subset {\mathbb {R}}^{n}$ be open and bounded, with $\partial \Omega$ Lipschitz. Assume $\left\{ f_{j}\right\} _{j=1}^{\infty }$ is a sequence in $B V(\Omega )$ satisfying

$$\begin{aligned} \sup _{j}\left\| f_{j}\right\| _{B V(\Omega )}<\infty . \end{aligned}$$

Then there exists a subsequence $\left\{ f_{j_{k}}\right\} _{k=1}^{\infty }$ and a function $f \in B V(\Omega )$ such that

$$\begin{aligned} f_{j_{k}} \rightarrow f \text{ in } L^{1}(\Omega ), \end{aligned}$$

as $k \rightarrow \infty$.

At last, we give a proposition (c.f. Corollary 1.29 of Ambrosio et al. 2000).

Proposition A.2

(polar decomposition) Let $\mu$ be a ${\mathbb {R}}^m$-valued measure on the measure space $(X, {\mathcal {E}})$, then there exists a unique ${\mathbb {S}}^{m-1}$-valued function $f \in \left[ L^1(X,|\mu |)\right] ^m$ such that $\mu =f|\mu |$, where ${\mathbb {S}}^{m-1}$ is $(m-1)$-dimensional unit sphere.

Appendix B: Proof of Lemma 4.2

Firstly, we recall the definition and properties of mollifiers (Giusti 1984)

A function $\eta (x)$ is called a mollifier if

• (i) $\eta (x) \in C_{0}^{\infty }\left( {\mathbb {R}}^{n}\right)$,

• (ii) $\eta$ is zero outside a compact subset of $B_{1}=\left\{ x \in {\mathbb {R}}^{n}:|x|<1\right\}$,

• (iii) $\int \eta (x) d x=1$.

If in addition we have

• (iv) $\eta (x) \geqslant 0$,

• (v) $\eta (x)=\mu (|x|)$ for some function $\mu : {\mathbb {R}}^{+} \rightarrow {\mathbb {R}}$,

then $\eta$ is a positive symmetric mollifier. An example of positive symmetric mollifiers is the function

$$\begin{aligned} \eta (x)= {\left\{ \begin{array}{ll}0 &{} |x| \geqslant 1, \\ C \exp \left( \frac{1}{|x|^{2}-1}\right) &{} |x|<1,\end{array}\right. } \end{aligned}$$

where C is a normalizing constant such that $\int \eta (x) d x=1$.

Given a positive symmetric mollifier $\eta$ and a function $f \in L_{\text{ loc } }^{1}\left( {\mathbb {R}}^{n}\right)$, define for each $\varepsilon >0$

$$\begin{aligned}{} & {} \eta _{\varepsilon }(x):=\varepsilon ^{-n} \eta \left( \frac{x}{\varepsilon }\right) , \\{} & {} f_{\varepsilon }(x):=(\eta _{\varepsilon } * f)(x)=\varepsilon ^{-n} \int _{{\mathbb {R}}^{n}} \eta \left( \frac{x-z}{\varepsilon }\right) f(z) \textrm{d}z=\int _{{\mathbb {R}}^{n}} \eta (w) f(x+\varepsilon w) \textrm{d}w. \end{aligned}$$

It is straightforward to have the following two properties of mollifiers:

(a) $f(x) \geqslant 0\ a.e.$ in $\Omega$ $\ \Rightarrow f_{\varepsilon }(x) \geqslant 0$ if $\varepsilon$ is small enough,

(b) ${\text {Supp}} f \subseteq A \Rightarrow {\text {Supp}} f_{\varepsilon } \subseteq A_{\varepsilon }=\{x: {\text {dist}}(x, A) \leqslant \varepsilon \}$.

Using the technique of mollifiers, Lemma B.1 of Ref. Bildhauer (2003) can be established.

Lemma B.1

Let $h \in B V\left( \Omega \right)$. Then there is a sequence $\left\{ h_{j}\right\}$ in $C^{\infty }\left( \Omega \right)$ satisfying

$$\begin{aligned} \begin{aligned}&\lim _{j \rightarrow \infty } \int _{\Omega }\left| h_{j}-h\right| \textrm{d} x=0 \\&\lim _{j \rightarrow \infty } \int _{\Omega } \sqrt{1+\left| \nabla h_{j}\right| ^{2}} \mathrm {~d} x=\int _{\Omega } \sqrt{1+|D h|^{2}} \end{aligned} \end{aligned}$$

Moreover, the trace of each $h_{j}$ on $\partial \Omega$ coincides with the trace of h.

Based on this lemma, the remaining problem is to show $h_j\geqslant 0$ and $\lim _{m\rightarrow \infty }{\mathcal {L}}^n({\text {Supp}}(h_j))\leqslant {\mathcal {L}}^n({\text {Supp}}(h)).$

From the proof of Lemma B.1 in Ref. Bildhauer (2003), we know the recovery sequence is

$$\begin{aligned} h_{j}=\sum _{i=1}^{\infty } \eta _{\varepsilon _{j,i}} *\left( \varphi _{i} h\right) , \end{aligned}$$

where $\{\varepsilon _{j,i}\}$ are sufficiently small. $\{\varphi _{i}\}$ is a partition of unity, i.e., for a covering $\{U_i\}$ of $\Omega$,

$$\begin{aligned} \varphi _{i} \in C_{0}^{\infty }\left( U_{i}\right) , \quad 0 \leqslant \varphi _{i} \leqslant 1, \quad \sum _{i=1}^{\infty } \varphi _{i}=1. \end{aligned}$$

Since $\varphi _i\geqslant 0$ and $h\geqslant 0 \ a.e.$ in $\Omega$, from property (a) of the mollifiers, we know $h_j\geqslant 0$.

For any $\delta >0$, we can select a sequence $\{\varepsilon _{j,i}\}$ such that $\varepsilon _{j,i}<\delta$. From property (b) of the mollifiers and $h\geqslant 0 \ a.e.$ in $\Omega$,

$$\begin{aligned} \lim \limits _{j\rightarrow \infty }{\mathcal {L}}^n({\text {Supp}}(h_j))&= \lim \limits _{j\rightarrow \infty }{\mathcal {L}}^n\bigg ({\text {Supp}}(\sum _{i=1}^{\infty } \eta _{\varepsilon _{j,i}} *\left( \varphi _{i} h\right) )\bigg )\\&= \lim \limits _{j\rightarrow \infty }{\mathcal {L}}^n\bigg (\cup _i{\text {Supp}}( \eta _{\varepsilon _{j,i}} *\left( \varphi _{i} h\right) )\bigg )\\&\leqslant \lim \limits _{j\rightarrow \infty }{\mathcal {L}}^n\big (\cup _i\{x:{\text {dist}}(x,{\text {Supp}}(\varphi _{i}h))<\delta \} \big )\\&\leqslant \lim \limits _{j\rightarrow \infty }{\mathcal {L}}^n\big (\{x:{\text {dist}}(x,{\text {Supp}}(h))<\delta \}\big )\\&={\mathcal {L}}^n\big (\{x:{\text {dist}}(x,{\text {Supp}}(h))<\delta \}\big ), \end{aligned}$$

Let $\delta \rightarrow 0$,

$$\begin{aligned} \lim _{j\rightarrow \infty }{\mathcal {L}}^n({\text {Supp}}(h_j))\leqslant {\mathcal {L}}^n({\text {Supp}}(h)). \end{aligned}$$