Dynamics of two-strain influenza model with cross-immunity and no quarantine class

Abstract

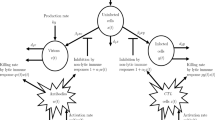
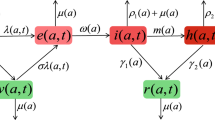
The question about whether a periodic solution can exists for a given epidemiological model is a complicated one and has a long history (Hethcote and Levin, Applied math. ecology, biomathematics, vol 18. Springer, Berlin, pp 193–211, 1989). For influenza models, it is well known that a periodic solution can exists for a single-strain model with periodic contact rate (Aron and Schwartz, J Math Biol 110:665–679, 1984; Kuznetsov and Piccardi, J Math Biol 32:109–121, 1994), or a multiple-strain model with cross-immunity and quarantine class or age-structure (Nuño et al., Mathematical epidemiology. Lecture notes in mathematics, vol 1945. Springer, Berlin, 2008, chapter 13). In this paper, we prove the local asymptotic stability of the interior steady-state of a two-strain influenza model with sufficiently close cross-immunity and no quarantine class or age-structure. We also show that if the cross-immunity between two strains are far apart; then it is possible for the interior steady-state to lose its stability and bifurcation of periodic solutions can occur. Our results extend those obtained by Nuño et.al. (SIAM J Appl Math 65:964–982, 2005). This problem is important because understanding the reasons behind periodic outbreaks of seasonal flu is an important issue in public health.

Appendices

Appendix 1: Proof of (5.4)

We show how to simplify E and G. Let $G_i := \sigma _{ij}\sigma _{ji}(\sigma _{ji}x_{i1}+1) + \mathcal {R}_i^0S\sigma _{ij}(1-\sigma _{ji})\,[x_{i1}(1+\sigma _{ji})+x_{j1}(1+\sigma _{ij})+2].$ Then $G = x_{11}x_{21}\gamma _1^2\gamma _2^2(G_1+G_2)$. Using (3.7), (3.8), and $S(1+x_{11}+x_{21}) = 1$, we have, after some algebra,

$$\begin{aligned} G_i= & {} \sigma _{ij}\sigma _{ji}\mathcal {R}_i^0S\,[\sigma _{ji}(x_{11}+x_{21})+1]+ \mathcal {R}_i^0\sigma _{ij}(1-\sigma _{ji})\,[S(\sigma _{ji}x_{i1}+\sigma _{ij}x_{j1}+1)+1]\\= & {} \mathcal {R}_i^0S\sigma _{ij}\,(\sigma _{ji}x_{i1}+\sigma _{ij}x_{j1}+1) + \mathcal {R}_i^0 S \sigma _{ij}\sigma _{ji}x_{j1}(\sigma _{ji}-\sigma _{ij}) + \mathcal {R}_i^0\sigma _{ij}(1-\sigma _{ji})\\= & {} \sigma _{ij}(\sigma _{ji}x_{i1}+1) + \mathcal {R}_i^0S\sigma _{ij}x_{j1}(1-\sigma _{ji})(\sigma _{ij}-\sigma _{ji})+\mathcal {R}_i^0\sigma _{ij}(1-\sigma _{ji})\\= & {} \mathcal {R}_i^0 \sigma _{ij}\{1+S(1-\sigma _{ji})\,[(\sigma _{ij}-\sigma _{ji})x_{j1}+1]\}. \end{aligned}$$

Similarly, $E = -\gamma _1\gamma _2(x_{11}\gamma _1E_1+x_{21}\gamma _2E_2)$, where

$$\begin{aligned} E_i= & {} \sigma _{ji}(\sigma _{ji}x_{i1}+1) + \mathcal {R}_i^0S(1-\sigma _{ji})(\sigma _{ji}x_{i1}+x_{11}+x_{21}+1)\\&+\, (\mathcal {R}_1^0S)(\mathcal {R}_2^0S)\sigma _{ji}x_{ji}(1-\sigma _{ij})\\= & {} \sigma _{ji}\mathcal {R}_i^0S[\sigma _{ji}(x_{11}+x_{21})+1] + \mathcal {R}_i^0(1-\sigma _{ji})(S\sigma _{ji}x_{i1}+1)\nonumber \\&+\, (\mathcal {R}_1^0S)(\mathcal {R}_2^0)\sigma _{ji}x_{ji}(1-\sigma _{ij})\\= & {} \mathcal {R}_i^0 S\sigma _{ji}(\sigma _{ji}x_{j1}+x_{i1}+1) + \mathcal {R}_i^0(1-\sigma _{ji})+(\mathcal {R}_1^0S)(\mathcal {R}_2^0S)\sigma _{ji}x_{ji}(1-\sigma _{ij})\\= & {} \mathcal {R}_i^0\sigma _{ji}[1-S(1-\sigma _{ji})x_{j1}] + \mathcal {R}_i^0(1-\sigma _{ji})+(\mathcal {R}_1^0S)(\mathcal {R}_2^0S)\sigma _{ji}x_{ji}(1-\sigma _{ij})\\= & {} \mathcal {R}_i^0\{1-S\sigma _{ji}x_{ji}[1-\sigma _{ji}-\mathcal {R}_j^0S(1-\sigma _{ij})]\}. \end{aligned}$$

One can then prove (1) by using mathematical software to show that $\mathcal {H}$, defined by (5.2) with E and G given above, is same as the right side of (5.4) with $q_{ij}$ defined by (5.3).

Appendix 2: Proof of (5.5)

Let $e_i = \mathcal {R}_i^0J_j$. Then $Q = q_{11}q_{22} - q_{12}^2/4 >0$ is equivalent to

$$\begin{aligned} T := 2h_1e_2L + 2 h_2e_1L - L^2 - 4Ke_1 e_2 - (h_1e_2 - h_2 e_1)^2 > 0. \end{aligned}$$

Recall from Lemma 5.4 $D_i = \mathcal {R}_i^0(x_{j1}^0\sigma )(1-\mathcal {R}_j^0S^0)$. Then $e_i = \mathcal {R}_i^0 - D_iS^0(1-\sigma )$. Using mathematical software, one can show that

$$\begin{aligned} T = T_1 + T_{21}D_1 + T_{22}D_2 - T_{23}, \end{aligned}$$

where

$$\begin{aligned} T_1= & {} (\mathcal {R}_1^0+\mathcal {R}_2^0)\sigma (S^0)^2(1-\sigma )^2\,[4\mathcal {R}_1^0\mathcal {R}_2^0 - (\mathcal {R}_1^0+\mathcal {R}_2^0)\sigma ]\nonumber \\ T_{2i}= & {} [4\mathcal {R}_1^0\mathcal {R}_2^0\sigma - 2(\mathcal {R}_1^0+\mathcal {R}_2^0)\sigma (\mathcal {R}_j^0S^0(1-\sigma )+\sigma )](S^0)^2(1-\sigma )^2\nonumber \\ T_{23}= & {} [\sigma ^2(D_1+D_2)^2 + 4(\mathcal {R}_1^0+\mathcal {R}_2^0)\sigma S^0(1-\sigma )D_1D_2](S^0)^2(1-\sigma )^2.\nonumber \end{aligned}$$

Note that $T_1, T_{2i}$ and $T_{23}$ have the common factor $\sigma (S^0)^2(1-\sigma )^2$. Let $T_1^{\,\prime }, T_{2i}^{\,\prime }$ and $T_{23}^{\,\prime }$ be $T_1, T_{2i}$ and $T_{23}$ without this common factor, respectively. Then the first term in (5.5) is $T_1^{\,\prime }$ and the last two terms equal $T_{23}^{\,\prime }$. If we use mathematical software to calculate the difference between $T_{21}^{\,\prime }D_1 + T_{22}^{\,\prime }D_2$ and the second term of (5.5), we obtain $2S^0(1-\sigma )(\mathcal {R}_1^0-\mathcal {R}_2^0)(D_1\mathcal {R}_2^0- D_2\mathcal {R}_1^0)$. This expression equals zero because in the discussion after (5.6), we note that $x_{21}^0\sigma (1-\mathcal {R}_2^0S^0) = x_{11}^0\sigma (1-\mathcal {R}_1^0S^0)$, which implies that $D_1\mathcal {R}_2^0 = D_2\mathcal {R}_1^0$. The proof of Lemma 5.4 is complete.