Integrability of the Zakharov-Shabat Systems by Quadrature

Abstract

We consider the general two-dimensional Zakharov-Shabat systems, which appear in application of the inverse scattering transform (IST) to an important class of nonlinear partial differential equations (PDEs) called integrable systems. We study their integrability in the meaning of differential Galois theory, i.e., their solvability by quadrature. It becomes a key for obtaining analytical expressions for solutions to the PDEs by using the IST. For a wide class of potentials, we prove that they are integrable in that meaning if and only if the potentials are reflectionless. It is well known that for such potentials particular solutions called n-solitons in the original PDEs are yielded by the IST.

Appendices

Appendix A. Relations on Scattering and Reflection Coefficients between (1.1) and (2.6)

Following Sect. 3d of [24] basically, we define the scattering and reflection coefficients for (2.6) (see also Sect. 9.1 of [1]). Equation (2.6) has the Jost solutions

$$\begin{aligned} \begin{aligned}&{\hat{\phi }}(x;k)\sim e^{-ikx}\quad \text {as }x\rightarrow -\infty ,\\&{\hat{\psi }}(x;k)\sim e^{ikx}\quad \text {as }x\rightarrow +\infty . \end{aligned} \end{aligned}$$

(A.1)

Since

$$\begin{aligned} \begin{aligned}&{\hat{\phi }}(x;-k)\sim e^{ikx}\quad \text {as }x\rightarrow -\infty ,\\&{\hat{\psi }}(x;-k)\sim e^{-ikx}\quad \text {as }x\rightarrow +\infty , \end{aligned} \end{aligned}$$

we have the relations

$$\begin{aligned} \begin{aligned}&\phi (x;k)=-\frac{i}{2k}\begin{pmatrix} -{\hat{\phi }}_x(x;k)+ik {\hat{\phi }}(x;k)\\ {\hat{\phi }}(x;k) \end{pmatrix},\\&{\bar{\phi }}(x;k)=\begin{pmatrix} -{\hat{\phi }}_x(x;-k)+ik {\hat{\phi }}(x;-k)\\ {\hat{\phi }}(x;-k) \end{pmatrix},\\&\psi (x;k)=\begin{pmatrix} -{\hat{\psi }}_x(x;k)+ik {\hat{\psi }}(x;k)\\ {\hat{\psi }}(x;k) \end{pmatrix},\\&{\bar{\psi }}(x;k)=-\frac{i}{2k}\begin{pmatrix} -{\hat{\psi }}_x(x;-k)+ik {\hat{\psi }}(x;-k)\\ {\hat{\psi }}(x;-k) \end{pmatrix} \end{aligned} \end{aligned}$$

(A.2)

between the Jost solutions to (1.1) and (2.6) by (2.7). Define the scattering coefficients ${\hat{a}}(k)$ and ${\hat{b}}(k)$ for (2.6) as

$$\begin{aligned} {\hat{\phi }}(x;k)={\hat{a}}(k){\hat{\psi }}(x;-k)+{\hat{b}}(k){\hat{\psi }}(x;k) \end{aligned}$$

(A.3)

like (2.5). Since

$$\begin{aligned} {\hat{\phi }}(x;-k)={\hat{a}}(-k){\hat{\psi }}(x;k)+{\hat{b}}(-k){\hat{\psi }}(x;-k), \end{aligned}$$

we have

$$\begin{aligned} {\hat{a}}(k){\hat{a}}(-k)-{\hat{b}}(k){\hat{b}}(-k)=1 \end{aligned}$$

(A.4)

like (4.2). From (A.2) we obtain

$$\begin{aligned}&\phi (x;k)={\hat{a}}(k){\bar{\psi }}(x;k)-\frac{i}{2k}{\hat{b}}(k)\psi (x;k),\\&{\bar{\phi }}(x;k)={\hat{a}}(-k)\psi (x;k)+2ik{\hat{b}}(-k){\bar{\psi }}(x;k), \end{aligned}$$

which are compared with (2.5) to yield

$$\begin{aligned} a(k)={\hat{a}}(k),\quad {\bar{a}}(k)={\hat{a}}(-k),\quad b(k)=-\frac{i}{2k}{\hat{b}}(k),\quad {\bar{b}}(k)=2ik{\hat{b}}(-k). \end{aligned}$$

(A.5)

Moreover, for the reflection coefficients we have

$$\begin{aligned} \rho (k)=-\frac{i}{2k}{\hat{\rho }}(k),\quad {\bar{\rho }}(k)=2ik{\hat{\rho }}(-k), \end{aligned}$$

(A.6)

where ${\hat{\rho }}(k)={\hat{b}}(k)/{\hat{a}}(k)$.

Appendix B. Condition for the Simplicity of Zeros of

We consider the linear Schrödinger equation (2.6) and follow an approach in the proof of Theorem 1 of [14] to provide a necessary and sufficient condition for a zero of the scattering coefficient ${\hat{a}}(k)$ to be simple.

Let ${\hat{\phi }}(x;k),{\hat{\psi }}(x;k)$ be the Jost solutions satisfying (A.1). Since

$$\begin{aligned}{}[{\hat{\psi }}(x;k),{\hat{\psi }}(x;-k)] :={\hat{\psi }}_x(x,k){\hat{\psi }}(x;-k)-{\hat{\psi }}(x,k){\hat{\psi }}_x(x;-k)=2ik \end{aligned}$$

by (A.1), we have

$$\begin{aligned}{}[{\hat{\psi }}(x;k),{\hat{\phi }}(x;k)]=[{\hat{\psi }}(x,k),{\hat{a}}(k){\hat{\psi }}(x;-k)+{\hat{b}}(k){\hat{\psi }}(x;k)] =2ik{\hat{a}}(k) \end{aligned}$$

by (A.3). Hence,

$$\begin{aligned} \frac{\textrm{d}}{\textrm{d}k}(2ik{\hat{a}}(k)) =&\quad 2i{\hat{a}}(k)+2ik{\hat{a}}_k(k)\nonumber \\ =&\quad [{\hat{\psi }}_k(x;k),{\hat{\phi }}(x;k)]+[{\hat{\psi }}(x;k),{\hat{\phi }}_k(x;k)]. \end{aligned}$$

(B.1)

On the other hand, since ${\hat{\psi }}(x;k)$ is a solution to (2.6), we immediately have

$$\begin{aligned} {\hat{\psi }}_{kxx}(x;k)+q(x){\hat{\psi }}_k(x;k) =-k^2{\hat{\psi }}_k(x;k)-2k{\hat{\psi }}(x;k), \end{aligned}$$

so that

$$\begin{aligned} \frac{\textrm{d}}{\textrm{d}x}[{\hat{\psi }}_k(x;k),{\hat{\phi }}(x;k)]&= {\hat{\psi }}_{kxx}(x;k){\hat{\phi }}(x;k)-{\hat{\psi }}_k(x;k){\hat{\phi }}_{xx}(x;k)\nonumber \\&= -2k{\hat{\psi }}(x;k){\hat{\phi }}(x;k). \end{aligned}$$

(B.2)

Similarly, we have

$$\begin{aligned} \frac{\textrm{d}}{\textrm{d}x}[{\hat{\psi }}(x;k),{\hat{\phi }}_k(x;k)]=2k{\hat{\psi }}(x;k){\hat{\phi }}(x;k). \end{aligned}$$

(B.3)

Let $k=k_0$ be a zero of ${\hat{a}}(k)$ with $\textrm{Im}\,k_0>0$. Then by (A.1) and (A.3) we have

$$\begin{aligned} \lim _{x\rightarrow +\infty }{\hat{\psi }}_k(x;k_0){\hat{\phi }}(x;k_0)=0,\quad \lim _{x\rightarrow -\infty }{\hat{\psi }}(x;k_0){\hat{\phi }}_k(x;k_0)=0. \end{aligned}$$

Hence, it follows from (B.2) and (B.3) that

$$\begin{aligned}&[{\hat{\psi }}_k(x;k_0),{\hat{\phi }}(x;k_0)] =2k_0\int _x^\infty {\hat{\psi }}(x;k_0){\hat{\phi }}(x;k_0)\textrm{d}x,\\&[{\hat{\psi }}(x;k_0),{\hat{\phi }}_k(x;k_0)] =2k_0\int _{-\infty }^x{\hat{\psi }}(x;k_0){\hat{\phi }}(x;k_0)\textrm{d}x, \end{aligned}$$

which yield

$$\begin{aligned} i{\hat{a}}_k(k_0)=\int _{-\infty }^\infty {\hat{\psi }}(x;k_0){\hat{\phi }}(x;k_0)\textrm{d}x \end{aligned}$$

along with (B.1). Using (A.3), we have the following proposition.

Proposition B .4

The zero $k=k_0$ of ${\hat{a}}(k)$ with $\textrm{Im}\,k_0>0$ is simple if and only if

$$\begin{aligned} \int _{-\infty }^\infty {\hat{\psi }}(x;k_0)^2\textrm{d}x\ne 0. \end{aligned}$$

(B.4)

Remark B .5

Assume that q(x) is real on $\mathbb {R}$ and let $k=k_0$ be a zero of ${\hat{a}}(k)$. Then $k_0$ is purely imaginary as stated in Remark 5.4(ii) and we can take a real function on $\mathbb {R}$ as ${\hat{\psi }}(x;k_0)$, which is a solution to (2.6) with $k=k_0$ such that $\lim _{x\rightarrow {\pm } 0}w(x)=0$. Hence, condition (B.4) holds. Thus, any zero of ${\hat{\psi }}(x;k_0)$ is simple, as shown in [14].

Appendix C. Computation of 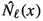, $\ell$ = $1,\ldots ,n$, in a Simple Case

We consider the linear Schrödinger equation (2.6) and compute ${\hat{N}}_\ell (x)$, $\ell =1,\ldots ,n$, which were defined in Sect. 4.2, when the zeros $k=k_j$, $j=1,\ldots ,n$, of ${\hat{a}}(k)$ are all simple.

Substituting (4.11) into (4.10) and setting $k=k_\ell$, we have

$$\begin{aligned} {\hat{N}}_\ell (x) =e^{2ik_\ell x}\biggl ( 1-\sum _{j=1}^n\frac{{\hat{C}}_j{\hat{N}}_j(x)}{k_\ell +k_j}\biggr ),\quad \ell =1,\ldots ,n, \end{aligned}$$

(C.1)

where

$$\begin{aligned} {\hat{C}}_j=\frac{{\hat{b}}(k_j)}{{\hat{a}}_k(k_j)},\quad j=1,\ldots ,n. \end{aligned}$$

Let

$$\begin{aligned}&\Delta (x)\\&=\begin{vmatrix} 1+(2k_1)^{-1}{\hat{C}}_1e^{2ik_1x}&(k_1+k_2)^{-1}{\hat{C}}_2e^{2ik_1x}&\cdots&(k_1+k_n)^{-1}{\hat{C}}_ne^{2ik_1x}\\ (k_2+k_1)^{-1}{\hat{C}}_1e^{2ik_2x}&1+(2k_2)^{-1}{\hat{C}}_2e^{2ik_2x}&\cdots&(k_2+k_n)^{-1}{\hat{C}}_ne^{2ik_2x}\\ \vdots&\vdots&\ddots&\vdots \\ (k_n+k_1)^{-1}{\hat{C}}_1e^{2ik_nx}&(k_n+k_1)^{-1}{\hat{C}}_2e^{2ik_nx}&\cdots&1+(2k_n)^{-1}{\hat{C}}_ne^{2ik_2x} \end{vmatrix} \end{aligned}$$

and let $\Delta _\ell (x)$, $\ell =1,\ldots ,n$, be the determinants of the matrices obtained by replacing the $\ell$th column of $\Delta (x)$ with

$$\begin{aligned} \begin{pmatrix} e^{2ik_1x}\\ e^{2ik_2x}\\ \vdots \\ e^{2ik_nx} \end{pmatrix} \end{aligned}$$

Using Cramer’s rule, we represent the solution to the system of linear algebraic equations (C.1) as

$$\begin{aligned} {\hat{N}}_\ell (x)=\Delta _\ell (x)/\Delta (x),\quad \ell =1,\ldots ,n. \end{aligned}$$

In particular, ${\hat{N}}_\ell (x)$, $\ell =1,\ldots ,n$, are rational functions of $e^{2ik_jx}$, $j=1,\ldots ,n$.