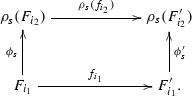Abstract
We will adopt an elementary approach to \(\fancyscript{D}\)-modules on Ran spaces in terms of two-limits; the aim here is to define the category of coherent \(\fancyscript{D}\)-modules, characteristic varieties and non-characteristic maps. An application will be the proof of the Cauchy–Kowaleski–Kashiwara theorem in this setting.
Similar content being viewed by others
References
Beilinson, A., Drinfeld, V.: Chiral Algebras, vol. 51. A. M. S. Colloquium Publications. American Mathematical Society, Providence (2004)
Francis, J., Gaitsgory, D.: Chiral Koszul Duality (2011). arXiv:1103.5803
Guillermou, S., Schapira, P.: Operations on Projective Limits of Categories of Sheaves (2006, unpublished)
Kashiwara, M.: Algebraic Study of Systems of Partial Differential Equations. Thesis, Tokyo University (1970). Translated by A. D’Agnolo and J-P. Schneiders, Mémoires Soc. Math. France 63 (1995)
Kashiwara, M.: On the maximally overdetermined system of linear differential equations I. Publ. Res. Inst. Math. Sci. 10, 563–579 (1974/1975)
Kashiwara, M.: D-Modules and Microlocal Calculus, Translations of Mathematical Monographs, vol. 217. American Mathematical Society, Providence (2003)
Kashiwara, M., Schapira, P.: Sheaves on Manifolds, vol. 292. Grundlehren der Mathematischen Wissenschaften, Springer, Berlin (1990), x+512 pp
Kashiwara, M., Schapira, P.: Categories and Sheaves, Grundlehren der Mathematischen Wissenschaften [Fundamental Principles of Mathematical Sciences], vol. 332. Springer, Berlin (2006)
Kashiwara, M., Schapira, P.: Deformation Quantization Modules (2010). arXiv:1003.3304
S-G-A 4: Théorie des topos et cohomologie étale des schémas, Sém. Géom. Algébrique (1963–1964) by M. Artin, A. Grothendieck and J.-L. Verdier, Lecture Notes in Mathematics vols. 260, 270, 305, Springer (1972/1973)
Acknowledgments
I am deeply grateful to Pierre Schapira for having called my attention on the study of coherent \(\fancyscript{D}\)-modules over Ran spaces. I have much benefited from the reading of the unpublished manuscript [3] and I wish to kindly thank the authors. Moreover I thank the Luxembourgian National Research Fund for support via AFR Grant Ph.D. 09-072.
Author information
Authors and Affiliations
Corresponding author
Appendix
Appendix
There are two other natural categories, \(\mathrm{{\mathfrak {S}} }^+(\mathcal{{I}})\) and \(\mathrm{{\mathfrak {S}} }^-(\mathcal{{I}})\).
Definition 6.13
-
(a)
An object \(F\) of \(\mathrm{{\mathfrak {S}} }^+(\mathcal{{I}})\) (resp. \(\mathrm{{\mathfrak {S}} }^-(\mathcal{{I}})\)) is a family \(\{(F_i, \phi _s)\}_{i,s}\) (\(i\in \mathcal{{I}}\), \(s\in \mathrm{Mor }(\mathcal{{I}})\)) where
-
(i)
for any \(i\in \mathcal{{I}}\), \(F_i\) is an object of \(\mathrm{{\mathfrak {S}} }(i)\),
-
(i)
for any morphism \(s:i_1\xrightarrow []{}i_2\) in \(\mathcal{{I}}\), \(\phi _s :F_{i_1} \xrightarrow []{}\rho _s (F_{i_2})\) (resp. \(\phi _s :\rho _s (F_{i_2}) \xrightarrow []{}F_{i_1}\)) is a morphism such that
-
for all \(i \in \mathcal{{I}}\), \(\phi _{{{\mathrm{id}}}_i} = {{\mathrm{id}}}_{F_i}\),
-
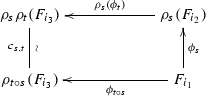
for any sequence \(i_1\xrightarrow []{s}i_2\xrightarrow []{t}i_3\) of morphisms in \(\mathcal{{I}}\), the following diagram commutes
 (6.3)
(6.3)(resp. the following diagram commutes
 (6.4)
(6.4)
-
-
(i)
-
(b)
A morphism \(f:\{(F_i,\phi _s)\}_{i,s}\xrightarrow []{}\{(F'_i,\phi '_s)\}_{i,s}\) in \(\mathrm{{\mathfrak {S}} }^+(\mathcal{{I}})\) (resp. \(\mathrm{{\mathfrak {S}} }^-(\mathcal{{I}})\)) is a family of morphisms \(f_i:F_i\xrightarrow []{}F'_i\) such that for any \(s:i_1\xrightarrow []{}i_2\), the diagram below commutes:
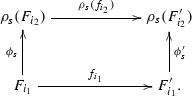 (6.5)
(6.5)(resp. the diagram below commutes:
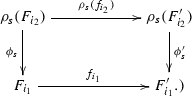 (6.6)
(6.6)
We consider \(\mathrm{{\mathfrak {S}} }(\mathcal{{I}})\) as the full subcategory of \(\mathrm{{\mathfrak {S}} }^+(\mathcal{{I}})\) or \(\mathrm{{\mathfrak {S}} }^-(\mathcal{{I}})\) consisting of objects \(\{(F_i, \phi _s)\}_{i\in \mathcal{{I}},s\in \mathrm{Mor }(\mathcal{{I}})}\) such that for all \(s\in \mathrm{Mor }(\mathcal{{I}})\), the morphisms \(\phi _s\) are isomorphisms and we denote by \(\iota ^+_\mathcal{{I}}:\mathrm{{\mathfrak {S}} }(\mathcal{{I}}) \xrightarrow []{}\mathrm{{\mathfrak {S}} }^+(\mathcal{{I}})\) and \(\iota ^-_\mathcal{{I}}:\mathrm{{\mathfrak {S}} }(\mathcal{{I}}) \xrightarrow []{}\mathrm{{\mathfrak {S}} }^-(\mathcal{{I}})\) the natural faithful functors.
Rights and permissions
About this article
Cite this article
Bonavolontà, G. The Cauchy problem for \(\fancyscript{D}\)-modules on Ran spaces. Math. Z. 280, 149–163 (2015). https://doi.org/10.1007/s00209-015-1416-9
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00209-015-1416-9