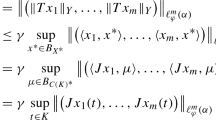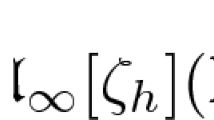Skip to main content
SpringerLink
Log in

There is no largest proper operator ideal

  • Published:
Mathematische Annalen Aims and scope Submit manuscript

Abstract

An operator ideal is proper if the only invertible operators it contains have finite rank. We answer a problem posed by Pietsch (Operator ideals, North-Holland, Amsterdam, 1980) by proving (i) that the ideal of inessential operators is not maximal among proper operator ideals, and (ii) that there is no largest proper operator ideal. Our proof is based on an extension of the construction by Aiena and González (Math Z 233:471–479, 2000), of an improjective but essential operator on Gowers–Maurey’s shift space \(X_S\) (Math Ann 307:543–568, 1997), through a new analysis of the algebra of operators on powers of \(X_S\). We also prove that certain properties hold for general \(\mathbb {C}\)-linear operators if and only if they hold for these operators seen as real: for example this holds for operators belonging to the ideals of strictly singular, strictly cosingular, or inessential operators, answering a question of González and Herrera (Stud Math 183(1):1–14, 2007). This gives us a frame to extend the negative answer to the problem of Pietsch to the real setting.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Data Availability

Not applicable.

References

  1. Aiena, P., González, M.: Examples of improjective operators. Math. Z. 233, 471–479 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  2. Anisca, R.: Subspaces of \(L_p\) with more than one complex structure. Proc. Am. Math. Soc. (131) 9, 2819–2829 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  3. Anisca, R., Ferenczi, V., Moreno, Y.: On the classification of complex structures and positions in Banach spaces. J. Funct. Anal. 272, 3845–3868 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  4. Blackadar, B.: K-theory for operator algebras. MSRI Publications 5, Springer, Berlin (1986)

  5. Bourgain, J.: Real isomorphic Banach spaces need not be complex isomorphic. Proc. Am. Math. Soc. (96) 2, 221–226 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  6. Burlando, L.: On subspaces of direct sums of infinite sequences of Banach spaces. Atti Accad. Ligure Sci. Lett. 46, 96–105 (1989)

    MathSciNet  MATH  Google Scholar 

  7. Cuellar Carrera, W.: Um espaço de Banach não isomorfo a seu conjugado complexo (A Banach space not isomorphic to its complex conjugate). Master’s Dissertation, Universidade de São Paulo (2011)

  8. Cuellar Carrera, W.: A Banach space with a countable infinite number of complex structures. J. Funct. Anal. 267(5), 1462–1487 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  9. Cuellar Carrera, W.: Complex structures on Banach spaces with a subsymmetric basis. J. Math. Anal. App. 440(2), 624–635 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  10. Dieudonné, J.: Complex structures on real Banach spaces. Proc. Am. Math. Soc. (3) 1, 162–164 (1952)

    Article  MathSciNet  MATH  Google Scholar 

  11. Edelstein, I.E., Wojtaszczyk, P.: On projections and unconditional bases in direct sums of Banach spaces. Stud. Math. 56, 263–276 (1976)

    Article  MathSciNet  MATH  Google Scholar 

  12. Ferenczi, V.: Uniqueness of complex structure and real hereditarily indecomposable Banach spaces. Adv. Math. 213(1), 462–488 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  13. Ferenczi, V., Galego, E.M.: Even infinite-dimensional real Banach spaces. J. Funct. Anal. 253, 534–549 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  14. Ferenczi, V., Galego, E.M.: Countable groups of isometries. Trans. Am. Math. Soc. 362(8), 4385–4431 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  15. González, M.: On essentially incomparable Banach spaces. Math. Z. 215, 621–629 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  16. González, M., Herrera, J.: Decompositions for real Banach spaces with small spaces of operators. Stud. Math. 183(1), 1–14 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  17. Gowers, W.T., Maurey, B.: The unconditional basic sequence problem. J. Am. Math. Soc. (6) 4, 851–874 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  18. Gowers, W.T., Maurey, B.: Banach spaces with small spaces of operators. Math. Ann. 307, 543–568 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  19. Horváth, B.: Banach spaces whose algebras of operators are Dedekind-finite but they do not have stable rank one, Banach Algebras and Applications (Proceedings of the International Conference held at the University of Oulu, July 3–11, 2017). De Gruyter, pp. 165–176 (2020)

  20. Kalton, N.J.: An elementary example of a Banach space not isomorphic to its complex conjugate. Can. Math. Bull. 38(2), 218–222 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  21. Kalton, N.J.: Twisted Hilbert spaces and unconditional structure. J. Inst. Math. Jussieu 2(3), 401–408 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  22. Kalton, N.J., Peck, N.T.: Twisted sums of sequence spaces and the three space problem. Trans. Am. Math. Soc. 255, 1–30 (1979)

    Article  MathSciNet  MATH  Google Scholar 

  23. Koszmider, P., Martin, M., Meri, J.: Extremely non-complex \(C(K)\)-spaces. J. Math. Anal. Appl. 350(2), 601–615 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  24. Lebow, A., Schechter, M.: Semigroups of operators and measures of noncompactness. J. Funct. Anal. 7, 1–26 (1971)

    Article  MathSciNet  MATH  Google Scholar 

  25. Lindenstrauss, J., Tzafriri, L.: Classical Banach Spaces. Springer, New York (1979)

    Book  MATH  Google Scholar 

  26. Maurey, B.: Operator theory and exotic Banach spaces. 1996. Notes available on the author’s webpage

  27. Maurey, B.: Banach spaces with few operators. In: Handbook of the Geometry of Banach Spaces, Vol. 2. Elsevier, Oxford, pp. 1247–1297 (2003) (Edited by William B. Johnson and Joram Lindenstrauss)

  28. Maurey, B.: Théorie spectrale et opérateurs sur un espace HI réel. 2020. Notes available on the author’s webpage

  29. Pietsch, A.: Operator Ideals. North-Holland, Amsterdam (1980)

    MATH  Google Scholar 

  30. Szarek, S.: A super reflexive Banach space which does not admit complex structure. Proc. Am. Math. Soc. (97) 3, 437–444 (1986)

    Article  MATH  Google Scholar 

  31. Tarafdar, E.: Improjective operators and ideals in a category of Banach spaces. J. Austral. Math. Soc. 14, 274–292 (1972)

    Article  MathSciNet  MATH  Google Scholar 

  32. Wiener, N.: On the representation of functions by trigonometrical integrals. Math. Z. 24(1), 575–616 (1926)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The author warmly thanks Manuel González for drawing his attention to the theory of proper operator ideals and the questions of Pietsch, for suggesting to compare the real and complex versions of classical ideals, as well as for useful comments on a first draft of this paper. The author also thanks Antonio Martínez Abejon and Christina Brech for observations and questions leading to improvements of this article. Finally the author is greatly indebted to the anonymous referee, who made the effort of carefully reading the results and correcting numerous imprecisions and mistakes of the original version; their suggestions certainly improved the quality of this paper.

Funding

Partially supported by FAPESP, project 2016/25574-8, and CNPq, grant 303731/2019-2.

Author information

Authors and Affiliations

  1. Departamento de Matemática, Instituto de Matemática e Estatística, Universidade de São Paulo, Rua do Matão 1010, São Paulo, SP, 05508-090, Brazil

    Valentin Ferenczi

  2. Equipe d’Analyse Fonctionnelle, Institut de Mathématiques de Jussieu, Sorbonne Université-UPMC, Case 247, 4 Place Jussieu, 75252, Paris Cedex 05, France

    Valentin Ferenczi

Authors
  1. Valentin Ferenczi
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

Not applicable.

Corresponding author

Correspondence to Valentin Ferenczi.

Ethics declarations

Conflict of interest

On behalf of all authors, the corresponding author states that there is no conflict of interest.

Code availability

Not applicable.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

The author acknowledges the support of Fundação de Amparo à Pesquisa do Estado de São Paulo, project 2016/25574-8, and of Conselho Nacional de Desenvolvimento Científico e Tecnológico, Grant 303731/2019-2.

Rights and permissions

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ferenczi, V. There is no largest proper operator ideal. Math. Ann. 387, 1043–1072 (2023). https://doi.org/10.1007/s00208-022-02470-0

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00208-022-02470-0

Mathematics Subject Classification

Search

Navigation