A finite axiomatization of positive MV-algebras

Abstract

Positive MV-algebras are the subreducts of MV-algebras with respect to the signature $\{\oplus , \odot , \vee , \wedge , 0, 1\}$. We provide a finite quasi-equational axiomatization for the class of such algebras.

Appendix A. Free MV-extensions

By standard results in general algebra, the forgetful functor U from MV-algebras to positive MV-algebras has a left adjoint F. For every positive MV-algebra $\mathbf {A}$, it is immediate that the component $\iota _{\mathbf {A}} :\mathbf {A} \rightarrow UF(\mathbf {A})$ at $\mathbf {A}$ of the unit is injective, and that the image of $\iota _{\mathbf {A}}$ generates the MV-algebra $F(\mathbf {A})$. We speak of the pair $(F(\mathbf {A}), \iota _{\mathbf {A}})$ (or simply of $F(\mathbf {A})$, leaving $\iota _{\mathbf {A}}$ understood) as the free MV-extension of $\mathbf {A}$. In this section we prove that, given a positive subreduct $\mathbf {A}$ of an MV-algebra $\mathbf {B}$ such that A generates $\mathbf {B}$, and denoting with i the inclusion of A into B, $(\mathbf {B}, i)$ is the free MV-extension of $\mathbf {A}$; moreover, under the same conditions, for every $x \in B$, there are $a_1, \dots , a_n, b_1, \dots , b_n \in A$ such that $x = \bigoplus _{j = 1}^{n} (a_j \odot \lnot b_j)$.

The following Lemma corresponds, essentially, to the fact that Mundici’s bijection between $\mathbf {G}$ and $\Xi (\Gamma (\mathbf {G}))$ preserves $+$. We refer to [2, Proposition 4.67] for a proof in the setting of unital commutative distributive $\ell$-monoids.

Lemma A.1

Let $\mathbf {G}$ be a unital abelian $\ell$-group, and let $x, y \in G$. For each $n \in \mathbb {Z}$, set $x_n \,{:}{=}\,((x -n) \vee 0) \wedge 1)$ and $y_n \,{:}{=}\,((x -n) \vee 0) \wedge 1)$. We have

$$\begin{aligned} ((x + y) \vee 0) \wedge 1 = \bigoplus _{n \in \mathbb {Z}} (x_n \odot y_{-n-1}) = \bigodot _{n \in \mathbb {Z}} (x_n \oplus y_{-n}). \end{aligned}$$

(The expression $\bigoplus _{n \in \mathbb {Z}} (x_n \odot y_{-n-1})$ makes sense because for all but finitely many $n \in \mathbb {Z}$ we have $x_n \odot y_{-n-1} = 0$. Analogously, the expression $\bigodot _{n \in \mathbb {Z}} (x_n \oplus y_{-n})$ makes sense because for all but finitely many $n \in \mathbb {Z}$ we have $x_n \oplus y_{-n} = 1$.)

Lemma A.2

Let $\mathbf {G}$ be a unital abelian $\ell$-group. Set

$$\begin{aligned} \mathcal {M} \,\,{:}{=}\,\, \{ M \subseteq G \mid M \text { is closed under }+, \vee , \wedge , 0, 1, -1 \} \end{aligned}$$

and

$$\begin{aligned} \mathcal {A}\, \,{:}{=}\,\,\{ A \subseteq \Gamma (\mathbf {G}) \mid A \text { is closed under } \oplus , \odot , \vee , \wedge , 0, 1 \}. \end{aligned}$$

• (1) The sets $\mathcal {M}$ and $\mathcal {A}$ are in bijection, as witnessed by the functions

  

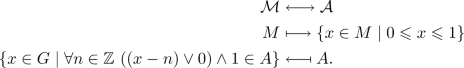
• (2) The bijection in (1) restricts to the subsets

  $$\begin{aligned} \{M \in \mathcal {M} \mid M \text { is closed under }-\} \text { and }\{A \in \mathcal {A} \mid A \text { is closed under }\lnot \}. \end{aligned}$$

• (3) For every $M \in \mathcal {M}$, M generates the unital abelian $\ell$-group $\mathbf {G}$ if and only if $\{ x \in M \mid 0 \leqslant x \leqslant 1 \}$ generates the MV-algebra $\Gamma (\mathbf {G})$.

Proof

The fact that the function $f :\mathcal {M} \rightarrow \mathcal {A}$ is well-defined is immediate.

Let us prove that the function $g :\mathcal {A} \rightarrow \mathcal {M}$ is well-defined. Let $A \in \mathcal {A}$, and set

$$\begin{aligned} M \,\,{:}{=}\,\, \{ x \in G \mid \forall n \in \mathbb {Z}\ ((x - n) \vee 0) \wedge 1 \in A \}. \end{aligned}$$

The set M is closed under $+$ by Lemma A.1. Moreover, it is closed under $\vee$ because, for all $x, y \in M$, we have $((x \vee y) \vee 0) \wedge 1 = ((x \vee 0) \wedge 1) \vee ((x \vee 0) \wedge 1)$. Analogously, it is closed under $\wedge$. It is not difficult to prove that $0, 1, -1 \in M$. Therefore, $M \in \mathcal {M}$, and thus g is well-defined.

It is easy to see that the composite $f \circ g :\mathcal {A} \rightarrow \mathcal {A}$ is the identity on $\mathcal {A}$. To prove that $g \circ f$ is the identity on $\mathcal {M}$, let $M \in \mathcal {M}$. We shall prove that

$$\begin{aligned} M = \{x \in G \mid \forall n \in \mathbb {Z}\ (x - n) \vee 0) \wedge 1 \in M\}. \end{aligned}$$

The left-to-right inclusion is immediate. For the converse inclusion, let $x \in G$ be such that, for every $n \in \mathbb {Z}$, $((x - n) \vee 0) \wedge 1 \in M$. Let $n \in \mathbb {N}$ be such that $-n \leqslant x \leqslant n$. Then (cf. Lemma 3.6)

$$\begin{aligned} x = -n + \sum _{i = -n}^{n-1} (((x - i) \vee 0) \wedge 1), \end{aligned}$$

and thus $x \in M$. This proves (1).

If $M \in \mathcal {M}$ is closed under −, f(M) is easily seen to be closed under $\lnot$. If $A \in \mathcal {A}$ is closed under $\lnot$, g(A) is closed under − because, for every $x \in g(A)$ and every $n \in \mathbb {Z}$, we have

$$\begin{aligned} ((-x - n) \vee 0) \wedge 1 = 1 - (((x + (n+1)) \vee 0) \wedge 1) = \lnot (((x + (n+1)) \vee 0) \wedge 1). \end{aligned}$$

This proves (2).

Since the bijection in (1) preserves the inclusion order in both directions, we have (3). $\square$

It is a well-known fact that, if we consider a Boolean algebra $\mathbf {B}$ generated by a bounded distributive sublattice $\mathbf {D}$, any element of B can be written as a finite join of elements of the form $x \wedge \lnot y$ (or, equivalently, as a finite meet of elements of the form $x \vee \lnot y$) for $x,y \in D$. An analogous result holds for positive MV-algebras and MV-algebras. Whereas the result for Boolean algebras can be derived by standard applications of the distributive and De Morgan’s laws, the corresponding one for MV-algebras is not straightforward. We shall present it in the next theorem.

Theorem A.3

Let $\mathbf {A}$ be a positive subreduct of an MV-algebra $\mathbf {B}$, and suppose that A generates the MV-algebra $\mathbf {B}$. For every $x \in B$ there are $n,m \in \mathbb {N}$ and $s_1, \dots , s_n, t_1, \dots , t_n, u_1, \dots , u_m, v_1, \dots , v_m \in A$ such that

$$\begin{aligned} x = \bigoplus _{i = 1}^{n} s_i \odot \lnot t_i = \bigodot _{i = 1}^{m} u_i \oplus \lnot v_i. \end{aligned}$$

Proof

By Lemma A.2, the set

$$\begin{aligned} M \,{:}{=}\,\{ x \in G \mid \forall n \in \mathbb {Z}\ ((x - n) \vee 0) \wedge 1 \in A \} \end{aligned}$$

is closed under $+$, $\vee$, $\wedge$, 0, 1 and $-1$ and generates the unital abelian $\ell$-group $\mathbf {G}$. Let $z \in B$. By Lemma 4.2, there are $x,y \in M$ such that $z = x - y$. For every $n \in \mathbb {N}$, we have

$$\begin{aligned} ((-y - n + 1) \vee 0) \wedge 1&= 1 - (1 - (((-y - n + 1) \vee 0) \wedge 1))\\&= 1 - (1 + (((y + n - 1) \wedge 0) \vee -1))\\&= 1 - (((y + n) \wedge 1) \vee 0)\\&= 1 - (((y + n) \vee 0) \wedge 1)\\&= \lnot (((y + n) \vee 0) \wedge 1). \end{aligned}$$

Therefore, by Lemma A.1, we have

$$\begin{aligned} z&= \bigoplus _{n \in \mathbb {Z}} \big ((((x - n) \vee 0) \wedge 1) \odot (((-y - n + 1) \vee 0) \wedge 1)\big )\\&= \bigoplus _{n \in \mathbb {Z}} \big ((((x - n) \vee 0) \wedge 1) \odot \lnot (((y + n) \vee 0) \wedge 1)\big ). \end{aligned}$$

Since $x,y \in M$, $((x - n) \vee 0) \wedge 1, ((y + n) \vee 0) \wedge 1 \in A$. This proves the first equality in the statement. The second one is analogous. $\square$

Theorem A.4

Let M be a generating subset of a unital abelian $\ell$-group $\mathbf {G}$, and suppose that M is closed under $+$, $\vee$, $\wedge$, 0, 1 and $-1$. For every unital Abelian $\ell$-group $\mathbf {H}$ and every function $f :M \rightarrow H$ that preserves $+$, $\vee$, $\wedge$, 0, 1 and $-1$, there exists a unique morphism $g :\mathbf {G} \rightarrow \mathbf {H}$ of unital abelian $\ell$-groups that extends f.

Proof

This follows from Lemma 4.2. For every $z \in G$ we define the morphism g as $g(z) = f(x) - f(y)$ where x and y are elements of M such that $z=x-y$. The fact that g is a well-defined morphism follows from the fact that $x - y = u - v$ is equivalent to $x + v = u + y$. $\square$

The following theorem generalizes an analogous result for bounded distributive lattices, namely that the inclusion of a bounded distributive lattice into a Boolean algebra that is generated by the image of such inclusion is universal, i.e. it is a so-called Booleanization, or free Boolean extension: see [11, Theorem 4.1] for a version of this result for (not necessarily bounded) distributive lattices.

Theorem A.5

Let $\mathbf {A}$ be a positive MV-algebra, $\mathbf {B}$ an MV-algebra, and $i :A \hookrightarrow B$ an injective function that preserves $\oplus$, $\odot$, $\vee$, $\wedge$, 0 and 1 and such that its image generates the MV-algebra $\mathbf {B}$. Then $(\mathbf {B}, i)$ is the free MV-extension of $\mathbf {A}$.

Proof

Without loss of generality, we may suppose $A \subseteq B$ and i to be the inclusion of A into B. Let $\mathbf {C}$ be an MV-algebra, and let $f :A \rightarrow C$ be a function that preserves $\oplus$, $\odot$, $\vee$, $\wedge$, 0 and 1. We shall prove that there exists a unique MV-homomorphism $g :\mathbf {B} \rightarrow \mathbf {C}$ that extends f. Uniqueness follows from the fact that A generates the MV-algebra $\mathbf {B}$. Let us prove existence. By the equivalence in Theorem 3.4, we obtain morphisms of unital commutative distributive $\ell$-monoids $\Xi (i) :\Xi (\mathbf {A}) \rightarrow \Xi (\mathbf {B})$ and $\Xi (f) :\Xi (\mathbf {A}) \rightarrow \Xi (\mathbf {C})$. By Proposition 3.7, $\Xi (i)$ is injective, and we may suppose $\Xi (i)$ to be an inclusion. By Theorem A.4, there exists a unique morphism $g' :\Xi (\mathbf {B}) \rightarrow \Xi (\mathbf {C})$ of unital abelian $\ell$-groups that extends $\Xi (f)$. Then, $g \,{:}{=}\,\Gamma (g')$ is an MV-homomorphism that extends f. $\square$