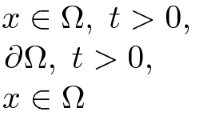Abstract
In this paper, we consider a class of p(x)-Laplacian equations with logarithmic source terms. Using the potential well method combined with the Nehari manifold, we prove some results on the global existence and blow-up of weak solutions in the subcritical case. Moreover, we also obtain decay estimates for the global weak solutions. Otherwise, we give an upper bound for the maximal existence time of the blow-up weak solutions under different levels of initial energy. The main difficulties here not only come from the usual difficulties in variable exponent spaces but also from the sign-changing property of the logarithmic function. Our results improve (Boudjeriou in J Elliptic Parabol Equ 6:773–794 2020) and fill the gaps in Liu et al. (Nonlinear Anal Real World Appl 64:103449, 2022). Notice that the methods here are different from Boudjeriou (2020) and can be used to extend (Zeng et al. in J Nonlinear Math Phys 29:41–57, 2022).
Similar content being viewed by others
References
Aboulaich, R., Meskine, D., Souissi, A.: New diffusion models in image processing. Comput. Math. Appl. 56, 874–882 (2008)
Antontsev, S., Rodrigues, J.: On stationary thermo-rheological viscous flows. Ann. Univ. Ferrara Sez. VII Sci. Mat. 52(1), 19–36 (2006)
Bollt, E., Chartrand, R., Esedo\(\bar{\text{g}}\)lu, S., Schultz, P., Vixie, K.: Graduated adaptive image denoising: local compromise between total variation and isotropic diffusion. Adv. Comput. Math. 31(1–3), 61–85 (2009)
Boudjeriou, T.: Global existence and blow-up for the fractional p-Laplacian with logarithmic nonlinearity. Mediterr. J. Math. 17, 162 (2020)
Boudjeriou, T.: On the diffusion \(p(x)\)-Laplacian with logarithmic nonlinearity. J. Elliptic Parabol. Equ. 6, 773–794 (2020)
Cao, Y., Liu, C.H.: Initial boundary value problem for a mixed pseudoparabolic p-Laplacian type equation with logarithmic nonlinearity. Electron. J. Differ. Equ. 116(2018), 1–19 (2018)
Chen, H., Luo, P., Liu, G.: Global solution and blow up of a semilinear heat equation with logarithmic nonlinearity. J. Math. Anal. Appl. 422(1), 84–98 (2015)
Chen, Y., Levine, S., Rao, M.: Variable exponent, linear growth functionals in image restoration. SIAM J. Appl. Math. 66, 1383–1406 (2006)
Diening, L., Harjulehto, P., Hästö, P., Růžička, M.: Lebesgue and Sobolev Spaces with Variable Exponents. Lecture Notes in Mathematics 2017. Springer, Heidelberg (2011)
Ding, H., Zhou, J.: Global existence and blow-up for a mixed pseudoparabolic \(p\)-Laplacian type equation with logarithmic nonlinearity. J. Math. Anal. Appl. 2(478), 393–420 (2019)
Ding, H., Zhou, J.: Global existence and blow-up for a mixed pseudo-parabolic \(p\)-Laplacian type equation with logarithmic nonlinearity-II. Appl. Anal. 100, 2641–2658 (2019)
Edmunds, D.E., Rákosník, J.: Sobolev embeddings with variable exponent. Stud. Math. 143, 267–293 (2000)
Edmunds, D.E., Rákosník, J.: Sobolev embeddings with variable exponent II. Math. Nachr. 246(247), 53–67 (2002)
Fan, X., Shen, J., Zhao, D.: Sobolev embedding theorems for spaces \(W^{k, p(x)}\left(\Omega \right)\). J. Math. Anal. Appl. 262, 749–760 (2001)
Kováčik, O., Rákosník, J.: On spaces \(L^{p(x)}\) and \(W^{k, p(x)}\). Czechoslovak Math. J. 41, 592–618 (1991)
Li, F., Li, Z., Pi, L.: Variable exponent functionals in image restoration. Appl. Math. Comput. 216(3), 870–882 (2010)
Li, P.P., Liu, C.C.: A class of fourth-order parabolic equation with logarithmic nonlinearity. J. Inequal. Appl. 2018, 328 (2018)
Liu, B., Zhang, M., Li, F.: Singular properties of solutions for a parabolic equation with variable exponents and logarithmic source. Nonlinear Anal. Real World Appl. 64, 103449 (2022)
Nhan, L.C., Chuong, Q.V., Truong, L.X.: Potential well method for \(p(x)\)-Laplacian equations with variable exponent sources. Nonlinear Anal. Real World Appl. 56, 103155 (2020)
Nhan, L.C., Truong, L.X.: Existence and nonexistence of global solutions for doubly nonlinear diffusion equations with logarithmic nonlinearity. Electron. J. Qual. Theory Differ. Equ. 67, 1–25 (2018)
Nhan, L.C., Truong, L.X.: Global solution and blow-up for a class of \(p\)-Laplacian evolution equations with logarithmic nonlinearity. Acta Appl. Math. 151, 149–169 (2017)
Payne, L.E., Sattinger, D.H.: Saddle points and instability of nonlinear hyperbolic equations. Isr. J. Math. 22, 273–303 (1975)
Rajagopal, K., Růžička, M.: Mathematical modeling of electrorheological materials. Contin. Mech. Thermodyn. 13, 59–78 (2001)
Rajagopal, K., Růžička, M.: On the modeling of electrorheological materials. Mech. Res. Commun. 23, 401–407 (1996)
Růžička, M.: Electrorheological Fluids: Modeling and Mathematical Theory. Lecture Notes in Mathematics, vol. 1748. Springer, Berlin (2000)
Sattinger, D.H.: On global solution of nonlinear hyperbolic equations. Arch. Ration. Mech. Anal. 30(2), 148–172 (1968)
Wineman, A., Rajagopal, K.: On constitutive equations for electrorheological materials. Contin. Mech. Thermodyn. 7, 1–22 (1995)
Zeng, F., Deng, Q., Wang, D.: Global existence and blow-up for the pseudo-parabolic \(p(x)\)-Laplacian equation with logarithmic nonlinearity. J. Nonlinear Math. Phys. 29, 41–57 (2022)
Acknowledgements
The first author (Quach Van Chuong) is supported by Dong Nai University (DNU), Vietnam. The second author (Le Cong Nhan) is supported by Ho Chi Minh City University of Technology and Education (HCMUTE), Vietnam. The third author (Le Xuan Truong) is supported by University of Economics Ho Chi Minh City (UEH), Vietnam.
Author information
Authors and Affiliations
Contributions
All authors wrote the main manuscript text and reviewed the manuscript.
Corresponding author
Ethics declarations
Conflict of Interest
The authors declare no competing interests.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Van Chuong, Q., Nhan, L.C. & Truong, L.X. On Global Solution for a Class of p(x)-Laplacian Equations with Logarithmic Nonlinearity. Mediterr. J. Math. 21, 64 (2024). https://doi.org/10.1007/s00009-024-02604-5
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1007/s00009-024-02604-5