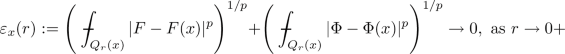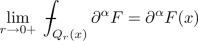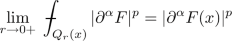Some Results About the Structure of Primitivity Domains for Linear Partial Differential Operators with Constant Coefficients

Abstract

Let G(D) be a linear partial differential operator on ${\mathbb {R}}^n$, with constant coefficients. Moreover let $\Omega \subset {\mathbb {R}}^n$ be open and $F\in L^1_{\text {loc}} (\Omega , {\mathbb {C}}^N)$. Then any set of the form

$$\begin{aligned} A_{f,F}:= \{ x\in \Omega \, \vert \, (G(D)f)(x)=F(x)\}, \text { with }f\in W^{g,1}_{\text {loc}}(\Omega , {\mathbb {C}}^k) \end{aligned}$$

is said to be a G-primitivity domain of F. We provide some results about the structure of G-primitivity domains of F at the points of the (suitably defined) G-nonintegrability set of F. A Lusin type theorem for G(D) is also provided. Finally, we give applications to the Maxwell type system and to the multivariate Cauchy-Riemann system.

1 Introduction

Let:

• $G=[G_{jl}]$ be a matrix of polynomials in ${\mathbb {C}}[\xi _1,\ldots , \xi _n]$ of dimension $N\times k$, with $\deg G:= \max _{j,l} (\deg G_{jl})=g\ge 1$;

• $(x_1,\ldots , x_n)$ be the standard coordinates of ${\mathbb {R}}^n$ and G(D) denote the system $[G_{jl}(D)]$, where $G_{jl}(D)$ is the linear partial differential operator with constant coefficients obtained by replacing each $\xi _q$ in $G_{jl}(\xi _1,\ldots ,\xi _n)$ with $-i \partial /\partial x_q$;

• $\Omega \subset {\mathbb {R}}^n$ be open and $F\in L^1_{\text {loc}} (\Omega , {\mathbb {C}}^N)$;

• m be a positive integer and $\Sigma _m$ denote the family of all matrices S of polynomials in ${\mathbb {C}}[\xi _1,\ldots , \xi _n]$, with N columns, satisfying $\deg S\le m$ and $SG=0$.

Then any set of the form

$$\begin{aligned} A_{f,F}:= \{ x\in \Omega \, \vert \, (G(D)f)(x)=F(x)\},\; \text { with} f\in W^{g,1}_{\text {loc}}(\Omega , {\mathbb {C}}^k) \end{aligned}$$

is said to be a G-primitivity domain of F and the following simple fact holds: If $F\in W^{m,1}_{\text {loc}}(\Omega , {\mathbb {C}}^N)$ and there is an open ball $B\subset \Omega$ such that almost all of B is covered by a G-primitivity domain $A_{f,F}$ (i.e., ${\mathcal L}^n (B{\!\setminus }\! A_{f,F})=0$), with $f\in W^{g+m,1}_{\text {loc}}(\Omega ,{\mathbb {C}}^k)$, then one has $S(D)F=0$ a.e. in B for all $S\in \Sigma _m$. This property, which can be readily extended to the case of $f\in W^{g,1}_{\text {loc}}(\Omega ,{\mathbb {C}}^k)$ (cf. Proposition 3.1), has naturally led us to expect that the structure of the G-primitivity domains of F may be somewhat singular at the points of

$$\begin{aligned} \Upsilon _{F}^{m}:=\bigcup _{S\in \Sigma _m} \Upsilon _{F,S}, \text { where } \Upsilon _{F,S}:=\{ x\in \Omega \,\vert \, (S(D)F)(x)\not =0\}, \end{aligned}$$

that (just for this reason) will be called the G-nonintegrability set of F. To confirm this intuition we first obtained the following results (cf. Corollary 3.2):

• (1) If $F\in W^{m, p} ({\mathbb {R}}^n, {\mathbb {C}}^N)$ and $f\in W^{g+m, p}({\mathbb {R}}^n, {\mathbb {C}}^k)$, with $p\in (1,+\infty )$, then one has ${\mathcal L}^n (A_{f,F}\cap \Upsilon _F^{m})=0$;

• (2) If $F\in W^{m+1, p} ({\mathbb {R}}^n, {\mathbb {C}}^N)$ and $f\in W^{g+m+1, p}({\mathbb {R}}^n, {\mathbb {C}}^k)$, with $p\in (1,n)$, then the set $A_{f,F}\cap \Upsilon _F^{m}$ is $(n-1)$-rectifiable (cf. [13, 17]), so that its Hausdorff dimension is less or equal to $n-1$.

Things can obviously improve if we consider a wider class of functions f. For example, if $F\in W_{\text {loc}}^{m,1}(\Omega , {\mathbb {C}}^N)$ then it may very well happen to come across $f\in W^{g, p}({\mathbb {R}}^n, {\mathbb {C}}^k)$ such that ${\mathcal L}^n (A_{f,F}\cap \Upsilon _F^{m})>0$ (cf. (4) below). However, even in this case, the structure of $A_{f,F}$ at points of $\Upsilon _F^{m}$ is significantly affected by the G-nonintegrability properties. In particular, the following fact holds (cf. Corollary 3.5):

• (3) Let $F\in W_{\text {loc}}^{m,p} (\Omega , {\mathbb {C}}^N)$ and $f\in W_{\text {loc}}^{g,p} (\Omega , {\mathbb {C}}^k)$, with $p\in (1,+\infty )$. Then, at a.e. point of $\Upsilon _{F}^m$, the set $A_{f,F}$ has density lower than $n+pm/(p-1)$.

In Sect. 4 we provide a Lusin type result which extends [2, Theorem 1] to a certain class of linear partial differential operators with constant coefficients (cf. Theorem 4.1). The assumptions that define this class are quite stringent. In particular, it is required that $k=1$ and that the components of G be different from each other. Moreover the following cohercivity condition is required: there exist a nonnegative integer $l\le g$ and a positive real number $c_*$ such that

$$\begin{aligned} \Vert G(D)\varphi \Vert _{\infty ,\Omega } \ge c_* \mathop {\max }\limits _{\begin{array}{c} \alpha \in {\mathbb {N}}^n\\ \vert \alpha \vert = l \end{array}}\Vert \partial ^{\alpha }\varphi \Vert _{\infty ,\Omega } \end{aligned}$$

for all $\varphi \in C_c^\infty (\Omega ,{\mathbb {C}})$. Despite these limitations, we believe that Theorem 4.1 may have some interesting applications. In support of this assertion, in Sect. 5 we actually provide two examples of application, respectively to the Maxwell type system and to the multivariate Cauchy-Riemann system. In a corollary to Theorem 4.1, we prove that (cf. Corollary 4.1):

• (4) Under the assumptions of Theorem 4.1 with $l=g$ and $F\in L_{\text {loc}}^1 (\Omega , {\mathbb {C}}^N)$, one has

  $$\begin{aligned} \sup _{f\in C_0^g(\Omega , {\mathbb {C}})}{\mathcal L}^n(A_{f,F}) ={\mathcal L}^n (\Omega ). \end{aligned}$$

Thus, under suitable conditions, there are G-primitivity domains of F arbitrarily close in measure to $\Omega$, even if $F\in W_{\text {loc}}^{m,1}(\Omega , {\mathbb {C}}^N)$ and ${\mathcal L}^n(\Upsilon _{F}^{m})>0$ (even if $\Upsilon _{F}^{m}=\Omega$, which is the least favorable case for the “G-integrability of F”!).

2 Notation and Preliminaries

2.1 General Notation

$B_r(x)$ is the open ball in ${\mathbb {R}}^n$ with center x and radius r. The open cube of side 2r centered at x in ${\mathbb {R}}^n$, that is $(-r,r)^n + x$, is denoted by $Q_r(x)$. For $z=(z_1,\ldots ,z_N)\in {\mathbb {C}}^N$, we set $\vert z\vert :=(\vert z_1\vert ^2+\cdots + \vert z_N\vert ^2)^{1/2}$. The Lebesgue outer measure and the s-dimensional Hausdorff outer measure in ${\mathbb {R}}^n$ will be denoted by ${\mathcal L}^n$ and ${\mathcal H}^s$, respectively. If $E\subset {\mathbb {R}}^n$ is a Lebesgue measurable set and $u_j,v_j: E\rightarrow {\mathbb {R}}$ ($j=1,\ldots ,N$) are Lebesgue measurable functions, we say that $(u_1+iv_1,\ldots , u_N+iv_N):E\rightarrow {\mathbb {C}}^N$ is Lebesgue measurable. If $f:E\rightarrow {\mathbb {C}}^N$ is a Lebesgue measurable function and $p\in [1,+\infty )$, then we define

$$\begin{aligned} \Vert f\Vert _{p,E}:=\bigg (\int _E\vert f\vert ^p\, d{\mathcal L}^n\bigg )^{\frac{1}{p}} \end{aligned}$$

while $\Vert f\Vert _{\infty , E}$ is defined as the infimum (which is actually a minimum) of the numbers $M\in [0,+\infty ]$ satisfying

$$\begin{aligned} {\mathcal L}^n(\{x\in E: \vert f(x)\vert > M\})=0. \end{aligned}$$

If $\Omega \subset {\mathbb {R}}^n$ is open and $u,v:\Omega \rightarrow {\mathbb {R}}$ are Lebesgue integrable (resp. p-summable, locally p-summable; $p\in [1,+\infty )$) on $\Omega$, then we say that $u+iv$ is Lebesgue integrable (resp. p-summable, locally p-summable) on $\Omega$ and define (omitting for simplicity to specify explicitly the measure, which is obviously the Lebesgue measure ${\mathcal L}^n$)

$$\begin{aligned} \int _\Omega (u+iv):=\int _\Omega u\, +\, i\int _\Omega v. \end{aligned}$$

The space of p-summable functions on $\Omega$ and the space locally p-summable functions on $\Omega$ will be denoted by $L^p (\Omega ,{\mathbb {C}})$ and $L^p_{\text {loc}}(\Omega ,{\mathbb {C}})$, respectively. If $f_1,\ldots ,f_k: \Omega \rightarrow {\mathbb {C}}$ are Lebesgue integrable (resp. p-summable, locally p-summable) on $\Omega$, then we say that $f=(f_1,\ldots ,f_k)^t$ is Lebesgue integrable (resp. p-summable, locally p-summable) on $\Omega$ and define

$$\begin{aligned} \int _\Omega f:=\left( \int _\Omega f_1,\ldots , \int _\Omega f_k \right) ^t. \end{aligned}$$

We also set

$$\begin{aligned}&L^p(\Omega ,{\mathbb {C}}^k):=\{ (f_1,\ldots ,f_k)^t\,\vert \, f_j\in L^p(\Omega ,{\mathbb {C}}) \text { for }1\le j\le k\}, \\&L^p_{\text {loc}}(\Omega ,{\mathbb {C}}^k):=\{ (f_1,\ldots ,f_k)^t\,\vert \, f_j\in L^p_{\text {loc}}(\Omega ,{\mathbb {C}}) \text { for }1\le j\le k\}. \end{aligned}$$

The coordinates of ${\mathbb {R}}^n$ are denoted by $(x_1,\ldots , x_n)$ and we set for simplicity $\partial _j:= \partial /\partial x_j$. For $\alpha = (\alpha _1,\ldots ,\alpha _n)\in {\mathbb {N}}^n$, define

$$\begin{aligned} \vert \alpha \vert := \alpha _1+\ldots +\alpha _n,\quad \alpha !:= \alpha _1 !\cdots \alpha _n !, \quad \partial ^\alpha :=\frac{\partial ^{\vert \alpha \vert }}{\partial x_1^{\alpha _1}\cdots \partial x_n^{\alpha _n}}. \end{aligned}$$

Similarly, if $(\xi _1,\ldots ,\xi _n)\in {\mathbb {R}}^n$ then we write

$$\begin{aligned} \xi ^\alpha :=\xi _1^{\alpha _1}\cdots \xi _n^{\alpha _n}. \end{aligned}$$

If $\Omega \subset {\mathbb {R}}^n$ is open, $m\in {\mathbb {N}}$ and $k\in {\mathbb {N}}{\! \setminus }\! \{ 0\}$, then we set

$$\begin{aligned} C^m(\Omega , {\mathbb {C}}):= \{ u+ iv\, \vert \, u,v\in C^m(\Omega )\},\quad C_c^m(\Omega , {\mathbb {C}}):= \{ u+ iv\,\vert \, u,v\in C_c^m(\Omega )\} \end{aligned}$$

and

$$\begin{aligned}&C^m(\Omega , {\mathbb {C}}^k):=\{(f_1,\ldots ,f_k)^t\,\vert \, f_1,\ldots ,f_k\in C^m(\Omega , {\mathbb {C}})\}, \\&C_c^m(\Omega , {\mathbb {C}}^k):=\{(f_1,\ldots ,f_k)^t\,\vert \, f_1,\ldots ,f_k\in C_c^m(\Omega , {\mathbb {C}})\}. \end{aligned}$$

For $\alpha \in {\mathbb {N}}^n$ and $f=(f_1,\ldots ,f_k)^t\in C^{\vert \alpha \vert }(\Omega , {\mathbb {C}}^k)$, we set

$$\begin{aligned} \partial ^\alpha f:= (\partial ^\alpha f_1,\ldots , \partial ^\alpha f_k)^t. \end{aligned}$$

The norm in $C^m(\Omega , {\mathbb {C}}^k)$ is defined as

$$\begin{aligned} C^m(\Omega , {\mathbb {C}}^k)\ni f \mapsto \Vert f\Vert _{C^m(\Omega , {\mathbb {C}}^k)}:=\mathop \sum \limits _{\begin{array}{c} \alpha \in {\mathbb {N}}^n\\ \vert \alpha \vert \le m \end{array}}\Vert \partial ^\alpha f\Vert _{\infty ,\Omega }. \end{aligned}$$

The closure of $C_c^\infty (\Omega , {\mathbb {C}}^k)$ in $(C^m(\Omega , {\mathbb {C}}^k); \Vert \cdot \Vert _{C^m(\Omega , {\mathbb {C}}^k)})$ will be denoted by $C_0^m(\Omega , {\mathbb {C}}^k)$. For simplicity, we will write $C(\Omega , {\mathbb {C}}^k)$, $C_c(\Omega , {\mathbb {C}}^k)$ and $C_0(\Omega , {\mathbb {C}}^k)$ in place of $C^0(\Omega , {\mathbb {C}}^k)$, $C_c^0(\Omega , {\mathbb {C}}^k)$ and $C_0^0(\Omega , {\mathbb {C}}^k)$, respectively. If m is a positive integer and $p\in [1,+\infty )$, then we set

$$\begin{aligned} W^{m,p}_{\text {loc}}(\Omega , {\mathbb {C}}):= \{ u+ iv\,\vert \, u,v\in W^{m,p}_{\text {loc}} (\Omega )\} \end{aligned}$$

and

$$\begin{aligned} W^{m,p}_{\text {loc}}(\Omega , {\mathbb {C}}^k):=\{(f_1,\ldots ,f_k)^t\,\vert \, f_1,\ldots ,f_k\in W^{m,p}_{\text {loc}}(\Omega , {\mathbb {C}})\}. \end{aligned}$$

If $f\in W^{m,1}_{\text {loc}}(\Omega , {\mathbb {C}})$ and $\alpha \in {\mathbb {N}}^n$ with $\vert \alpha \vert \le m$, then $\partial ^\alpha f$ will denote the precise representative of the $\alpha ^{\text {th}}$ weak derivative of f (cf. [12, 18]). In particular, $\partial ^0 f$ is the precise representative of f. If $f=(f_1,\ldots ,f_k)^t\in W^{m,1}_{\text {loc}}(\Omega , {\mathbb {C}}^k)$ and $\alpha \in {\mathbb {N}}^n$ with $\vert \alpha \vert \le m$, then $\partial ^\alpha f:= (\partial ^\alpha f_1,\ldots , \partial ^\alpha f_k)^t$. If $d\ge 0$, $p>1$ and $E\subset {\mathbb {R}}^n$, then $B_{d,p}(E)$ denotes the Bessel capacity of E (cf. Section 2.6 in [21]). Recall that $B_{0,p}={\mathcal L}^n$.

2.2 Linear Partial Differential Operators

Let

$$\begin{aligned} P(\xi _1,\ldots ,\xi _n)=\mathop \sum \limits _{\begin{array}{c} \alpha \in {\mathbb {N}}^n\\ \vert \alpha \vert \le d \end{array}} c_\alpha \, \xi ^\alpha \in {\mathbb {C}}[\xi _1,\ldots , \xi _n]. \end{aligned}$$

If $c_\alpha \not =0$ for some $\alpha \in {\mathbb {N}}^n$ with $\vert \alpha \vert =d$, then the number d is said to be the total degree of P and is denoted by $\deg P$. As usual (cf. [5, 15]), P(D) is the differential operator obtained by replacing each variable $\xi _j$ with $-i\partial _j$, namely

$$\begin{aligned} P(D):= \mathop \sum \limits _{\begin{array}{c} \alpha \in {\mathbb {N}}^n\\ \vert \alpha \vert \le d \end{array}} (-i)^{\vert \alpha \vert }c_\alpha \, \partial ^\alpha . \end{aligned}$$

(2.1)

Also define

$$\begin{aligned} P^*(\xi ):= P(-\xi )= \mathop \sum \limits _{\begin{array}{c} \alpha \in {\mathbb {N}}^n\\ \vert \alpha \vert \le d \end{array}} ( -1)^{\vert \alpha \vert }c_\alpha \, \xi ^\alpha \in {\mathbb {C}}[\xi _1,\ldots , \xi _n]. \end{aligned}$$

Observe that if $P,Q\in {\mathbb {C}}[\xi _1,\ldots , \xi _n]$ then these identities holds:

$$\begin{aligned} (P+Q)^*=P^* + Q^*,\quad (PQ)^* =P^*Q^* \end{aligned}$$

(2.2)

and

$$\begin{aligned} (PQ)(D)=P(D)Q(D). \end{aligned}$$

(2.3)

Now consider $P\in {\mathbb {C}}[\xi _1,\ldots , \xi _n]$, with $d:=\deg P\ge 1$, an open set $\Omega \subset {\mathbb {R}}^n$ and

$$\begin{aligned} \varphi \in C^d_c(\Omega ,{\mathbb {C}}),\, \psi \in W_{\text {loc}}^{d, 1} (\Omega , {\mathbb {C}}). \end{aligned}$$

Then $(P(D)\psi ) \varphi$ and $(P^*(D)\varphi ) \psi$ are obviously Lebesgue summable on $\Omega$ and a trivial computation shows that

$$\begin{aligned} \int _\Omega (P(D)\psi ) \varphi = \int _\Omega (P^*(D)\varphi ) \psi . \end{aligned}$$

(2.4)

If $M=[P_{jl}]$ is a matrix of polynomials in ${\mathbb {C}}[\xi _1,\ldots , \xi _n]$ of dimension $N\times k$, then we set

$$\begin{aligned} \deg M:=\max _{(j,l)}\deg P_{jl},\quad M(D):= [P_{jl}(D)]. \end{aligned}$$

For all $(j,l)\in \{1,\ldots , N\}\times \{1,\ldots , k\}$ the polynomial $P_{jl}$ can be written as follows

$$\begin{aligned} P_{jl}(\xi _1,\ldots ,\xi _n)=\mathop \sum \limits _{\begin{array}{c} \alpha \in {\mathbb {N}}^n\\ \vert \alpha \vert \le m \end{array}} c_\alpha ^{(jl)}\, \xi ^\alpha , \end{aligned}$$

where $c_\alpha ^{(jl)}\in {\mathbb {C}}$ and $m:=\deg M$. If $f\in W^{m,p}_{\text {loc}} (\Omega , {\mathbb {C}}^k)$, with $m=\deg M$ and $\Omega \subset {\mathbb {R}}^n$ open, then one has

$$\begin{aligned} M(D)f = \mathop \sum \limits _{\begin{array}{c} \alpha \in {\mathbb {N}}^n\\ \vert \alpha \vert \le m \end{array}} (-i)^{\vert \alpha \vert } C_\alpha \partial ^\alpha f \end{aligned}$$

where $C_\alpha$ is the matrix of dimension $N\times k$ whose entries are the numbers $c_\alpha ^{(jl)}$, with $(j,l)\in \{1,\ldots , N\}\times \{1,\ldots , k\}$.

2.3 Distributions

Let $\Omega$ be an open subset of ${\mathbb {R}}^n$. We recall that a linear functional $T:C_c^\infty (\Omega ,{\mathbb {C}})\rightarrow {\mathbb {C}}$ is said to be a distribution on $\Omega$ if one has $\lim _{j\rightarrow \infty }T(\varphi _j)= T(\varphi )$ for every sequence $\{\varphi _j\}_{j=1}^\infty \subset C_c^\infty (\Omega ,{\mathbb {C}})$ and $\varphi \in C_c^\infty (\Omega ,{\mathbb {C}})$ such that

• (i) There exists a compact set $K\subset \Omega$ such that $\text {supp}\, \varphi _j\subset K$, for all j;

• (ii) One has $\lim _{j\rightarrow \infty }\Vert \partial ^{\alpha }\varphi _j - \partial ^{\alpha }\varphi \Vert _{\infty ,\Omega }=0$, for all $\alpha \in {\mathbb {N}}^n$.

If conditions (i) and (ii) are satisfied we say that the sequence $\{\varphi _j\}_{j=1}^\infty$ converges to $\varphi$ in $C_c^\infty (\Omega ,{\mathbb {C}})$. The set of all distributions on $\Omega$, denoted by ${\mathcal D}'(\Omega )$, is obviously a vector space with addition and scalar multiplication defined by

$$\begin{aligned} (T_1+T_2)(\varphi ) = T_1(\varphi ) + T_2(\varphi ),\quad (\lambda T) (\varphi )=\lambda T(\varphi ) \end{aligned}$$

for all $T_1, T_2, T\in {\mathcal D}'(\Omega )$, $\lambda \in {\mathbb {C}}$ and $\varphi \in C_c^\infty (\Omega ,{\mathbb {C}})$. For every $u\in L^1_{\text {loc}}(\Omega ,{\mathbb {C}})$ one can define $T_u\in {\mathcal D}'(\Omega )$ as

$$\begin{aligned} T_u(\varphi ):=\int _\Omega u\varphi ,\quad \varphi \in C_c^\infty (\Omega ,{\mathbb {C}}). \end{aligned}$$

We recall that, if $P\in {\mathbb {C}}[\xi _1,\ldots , \xi _n]$, $T\in {\mathcal D}'(\Omega )$ and set

$$\begin{aligned} {[}P(D)T](\varphi ):= T(P^*(D)\varphi ), \quad \varphi \in C_c^\infty (\Omega ,{\mathbb {C}}) \end{aligned}$$

then $P(D)T\in {\mathcal D}'(\Omega )$. In particular, if $u\in L^1_{\text {loc}}(\Omega ,{\mathbb {C}})$ then one has

$$\begin{aligned} {[}P(D)T_u](\varphi )=\int _\Omega (P^*(D)\varphi ) u,\quad \varphi \in C_c^\infty (\Omega ,{\mathbb {C}}). \end{aligned}$$

Hence, in the special case when $u\in C^m(\Omega ,{\mathbb {C}})$ with $m = \deg P$, recalling (2.4), we find the following regularity identity

$$\begin{aligned} P(D)T_u =T_{P(D)u}. \end{aligned}$$

(2.5)

We shall use the weak topology in ${\mathcal D}'(\Omega )$, according to which

$$\begin{aligned} \lim _{j\rightarrow \infty }T_j = T\qquad ( T, T_j\in {\mathcal D}'(\Omega )) \end{aligned}$$

means that

$$\begin{aligned} \lim _{j\rightarrow \infty }T_j(\varphi ) = T(\varphi ), \text { for all} \varphi \in C_c^\infty (\Omega ,{\mathbb {C}}). \end{aligned}$$

The map

$$\begin{aligned} L^1_{\text {loc}}(\Omega , {\mathbb {C}})\ni u\mapsto T_u\in {\mathcal D}'(\Omega ) \end{aligned}$$

is continuous. More precisely, if $\{u_j\}_{j=1}^\infty$ converges to u in $L^1_{\text {loc}}(\Omega , {\mathbb {C}})$, namely $u_j, u\in L^1_{\text {loc}}(\Omega , {\mathbb {C}})$ and

$$\begin{aligned} \lim _{j\rightarrow \infty }\int _K \vert u_j - u\vert =0 \end{aligned}$$

for all compact set $K\subset \Omega$, then one has

$$\begin{aligned} \lim _{j\rightarrow \infty }T_{u_j} = T_u. \end{aligned}$$

(2.6)

Let $G=[G_{lh}]$ be a matrix of polynomials in ${\mathbb {C}}[\xi _1,\ldots , \xi _n]$ of dimension $N\times k$ and let

$$\begin{aligned} f =(f_1,\ldots , f_k)^t \in L^1_{\text {loc}} (\Omega , {\mathbb {C}}^k), \quad \Phi =(\Phi _1,\ldots ,\Phi _N)^t \in L^1_{\text {loc}} (\Omega , {\mathbb {C}}^N) \end{aligned}$$

be such that equality $G(D)f=\Phi$ holds in the sense of distributions, that is

$$\begin{aligned} \sum _{q=1}^k G_{lq}(D) T_{f_q} = T_{\Phi _l} \end{aligned}$$

for all $l=1,\ldots , N$, i.e.,

$$\begin{aligned} \sum _{q=1}^k\int _\Omega (G_{lq}^*(D)\varphi )f_q = \int _{\Omega } \varphi \Phi _l \end{aligned}$$

(2.7)

for all $\varphi \in C_c^\infty (\Omega ,{\mathbb {C}})$ and $l=1,\ldots ,N$. Observe that if $S=[S_{jl}]$ is another matrix of polynomials in ${\mathbb {C}}[\xi _1,\ldots , \xi _n]$ of dimension $h\times N$, then (2.7), (2.3) and (2.2) yield

$$\begin{aligned} \begin{aligned} \sum _{l=1}^N \int _{\Omega } \left( S_{jl}^*(D)\varphi \right) \Phi _l&= \sum _{l=1}^N\sum _{q=1}^k\int _\Omega \left( G_{lq}^*(D) S_{jl}^*(D)\varphi \right) f_q\\&= \sum _{q=1}^k\int _\Omega \bigg (\bigg (\sum _{l=1}^N S_{jl} G_{lq}\bigg )^* (D)\, \varphi \bigg ) f_q \end{aligned} \end{aligned}$$

namely

$$\begin{aligned} \sum _{l=1}^N \int _{\Omega } \left( S_{jl}^*(D)\varphi \right) \Phi _l = \sum _{q=1}^k\int _\Omega \left( (SG)_{jq}^* (D)\, \varphi \right) f_q \end{aligned}$$

(2.8)

for all $\varphi \in C_c^\infty (\Omega ,{\mathbb {C}})$ and for all $j=1,\ldots , h$. In the special case when $f\in W_{\text {loc}}^{g+s,1}(\Omega ,{\mathbb {C}}^k)$, with $g:=\deg G$ and $s:=\deg S$, identity (2.8) provides

$$\begin{aligned} \int _\Omega \left( \sum _{l=1}^N S_{jl}(D)\left[ \sum _{q=1}^k G_{lq}(D) f_q\right] \right) \varphi = \int _\Omega \left( \sum _{q=1}^k (SG)_{jq}(D) f_q \right) \varphi \end{aligned}$$

for all $\varphi \in C_c^\infty (\Omega ,{\mathbb {C}})$ and for all $j=1,\ldots , h$, that is

$$\begin{aligned} S(D)\left[ G(D)f \right] =(SG)(D) f \text { a.e. in }\Omega . \end{aligned}$$

(2.9)

2.4 Superdensity

A point $x\in {\mathbb {R}}^n$ is said to be a m-density point of $E\subset {\mathbb {R}}^n$ (where $m\in [n,+\infty )$) if

$$\begin{aligned} {\mathcal L}^n (B_r(x)\!\setminus \! E)=o(r^{m})\qquad (\text {as }r\rightarrow 0+). \end{aligned}$$

The set of all m-density points of E is denoted by $E^{(m)}$.

Remark 2.1

The following properties hold:

• Every interior point of $E\subset {\mathbb {R}}^n$ is an m-density point of E, for all $m\in [n,+\infty )$. Thus, whenever E is open, one has $E\subset E^{(m)}$ for all $m\in [n,+\infty )$;

• If $E\subset {\mathbb {R}}^n$ and $n\le m_1\le m_2<+\infty$, then $E^{(m_2)}\subset E^{(m_1)}$. In particular, one has $E^{(m)}\subset E^{(n)}$ for all $m\in [n,+\infty )$;

• If $A, B\subset {\mathbb {R}}^n$ then $(A\cap B)^{(m)}=A^{(m)}\cap B^{(m)}$, for all $m\in [n,+\infty )$;

• For all $A\subset {\mathbb {R}}^n$ and $m\in [n,+\infty )$, the set $A^{(m)}$ is ${\mathcal L}^n$-measurable (cf. [7, Proposition 3.1]).

Remark 2.2

Let $E\subset {\mathbb {R}}^n$. Then one has the following inequality

$$\begin{aligned} \frac{{\mathcal L}^n(B_r(x)\cap E)}{{\mathcal L}^n(B_r(x))} \ge 1 - \frac{{\mathcal L}^n(B_r(x)\! \setminus \! E)}{{\mathcal L}^n(B_r(x))}\qquad (r>0) \end{aligned}$$

where equality holds if E is ${\mathcal L}^n$-measurable. Thus:

• If $x\in E^{(n)}$, then x is a Lebesgue density point of E;

• If E is ${\mathcal L}^n$-measurable and x is a Lebesgue density point of E, then $x\in E^{(n)}$.

In particular, if E is ${\mathcal L}^n$-measurable then: $x\in E^{(n)}$ if and only if x is a Lebesgue density point of E, hence

$$\begin{aligned} {\mathcal L}^n(E\Delta E^{(n)})=0 \end{aligned}$$

(2.10)

e.g., cf. Corollary 1.5 in [20, Chapter 3]. It follows that ${\mathcal L}^n(B_r(x)\! \setminus \! E^{(n)})={\mathcal L}^n(B_r(x)\! \setminus \! E)$, for all $r>0$, hence

$$\begin{aligned} (E^{(n)})^{(n)}=E^{(n)}. \end{aligned}$$

A remarkable family of superdense sets is the class of finite perimeter sets. Indeed Theorem 1 in [12, Section 6.1.1] states that almost every point in a set $E\subset {\mathbb {R}}^n$ (with $n\ge 2$) of finite perimeter is a $m_0$-density point of E, with

$$\begin{aligned} m_0:=n+1+\frac{1}{n-1}. \end{aligned}$$

The number $m_0$ is also the maximum order of density common to all sets of finite perimeter. More precisely one has this result, cf. [6, Lemma 4.1] and [7, Proposition 4.1].

Proposition 2.1

The following facts hold ($n\ge 2$):

• (1) If E is a set of locally finite perimeter in ${\mathbb {R}}^n$, then ${\mathcal L}^n$-almost every point in E belongs to $E^{(m_0)}$;

• (2) For all $m>m_0$ there exists a compact set $F_m$ of finite perimeter in ${\mathbb {R}}^n$ such that ${\mathcal L}^n(F_m)>0$ and $F_m^{(m)}=\emptyset$.

2.5 A class of cut-off functions

Consider $r >0$, $\rho \in (0,1)$ and a function $\psi \in C^\infty ({\mathbb {R}})$ such that

$$\begin{aligned} 0\le \psi \le 1,\quad \psi \vert _{(-\infty , 0]}\equiv 1, \quad \psi \vert _{[1,+\infty )}\equiv 0. \end{aligned}$$

If define $\varphi _{\rho ,r}:{\mathbb {R}}^n\rightarrow {\mathbb {R}}$ by

$$\begin{aligned} \varphi _{\rho ,r}(x):=\prod _{j=1}^n \psi \left( \frac{\vert x_j\vert - \rho r}{(1-\rho ) r} \right) ,\text { for all } x=(x_1,\ldots ,x_n)\in {\mathbb {R}}^n, \end{aligned}$$

then one obviously has

$$\begin{aligned} \varphi _{\rho , r}\in C^\infty ({\mathbb {R}}^n, [0,1]),\quad \varphi _{\rho , r}\vert _{Q_{\rho r}(0)}\equiv 1,\quad \varphi _{\rho , r}\vert _{{\mathbb {R}}^n \!{\setminus }\! Q_{r}(0)}\equiv 0. \end{aligned}$$

(2.11)

Moreover, a standard computation yields

$$\begin{aligned} \left| \partial ^\alpha \varphi _{\rho ,r} (x) \right| =\frac{1}{(1-\rho )^{\vert \alpha \vert }r^{\vert \alpha \vert }}\prod _{j=1}^n \left| \psi ^{(\alpha _j)}\left( \frac{\vert x_j\vert - \rho r}{(1-\rho )r} \right) \right| , \end{aligned}$$

for all $\alpha \in {\mathbb {N}}^n$ and $x=(x_1,\ldots ,x_n)\in {\mathbb {R}}^n$, hence

$$\begin{aligned} \Vert \partial ^\alpha \varphi _{\rho ,r} \Vert _{\infty ,{\mathbb {R}}^n} \le \frac{C(\alpha )}{(1-\rho )^{\vert \alpha \vert }r^{\vert \alpha \vert }} \end{aligned}$$

(2.12)

for all $\alpha \in {\mathbb {N}}^n$, where $C(\alpha )$ is a number depending only on $\alpha$ (and n).

3 Some structure results for G-primitivity domains

Throughout this section, $\Omega$ is an open subset of ${\mathbb {R}}^n$ while $G=[G_{jl}]$ is a matrix of polynomials in ${\mathbb {C}}[\xi _1,\ldots , \xi _n]$ of dimension $N\times k$. Let us assume

$$\begin{aligned} g:= \deg G = \max _{(jl)} \deg G_{jl}\ge 1. \end{aligned}$$

Moreover, for any couple of integers $m, h\ge 1$, let ${\mathcal M}_{m,h}$ denote the family of all matrices S of polynomials in ${\mathbb {C}}[\xi _1,\ldots , \xi _n]$ of dimension $h\times N$ such that $\deg S\le m$. We also define

$$\begin{aligned} \Sigma _{m,h}:=\{ S\in {\mathcal M}_{m,h}\,\vert \, SG=0\},\quad \Sigma _m:=\bigcup _{h=1}^{+\infty } \Sigma _{m,h}. \end{aligned}$$

Definition 3.1

Let $F\in L_{\text {loc}}^1 (\Omega , {\mathbb {C}}^N)$. Then any set of the form

$$\begin{aligned} A_{f,F}:=\{ x\in \Omega \, \vert \, (G(D)f)(x)=\partial ^0 F(x)\}, \text { with }f\in W_{\text {loc}}^{g,1} (\Omega , {\mathbb {C}}^k), \end{aligned}$$

is called “ G-primitivity domain of F”. For $F \in W^{m,1}_{\text {loc}} (\Omega , {\mathbb {C}}^N)$, with $m\ge 1$, we define

$$\begin{aligned} \Upsilon _{F}^{m}:=\bigcup _{S\in \Sigma _m} \Upsilon _{F,S}, \text { where } \Upsilon _{F,S}:=\{ x\in \Omega \,\vert \, (S(D)F)(x)\not =0\}. \end{aligned}$$

We shall refer to $\Upsilon _{F}^{m}$ as the “ G-nonintegrability set of F”.

Remark 3.1

If $F\in C(\Omega ,{\mathbb {C}}^N)$ and $f\in C^g(\Omega , {\mathbb {C}}^k)$ then one has

$$\begin{aligned} A_{f,F}\cap \Upsilon _F^0=\emptyset . \end{aligned}$$

(3.1)

Indeed, let $S\in \Sigma _0$ and observe that it must coincide with a matrix M whose entries are all in ${\mathbb {C}}$. Then, for all $x\in A_{f,F}$, one has

$$\begin{aligned} (S(D)F)(x) =M(F(x)) = M((G(D)f)(x))=((SG)(D)f)(x)=0 \end{aligned}$$

that is $A_{f,F}\cap \Upsilon _{F,S}=\emptyset$. Now (3.1) follows from the arbitrariness of $S\in \Sigma _0$.

Remark 3.2

The family ${\mathcal M}_{m,h}$ is a finite dimensional vector space on the field ${\mathbb {C}}$. Let us consider $S=[S_{jl}]\in {\mathcal M}_{m,h}$ with

$$\begin{aligned} S_{jl}(\xi _1,\ldots ,\xi _n)=\mathop \sum \limits _{\begin{array}{c} \alpha \in {\mathbb {N}}^n\\ \vert \alpha \vert \le m \end{array}} c_\alpha ^{(jl)}\xi ^\alpha \end{aligned}$$

and define

$$\begin{aligned} \vert \,\vert S\vert \,\vert :=\sum _{j, l, \alpha } \vert c_\alpha ^{(jl)}\vert . \end{aligned}$$

Then $S\mapsto \vert \,\vert S\vert \,\vert$ is a norm in ${\mathcal M}_{m,h}$. Obviously $\Sigma _{m,h}$ is a closed vector subspace of $({\mathcal M}_{m,h},\vert \,\vert \cdot \vert \,\vert )$, normed by the restriction of $\vert \,\vert \cdot \vert \,\vert$ to $\Sigma _{m,h}$. In particular it is separable, i.e., it has a countable subset $\Sigma _{m,h}^*$ which is dense with respect to the norm topology. Observe that for all $F \in W^{m,1}_{\text {loc}} (\Omega , {\mathbb {C}}^N)$ one has

$$\begin{aligned} \bigcup _{S\in \Sigma _{m,h}} \Upsilon _{F,S}=\bigcup _{S\in \Sigma _{m,h}^*} \Upsilon _{F,S}\qquad (h=1,2,\ldots ) \end{aligned}$$

hence

$$\begin{aligned} \Upsilon _F^m= \bigcup _{h=1}^{+\infty }\bigcup _{S\in \Sigma _{m,h}} \Upsilon _{F,S}= \bigcup _{h=1}^{+\infty }\bigcup _{S\in \Sigma _{m,h}^*} \Upsilon _{F,S} \end{aligned}$$

that is

$$\begin{aligned} \Upsilon _{F}^{m}=\bigcup _{S\in \Sigma _m^*} \Upsilon _{F,S},\text { with } \Sigma _m^*:= \bigcup _{h=1}^{+\infty } \Sigma _{m,h}^*. \end{aligned}$$

(3.2)

From (2.9) and (3.2) we get at once the following property, that is the original reason why $\Upsilon _{F}^{m}$ is called G-nonintegrability set of F: Let $F \in W^{m,1}_{\text {loc}} (\Omega , {\mathbb {C}}^N)$ and assume that there is an open ball $B\subset \Omega$ and $f\in W^{g+m,1}_{\text {loc}}(\Omega ,{\mathbb {C}}^k)$ such that $G(D)f=F$ a.e. in B. Then ${\mathcal L}^n(B\cap \Upsilon _{F}^{m})=0$. The next result extends such a property and will be further generalized in Corollary 3.2 and Corollary 3.5 below (cf. Remark 3.5).

Proposition 3.1

Let $F=(F_1,\ldots , F_N)^t \in W^{m,1}_{\text {loc}} (\Omega , {\mathbb {C}}^N)$, with $m\ge 1$. Assume that there is an open ball $B\subset \Omega$ such that almost all of B is covered by a G-primitivity domain $A_{f,F}$ with $f=(f_1,\ldots ,f_k)^t\in W^{g,1}_{\text {loc}}(\Omega ,{\mathbb {C}}^k)$, i.e. ${\mathcal L}^n (B \! \setminus \! A_{f,F})=0$. Then ${\mathcal L}^n(B\cap \Upsilon _{F}^{m})=0$.

Proof

Let S be chosen arbitrarily in $\Sigma _m^*$, whereby there is $h\ge 1$ such that $S\in \Sigma _{m,h}^*$. From (2.4) and (2.8) (with $\Phi =F$), we get

$$\begin{aligned} \begin{aligned} \int _B (S(D)F)_j\varphi&=\sum _{l=1}^N \int _B (S_{jl}(D)F_l) \varphi = \sum _{l=1}^N \int _{B} \left( S_{jl}^*(D)\varphi \right) F_l\\&= \sum _{q=1}^k\int _B \left( (SG)_{jq}^* (D)\, \varphi \right) f_q =0 \end{aligned} \end{aligned}$$

for all $\varphi \in C^\infty _c(B)$ and $j=1,\ldots , h$. Hence $S(D)F=0$ a.e. in B, that is ${\mathcal L}^n(B\cap \Upsilon _{F,S})=0$. The conclusion follows from the arbitrariness of $S\in \Sigma _m^*$ and (3.2). $\square$

Remark 3.3

Let $F \in W^{m,1}_{\text {loc}} (\Omega , {\mathbb {C}}^N)$ and $f \in W^{m+g,1}_{\text {loc}}(\Omega ,{\mathbb {C}}^k)$ be such that $G(D)f=F$ a.e. in $\Omega$, i.e., ${\mathcal L}^n(\Omega \!\setminus \! A_{f,F})=0$. In this special case, Proposition 3.1 leads to ${\mathcal L}^n(\Upsilon _F^m)=0$, i.e., the obvious compatibility condition $S(D)F=0$ a.e. in $\Omega$, for all $S\in \Sigma _m$.

Remark 3.4

In general, the problem of determining S such that $SG = 0$ is not easy and for an account about its resolution we refer the reader to algebraic analysis literature, e.g., [5] (and the references therein), where it is addressed also through the use of specific software. In this regard it must be remembered that a particularly significant case is when S is the matrix yielded by the first syzygies of G, also considered in Corollary 3.3, Corollary 3.7, Example 3.1 and in [10, Sect. 5.2] (Maxwell type system), [10, Sect.5.3] (multivariable Cauchy-Riemann system). In this case, the identity $(S(D)F)(x)=0$ for each $x\in \Omega$, under the further assumption that $\Omega$ is convex, is a necessary and sufficient condition for the existence of a solution f to the partial differential equation $G(D)f=F$ in the frameworks corresponding to a large class of sheaves of functions, cf. [5, Theorem 2.1.1].

3.1 Structure of $A_{f,F}$ at Points of the G-nonintegrability Set of $F\in W^{m+d, p}$: The Case of $f\in W^{g+m+d, p}$

In paper [10] we have proved the following result.

Theorem 3.1

Let $F\in C^m (\Omega , {\mathbb {C}}^N)$ and $f\in C^{g+m}(\Omega , {\mathbb {C}}^k)$. Define $A_{f,F}^*$ as the set of all $x\in A_{f,F}$ satisfying the following property: There exists S, possibly depending on x, such that $1\le \deg S \le m$, $SG=0$ and $(S(D)F)(x)\not =0$. Then the set $A_{f,F}^*$ is covered by a finite family of $(n-1)$-dimensional regularly imbedded $C^1$ submanifolds of ${\mathbb {R}}^n$.

Corollary 3.1

Let $F\in C^m (\Omega , {\mathbb {C}}^N)$ and $f\in C^{g+m}(\Omega , {\mathbb {C}}^k)$. Then the set $A_{f,F}\cap \Upsilon _F^m$ is covered by a finite family of $(n-1)$-dimensional regularly imbedded $C^1$ submanifolds of ${\mathbb {R}}^n$.

Proof

Since $\{S\in \Sigma _m\,\vert \, \deg S=0\} = \Sigma _0$, one has

$$\begin{aligned} \Upsilon _F^0 =\bigcup _{S\in \Sigma _0}\Upsilon _{F,S} =\mathop \bigcup \limits _{\begin{array}{c} S\in \Sigma _m\\ \deg S=0 \end{array}}\Upsilon _{F,S}. \end{aligned}$$

Then, also recalling (3.1), we obtain

$$\begin{aligned} A_{f,F}^*=A_{f,F}\cap \left( \mathop \bigcup \limits _{\begin{array}{c} S\in \Sigma _m\\ \deg S\ge 1 \end{array}} \Upsilon _{F,S} \right) = A_{f,F}\cap (\Upsilon _F^m \!{\setminus } \! \Upsilon _F^0) = A_{f,F}\cap \Upsilon _F^m \end{aligned}$$

and the conclusion follows from Theorem 3.1. $\square$

From Corollary 3.1 we get, quite easily, this result in the context of Sobolev functions.

Corollary 3.2

Let $F\in W^{m+d, p} ({\mathbb {R}}^n, {\mathbb {C}}^N)$ and $f\in W^{g+m+d, p}({\mathbb {R}}^n, {\mathbb {C}}^k)$, with $m\ge 1$, $p\in (1,+\infty )$ and $d\in \{0, 1\}$. The following facts hold:

• (1) If $d=0$ then ${\mathcal L}^n (A_{f,F}\cap \Upsilon _F^{m})=0$;

• (2) If $d=1$ and $p<n$, then $A_{f,F}\cap \Upsilon _F^{m}$ is $(n-1)$-rectifiable (cf. [13, 17]), so that its Hausdorff dimension is less or equal to $n-1$.

Proof

Let $d\in \{ 0,1\}$ and $p\in (1,+\infty )$ be such that $pd<n$. Then, recalling a well known Lusin-type approximation result for Sobolev functions (cf. Theorem 3.10.5 in [21]), we can find

$$\begin{aligned} F^{l}\in C^m({\mathbb {R}}^n, {\mathbb {C}}^N),\ f^{l}\in C^{g+m}({\mathbb {R}}^n, {\mathbb {C}}^k)\qquad (l=1,2,\ldots ) \end{aligned}$$

such that

$$\begin{aligned} B_{d,p}({\mathbb {R}}^n \!{\setminus }\! E^{l}) \le \frac{1}{l}, \end{aligned}$$

(3.3)

where

$$\begin{aligned} E^{l}:= E^{l}_1\cap E^{l}_2 \end{aligned}$$

with

$$\begin{aligned} E^{l}_1:=\bigcap _{\vert \alpha \vert \le m}\{ x\in {\mathbb {R}}^n: \partial ^\alpha F(x)=\partial ^\alpha F^{l}(x)\}, \end{aligned}$$

and

$$\begin{aligned} E^{l}_2:=\bigcap _{\vert \alpha \vert \le g+m}\{ x\in {\mathbb {R}}^n: \partial ^\alpha f (x)=\partial ^\alpha f^{l}(x)\}. \end{aligned}$$

Now consider an arbitrary $S\in \Sigma _{m}^*$, define for simplicity

$$\begin{aligned} A_S:= A_{f,F}\cap \Upsilon _{F,S}, \quad A_S^l:= A_{f^{l},F^{l}}\cap \Upsilon _{F^{l},S}, \quad E:=\bigcup _{l=1}^\infty E^{l} \end{aligned}$$

and observe that

$$\begin{aligned} \begin{aligned} E^{l}\cap A_S \subset E^{l}\cap A_S^l \subset A_S^l \qquad (l=1,2,\ldots ). \end{aligned} \end{aligned}$$

Then

$$\begin{aligned} A_S = (A_S\cap E) \cup (A_S \!{\setminus }\! E) \subset \bigg ( \bigcup _{l=1}^{+\infty } A_S^l \bigg ) \cup ({\mathbb {R}}^n \!{\setminus }\! E) \end{aligned}$$

for all $S\in \Sigma _m^*$, hence

$$\begin{aligned} \bigcup _{S\in \Sigma _m^*} A_S \subset \left( \bigcup _{S\in \Sigma _m^*} \bigcup _{l=1}^{+\infty } A_S^l \right) \cup ({\mathbb {R}}^n{\setminus }E) = \left( \bigcup _{l=1}^{+\infty } \bigcup _{S\in \Sigma _m^*}A_S^l \right) \cup ({\mathbb {R}}^n{\setminus }E). \end{aligned}$$

Recalling (3.2), we get

$$\begin{aligned} A_{f,F}\cap \Upsilon _F^{m} \subset \bigcup _{l=1}^{+\infty } \left( A_{f^l,F^l}\cap \Upsilon _{F^l}^{m} \right) \cup ({\mathbb {R}}^n{\setminus }E). \end{aligned}$$

(3.4)

But for all $l=1, 2, \ldots$ there is a finite family of $(n-1)$-dimensional regularly imbedded $C^1$ submanifolds of ${\mathbb {R}}^n$ that covers $A_{f^l,F^l}\cap \Upsilon _{F^l}^{m}$, by Corollary 3.1. Moreover $B_{d,p}({\mathbb {R}}^n\setminus E)=0$, by (3.3). Thus:

• If $d=0$ and $p\in (1,+\infty )$, one has ${\mathcal L}^n({\mathbb {R}}^n\setminus E)=0$. Hence ${\mathcal L}^n (A_{f,F}\cap \Upsilon _F^{m})=0$, by (3.4);

• If $d=1$ and $1<p<n$, one has ${\mathcal H}^{n-1}({\mathbb {R}}^n\setminus E)=0$ (cf. Theorem 2.6.16 in [21]). Hence $A_{f,F}\cap \Upsilon _F^{m}$ is $(n-1)$-rectifiable, by (3.4).

$\square$

Corollary 3.3

Let us consider the special case when $k=1$, namely $G:=(G_1,\ldots , G_N)^t$ and $g= \deg G \ge 1$. Moreover, let $F=(F_1,\ldots , F_N)^t\in W^{g+d, p} ({\mathbb {R}}^n, {\mathbb {C}}^N)$ and $f\in W^{2\,g+d, p}({\mathbb {R}}^n, {\mathbb {C}}^k)$, with $p\in (1,+\infty )$ and $d\in \{0, 1\}$. Assume that for ${\mathcal H}^{n-d}$-a.e. $x\in A_{f,F}$ there exist $j,l\in \{1,\ldots , N\}$ such that

$$\begin{aligned} (G_{j}(D)F_{l})(x)\not = (G_{l}(D)F_{j})(x). \end{aligned}$$

(3.5)

The following facts hold:

• (1) If $d=0$ then ${\mathcal L}^n (A_{f,F})=0$;

• (2) If $d=1$ and $p<n$, then $A_{f,F}$ is $(n-1)$-rectifiable.

Proof

Let $S=[S_{jl}]$ be the matrix yielded by the first syzygies of G (cf. Example 2.1.1 in [5]), which can be obtained as follows. First of all, set $h:=N(N-1)/2$ and let $\{ (r_j, s_j) \}_{j=1}^h$ be the set of all the couples

$$\begin{aligned} (r,s)\in {\mathbb {N}}^2, \text { with }1\le r < s\le N \end{aligned}$$

ordered in some way (e.g. lexicographically). Then $S=[ S_{jl}]$ is the matrix of polynomials in ${\mathbb {C}}[\xi _1,\ldots , \xi _n]$ of dimension $h\times N$ such that

$$\begin{aligned} S_{jl}:={\left\{ \begin{array}{ll} G_{s_j}&{} \text {if }l=r_j \\ -G_{r_j}&{} \text {if }l=s_j \\ 0 &{} \text {otherwise}. \end{array}\right. } \qquad (j=1,\ldots , h). \end{aligned}$$

Observe that $\deg S =g$. Moreover, by assumption (3.5), one has

$$\begin{aligned} {\mathcal H}^{n-d}(Z)=0, \text { with }Z:=\{ x\in A_{f,F}\,\vert \, (S(D)F)(x)=0 \}. \end{aligned}$$

Since $A_{f,F}{\setminus } Z = A_{f,F}\cap \Upsilon _{F,S}$, one also has

$$\begin{aligned} A_{f,F} =( A_{f,F}\cap \Upsilon _{F,S})\cup Z \subset ( A_{f,F}\cap \Upsilon _{F}^{g})\cup Z. \end{aligned}$$

Hence (1) and (2) follow at once from Corollary 3.2. $\square$

3.2 Structure of $A_{f,F}$ at Points of the G-nonintegrability Set of $F\in W^{m, p}$: The Case of $f\in W^{g, p}$

In Sect. 3.1 we have proved that if $F\in W^{m, p}({\mathbb {R}}^n, {\mathbb {C}}^N)$ then every G-primitivity domain $A_{f,F}$ with $f\in W^{g+m, p}({\mathbb {R}}^n, {\mathbb {C}}^k)$ intersects the G-nonintegrability set of F in a set of Lebesgue measure zero. Things change if one considers $f\in W^{g, p}({\mathbb {R}}^n, {\mathbb {C}}^k)$. In fact, as we will see, it can happen to come across functions $f\in W^{g, p}({\mathbb {R}}^n, {\mathbb {C}}^k)$ such that ${\mathcal L}^n(A_{f,F}\cap \Upsilon _F^{m})>0$ (cf. Theorem 4.1 and Corollary 4.1 in the next section). However, as Corollary 3.4 below shows, even in this case the G-nonintegrability properties strongly shape the structure of $A_{f,F}$ at points of $\Upsilon _F^{m}$. More precisely: if $S\in \Sigma _m$ then, at a.e. point of $\Upsilon _{F,S}$, the set $A_{f,F}$ has density lower than $n+p\deg S/(p-1)$. Consequently, at a.e. point of $\Upsilon _{F}^{m}$, the set $A_{f,F}$ has density lower than $n+pm/(p-1)$, cf. Corollary 3.5.

Theorem 3.2

Let $p\in (1,+\infty )$ and consider

$$\begin{aligned} f =(f_1,\ldots , f_k)^t \in L_{\text {loc}}^1 (\Omega , {\mathbb {C}}^k), \quad \Phi =(\Phi _1,\ldots ,\Phi _N)^t \in L_{\text {loc}}^p (\Omega , {\mathbb {C}}^N) \end{aligned}$$

such that $G(D)f=\Phi$ holds in the sense of distributions. Moreover, let $F=(F_1,\ldots , F_N)^t\in W_{\text {loc}}^{m,p} (\Omega , {\mathbb {C}}^N)$ with $m\ge 1$ and define

$$\begin{aligned} B_{\Phi , F}:=\{ x\in \Omega \, \vert \, \Phi (x)=F(x)\}. \end{aligned}$$

Then there exists a null measure set $Z\subset \Omega$ such that

$$\begin{aligned} S(D)F=0 \text { in }\Omega \cap B_{\Phi , F}^{(n+\delta _S)}\setminus Z, \end{aligned}$$

for all $S\in \Sigma _m$, where $\delta _S:= p \deg S/(p-1)$.

Proof

First of all, we observe that:

• There exists a null measure set $Z_1\subset \Omega$ such that $\vert F(x)\vert , \vert \Phi (x)\vert < +\infty$ and

  

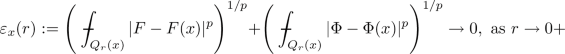
  for all $x\in \Omega \setminus Z_1$ (cf. Corollary 1 in [12, Sect.1.7.1]);

• There exists a null measure set $Z_2\subset \Omega$ such that $\vert \partial ^\alpha F (x)\vert <+\infty$,

  

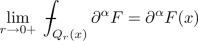
  and

  

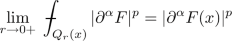
  for all $x\in \Omega \setminus Z_2$ and for all $\alpha \in {\mathbb {N}}^n$ with $\vert \alpha \vert \le m$ (cf. Theorem 1 in [12, Sect.1.7.1]);

• From (2.10) it follows that $Z_3:=B_{\Phi ,F}^{(n)}{\setminus }B_{\Phi ,F}$ is a null measure set, while

  $$\begin{aligned} B_{\Phi ,F}^{(n+\delta _S)}{\setminus }B_{\Phi ,F}\subset Z_3, \text { for all }S\in \Sigma _m \end{aligned}$$

  (3.6)

  by Remark 2.1.

Let us define $Z:=Z_1\cup Z_2\cup Z_3$. Moreover, consider $S\in \Sigma _m$ and $x\in \Omega \cap B_{\Phi ,F}^{(n+\delta _S)}{\setminus }Z$. Also, consider $\rho \in (1/2, 1)$ and let $r\in (0,1)$ be small enough so that $\overline{Q_r (x)}\subset \Omega$. Recall from Sect. 2.5 that a function $\varphi _{\rho , r, x}\in C^\infty _c ({\mathbb {R}}^n, [0,1])$ has to exist such that

$$\begin{aligned} \varphi _{\rho , r, x}\vert _{Q_{\rho r}(x)}\equiv 1,\quad \varphi _{\rho , r, x}\vert _{{\mathbb {R}}^n\setminus Q_{r}(x)}\equiv 0 \end{aligned}$$

and

$$\begin{aligned} \Vert \partial ^\alpha \varphi _{\rho ,r, x} \Vert _{\infty ,{\mathbb {R}}^n} \le \frac{C(\alpha )}{(1-\rho )^{\vert \alpha \vert }r^{\vert \alpha \vert }} \end{aligned}$$

(3.7)

for all $\alpha \in {\mathbb {N}}^n$, where $C(\alpha )$ is a number depending only on $\alpha$ (and n). In the formulas below we set for simplicity

$$\begin{aligned} Q_r:=Q_r(x), \quad Q_{\rho r}:= Q_{\rho r} (x), \quad \varphi _{\rho , r}:=\varphi _{\rho , r, x}. \end{aligned}$$

Then, by (2.4), we obtain (for all $j\in \{ 1,\ldots , h\}$, where h is the positive integer such that $S\in \Sigma _{m,h}$)

$$\begin{aligned} \begin{aligned} \int _{Q_r}(S(D)F)_j \varphi _{\rho , r}&=\sum _{l=1}^N \int _{Q_r}(S_{jl}(D)F_l) \varphi _{\rho , r} = \sum _{l=1}^N \int _{Q_r}(S_{jl}^*(D)\varphi _{\rho , r})F_l\\&= \sum _{l=1}^N \int _{Q_r{\setminus }B_{\Phi ,F}}(S_{jl}^*(D)\varphi _{\rho , r})F_l + \sum _{l=1}^N \int _{Q_r\cap B_{\Phi ,F}}(S_{jl}^*(D)\varphi _{\rho , r})\Phi _l \end{aligned} \end{aligned}$$

that is

$$\begin{aligned} \int _{Q_r}(S(D)F)_j \varphi _{\rho , r} =I_{r,j}+ \sum _{l=1}^N \int _{Q_r{\setminus }B_{\Phi ,F}}(S_{jl}^*(D)\varphi _{\rho , r})(F_l - \Phi _l) \end{aligned}$$

(3.8)

where

$$\begin{aligned} I_{r,j}:= \sum _{l=1}^N \int _{Q_r}(S_{jl}^*(D)\varphi _{\rho , r})\Phi _l = \sum _{q=1}^k\int _{Q_r} \left( (SG)_{jq}^* (D)\, \varphi _{\rho , r}\right) f_q=0 \end{aligned}$$

(3.9)

by (2.8) and the assumption $SG=0$. By assumption and by (3.6), one also has

$$\begin{aligned} x\in B_{\Phi ,F}^{(n+\delta _S)}\setminus Z_3\subset B_{\Phi ,F}^{(n+\delta _S)}\cap B_{\Phi ,F} \end{aligned}$$

hence

$$\begin{aligned} \Phi (x)= F(x),\quad {\mathcal L}^n (Q_r{\setminus }B_{\Phi ,F}) = o (r^{n+\delta _S}). \end{aligned}$$

(3.10)

From (3.7), (3.8), (3.9), (3.10) and Hölder inequality, we get

$$\begin{aligned} \begin{aligned} \left| \int _{Q_r}(S(D)F)_j \varphi _{\rho , r}\right|&=\left| \sum _{l=1}^N \int _{Q_r{\setminus }B_{\Phi ,F}}(S_{jl}^*(D)\varphi _{\rho , r})(F_l - \Phi _l )\right| \\&\le \sum _{l=1}^N \bigg (\int _{Q_r{\setminus }B_{\Phi ,F}}\vert S_{jl}^*(D)\varphi _{\rho , r}\vert \,\vert F_l - F_l (x)\vert \\&\quad + \int _{Q_r{\setminus }B_{\Phi ,F}}\vert S_{jl}^*(D)\varphi _{\rho , r}\vert \, \vert \Phi _l - \Phi _l (x) \vert \bigg ) \\&\le \sum _{l=1}^N \Vert S_{jl}^*(D)\varphi _{\rho ,r}\Vert _{\infty , Q_r} \bigg [ \bigg (\int _{Q_r}\vert F- F(x)\vert ^p\bigg )^{1/p}\\&\quad + \bigg (\int _{Q_r}\vert \Phi - \Phi (x)\vert ^p\bigg )^{1/p} \bigg ]\, {\mathcal L}^n (Q_r{\setminus }B_{\Phi ,F})^{1-1/p}\\&\le \varepsilon _x(r) r^{n/p}\, o(r^{n+\delta _S})^{1-1/p} \mathop \sum \limits _{\begin{array}{c} \alpha \in {\mathbb {N}}^n\\ \vert \alpha \vert \le \deg S \end{array}} \frac{1}{(1-\rho )^{\vert \alpha \vert }r^{\vert \alpha \vert }} \end{aligned} \end{aligned}$$

that is

$$\begin{aligned} \left| \int _{Q_r}(S(D)F)_j \varphi _{\rho , r}\right| \le \frac{\varepsilon _x(r) o(r^{n+\delta _S (p-1)/p})}{(1-\rho )^{\deg S}r^{\deg S}} = \frac{\varepsilon _x(r) o(r^{n})}{(1-\rho )^{\deg S}}. \end{aligned}$$

(3.11)

On the other hand

$$\begin{aligned} \begin{aligned} \left| \int _{Q_r}(S(D)F)_j \varphi _{\rho , r}\right|&\ge \left| \int _{Q_{\rho r}}(S(D)F)_j \varphi _{\rho , r}\right| -\left| \int _{Q_r{\setminus }Q_{\rho r}}(S(D)F)_j \varphi _{\rho , r}\right| \\&= \left| \int _{Q_{\rho r}}(S(D)F)_j \right| -\left| \int _{Q_r{\setminus }Q_{\rho r}}(S(D)F)_j \varphi _{\rho , r}\right| \end{aligned} \end{aligned}$$

(3.12)

and

(3.13)

where $C_1$ and $C_2$ do not depend on r and $\rho$.

From (3.11), (3.12) and (3.13) it follows that

where $C_3$ does not depend on r and $\rho$. Recalling that $x\in \Omega {\setminus }(Z_1\cup Z_2)$ and passing to the limit for $r\rightarrow 0+$, we obtain

$$\begin{aligned} \rho ^n \left| (S(D)F)_j (x)\right| \le C_3\, (1-\rho ^n)^{1-1/p} \sum _{\vert \alpha \vert \le m}\vert \partial ^\alpha F (x)\vert \end{aligned}$$

for all $j\in \{ 1,\ldots , h\}$. We conclude by passing to the limit for $\rho \rightarrow 1-$. $\square$

Corollary 3.4

Let $F\in W_{\text {loc}}^{m,p} (\Omega , {\mathbb {C}}^N)$, with $m\ge 1$ and $p\in (1,+\infty )$. Then one has ${\mathcal L}^n( A_{f,F}^{(n+\delta _S)} \cap \Upsilon _{F,S})=0$ for all $f\in W_{\text {loc}}^{g,p} (\Omega , {\mathbb {C}}^k)$ and $S\in \Sigma _m$, where $\delta _S:= p \deg S/(p-1)$.

Proof

Let us consider $f\in W_{\text {loc}}^{g,p} (\Omega , {\mathbb {C}}^k)$ and $S\in \Sigma _m$. Moreover set $\Phi := G(D)f$ and observe that $B_{\Phi ,F}=A_{f, F}$. Then one has

$$\begin{aligned} (S(D)F)(x)=0 \text { for all }x\in \Omega \cap A_{f,F}^{(n+\delta _S)}\setminus Z \end{aligned}$$

by Theorem 3.2. It follows that

$$\begin{aligned} \emptyset =\Upsilon _{F,S} \cap (\Omega \cap A_{f,F}^{(n+\delta _S)}\setminus Z) =\Upsilon _{F,S}\cap A_{f,F}^{(n+\delta _S)}\setminus Z \end{aligned}$$

hence $\Upsilon _{F,S}\cap A_{f,F}^{(n+\delta _S)}\subset Z$. $\square$

Corollary 3.5

Let $F\in W_{\text {loc}}^{m,p} (\Omega , {\mathbb {C}}^N)$, with $m\ge 1$ and $p\in (1,+\infty )$. Then one has ${\mathcal L}^n(A_{f,F}^{(n+pm/(p-1))} \cap \Upsilon _{F}^{m})=0$ for all $f\in W_{\text {loc}}^{g,p} (\Omega , {\mathbb {C}}^k)$.

Proof

Let $f\in W_{\text {loc}}^{g,p} (\Omega , {\mathbb {C}}^k)$. By Remark 2.1 one has

$$\begin{aligned} A_{f,F}^{(n+pm/(p-1))}\subset A_{f,F}^{(n+\delta _S)} \end{aligned}$$

for all $S\in \Sigma _m$, with $\delta _S:= p \deg S/(p-1)$. Hence and recalling (3.2), we obtain

$$\begin{aligned}&A_{f,F}^{(n+pm/(p-1))}\cap \Upsilon _{F}^{m}\\&=\bigcup _{S\in \Sigma _m^*} \left( A_{f,F}^{(n+pm/(p-1))}\cap \Upsilon _{F,S}\right) \subset \bigcup _{S\in \Sigma _m^*} \left( A_{f,F}^{(n+\delta _S)}\cap \Upsilon _{F,S}\right) . \end{aligned}$$

The conclusion follows from Corollary 3.4. $\square$

Remark 3.5

For $F\in W_{\text {loc}}^{m,p} (\Omega , {\mathbb {C}}^N)$ and $f\in W_{\text {loc}}^{g,p} (\Omega , {\mathbb {C}}^k)$, with $p>1$, the property stated in Proposition 3.1 follows at once from Corollary 3.4. Indeed, under the assumptions of Proposition 3.1, one has $B\subset \Omega \cap A_{f,F}^{(n+d)}\subset A_{f,F}^{(n+d)}$ for all $d\ge 0$. From Corollary 3.5 it follows that ${\mathcal L}^n(\Upsilon _{F,S}\cap B)=0$.

Corollary 3.6

Let $F\in W^{1,p}_{\text {loc}}(\Omega , {\mathbb {C}}^N)$ with $p\in [n,+\infty )$ and assume that there exist S and a set of locally finite perimeter $E\subset \Omega$ (of positive measure) such that $SG=0$, $\deg S=1$ and $S(D)F\not =0$ a.e. in E. Then there is no function $f\in W^{g,p}_{\text {loc}}(\Omega , {\mathbb {C}}^k)$ such that $G(D)f=F$ a.e. in E.

Proof

Suppose (by absurd) that there exists $f\in W^{g,p}_{\text {loc}}(\Omega , {\mathbb {C}}^k)$ such that $G(D)f=F$ a.e. in E, that is ${\mathcal L}^n(E\setminus A_{f,F})=0$. Thus $E^{(r)}\subset A_{f,F}^{(r)}$ for all $r\ge n$, in particular

$$\begin{aligned} E^{(n+n/(n-1))}\subset A_{f,F}^{(n+n/(n-1))} \end{aligned}$$

hence

$$\begin{aligned} E\cap E^{(n+n/(n-1))}\subset E\cap A_{f,F}^{(n+n/(n-1))}. \end{aligned}$$

(3.14)

On the other hand, Corollary 3.4 yields

$$\begin{aligned} {\mathcal L}^n \left( E\cap A_{f,F}^{(n+p/(p-1))}\right) =0. \end{aligned}$$

(3.15)

Moreover, since $p\ge n$, one has $n+p/(p-1)\le n+n/(n-1)$ so that

$$\begin{aligned} A_{f,F}^{(n+n/(n-1))} \subset A_{f,F}^{(n+p/(p-1))} \end{aligned}$$

(3.16)

by the second point in Remark 2.1. From (3.14), (3.15) and (3.16) we get now

$$\begin{aligned} {\mathcal L}^n \left( E\cap E^{(n+n/(n-1))} \right) =0 \end{aligned}$$

which contradicts (1) of Proposition 2.1. $\square$

Corollary 3.7

Let $k=1$, namely $G:=(G_1,\ldots , G_N)^t$ and $g=\deg G \ge 1$. Moreover let $f\in W_{\text {loc}}^{g,p} (\Omega , {\mathbb {C}})$ and $F=(F_1,\ldots ,F_N)^t\in W_{\text {loc}}^{g,p}(\Omega ,{\mathbb {C}}^N)$, with $p\in (1,+\infty )$. Then one has

$$\begin{aligned} G_j(D)F_l = G_l(D)F_j \text { a.e. in }\Omega \cap A_{f,F}^{(n+ \delta )} \end{aligned}$$

for all $j,l\in \{1,\ldots , N\}$, with $\delta := pg/(p-1)$.

Proof

Let $S=[S_{jl}]$ be the matrix considered in the proof of Corollary 3.3. Since $S\in \Sigma _g$, the conclusion follows at once from Corollary 3.4.

Example 3.1

From Corollary 3.7 with $N=n$ and $G_j(\xi _1,\ldots ,\xi _n)=i \xi _j$, i.e., G(D) is the gradient operator, we get immediately the following result which generalizes the obvious property of equality of mixed partial derivatives for Sobolev functions (cf. (i) of Theorem 1 in [11, Sect.5.2.3]): Let $f\in W^{1,p}_{\text {loc}}(\Omega , {\mathbb {C}})$ and $F\in W^{1,p}_{\text {loc}}(\Omega , {\mathbb {C}}^n)$, with $p\in (1,+\infty )$. Then, for all $j,l=1,\ldots , n$, one has $\partial _j F_l = \partial _l F_j$ a.e. in $\Omega \cap A_{f,F}^{(n+p/(p-1))}$.

4 A Lusin Type Result for a Class of Linear Partial Differential Operators

The proofs of Lemma 4.1 and Theorem 4.1 below go along the lines of those of Lemma 4.1 and Theorem 4.1 in [10], respectively. Several steps are actually the same, but the intertwining of these replicas with the new arguments, as well as the complexity of the proof, make it (in our opinion) impossible to cut the presentation without compromising clarity. For this reason we have decided to provide them in full.

Lemma 4.1

Let $G_1,\ldots ,G_N\in {\mathbb {C}}[\xi _1,\ldots , \xi _n]$ and define $G(D):=(G_1(D),\ldots ,G_N(D))^t$. Assume that there exist $\alpha ^{(1)},\ldots , \alpha ^{(N)}\in {\mathbb {N}}^n$ such that

$$\begin{aligned} G_r(D) x^{\alpha ^{(s)}}= {\left\{ \begin{array}{ll} 0&{} \text {if }s\not =r\\ c_r\in {\mathbb {C}}{\setminus }\{0\}&{} \text {if }s= r \end{array}\right. } \end{aligned}$$

and

$$\begin{aligned} \min _j \vert \alpha ^{(j)}\vert \ge g:=\max _j \mathrm{deg}\, G_j. \end{aligned}$$

(4.1)

Moreover consider an open set $\Omega \subset {\mathbb {R}}^n$ such that ${\mathcal L}^n(\Omega )<+\infty$, a bounded function $f=(f_1,\ldots ,f_N)^t \in C(\Omega , {\mathbb {C}}^N)$, $\varepsilon \in (0,1/2)$ and $\eta >0$. Then there exist a compact set $K\subset \Omega$ and a function $v\in C_c^\infty (\Omega , {\mathbb {C}})$ such that

• (1) ${\mathcal L}^n (\Omega \setminus K)\le \varepsilon {\mathcal L}^n(\Omega )$;

• (2) $\Vert G(D)v - f\Vert _{\infty , K}\le \eta$;

• (3) $\Vert G(D)v\Vert _{p,\Omega }\le C\, \varepsilon ^{\frac{1}{p} -g} \Vert f\Vert _{p,\Omega }$ for all $p\in [1, +\infty )$, where C is a constant not depending on $f, \varepsilon , \eta , p$.

Proof

According to the first steps in the proof of [2, Lemma 7], we can find $\delta \in (0,1)$ and a compact set $K\subset \Omega$ with the following properties:

• The estimate (1) holds and

  $$\begin{aligned} K=\bigcup _{j\in J}Q_j, \end{aligned}$$

  where $\{Q_j\}_{j\in J}$ is a finite family of closed cubes of side $(1-\varepsilon /2n)\delta$, whose centers $y_j$ belong to the lattice $(\delta {\mathbb {Z}})^n$;

• For $j\in J$, let $T_j$ be the closed cube of side $\delta$ centered at $y_j$. Then, for all $j\in J$, one has $T_j\subset \Omega$ and

  $$\begin{aligned} \vert f(x)-f(y)\vert \le \eta , \text { whenever } x,y\in T_j. \end{aligned}$$

  (4.2)

Now, for all $j\in J$ and $x\in {\mathbb {R}}^n$, set

$$\begin{aligned} \Phi _j(x):=\varphi _{\rho ,\delta /2} (x-y_j), \text { with } \rho :=1-\frac{\varepsilon }{2n}, \end{aligned}$$

and observe that

$$\begin{aligned} \Phi _j\in C^\infty ({\mathbb {R}}^n, [0,1]),\quad \Phi _j\vert _{Q_j}\equiv 1,\quad \Phi _j\vert _{{\mathbb {R}}^n{\setminus }T_j}\equiv 0, \end{aligned}$$

(4.3)

by (2.11). Moreover

$$\begin{aligned} \Vert \partial ^\alpha \Phi _j \Vert _{\infty ,{\mathbb {R}}^n} \le C(\alpha ) \varepsilon ^{-\vert \alpha \vert }\delta ^{-\vert \alpha \vert } \end{aligned}$$

(4.4)

for all $\alpha \in {\mathbb {N}}^n$, by (2.12). Then define

and the function

$$\begin{aligned} v(x):=\sum _{j\in J}\Phi _j(x)\sum _{s=1}^N\frac{\mu _{sj}}{c_s} (x-y_j)^{\alpha ^{(s)}}, \quad x\in \Omega . \end{aligned}$$

One obviously has $v\in C_c^{\infty }(\Omega , {\mathbb {C}})$, by (4.3). To prove (2) and (3), we need the explicit expressions of the polynomials $G_r$, that is

$$\begin{aligned} G_r(\xi _1,\ldots ,\xi _n)=\mathop \sum \limits _{\begin{array}{c} \alpha \in {\mathbb {N}}^n\\ \vert \alpha \vert \le g \end{array}} c_{\alpha }^{(r)}\xi ^\alpha \quad (c_{\alpha }^{(r)}\in {\mathbb {C}}) \end{aligned}$$

where the coefficients $c_{\alpha }^{(r)}$ are assumed to be zero when $\vert \alpha \vert$ exceeds the degree of $G_r$. Recalling (2.1), we find (for $x\in \Omega$)

$$\begin{aligned}{}[G_r(D)v](x) =\sum _{j\in J}\sum _{s=1}^N\mathop \sum \limits _{\begin{array}{c} \alpha \in {\mathbb {N}}^n\\ \vert \alpha \vert \le g \end{array}} \frac{(-i)^{\vert \alpha \vert } c_\alpha ^{(r)} \mu _{sj}}{c_s} \partial ^\alpha \left[ \Phi _j(x) (x-y_j)^{\alpha ^{(s)}} \right] \end{aligned}$$

where, for suitable integer coefficients $k_\beta ^{(\alpha )}$ (which coincide with 1 for $\beta =0$ and $\beta =\alpha$), one has

$$\begin{aligned} \begin{aligned} \partial ^\alpha \left[ \Phi _j(x) (x-y_j)^{\alpha ^{(s)}} \right]&= \mathop \sum \limits _{\begin{array}{c} \beta \in {\mathbb {N}}^n\\ \beta \le \alpha \end{array}} k_\beta ^{(\alpha )} \partial ^\beta \Phi _j(x) \, \partial ^{\alpha -\beta }[(x-y_j)^{\alpha ^{(s)}}]\\&= \Phi _j(x) \, \partial ^{\alpha }[(x-y_j)^{\alpha ^{(s)}} ]\\&+\mathop \sum \limits _{\begin{array}{c} \beta \in {\mathbb {N}}^n\\ 0<\beta \le \alpha \end{array}} k_\beta ^{(\alpha )} \partial ^\beta \Phi _j(x) \, \partial ^{\alpha -\beta }[(x-y_j)^{\alpha ^{(s)}}]. \end{aligned} \end{aligned}$$

It follows that (for $x\in \Omega$)

$$\begin{aligned} \begin{aligned}{}[G_r(D)v](x)&=\sum _{j\in J} \Phi _j(x)\sum _{s=1}^N \frac{\mu _{sj}}{c_s}\, G_r(D) [(x-y_j)^{\alpha ^{(s)}}] \\&\quad + \sum _{j\in J}\sum _{s=1}^N\mathop \sum \limits _{\begin{array}{c} \alpha \in {\mathbb {N}}^n\\ 1\le \vert \alpha \vert \le g \end{array}} \frac{(-i)^{\vert \alpha \vert } c_\alpha ^{(r)} \mu _{sj}}{c_s} \mathop \sum \limits _{\begin{array}{c} \beta \in {\mathbb {N}}^n\\ 0<\beta \le \alpha \end{array}} k_\beta ^{(\alpha )} \partial ^\beta \Phi _j(x) \, \partial ^{\alpha -\beta }[(x-y_j)^{\alpha ^{(s)}}]\\&=\sum _{j\in J} \Phi _j(x) \mu _{rj} \\&\quad + \sum _{j\in J}\sum _{s=1}^N\mathop \sum \limits _{\begin{array}{c} \alpha \in {\mathbb {N}}^n\\ 1\le \vert \alpha \vert \le g \end{array}} \mathop \sum \limits _{\begin{array}{c} \beta \in {\mathbb {N}}^n\\ 0<\beta \le \alpha \end{array}} \frac{(-i)^{\vert \alpha \vert } c_\alpha ^{(r)} k_\beta ^{(\alpha )}}{c_s} \, \mu _{sj}\, \partial ^\beta \Phi _j(x) \, \partial ^{\alpha -\beta }[(x-y_j)^{\alpha ^{(s)}}]. \end{aligned} \end{aligned}$$

(4.5)

In the formulae below, $C_1, C_2, \ldots$ will denote constants which do not depend on $f, \varepsilon , \eta , p$. From the previous identity, we obtain (for all $j\in J$ and $x\in \Omega$)

$$\begin{aligned} \begin{aligned} \vert [G(D)v](x)\vert&\le \sum _{j\in J} \Phi _j (x) \vert \mu _j\vert \\&\quad + C_1 \sum _{j\in J} \vert \mu _j \vert \sum _{s=1}^N\mathop \sum \limits _{\begin{array}{c} \alpha \in {\mathbb {N}}^n\\ 1\le \vert \alpha \vert \le g \end{array}} \mathop \sum \limits _{\begin{array}{c} \beta \in {\mathbb {N}}^n\\ 0<\beta \le \alpha \end{array}} \vert \partial ^\beta \Phi _j (x)\vert \,\sup _{\xi \in T_j}\vert \partial ^{\alpha -\beta }[(\xi -y_j)^{\alpha ^{(s)}}]\vert \end{aligned} \end{aligned}$$

where

$$\begin{aligned} \sup _{\xi \in T_j}\vert \partial ^{\alpha -\beta }[(\xi -y_j)^{\alpha ^{(s)}}]\vert \le C_2\, \delta ^{\vert \alpha ^{(s)}\vert -\vert \alpha \vert + \vert \beta \vert }. \end{aligned}$$

Hence, by also recalling (4.4):

$$\begin{aligned} \begin{aligned} \vert [G(D)v](x)\vert&\le \sum _{j\in J} \chi _{T_j}(x)\vert \mu _j \vert \\&\quad + C_3 \sum _{j\in J} \chi _{T_j{\setminus }Q_j}(x) \vert \mu _j\vert \sum _{s=1}^N\mathop \sum \limits _{\begin{array}{c} \alpha \in {\mathbb {N}}^n\\ 1\le \vert \alpha \vert \le g \end{array}} \mathop \sum \limits _{\begin{array}{c} \beta \in {\mathbb {N}}^n\\ 0<\beta \le \alpha \end{array}} \varepsilon ^{-\vert \beta \vert }\delta ^{-\vert \beta \vert }\,\delta ^{\vert \alpha ^{(s)}\vert -\vert \alpha \vert + \vert \beta \vert } \end{aligned} \end{aligned}$$

for all $x\in \Omega$. Since $\delta \in (0,1)$, $\varepsilon \in (0,1/2)$ and (4.1) holds, it follows that

$$\begin{aligned} \begin{aligned} \vert [G(D)v](x)\vert&\le \sum _{j\in J} \vert \mu _j \vert \left( \chi _{T_j}(x) + C_3 \chi _{T_j{\setminus }Q_j}(x) \sum _{s=1}^N\mathop \sum \limits _{\begin{array}{c} \alpha \in {\mathbb {N}}^n\\ 1\le \vert \alpha \vert \le g \end{array}} \delta ^{g -\vert \alpha \vert } \mathop \sum \limits _{\begin{array}{c} \beta \in {\mathbb {N}}^n\\ 0<\beta \le \alpha \end{array}} \varepsilon ^{-\vert \beta \vert } \right) \\&\le \sum _{j\in J} \vert \mu _j \vert \left( \chi _{T_j}(x) + C_4 \varepsilon ^{-g} \chi _{T_j{\setminus }Q_j}(x) \right) \end{aligned} \end{aligned}$$

for all $x\in \Omega$. Thus

$$\begin{aligned} \Vert G(D)v\Vert _{p,\Omega } \le \big \Vert \sum _{j\in J} \vert \mu _j \vert \, \chi _{T_j}\big \Vert _{p,\Omega } + C_4 \varepsilon ^{-g}\big \Vert \sum _{j\in J} \vert \mu _j \vert \, \chi _{T_j{\setminus }Q_j}\big \Vert _{p,\Omega }. \end{aligned}$$

(4.6)

Moreover, by Jensen’s inequality, one has

hence

$$\begin{aligned} \big \Vert \sum _{j\in J} \vert \mu _j \vert \, \chi _{T_j}\big \Vert _{p,\Omega } \le \Vert f\Vert _{p,\Omega }. \end{aligned}$$

(4.7)

Analogously (recalling that $1-nt\le (1-t)^n$, for all $t\le 1$, hence $1-(1-\frac{\varepsilon }{2n})^n\le \frac{\varepsilon }{2}$) we get

hence

$$\begin{aligned} \big \Vert \sum _{j\in J} \vert \mu _j \vert \, \chi _{T_j{\setminus }Q_j}\big \Vert _{p,\Omega } \le \bigg (\frac{\varepsilon }{2}\bigg )^{1/p}\Vert f\Vert _{p,\Omega }. \end{aligned}$$

(4.8)

Finally, inequality (3) follows from (4.6), (4.7) and (4.8). $\square$

Remark 4.1

As we observed in Remark 4.1 of [10], when $N\ge 2$ the condition assumed for G in Lemma 4.1 forces the components $G_j$ to be different from each other.

Remark 4.2

Consider an open set $\Omega \subset {\mathbb {R}}^n$ with finite measure, $F\in L^\infty (\Omega ,{\mathbb {C}}^N)$ such that $\Vert F\Vert _{\infty ,\Omega }>0$ and recall that $\lim _{q\rightarrow +\infty }\Vert F\Vert _{q,\Omega } = \Vert F\Vert _{\infty ,\Omega }$ (cf. Theorem 2.8 in [1]). Then, as it is outlined in [2], an easy argument shows that the function $q\mapsto {\mathcal L}^n(\Omega )^{1/q}/ \Vert F\Vert _{q,\Omega }$ has a finite positive upper bound on $[1,+\infty )$.

Theorem 4.1

Let $G_1,\ldots , G_N\in {\mathbb {C}}[\xi _1,\ldots , \xi _n]$ and $\Omega \subset {\mathbb {R}}^n$ satisfy the same hypotheses as in Lemma 4.1. Moreover assume that there exist a nonnegative integer $m\le g=\deg G$ and a positive real number $c_*$ such that

$$\begin{aligned} \Vert G(D)\varphi \Vert _{\infty ,\Omega } \ge c_* \mathop {\max }\limits _{\begin{array}{c} \alpha \in {\mathbb {N}}^n\\ \vert \alpha \vert = m \end{array}}\Vert \partial ^{\alpha }\varphi \Vert _{\infty ,\Omega } \end{aligned}$$

(4.9)

for all $\varphi \in C_c^\infty (\Omega ,{\mathbb {C}})$. Then, for every Borel function $F:\Omega \rightarrow {\mathbb {C}}^N$ and for every $\varepsilon \in (0,1)$, there exist an open set ${\mathcal {O}}\subset \Omega$, $f\in C_0^m(\Omega ,{\mathbb {C}})$ and $\Phi \in C_0(\Omega ,{\mathbb {C}}^N)\cap L^\infty (\Omega ,{\mathbb {C}}^N)$ with the following properties:

• (1) ${\mathcal L}^n ({\mathcal {O}})\le \varepsilon {\mathcal L}^n (\Omega )$;

• (2) $\Phi =F$ a.e. in $\Omega {\setminus }{\mathcal {O}}$;

• (3) The equality $G(D)f=\Phi$ holds in the sense of distributions;

• (4) In the special case $m=g$ one has $G(D)f=\Phi$ in $\Omega$, hence $G(D)f=F$ a.e. in $\Omega {\setminus }{\mathcal {O}}$. Moreover one has

  $$\begin{aligned} \Vert G(D)f\Vert _{p,\Omega } \le C\, 2^{g+2} \, \varepsilon ^{\frac{1}{p}-g} \Vert F\Vert _{p,\Omega }, \text { for all }p\in [1,+\infty ) \end{aligned}$$

  (4.10)

  and

  $$\begin{aligned} \Vert G(D)f\Vert _{\infty ,\Omega } \le C\, 2^{g+2} \, \varepsilon ^{-g} \Vert F\Vert _{\infty ,\Omega }, \end{aligned}$$

  (4.11)

  where C is the constant of (3) in Lemma 4.1.

Proof

First of all observe that if $F=0$ a.e. in $\Omega$, then we can find an open set ${\mathcal {O}}$ verifying

$$\begin{aligned} F\vert _{\Omega \setminus {\mathcal {O}}}\equiv 0,\quad {\mathcal L}^n({\mathcal {O}})\le \varepsilon {\mathcal L}^n (\Omega ), \end{aligned}$$

so that statements (1–4) are obviously verified with $f\equiv 0$ and $\Phi \equiv 0$. Thus we can assume $\Vert F\Vert _{\infty ,\Omega }>0$. The proof below is divided into two steps.

Step 1: If $F\in C(\Omega ,{\mathbb {C}}^N)\cap L^\infty (\Omega ,{\mathbb {C}}^N)$.

Let us define $f_0:= F$ and show that there exist two sequences of functions

$$\begin{aligned} \{ f_j\}_{j=1}^\infty \subset C(\Omega ,{\mathbb {C}}^N)\cap L^\infty (\Omega ,{\mathbb {C}}^N), \quad \{ v_j\}_{j=1}^\infty \subset C_c^\infty (\Omega ,{\mathbb {C}}) \end{aligned}$$

and a sequence $\{ K_j\}_{j=1}^\infty$ of compact subsets of $\Omega$ satisfying the following properties, for all $j \ge 1$:

• (i) ${\mathcal L}^n(\Omega {\setminus }K_j)\le 2^{-j}\varepsilon \, {\mathcal L}^n (\Omega )$;

• (ii) $\Vert G(D)v_j - f_{j-1}\Vert _{\infty , K_j} \le 2^{-j (g+1)} s^{-1}$, where $s:=\sup _{q\in [1,+\infty )} {\mathcal L}^n(\Omega )^{1/q}/ \Vert F\Vert _{q,\Omega }$. Recall that $0<s<+\infty$, by Remark 4.2;

• (iii) $\Vert G(D)v_j \Vert _{p, \Omega } \le 2^{j(g -1/p)} C \varepsilon ^{1/p-g} \Vert f_{j-1}\Vert _{p,\Omega }$, for all $p\in [1,+\infty )$, where C is the constant of (3) in Lemma 4.1;

• (iv) $f_j(x)=f_{j-1} (x)-[G(D)v_j](x)$ for all $x\in K_j$ and $\Vert f_j\Vert _{\infty , \Omega } = \Vert f_{j-1}-G(D)v_j\Vert _{\infty , K_j}$.

Such a statement is proved by the following induction argument:

• First of all, use Lemma 4.1 to get a compact set $K_1\subset \Omega$ and $v_1\in C_c^\infty (\Omega ,{\mathbb {C}})$ such that (i), (ii) and (iii) hold with $j=1$. Then we get $f_1\in C(\Omega ,{\mathbb {C}}^N)\cap L^\infty (\Omega ,{\mathbb {C}}^N)$ satisfying (iv) with $j=1$, by extending the function

  $$\begin{aligned} f_0(x)-[G(D)v_1](x), \quad x\in K_1 \end{aligned}$$

  by means of Tietze’s theorem [19, 20.4].

• Now suppose to have

  $$\begin{aligned} \{ f_j\}_{j=1}^H\subset C(\Omega ,{\mathbb {C}}^N)\cap L^\infty (\Omega ,{\mathbb {C}}^N), \quad \{ v_j\}_{j=1}^H \subset C_c^\infty (\Omega ,{\mathbb {C}}) \end{aligned}$$

  and a family $\{ K_j\}_{j=1}^H$ of compact subsets of $\Omega$ such that the properties (i-iv) above are satisfied for $j=1,\ldots , H$, where H is any positive integer. By using again Lemma 4.1 we can find a compact set $K_{H+1}\subset \Omega$ and $v_{H+1}\in C_c^\infty (\Omega ,{\mathbb {C}})$ such that (i), (ii) and (iii) hold with $j=H+1$. Moreover, by Tietze’s theorem [19, 20.4], we get $f_{H+1}\in C(\Omega ,{\mathbb {C}}^N)\cap L^\infty (\Omega ,{\mathbb {C}}^N)$ which satisfies (iv) with $j=H+1$.

Now let

$$\begin{aligned} {\mathcal {O}}:= \Omega {\setminus }\bigcap _{j=1}^\infty K_j \end{aligned}$$

(4.12)

and note that (1) follows at once from (i) above. Moreover, from (iii), we get

$$\begin{aligned} \begin{aligned} \sum _{j=1}^\infty \Vert G(D)v_j\Vert _{p,\Omega }&\le C\varepsilon ^{\frac{1}{p}-g}\sum _{j=1}^\infty 2^{jg}\Vert f_{j-1}\Vert _{p,\Omega }\\&= 2^g C \varepsilon ^{\frac{1}{p}-g}\bigg ( \Vert F\Vert _{p,\Omega } + \sum _{j=1}^\infty 2^{jg}\Vert f_{j}\Vert _{p,\Omega } \bigg )\\&\le 2^g C \varepsilon ^{\frac{1}{p}-g}\bigg ( \Vert F\Vert _{p,\Omega } + \sum _{j=1}^\infty 2^{jg}\Vert f_{j}\Vert _{\infty ,\Omega }\, {\mathcal L}^n(\Omega )^{\frac{1}{p}} \bigg ) \end{aligned} \end{aligned}$$

for all $p\in [1,+\infty )$, where

$$\begin{aligned} \Vert f_{j}\Vert _{\infty ,\Omega }\, {\mathcal L}^n(\Omega )^{\frac{1}{p}} \le (2^{-j (g+1)} s^{-1}) (s \Vert F\Vert _{p,\Omega }) = 2^{-j (g+1)} \Vert F\Vert _{p,\Omega } \end{aligned}$$

by (ii) and (iv). Thus

$$\begin{aligned} \sum _{j=1}^\infty \Vert G(D)v_j\Vert _{p,\Omega } \le C\, 2^{g+1} \varepsilon ^{\frac{1}{p}-g} \Vert F\Vert _{p,\Omega }, \text { for all } p\in [1,+\infty ) \end{aligned}$$

(4.13)

and hence (cf. [1, Theorem 2.8])

$$\begin{aligned} \sum _{j=1}^\infty \Vert G(D)v_j\Vert _{\infty ,\Omega } \le C\, 2^{g+1} \varepsilon ^{-g} \Vert F\Vert _{\infty ,\Omega }, \end{aligned}$$

that is the series $\sum _{j=1}^\infty G(D)v_j$ converges totally in $L^\infty (\Omega ,{\mathbb {C}}^N)$. If define $u_H:=\sum _{j=1}^Hv_j\in C_c^\infty (\Omega , {\mathbb {C}})$ (for $H=1,2,\ldots$), then:

• There exists $\Phi =(\Phi _1,\ldots ,\Phi _N)^t \in C_0(\Omega ,{\mathbb {C}}^N)\cap L^\infty (\Omega , {\mathbb {C}}^N)$ such that

  $$\begin{aligned} \lim _{H\rightarrow \infty } \Vert G(D)u_H -\Phi \Vert _{\infty ,\Omega }=0; \end{aligned}$$

  (4.14)

• By assumption (4.9) and Poincaré’s inequality (cf. Theorem 3 of [11, Sect. 5.6]), we find that $f\in C_0^m(\Omega ,{\mathbb {C}})$ has to exist such that

  $$\begin{aligned} \lim _{H\rightarrow \infty } \Vert u_H - f\Vert _{C^m(\Omega ,{\mathbb {C}})}=0. \end{aligned}$$

  (4.15)

Now, recalling (iv) above, one can easily prove by induction on k that if $x\in \cap _{j=1}^\infty K_j$ and $H\ge 1$ then the following identity

$$\begin{aligned} F(x)-[G(D)u_H](x) =f_k(x)-\sum _{j=k+1}^H [G(D)v_j](x) \end{aligned}$$

holds for all $k=0,\ldots , H-1$. Hence, recalling also (4.12) and (ii) above, we obtain

$$\begin{aligned} \begin{aligned} \Vert F-G(D)u_H\Vert _{\infty ,\Omega {\setminus }{\mathcal {O}}}&=\Vert F-G(D)u_H\Vert _{\infty , \cap _j K_j}\\&=\Vert f_{H-1} - G(D)v_H\Vert _{\infty , \cap _j K_j}\\&\le 2^{-H (g+1)}s^{-1} \end{aligned} \end{aligned}$$

(4.16)

for all positive integers H. From (4.14), (4.16) and the inequality

$$\begin{aligned} \begin{aligned} \Vert F-\Phi \Vert _{\infty ,\Omega {\setminus }{\mathcal {O}}}&\le \Vert F-G(D)u_H\Vert _{\infty ,\Omega {\setminus }{\mathcal {O}}} + \Vert G(D)u_H-\Phi \Vert _{\infty ,\Omega {\setminus }{\mathcal {O}}}, \end{aligned} \end{aligned}$$

we get assertion (2).

By (4.14), (4.15), recalling the regularity identity (2.5) and the continuity property (2.6) for distributions, we obtain

$$\begin{aligned} G_j(D)T_f=\lim _{H\rightarrow \infty }G_j(D)T_{u_H} = \lim _{H\rightarrow \infty } T_{G_j(D)u_H}= T_{\Phi _j}\quad (j=1,\ldots , N) \end{aligned}$$

which proves (3). In particular, if $m=g$ then one has $G(D)f=\Phi$ in $\Omega$. Moreover, from (4.15) and (4.13), we get at once

$$\begin{aligned} \Vert G(D)f\Vert _{p,\Omega } =\lim _{H\rightarrow \infty } \Vert G(D)u_H\Vert _{p,\Omega } \le C\, 2^{g+1} \varepsilon ^{\frac{1}{p}-g} \Vert F\Vert _{p,\Omega }, \end{aligned}$$

(4.17)

for all $p\in [1,+\infty )$.

Step 2: If F is a Borel function.

Let $\varepsilon >0$ be fixed arbitrarily. Then, proceeding as in the proof of Theorem 1 in [2], we can find $F_1\in C(\Omega , {\mathbb {C}}^N)\cap L^\infty (\Omega , {\mathbb {C}}^N)$ and an open set ${\mathcal {O}}_1\subset \Omega$ satisfying

$$\begin{aligned} {\mathcal L}^n ({\mathcal {O}}_1)\le \frac{\varepsilon }{2}\,{\mathcal L}^n (\Omega ),\quad F_1\vert _{\Omega \setminus {\mathcal {O}}_1}= F\vert _{\Omega \setminus {\mathcal {O}}_1} \end{aligned}$$

and

$$\begin{aligned} \Vert F_1\Vert _{p,\Omega } \le 2\Vert F \Vert _{p,\Omega }, \text { for all }p\in [1,+\infty ]. \end{aligned}$$

(4.18)

By Step 1 we obtain another open set ${\mathcal {O}}_2\subset \Omega$, $f\in C_0^m(\Omega , {\mathbb {C}})$ and $\Phi \in C_0(\Omega , {\mathbb {C}}^N)\cap L^\infty (\Omega , {\mathbb {C}}^N)$ such that

• ${\mathcal L}^n ({\mathcal {O}}_2)\le \varepsilon /2\, {\mathcal L}^n (\Omega )$;

• $\Phi =F_1$ a.e. in $\Omega {\setminus }{\mathcal {O}}_2$;

• The equality $G(D)f=\Phi$ holds in the sense of distributions;

• In the special case $m=g$ one has $G(D)f=\Phi$ in $\Omega$, hence $G(D)f=F_1$ a.e. in $\Omega {\setminus }{\mathcal {O}}_2$. Moreover, by (4.17), one has

  $$\begin{aligned} \Vert G(D)f\Vert _{p,\Omega } \le C \, 2^{g+1} \varepsilon ^{\frac{1}{p}-g} \Vert F_1\Vert _{p,\Omega }, \text { for all }p\in [1,+\infty ). \end{aligned}$$

  (4.19)

  Letting p tend to $+\infty$ in (4.19), we also find

  $$\begin{aligned} \Vert G(D)f\Vert _{\infty ,\Omega } \le C \, 2^{g+1} \varepsilon ^{-g} \Vert F_1\Vert _{\infty ,\Omega } \end{aligned}$$

  (4.20)

  cf. [1, Theorem 2.8].

Now (4.10) and (4.11) follow from (4.19) and (4.20), respectively, by recalling (4.18). The conclusion follows by setting ${\mathcal {O}}:= {\mathcal {O}}_1\cup {\mathcal {O}}_2$. $\square$

The following remark is a very slight variant of Remark 4.3 in [10], we state it for the reader’s convenience.

Remark 4.3

The conclusions of Theorem 4.1 do not extend to families of polynomials $G_1,\ldots , G_N$ in which there are repeated elements (compare Remark 4.1). To prove it, let’s assume that there is a repetition, namely $G_r=G_s$ with $r\not = s$, and consider any $F=(F_1,\ldots , F_N)^t$ such that $F_r\equiv 0$ and $F_s\equiv 1$. Then at least one of statements (1),(2),(3) of Theorem 4.1 must fail to be true. Indeed (3) yields $T_{\Phi _r}=T_{\Phi _s}$, hence $\Phi _r=\Phi _s$ a.e. in $\Omega$. Then $1=0$ a.e. in $\Omega {\setminus }{\mathcal {O}}$, by (2). But this implies ${\mathcal L}^n({\mathcal {O}})={\mathcal L}^n(\Omega )$, which contradicts (1).

From Theorem 4.1 we get immediately the following property.

Corollary 4.1

Let $G_1,\ldots , G_N\in {\mathbb {C}}[\xi _1,\ldots , \xi _n]$ and $\Omega \subset {\mathbb {R}}^n$ satisfy the same hypotheses as in Lemma 4.1. Moreover assume that there exists a constant $c_*>0$ such that

$$\begin{aligned} \Vert G(D)\varphi \Vert _{\infty ,\Omega } \ge c_* \mathop {\max }\limits _{\begin{array}{c} \alpha \in {\mathbb {N}}^n\\ \vert \alpha \vert = g \end{array}}\Vert \partial ^{\alpha }\varphi \Vert _{\infty ,\Omega } \end{aligned}$$

for all $\varphi \in C_c^\infty (\Omega ,{\mathbb {C}})$. Then, for every $F\in L_{\text {loc}}^1 (\Omega , {\mathbb {C}}^N)$, one has

$$\begin{aligned} \sup _{f\in C_0^g(\Omega , {\mathbb {C}})}{\mathcal L}^n(A_{f,F}) ={\mathcal L}^n (\Omega ). \end{aligned}$$

Remark 4.4

Corollary 4.1 states that, under suitable assumptions, there are G-primitivity domains of F arbitrarily close in measure to $\Omega$, even if $F\in W_{\text {loc}}^{m,1}(\Omega , {\mathbb {C}}^N)$ and ${\mathcal L}^n(\Upsilon _{F}^{m})>0$ (even if $\Upsilon _{F}^{m}=\Omega$, which is the least favorable case for the “G-integrability of F”!).

5 Examples of Application

In this section we apply the theory developed above to three contexts already considered in [10, Section 5], where we dealt with the case of smooth functions. Some basic facts established in [10], including presentations of contexts, will also be useful here and will therefore be recalled for the convenience of the reader.

5.1 Alberti’s Theorem

Given a positive integer k, let ${{\mathcal T}}_k$ denote the set of n-tuples $\alpha \in {\mathbb {N}}^n$ such that $\vert \alpha \vert =k$ and set $N_k:=\#{\mathcal T}_k$. Moreover let $j\mapsto \alpha ^{(j)}$ be an arbitrarily chosen bijection from $\{1,\ldots ,N_k \}$ to ${{\mathcal T}}_k$. Then, by the same arguments as in Section 5 of [10] with Theorem 4.1 in place of [10, Theorem 4.1], we obtain the following well known result (cf. [2, 14, 16]).

Corollary 5.1

Let $\Omega$ be an open subset of ${\mathbb {R}}^n$ with finite measure and k be a positive integer. Then, for every Borel function $F:\Omega \rightarrow {\mathbb {R}}^{N_k}$ and for every $\varepsilon \in (0,1)$, there exist an open set ${\mathcal {O}}\subset \Omega$ and $f\in C_0^k(\Omega )$ with the following properties (let $f^{(k)}:=(\partial ^{\alpha ^{(1)}} f,\ldots , \partial ^{\alpha ^{(N_k)}} f)^t$):

• (1) ${\mathcal L}^n ({\mathcal {O}})\le \varepsilon {\mathcal L}^n (\Omega )$;

• (2) $f^{(k)}=F$ a.e. in $\Omega {\setminus }{\mathcal {O}}$;

• (3) There exists a constant C not depending on $F, \varepsilon , p$ such that

  $$\begin{aligned} \vert \,\vert f^{(k)}\vert \,\vert _{p, \Omega } \le C \, 2^{k+2}\varepsilon ^{\frac{1}{p} - k} \vert \,\vert F \vert \,\vert _{p, \Omega }, \mathrm{for all }p\in [1,+\infty ) \end{aligned}$$

  and

  $$\begin{aligned} \vert \,\vert f^{(k)}\vert \,\vert _{\infty , \Omega } \le C \, 2^{k+2}\varepsilon ^{- k} \vert \,\vert F \vert \,\vert _{\infty , \Omega }. \end{aligned}$$

5.2 Maxwell Type System

Let us recall that the electromagnetic field is characterized by the system

$$\begin{aligned} {\left\{ \begin{array}{ll} \nabla \cdot {\mathbf {E}} = \rho \\ \nabla \cdot {\mathbf {B}} =0\\ \nabla \times {\mathbf {E}}+\partial _t {\mathbf {B}} = 0\\ \nabla \times {\mathbf {B}}-\partial _t {\mathbf {E}} = {\mathbf {j}} \end{array}\right. } \end{aligned}$$

where ${\mathbf {E}}, {\mathbf {B}}, \rho$ and ${\mathbf {j}}$ are the electric field, the magnetic field, the electric charge density and the electric current density, respectively. The symbol of this system is the following matrix of polynomials in ${\mathbb {C}}[\xi _1,\xi _2,\xi _3,\xi _4]$

$$\begin{aligned} G(\xi _1, \xi _2, \xi _3,\xi _4) =[G_{jl}(\xi _1, \xi _2, \xi _3,\xi _4)]= \left[ \begin{array}{cccccc} i\xi _1 &{} i\xi _2 &{} i\xi _3 &{} 0 &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} i\xi _1 &{} i\xi _2 &{} i\xi _3 \\ 0 &{} -i\xi _3 &{} i\xi _2 &{} i\xi _4 &{} 0 &{} 0 \\ i\xi _3 &{} 0 &{} -i\xi _1 &{} 0 &{} i\xi _4 &{} 0 \\ -i\xi _2 &{} i\xi _1 &{} 0 &{} 0 &{} 0 &{} i\xi _4 \\ -i\xi _4 &{} 0 &{} 0 &{} 0 &{} -i\xi _3 &{} i\xi _2 \\ 0 &{} -i\xi _4 &{} 0 &{} i\xi _3 &{} 0 &{} -i\xi _1 \\ 0 &{} 0 &{} -i\xi _4 &{} -i\xi _2 &{} i\xi _1 &{} 0 \end{array}\right] , \end{aligned}$$

where $\xi _1, \xi _2, \xi _3$ are the symbols of the spatial differential operators $-i\partial _{x_1}, -i\partial _{x_2}, -i\partial _{x_3}$, while $\xi _4$ is the symbol of the time differential operator $-i\partial _{x_4}$ (for consistency with the notation introduced in the previous sections, we denote the time variable with $x_4$). In this case, a remarkable example of matrix in $\Sigma _1$ is the one associated to the first syzygies (cf. [5, Section 5.1])

$$\begin{aligned} \underline{S}(\xi _1, \xi _2, \xi _3,\xi _4) = [\underline{S}_{jl}(\xi _1, \xi _2, \xi _3,\xi _4)]= \left[ \begin{array}{cccccccc} 0 &{} i\xi _4 &{} i\xi _1 &{} i\xi _2 &{} i\xi _3 &{} 0 &{} 0 &{} 0 \\ i\xi _4 &{} 0 &{} 0 &{} 0 &{} 0 &{} i\xi _1 &{} i\xi _2 &{} i\xi _3 \end{array}\right] . \end{aligned}$$

Let us observe that $\deg G = \deg \underline{S} =1$.

Corollary 5.2

Let $\Omega$ be an open subset of ${\mathbb {R}}^4$ with finite measure. Then, for every Borel function $F=(F_1,\ldots ,F_8)^t: \Omega \rightarrow {\mathbb {C}}^8$ and for every $\varepsilon \in (0,1)$, there exist an open set ${\mathcal {O}}\subset \Omega$ and $f\in C_0^1(\Omega , {\mathbb {C}}^6)$ with the following properties:

• (1) ${\mathcal L}^4 ({\mathcal {O}})\le \varepsilon {\mathcal L}^4 (\Omega )$;

• (2) $G(D) f=F$ a.e. in $\Omega \setminus {\mathcal {O}}$;

• (3) In the special case when $F\in W^{l,p}_{\text {loc}}(\Omega , {\mathbb {C}}^8)$ with $l\ge 1$ and $p\in (1,+\infty )$, one has

  $$\begin{aligned} {\mathcal L}^n \left( A_{f,F}^{(n+pl/(p-1))}\cap \Upsilon _F^l\right) =0. \end{aligned}$$

  Hence, in particular,

  $$\begin{aligned} {\mathcal L}^n \left( A_{f,F}^{(n+p/(p-1))}\cap \Upsilon _{F,\underline{S}}\right) =0 \end{aligned}$$

  that is

  $$\begin{aligned} {\left\{ \begin{array}{ll} \partial _t F_2 + \nabla _x\cdot (F_3,F_4,F_5) =0 \\ \partial _t F_1 + \nabla _x\cdot (F_6,F_7,F_8)=0 \end{array}\right. } \end{aligned}$$

  almost everywhere in $\Omega \cap A_{f,F}^{(n+p/(p-1))}$.

Moreover:

• (4) Let $F\in W^{l+d, p}({\mathbb {R}}^4, {\mathbb {C}}^8)$ and $g\in W^{l+1+d,p} ({\mathbb {R}}^4,{\mathbb {C}}^6)$, with $l\ge 1$, $p\in (1,+\infty )$ and $d\in \{ 0,1\}$. The following facts hold:

  • If $d=0$ then ${\mathcal L}^4(A_{g,F}\cap \Upsilon _F^l)=0$, hence ${\mathcal L}^4(A_{g,F}\cap \Upsilon _{F,\underline{S}})=0$;

  • If $d=1$ and $p<4$ then $A_{g,F}\cap \Upsilon _F^l$ is 3-rectifiable, hence $A_{g,F}\cap \Upsilon _{F,\underline{S}}$ is 3-rectifiable.

Proof

As we observed in the proof of [10, Corollary 5.2], both matrices

$$\begin{aligned} H:=(H_1,H_2,H_3,H_4)^t:=(G_{11}, G_{41}, G_{51}, G_{61})^t \end{aligned}$$

and

$$\begin{aligned} K:=(K_1,K_2,K_3,K_4)^t:=(G_{24}, G_{34}, G_{74}, G_{84})^t. \end{aligned}$$

verify the assumptions of Lemma 4.1 and satisfy condition (4.9) with $m=1$. Hence, by Theorem 4.1, there exist two open sets ${\mathcal {O}}_1, {\mathcal {O}}_2\subset \Omega$ and $f_1, f_4\in C_0^1(\Omega , {\mathbb {C}})$ such that

$$\begin{aligned} {\mathcal L}^4({\mathcal {O}}_1)\le \frac{\varepsilon }{2}\,{\mathcal L}^4(\Omega ), \quad H(D)f_1 = (F_1,F_4,F_5,F_6)^t \text { a.e. in }\Omega {\setminus }{\mathcal {O}}_1 \end{aligned}$$

and

$$\begin{aligned} {\mathcal L}^4({\mathcal {O}}_2)\le \frac{\varepsilon }{2}\, {\mathcal L}^4(\Omega ), \quad K(D)f_4 = (F_2,F_3,F_7,F_8)^t \text { a.e. in }\Omega {\setminus }{\mathcal {O}}_2. \end{aligned}$$

Statements (1) and (2) follow by setting ${\mathcal {O}}:={\mathcal {O}}_1\cup {\mathcal {O}}_2$ and $f:=(f_1,0,0,f_4,0,0)^t$. As for (3), it follows immediately from Corollary 3.5. Finally, we obtain (4) from Corollary 3.2. $\square$

5.3 Multivariable Cauchy-Riemann system

Let $G_1,\ldots , G_N\in {\mathbb {C}}[\xi _1,\ldots ,\xi _{2N}]$ be defined as

$$\begin{aligned} G_j(\xi _1,\ldots ,\xi _{2N}):=\frac{i}{2}\, \xi _{2j-1} - \frac{1}{2}\, \xi _{2j} \qquad (j=1,\ldots ,N). \end{aligned}$$

Then $G=(G_1,\ldots ,G_N)^t$ is the symbol of the Cauchy-Riemann system in N complex variables $z_j=x_{2j-1} + i x_{2j}$ ($j=1,\ldots , N$), namely

$$\begin{aligned} G(D)= \begin{pmatrix} \frac{\partial }{\partial \bar{z}_1}\\ \vdots \\ \frac{\partial }{\partial \bar{z}_N} \end{pmatrix} = \begin{pmatrix} \frac{1}{2}\,\partial _1 +\frac{i}{2}\,\partial _2\\ \vdots \\ \frac{1}{2}\,\partial _{2N-1} +\frac{i}{2}\,\partial _{2N} \end{pmatrix}. \end{aligned}$$

Observe that $\deg G = 1$. Analogously as we have done for the Maxwell type system, we can consider the matrix associated to the first syzygies, namely the one of dimension $\frac{N(N-1)}{2}\times N$ used in the proof of Corollary 3.3. Also in this case we denote such a matrix by $\underline{S}$ and observe that $\deg \underline{S}=1$.

Corollary 5.3

Let $\Omega$ be an open subset of ${\mathbb {R}}^{2N}$ with finite measure. Then, for every Borel function $F=(F_1,\ldots , F_N)^t: \Omega \rightarrow {\mathbb {C}}^N$ and for every $\varepsilon \in (0,1)$, there exist an open set ${\mathcal {O}}\subset \Omega$, $f\in C_0(\Omega , {\mathbb {C}})$ and $\Phi \in C_0(\Omega , {\mathbb {C}}^N)\cap L^\infty (\Omega , {\mathbb {C}}^N)$ such that:

• (1) ${\mathcal L}^{2N}({\mathcal {O}})\le \varepsilon {\mathcal L}^{2N}(\Omega )$;

• (2) $\Phi =F$ a.e. in $\Omega {\setminus }{\mathcal {O}}$;

• (3) The equality $G(D)f=\Phi$ holds in the sense of distributions;

• (4) In the special case when $F\in W_{\text {loc}}^{l,p}(\Omega , {\mathbb {C}}^N)$ with $l\ge 1$ and $p\in (1,+\infty )$, there exists a null measure set $Z\subset \Omega$ such that

  $$\begin{aligned} S(D)F=0 \text { in }\Omega \cap B_{\Phi , F}^{(2N+\delta _S)}\setminus Z \end{aligned}$$

  for all $S\in \Sigma _l$ (where $\delta _S=p\deg S/(p-1)$). In particular one has $\underline{S}(D)F=0$ in $\Omega \cap B_{\Phi , F}^{(2N+\delta _{\underline{S}})}{\setminus }Z$, that is

  $$\begin{aligned} \frac{\partial F_k}{\partial \bar{z}_j} = \frac{\partial F_j}{\partial \bar{z}_k} \text { in }\Omega \cap B_{\Phi , F}^{(2N+p/(p-1))}\setminus Z \end{aligned}$$

  for all $j,k\in \{ 1,\ldots , N\}$.

Moreover:

• (5) Let $F\in W_{\text {loc}}^{l,p}(\Omega , {\mathbb {C}}^N)$ and $g\in W_{\text {loc}}^{1,p}(\Omega , {\mathbb {C}})$, with $l\ge 1$ and $p\in (1,+\infty )$. Then one has

  $$\begin{aligned} {\mathcal L}^{2N} \left( A_{g, F}^{(2N+pl/(p-1))}\cap \Upsilon _F^l \right) =0. \end{aligned}$$

  Hence, in particular,

  $$\begin{aligned} {\mathcal L}^{2N} \left( A_{g, F}^{(2N+p/(p-1))}\cap \Upsilon _{F, \underline{S}} \right) =0 \end{aligned}$$

  that is

  $$\begin{aligned} \frac{\partial F_k}{\partial \bar{z}_j} = \frac{\partial F_j}{\partial \bar{z}_k} \text { a.e. in } \Omega \cap A_{g, F}^{(2N+p/(p-1))} \end{aligned}$$

  for all $j,k\in \{ 1,\ldots , N\}$.

• (6) Let $F\in W^{l+d,p}({\mathbb {R}}^{2N}, {\mathbb {C}}^N)$ and $g\in W^{l+1+d,p}({\mathbb {R}}^{2N}, {\mathbb {C}})$, with $l\ge 1$, $p\in (1,+\infty )$ and $d\in \{0,1\}$. The following facts hold:

  • If $d=0$ then ${\mathcal L}^{2N}(A_{g,F}\cap \Upsilon _F^l)=0$, hence ${\mathcal L}^{2N}(A_{g,F}\cap \Upsilon _{F,\underline{S}})=0$;

  • If $d=1$ and $p<2N$ then $A_{g,F}\cap \Upsilon _F^l$ is $(2N-1)$-rectifiable, hence $A_{g,F}\cap \Upsilon _{F,\underline{S}}$ is $(2N-1)$-rectifiable.

Proof

Recall from the proof of [10, Corollary 5.3] that $G_1,\ldots ,G_N$ verify the hypotheses of Lemma 4.1 and (4.9) holds with $m=0$. Then (1), (2) and (3) follow at once by Theorem 4.1. Assertions (4) and (5) follow from Theorem 3.2 and Corollary 3.5, respectively. Finally, we get (6) by Corollary 3.2. $\square$