Skip to main content
SpringerLink
Log in

Quasiperiodic Route to Transient Chaos in Vibroimpact System

  • Chapter
  • First Online:
Nonlinear Dynamics, Chaos, and Complexity

Part of the book series: Nonlinear Physical Science ((NPS))

Abstract

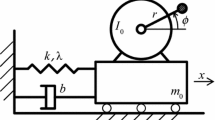
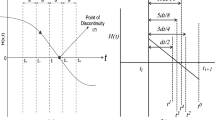
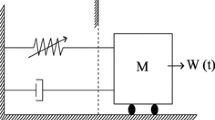
Dynamic behaviour of the nonsmooth systems is interesting and explored subject in nonlinear science. We studied quasiperiodic route to chaos in nonlinear nonsmooth discontinuous vibroimpact system. In narrow frequency range different oscillatory regimes have succeeded each other many times under very small control parameter varying. There were periodic subharmonic regimes—chatters, quasiperiodic, and chaotic regimes. There were the zones of transition from one regime to another, the zones of prechaotic or postchaotic motion. The hysteresis effects (jump phenomena) occurred for increasing and decreasing frequencies. The chaoticity of obtained regime has been confirmed by typical views of Poincaré map and Fourier spectrum, by the positive value of the largest Lyapunov exponent, and by the fractal structure of Poincaré map. Discontinuous bifurcations are also described—it is phenomenon unique for nonsmooth systems. It is discussed the largest Lyapunov estimation for nonsmooth vibroimpact system.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution
eBook
USD 16.99
Price excludes VAT (USA)
  • Available as EPUB and PDF
  • Read on any device
  • Instant download
  • Own it forever
Softcover Book
USD 99.99
Price excludes VAT (USA)
  • Compact, lightweight edition
  • Dispatched in 3 to 5 business days
  • Free shipping worldwide - see info
Hardcover Book
USD 99.99
Price excludes VAT (USA)
  • Durable hardcover edition
  • Dispatched in 3 to 5 business days
  • Free shipping worldwide - see info

Tax calculation will be finalised at checkout

Purchases are for personal use only

Institutional subscriptions

Similar content being viewed by others

Notes

  1. 1.

    We consider hysteresis effect as dependence of the system state on its history (the system manifests hysteretic features in the transition between different types of motion) [12, 16].

  2. 2.

    The mark (n,k) means nT-periodic vibration with k impacts per cycle [32], T is period of external loading \(T=2\pi /\omega \).

References

  1. Luo AC, Guo Y (2012) Vibro-impact dynamics. Wiley

    Google Scholar 

  2. Leine RI, Van Campen DH (2002) Discontinuous bifurcations of periodic solutions. Math Comput Model 36(3):259–273

    Article  MathSciNet  Google Scholar 

  3. Seydel R (2009) Practical bifurcation and stability analysis, vol 5. Springer Science & Business Media

    Google Scholar 

  4. Ivanov AP (2012) Analysis of discontinuous bifurcations in nonsmooth dynamical systems. Regul Chaotic Dyn 17(3–4):293–306

    Article  MathSciNet  Google Scholar 

  5. Leine RI, Van Campen DH, Van de Vrande BL (2000) Bifurcations in nonlinear discontinuous systems. Nonlinear Dyn 23(2):105–164

    Article  MathSciNet  Google Scholar 

  6. Di Bernardo M, Budd CJ, Champneys AR, Kowalczyk P, Nordmark AB, Tost GO, Piiroinen PT (2008) Bifurcations in nonsmooth dynamical systems. SIAM Rev 50(4):629–701

    Article  MathSciNet  Google Scholar 

  7. Brogliato B (ed) (2000) Impacts in mechanical systems: analysis and modelling, vol 551. Springer Science & Business Media

    Google Scholar 

  8. Alzate R (2008) Analysis and application of bifurcations in systems with impacts and chattering. Doctoral dissertation, Universita degli Studi di Napoli Federico II

    Google Scholar 

  9. Cheng J, Xu H (2006) Nonlinear dynamic characteristics of a vibro-impact system under harmonic excitation. J Mech Mater Struct 1(2):239–258

    Article  MathSciNet  Google Scholar 

  10. Volchenkov D, Leoncini X (eds) (2018) Regularity and stochasticity of nonlinear dynamical systems. Springer International Publishing

    Google Scholar 

  11. Kuznetsov SP (2001) Dynamical chaos. Fizmatlit, Moscow (2006), 356 pp

    Google Scholar 

  12. Moon FC (1987) Chaotic vibrations: an introduction for applied scientists and engineers. Research supported by NSF, USAF, US Navy, US Army, and IBM. Wiley-Interscience, New York, 322 pp

    Google Scholar 

  13. Luo AC (2016) Periodic flows to chaos in time-delay systems, vol 16. Springer

    Google Scholar 

  14. Afraimovich V, Machado JAT, Zhang J (eds) (2016) Complex motions and chaos in nonlinear systems. Springer International Publishing

    Google Scholar 

  15. Shvets AY, Sirenko VA (2015) New ways of transition to deterministic chaos in nonideal oscillating systems. Res Bull Natl Tech Univ Ukr “Kyiv Polytech Inst” 1(99):45–51

    Google Scholar 

  16. Serweta W, Okolewski A, Blazejczyk-Okolewska B, Czolczynski K, Kapitaniak T (2014) Lyapunov exponents of impact oscillators with Hertz’s and Newton’s contact models. Int J Mech Sci 89:194–206

    Article  Google Scholar 

  17. Manneville P, Pomeau Y (1980) Different ways to turbulence in dissipative dynamical systems. Phys D: Nonlinear Phenomena 1(2):219–226

    Article  MathSciNet  Google Scholar 

  18. Benettin G, Galgani L, Giorgilli A, Strelcyn JM (1980) Lyapunov characteristic exponents for smooth dynamical systems and for Hamiltonian systems; a method for computing all of them. Part 1: theory. Meccanica 15(1):9–20

    Google Scholar 

  19. Muller PC (1995) Calculation of Lyapunov exponents for dynamic systems with discontinuities. Chaos Solitons Fractals 5(9):1671–1681

    Article  MathSciNet  Google Scholar 

  20. Stefanski A, Dabrowski A, Kapitaniak T (2005) Evaluation of the largest Lyapunov exponent in dynamical systems with time delay. Chaos Solitons Fractals 23(5):1651–1659

    Article  MathSciNet  Google Scholar 

  21. De Souza SL, Caldas IL (2004) Calculation of Lyapunov exponents in systems with impacts. Chaos Solitons Fractals 19(3):569–579

    Article  Google Scholar 

  22. Ageno A, Sinopoli A (2005) Lyapunov’s exponents for nonsmooth dynamics with impacts: stability analysis of the rocking block. Int J Bifurc Chaos 15(06):2015–2039

    Article  MathSciNet  Google Scholar 

  23. Andreaus U, Placidi L, Rega G (2010) Numerical simulation of the soft contact dynamics of an impacting bilinear oscillator. Commun Nonlinear Sci Numer Simul 15(9):2603–2616

    Article  MathSciNet  Google Scholar 

  24. Komuro M, Kamiyama K, Endo T, Aihara K (2016) Quasi-periodic bifurcations of higher-dimensional tori. Int J Bifurc Chaos 26(07):1630016

    Article  MathSciNet  Google Scholar 

  25. Afraimovich VS, Shilnikov LP (1991) Invariant two-dimensional tori, their breakdown and stochasticity. Am Math Soc Transl 149(2):201–212

    Article  Google Scholar 

  26. Shilnikov A, Shilnikov L, Turaev D (2004) On some mathematical topics in classical synchronization: a tutorial. Int J Bifurc Chaos 14(07):2143–2160

    Article  MathSciNet  Google Scholar 

  27. Bakri T (2005) Torus breakdown and chaos in a system of coupled oscillators. Int J Non-Linear Mech

    Google Scholar 

  28. Verhulst F (2016) Torus break-down and bifurcations in coupled oscillators. Procedia IUTAM 19:5–10

    Article  Google Scholar 

  29. Bazhenov VA, Lizunov PP, Pogorelova OS, Postnikova TG, Otrashevskaia VV (2015) Stability and bifurcations analysis for 2-DOF vibroimpact system by parameter continuation method. Part I: loading curve. J Appl Nonlinear Dyn 4(4):357–370

    Google Scholar 

  30. Bazhenov VA, Lizunov PP, Pogorelova OS, Postnikova TG (2016) Numerical bifurcation analysis of discontinuous 2-DOF vibroimpact system. Part 2: Frequency-amplitude response. J Appl Nonlinear Dyn 5(3):269–281

    Google Scholar 

  31. Bazhenov VA, Pogorelova OS, Postnikova TG (2017) Stability and discontinious bifurcations in vibroimpact system: numerical investigations. LAP LAMBERT Academic Publishing GmbH and Co., KG Dudweiler, Germany

    Google Scholar 

  32. Lamarque CH, Janin O (2000) Modal analysis of mechanical systems with impact non-linearities: limitations to a modal superposition. J Sound Vib 235(4):567–609

    Article  Google Scholar 

  33. Bazhenov VA, Pogorelova OS, Postnikova TG (2016) Dangerous bifurcations in 2-DOF vibroimpact system. Bull Natl Tech Univ “Kharkiv Polytech Inst” (26):109–113

    Google Scholar 

  34. Zakrzhevsky M, Schukin I, Yevstignejev V (2007) Rare attractors in driven nonlinear systems with several degrees of freedom. Transp Eng 24

    Google Scholar 

  35. Lichtenberg AJ, Lieberman MA (2013) Regular and stochastic motion, vol 38. Springer Science & Business Media

    Google Scholar 

  36. Chirikov BV, Shepelyanskii DL (1982) Dynamics of some homogeneous models of classical Yang-Mills fields. Sov J Nucl Phys 36(6):908–915

    MathSciNet  MATH  Google Scholar 

  37. Wolf A, Swift JB, Swinney HL, Vastano JA (1985) Determining Lyapunov exponents from a time series. Phys D: Nonlinear Phenom 16(3):285–317

    Article  MathSciNet  Google Scholar 

  38. Bazhenov VA, Pogorelova OS, Postnikova TG (2018) Lyapunov exponents estimation for strongly nonlinear nonsmooth discontinuous vibroimpact system. In: Strength of materials and theory of structures (99) (in press)

    Google Scholar 

  39. Lai YC, Tél T (2011) Transient chaos: complex dynamics on finite time scales, vol 173. Springer Science & Business Media

    Google Scholar 

  40. Afraimovich VS, Neiman AB (2017) Weak transient chaos. Advances in dynamics, patterns, cognition. Springer, Cham, pp 3–12

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Kyiv National University of Construction and Architecture, 31, Povitroflotskiy avenu, Kyiv, Ukraine

    Victor Bazhenov, Olga Pogorelova & Tatiana Postnikova

Authors
  1. Victor Bazhenov
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Olga Pogorelova
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Tatiana Postnikova
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Victor Bazhenov .

Editor information

Editors and Affiliations

  1. Department of Mathematics and Statistics, Texas Tech University, Lubbock, TX, USA

    Dimitri Volchenkov

Rights and permissions

Reprints and permissions

Copyright information

© 2021 Higher Education Press

About this chapter

Check for updates. Verify currency and authenticity via CrossMark

Cite this chapter

Bazhenov, V., Pogorelova, O., Postnikova, T. (2021). Quasiperiodic Route to Transient Chaos in Vibroimpact System. In: Volchenkov, D. (eds) Nonlinear Dynamics, Chaos, and Complexity. Nonlinear Physical Science. Springer, Singapore. https://doi.org/10.1007/978-981-15-9034-4_3

Download citation

  • DOI: https://doi.org/10.1007/978-981-15-9034-4_3

  • Published:

  • Publisher Name: Springer, Singapore

  • Print ISBN: 978-981-15-9033-7

  • Online ISBN: 978-981-15-9034-4

  • eBook Packages: Physics and AstronomyPhysics and Astronomy (R0)

Publish with us

Policies and ethics

Search

Navigation