Singular Temperature Dependence of the Equation of State of Superconductors with Spin-Orbit Interaction in Low-Temperature Region – II

Abstract

We extend a previous study of the space and temperature dependence of the order parameter in an ultrathin superconducting film in a longitudinal magnetic field to the vicinity of the critical transition surface. In these films, the spin-orbit interaction can be sizeable. The calculations are restricted to the low-temperature regime. Special attention is given to the zero temperature limit where the state with the maximum value of the critical field is investigated for the given strength of the spin-orbit interaction.

1 Introduction

Very thin film deposited on a substrate can be in a superconducting state [1, 2], even in very strong longitudinal magnetic fields and with strong spin-orbit interactions. In paper [1], single atomic layer films of Pb on Si(111) substrate have been studied. Superconducting transition temperature was 1.83 K. The upper critical field was estimated to be 1450 G. The experimental data for order parameter Δ are in a good agreement with the BCS theory. In the paper [2], the superconductivity with Tc above 100K in the FeSe on the substrate SrTiO₃ was estimated. The temperature dependence of critical current J_c was fitted to the Ginzburg-Landau functional. It was found that J_c decreased with increasing magnetic field up to 11T at 3K.

The order parameter Δ in such a system is the function of three parameters: magnetic field, spin-orbit interaction, temperature. Equation Δ = 0 creates surface in this space: critical transition surface. The critical transition surface on the subspace spanned by magnetic field, spin-orbit interac- tion, and temperature was investigated in a low-temperature region in papers [3, 4]. The point T = 0 is a singular point for such a system. It is expected that near the transition surface the behavior of the order parameter also will be nontri- vial and will attract special interest for experimental investigations. In this paper, we present the results of theoretical investigation of the space and temperature dependence of the order parameter in the vicinity of critical transition surface. Note that critical values of the longitudinal magnetic field and spin-orbit interaction in the inhomogeneous state are nearly twice as large as the same values are for homogeneous state. We investigate the state starting from the state with the maximal value of the critical magnetic field for fixed value of the spin-orbit interaction at T = 0.

2 Equation System for the Superconducting Order Parameter

For a thin superconducting film, deposited on a substrate, the spin-orbit interaction can be taken in the form [5, 6]

$$ \hat{V}_{\text{SO}} = \frac{-i \hbar^{2}}{2m^{2}c^{2}}~\left( \left[ \frac{\partial U}{\partial \mathbf{r}} \times \frac{\partial}{\partial \mathbf{r}}\right] \hat{\mathbf{S}} \right) $$

(1)

where $\hat {\mathbf {S}}$ is the spin operator of an electron, m is the electron mass, c is the speed of light, and U is the self-consistent potential. In normal metals, the Green’s function is a 2 × 2 matrix and satisfied the equation

$$ \left( \frac{\partial}{\partial \tau} + \hat{L} \right) \hat{G} = \delta (\mathbf{r} - \mathbf{r}^{\prime}) \delta (\tau - \tau^{\prime}) $$

(2)

where τ is imaginary time and the operator $\hat {L}$ is

$$ \hat{L} = - \frac{\hbar^{2}}{2m}~ \frac{\partial^{2}}{\partial \mathbf{r}^{2}} + \hat{V}_{\text{SO}} - \mu - \frac{e \hbar}{mc} (\mathbf{HS})~~. $$

(3)

The last term is the Zeeman energy, μ is the chemical potential, and H is an in-plane external magnetic field. The eigenfunctions of operator $\hat {L}$ are of the form

$$ \left( \begin{array}{c} \psi^{(n)}_{1} \\ \psi^{(n)}_{2} \end{array}\right) = \exp \left( \frac{i p_{x}}{\hbar} x + \frac{i p_{y}}{\hbar} y \right) \chi_{n} (z) \left( \begin{array}{c} f^{(n)}_{1} \\ f^{(n)}_{2} \end{array}\right) . $$

(4)

The χ_n(z) are normalized eigenfunctions of the operator

$$ \left( - \frac{\hbar^{2}}{2m} \frac{\partial^{2}}{\partial z^{2}} + U (z) \right) \chi_{n} (z) = \epsilon_{n} \chi_{n} (z),~~~n = 0,1,2, \dots $$

(5)

In the subspace with given values of p_x, p_y, and n, the vector $(f^{(n)}_{1}, f^{(n)}_{2} )$ is a solution of the equation

$$\begin{array}{@{}rcl@{}} &&\left[ -\frac{\hbar^{2}}{4m^{2} c^{2}}~ \hat{\alpha}_{n} \left( \begin{array}{cc} 0 & p_{x} + ip_{y} \\ p_{x} - ip_{y} & 0 \end{array}\right) \right.\\ &&\left.- \left( \begin{array}{cc} 0 & h_{x} - ih_{y} \\ h_{x} + ih_{y} & 0 \end{array}\right) \right] \left( f_{1}^{(n)} \atop f_{2}^{(n)} \right) = \lambda^{(n)} \left( f_{1}^{(n)} \atop f_{2}^{(n)} \right) . \end{array} $$

(6)

with

$$ \hat{\alpha}_{n} = \int\limits^{\infty}_{- \infty}dz~ \frac{\partial U}{\partial z} \left| \chi_{n} (z) \right|^{2} , h = {{\mu}}_{B} \mathbf{H} , \mu_{B} = \frac{e \hbar}{2mc} . $$

(7)

Eigenvalues λ⁽ⁿ⁾ are

$$ \lambda^{(n)}_{\pm} = \pm \left\{ \frac{(\tilde{\alpha}_{n})^{2}}{4} {v^{2}_{F}} p^{2} + h^{2} + \left( h_{x} p_{y} - h_{y} p_{x} \right) v_{F} \cdot \tilde{\alpha}_{n} \right\}^{\frac{1}{2}} $$

(8)

where

$$ \tilde{\alpha}_{n} =\hat{ \alpha}_{n} \frac{\hbar}{2m^{2} c^{2} v_{F}} . $$

(9)

and v_F is Fermi velocity.

The eigenfunctions $f^{(n)}_{\pm }$ are found to be

$$\begin{array}{@{}rcl@{}} f^{(n)}_{+} & = & \frac{1}{\sqrt{2}} \left[ 1; -\frac{1}{\lambda^{(n)}_{+}} \left( \frac{\tilde{\alpha} v_{F}}{2} (p_{y} - ip_{x}) + \left( h_{x} + ih_{y} \right) \right) \right] \\[1ex] f^{(n)}_{-} & = & \frac{1}{\sqrt{2}} \left[\! -\frac{1}{\lambda^{(n)}_{-}} \left( \frac{\tilde{\alpha} v_{F}}{2} (p_{y} + ip_{x}) + \left( h_{x} \!-\! ih_{y} \right) \right) ; 1 \right] .\\ \end{array} $$

(10)

In a normal metal, Green’s function decomposes into four blocks (see also, consideration of normal metal without spin-orbit interaction [7])

$$ \left( \begin{array}{clc} \hat{G}^{(0)}_{++} & ; & 0 \\ 0 & ; & \hat{G}^{(0)}_{--} \end{array}\right), $$

and satisfies the equation

$$\begin{array}{@{}rcl@{}} \left( \begin{array}{clc} \frac{\partial}{\partial \tau} + \hat{L} &; & 0 \\ 0 &; & \frac{\partial}{\partial \tau} - \hat{L} \end{array}\right) \left( \begin{array}{l} \hat{G}^{(0)}_{++} \\ \hat{G}^{(0)}_{--} \end{array}\right) = \delta (\mathbf{r} - \mathbf{r}^{\prime}) \delta (\tau - \tau^{\prime}). \end{array} $$

(11)

From (3), (8), (10), and (11), we obtain

$$\begin{array}{@{}rcl@{}} \hat{G}^{(0)}_{++} & = & T \sum\limits_{\omega} \sum\limits_{n} \chi_{n} (z) \chi^{*}_{n} (z^{\prime}) \\ && \times \sum\limits_{\lambda^{(n)}_{+}} \int\limits^{\infty}_{- \infty} \frac{d p_{x} dp_{y}}{(2 \pi \hbar)^{2}} \left( f (\mathbf{p})^{(n)} \left( f (\mathbf{p})^{(n)} \right)^{+} \right)_{+}\\ &&\times \frac{\exp \left( \frac{i \mathbf{p}}{\hbar} (\mathbf{r} - \mathbf{r^{\prime}}) \right) \exp \left( -i \omega (\tau - \tau^{\prime}) \right)}{-i \omega + \frac{\mathbf{p}^{2}}{2m} + \epsilon_{n} - \mu + \lambda^{(n)}_{+} (\mathbf{p})} \\ \hat{G}^{(0)}_{--} & = & T \sum\limits_{\omega} \sum\limits_{n} \chi_{n} (z) \chi^{*}_{n} (z^{\prime}) \\ && \times \sum\limits_{\lambda^{(n)}_{-}} \int\limits^{\infty}_{- \infty} \frac{d p_{x} dp_{y}}{(2 \pi \hbar)^{2}} \left( f (\mathbf{p})^{(n)} \left( f (\mathbf{p})^{(n)} \right)^{+} \right)_{-}\\ &&\times \frac{\exp \left( \frac{i \mathbf{p}}{\hbar} (\mathbf{r} - \mathbf{r^{\prime}}) \right) \exp \left( -i \omega (\tau - \tau^{\prime}) \right)}{-i \omega - \frac{\mathbf{p}^{2}}{2m} - \epsilon_{n} + \mu - \lambda^{(n)}_{-} (\mathbf{p})}~. \end{array} $$

(12)

In (12), quantity $\left (f (\mathbf {p)}^{(n)}\left (f (\mathbf {p)})^{(n)}\right )^{+}\right )_{\pm }$ is matrix 2*2 with usual matrix product. In order to have a strong enough spin-orbit interaction, the film thickness should be very small (i.e., of the order of interatomic distances). Then, the energy distance in 𝜖_n is large. This circumstance enables us to keep only the term n = 0 in the sum over n. The function (10) forms a complete basis in terms of which we can express the electron-electron interaction as

$$ H_{\text{int}} \!=\! \frac{1}{2} \int d^{3} \mathbf{r} d^{3} \mathbf{r}^{\prime} V(\mathbf{r} - \mathbf{r}^{\prime})\! \left( \psi^{+}_{\nu} (\mathbf{r})\! \left( \psi^{+}_{\mu} (\mathbf{r}^{\prime}) \psi_{\mu} (\mathbf{r}^{\prime}) \right) \psi_{\nu} (\mathbf{r}) \right) $$

(13)

with indexes ν, μ = ±. We assume below that the potential V is δ-function like: $V (\mathbf {r} - \mathbf {r}^{\prime }) = V_{0} \delta (\mathbf {r} - \mathbf {r}^{\prime })$. We consider Cooper pairing of the type $\langle \psi _{+} (\mathbf {r}) \psi _{-} (\mathbf {r}^{\prime }) \rangle$ – electrons only, i.e., Cooper pairs formed from different Fermi surfaces [7, 8].

In superconductor, Green’s function $\tilde {\hat G}$ is matrix (4 × 4) and satisfies the equation

$$\begin{array}{@{}rcl@{}} \tilde{\hat G} &=& \left( \begin{array}{clc} \hat{G}_{++} & ;& \hat{F} \\ \hat{F^{+}} & ;& \hat{G}_{--} \end{array}\right),~ \left( \begin{array}{clc} \frac{\partial}{\partial \tau} + \hat{L} &; & \hat{{\Delta}} \\ \hat{{\Delta}}^{+} &; & \frac{\partial}{\partial \tau} - \hat{L} \end{array}\right)\\ \tilde{\hat G}& =& \delta (\mathbf{r} - \mathbf{r}^{\prime}) \delta (\tau - \tau^{\prime}) \quad . \end{array} $$

(14)

The operator $\hat {{\Delta }} (\mathbf {r})$ is [3]

$$ \hat{{\Delta}} (\mathbf{r}) = {\Delta} (\mathbf{r}) \left( 1;0 \atop 0;1 \right)Tr $$

(15)

where Tr denotes taking the trace. The self-consistency equation is

$$ {\Delta} = |V_{0}| \cdot Tr \hat{F} (\mathbf{r}, \mathbf{r}, \tau = \tau^{\prime}) \quad . $$

(16)

Equation system (12), (14), (15), (16) is a complete equation system for order parameter.

3 Inhomogeneous Pairing Near Critical Transition Surface

We solve the system of equations for the order parameter Δ(r) near the critical transition surface by the perturbation theory. From (14), we obtain

$$\begin{array}{@{}rcl@{}} \hat G_{++} & = & \hat G_{++}^{(0)} + \hat G_{++}^{(0)} \hat {\Delta} \hat G_{--}^{(0)} \hat {\Delta}^{+} \hat G_{++} ; \\ \hat G_{--} &=& \hat G_{--}^{(0)} + \hat G_{--}^{(0)} \hat {\Delta}^{+} \hat G_{++}^{(0)} \hat {\Delta} \hat G_{--} \\ \hat F^{+} & = & - \hat G_{--}^{(0)} \hat {\Delta}^{+} \left[ \hat G_{++}^{(0)} + \hat G_{++}^{(0)} \hat {\Delta} \hat G_{--}^{(0)} \hat {\Delta}^{+}_{0} \hat G_{++} \right];\\ \hat F &=& - \hat G_{++}^{(0)} \hat {\Delta} \left[ \hat G_{--}^{(0)} + \hat G_{--}^{(0)} \hat {\Delta}^{+} \hat G_{++}^{(0)} \hat {\Delta} \hat G_{--} \right]~. \end{array} $$

(17)

Such perturbation procedure can be easily continued up to the infinity. The behavior of the inhomogeneous order parameter near the critical surface is singular. As a result, a partial summation of “dangerous” terms should be made in the expansion (17). To do this, we express the order parameter Δ(r) in the inhomogeneous state in the form of a Fourier series

$$ {\Delta} (r) \!=\! \alpha_{1} \cos \left( (\mathbf{Q r}) / \hbar \right) + \alpha_{2} \cos \left( 3(\mathbf{Q r}) / \hbar \right) + \alpha_{3} \cos \left( 5(\mathbf{Q r}) / \hbar \right) + \dots $$

(18)

All terms inside the fifth order perturbation theory are presented in explicit form in “Appendix”. In each order of perturbation theory (see (17)), the value of separate element is determined by the product of phase factors $\exp (i k_{j} (\mathbf {Q r}_{j})/\hbar )(k_{j}$–integer number). For the term of function $Tr \hat {F} (\mathbf {r}, \mathbf {r}, \tau = \tau ^{\prime })$, proportional to $\cos ((\mathbf {Q r}) / \hbar )$, the largest value in each order of perturbation theory arises only from correlated product with phase factor equal to $\exp \{ i \mathbf {Q} [ (\mathbf {r}_{1} - \mathbf {r}_{2}) + \dots + (\mathbf {r}_{2K-1} - \mathbf {r}_{2K})] + \mathbf {r}_{2K + 1}\}$ (or to complex conjugate). For the term of function $Tr \hat {F} (\mathbf {r}, \mathbf {r}, \tau = \tau ^{\prime })$ proportional to $\cos (3(\mathbf {Q r}) / \hbar )$, the largest value in each order of perturbation theory arises from correlated product with phase factor of type $\exp \{ i \mathbf {Q} [ r_{1} + (\mathbf {r}_{2} - \mathbf {r}_{3}) + \dots + (\mathbf {r}_{2K-2} - \mathbf {r}_{2K - 1})] + \mathbf {r}_{2K} + \mathbf {r}_{2K + 1}\}$ (or complex conjugate). For other terms, similar rules exist also.

Partial summation over these terms can be made in all orders of perturbation theory. As result to the function $F_{1} (\mathbf {r}, \mathbf {r}, \tau = \tau ^{\prime })$, we obtain the next value (see “Appendix”)

$$\begin{array}{@{}rcl@{}} F_{1} (\mathbf{r}, \mathbf{r}, \tau = \tau^{\prime}) & = & T \sum\limits_{\omega} |\chi_{0} (z)|^{2} \int\limits^{+\infty}_{-\infty} \frac{m d \xi}{(2 \pi \hbar)^{2}} \int\limits^{2 \pi}_{0} d \varphi \\ && \left\{ \frac{\alpha_{1}}{2} \cos \left( \frac{(\mathbf{Qr})}{\hbar} \right) \left( \frac{1}{\xi^{2} - \left( i \omega - \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2} +{\alpha^{2}_{1}} / 4} + \frac{1}{\xi^{2} - \left( i \omega + \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2} +{\alpha^{2}_{1}} / 4} \right) \right. \\[2ex] && + \frac{\alpha_{2}}{2} \cos \left( \frac{3(\mathbf{Qr})}{\hbar} \right) \left( \frac{1}{\xi^{2} - \left( i \omega - \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2} } + \frac{1}{\xi^{2} - \left( i \omega + \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2}} \right) \\[2ex] && \left. + \frac{\alpha_{3}}{2} \cos \left( \frac{5(\mathbf{Qr})}{\hbar} \right) \left( \frac{1}{\xi^{2} - \left( i \omega - \frac{5(\mathbf{PQ})}{2m} - \lambda \right)^{2}} + \frac{1}{\xi^{2} - \left( i \omega + \frac{5(\mathbf{PQ})}{2m} - \lambda \right)^{2}} \right) \right\} \\[2ex] && - T \sum\limits_{\omega} \chi_{0} (z) \chi^{*}_{0} (z) \int\limits^{+\infty}_{-\infty} \frac{m d \xi}{(2 \pi \hbar)^{2}} \int\limits^{2 \pi}_{0} d \varphi \end{array} $$

$$\begin{array}{@{}rcl@{}}&& \left\{ \frac{{\alpha^{3}_{1}}}{8} \cos \left( \frac{3(\mathbf{Qr})}{\hbar} \right) \left( \frac{1}{\xi^{2} - \left( i \omega - \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2}} \cdot \frac{1}{\xi^{2} - \left( i \omega + \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2} +{\alpha^{2}_{1}} / 4} \right. \right. \\[2ex] && + \left. \frac{1}{\xi^{2} - \left( i \omega + \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2}} \cdot \frac{1}{\xi^{2} - \left( i \omega - \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2} +{\alpha^{2}_{1}} / 4} \right) \\[2ex] && + \frac{{\alpha^{3}_{1}}}{4} \cos \left( \frac{(\mathbf{Qr})}{\hbar} \right) \left[ \frac{1}{\left( -i \omega + \xi - \frac{3(\mathbf{PQ})}{2m} + \lambda \right) \left( i \omega + \xi - \frac{(\mathbf{PQ})}{2m} - \lambda \right) \left( \xi^{2} - \left( i \omega - \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2} + {\alpha^{2}_{1}} / 4 \right)} \right. \\[2ex] && \left. \left. + \frac{1}{\left( - i \omega + \xi + \frac{3(\mathbf{PQ})}{2m} + \lambda \right) \left( i \omega + \xi + \frac{(\mathbf{PQ})}{2m} - \lambda \right) \left( \xi^{2} - \left( i \omega + \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2} + {\alpha^{2}_{1}} / 4 \right)} \right] \right\} \end{array} $$

(19)

here $\left (\mathbf {PQ}\right )$ is a scalar product of vector P on Fermi surface and vector Q, φ is angle between P and H, λ = λ₊.

Terms F₂, F₃ can be considered as a small correction to the quantity $Tr \hat {F} (r, r, \tau = \tau ^{\prime })$. As a result, general equation to the order parameter near critical transition surface is

$$ \alpha_{1} \cos \left( \frac{(\mathbf{Qr})}{\hbar} \right) + \alpha_{2} \cos \left( \frac{3(\mathbf{Qr})}{\hbar} \right) = |V_{0}| \cdot F_{1} $$

(20)

Equations (19), (20) enable us to obtain expressions for free energy F on the subspace of the periodic function (18). The free energy F is in such a case a function of the parameters $\{ \alpha _{1}, \alpha _{2}; \dots ;~~PQ/2m \}$. For any value of temperature T, the parameter PQ/2m can be found from the equation

$$ \frac{\partial F}{\partial (PQ/2m)} = 0~~. $$

(21)

here P = P_F.

The system of equations (20) itself can be found from extremum condition of functional F over parameters $\{ \alpha _{1}, \alpha _{2}; \dots \}$, i.e.,

$$ \frac{\partial F}{\partial \alpha_{j}} = 0 ,\quad~~\{ \alpha_{j} = 1, 2, \dots \}~. $$

(22)

Below, we will consider low-temperature region T ≪ PQ/2m.

Note that integrals in (19) can be written down in a form more simple for integration. To do this, we note that in a low-temperature region the main contribution to the integrals arises from regions of φ close to π/2, with a₋ in denominator of integrals, or in the regions near the points $\{ \varphi _{0} = -\arcsin (|R_{2}|); \varphi _{1} = -\pi - \varphi _{0} \}$ if in denominator of integrals stay a₊. The quantities a_± are equal to

$$ a_{\pm} = \frac{p_{F} Q}{2m} \sin \varphi \pm \lambda . $$

(23)

In the vicinity of point $\varphi = \frac {\pi }{2}$, we have

$$\begin{array}{@{}rcl@{}} \varphi &=& \frac{\pi}{2} + \delta \varphi~;~\sin \varphi = 1 - \frac{\delta \varphi}{2}^{2};\\ a_{-} &=& - \frac{h}{2 \sqrt{\gamma_{1}}} \left\{ \frac{1-R_{2}}{2} (\delta \varphi)^{2} + \frac{\delta \gamma_{1}}{\gamma_{1}} \right\}~;~\frac{h}{2 \sqrt{\gamma_{1}}} \frac{\delta \gamma_{1}}{\gamma_{1}} = - \pi T \beta\\ \end{array} $$

(24)

The quantities {δγ₁/γ₁, β} have been found in paper [4]. For T = 0, eight branches are found in paper [3] with different values of Q on the plane of parameters (γ₁, γ₂), where

$$ \gamma_{1}=\left( \frac{2h}{v_{F}Q}\right)^{2} ,\gamma_{2}=\left( \frac{\tilde\alpha\epsilon_{F}}{h}\right)^{2} $$

(25)

Optimal are branches I, II. These branches are parametrized by one parameter R₂(− 1 < R₂ < − 1/2). Parameter γ₂ we consider as given. And for small values of T (not equal to 0), we can put [4]

$$ \frac{\delta\gamma_{1}}{\gamma_{1}}=-\beta Z \frac{1-R_{2}}{2} $$

(26)

The quantity β is dimensionless function of Z. Definition of function Z and exact equation for β are given in (49). In main approximation, we have

$$ \beta=\frac{1}{\pi} \ln\left( \frac{\pi}{(1-R_{2})\sqrt{Z}}\right) $$

(27)

In the vicinity of point $\varphi _{0} = - \arcsin (|R_{2}|)$, we have

$$\begin{array}{@{}rcl@{}} \sin \varphi &=& R_{2} + \sqrt{1 - {R^{2}_{2}}}~ \delta \varphi ;\\ a_{+} &=& \frac{h}{\sqrt{\gamma_{1}}} \left\{ - \sqrt{1 - {R^{2}_{2}}} ~\frac{1 - R_{2}}{2 R_{2}} \delta \varphi - \frac{\delta \gamma_{1} R_{2}}{2 \gamma_{1}} \right\}. \end{array} $$

(28)

And in the vicinity of point $\varphi _{1} = - \pi + \arcsin (|R_{2}|)$, we obtain

$$\begin{array}{@{}rcl@{}} \sin \varphi &=& R_{2} - \sqrt{1 - {R^{2}_{2}}}~ \delta \varphi ;\\ a_{+} &=& \frac{h}{\sqrt{\gamma_{1}}} \left\{ \sqrt{1 - {R^{2}_{2}}} ~\frac{1 - R_{2}}{2 R_{2}} \delta \varphi - \frac{\delta \gamma_{1} R_{2}}{2 \gamma_{1}} \right\} . \end{array} $$

(29)

Now, we define three integrals {J, J₁, J₂} that are parts of integrals in (19).

$$\begin{array}{@{}rcl@{}} J & = & T \sum\limits_{\omega} \left( \chi_{0} (z)\right)^{2} \int\limits^{\infty}_{- \infty} \frac{d \xi}{(2 \pi \hbar)^{2}} \int\limits^{2 \pi}_{0} d \varphi \left\{\! \left( \! \frac{1}{\xi^{2} + \frac{{\alpha_{1}^{2}}}{4} \!-\! (i \omega - a_{+})^{2}} \!+\! \frac{1}{\xi^{2} + \frac{{\alpha_{1}^{2}}}{4} \!-\! (i \omega + a_{-})^{2}} \right)\right.\\ &&\left. - \left( \frac{1}{\xi^{2} - (i \omega - a_{+})^{2}} + \frac{1}{\xi^{2} - (i \omega + a_{-})^{2}} \right)\vphantom{\frac{{\alpha_{1}^{2}}}{4} - (i \omega + a_{-})^{2}} \right\} \\ J_{1} & = & T \sum\limits_{\omega} \left( \chi_{0} (z)\right)^{2} \int\limits^{\infty}_{- \infty} \frac{d \xi}{(2 \pi \hbar)^{2}} \int\limits^{2 \pi}_{0} d \varphi \left\{ \frac{\xi^{2} - (i \omega + a_{-})^{2}}{\xi^{2} - (i \omega - \frac{3(\mathbf{PQ})}{2m} - \lambda)^{2}} \left( \frac{1}{\xi^{2} - (i \omega + a_{-})^{2}} - \frac{1}{\xi^{2} + \frac{{\alpha^{2}_{1}}}{4} - (i \omega + a_{-})^{2}} \right) \right. \\ &&+\left. \frac{\xi^{2} - (i \omega - a_{+})^{2}}{\xi^{2} - (i \omega + \frac{3(\mathbf{PQ})}{2m} - \lambda)^{2}} \left( \frac{1}{\xi^{2} - (i \omega - a_{+})^{2}} - \frac{1}{\xi^{2} + \frac{{\alpha^{2}_{1}}}{4} - (i \omega - a_{+})^{2}} \right) \right\} \\ J_{2} & = & T \sum\limits_{\omega} \left( \chi_{0} (z)\right)^{2} \int\limits^{\infty}_{- \infty} \frac{d \xi}{(2 \pi \hbar)^{2}} \int\limits^{2 \pi}_{0} d \varphi \left\{ \frac{- i \omega + \xi + a_{+}}{(- i \omega + \xi - \frac{3(\mathbf{PQ})}{2m} + \lambda)} \left( \frac{1}{\xi^{2} - (i \omega - a_{+})^{2}}- \frac{1}{\xi^{2} + \frac{{\alpha^{2}_{1}}}{4} - (i \omega - a_{+})^{2}} \right) \right. \\ && \left. + \frac{- i \omega + \xi - a_{-}}{(-i \omega + \xi + \frac{3(\mathbf{PQ})}{2m} + \lambda)^{2}} \left( \frac{1}{\xi^{2} - (i \omega + a_{-})^{2}}- \frac{1}{\xi^{2} + \frac{{\alpha^{2}_{1}}}{4} - (i \omega + a_{-})^{2}} \right) \right\} \end{array} $$

(30)

More simple for calculations are derivatives of integrals {J, J₁, J₂} over parameter α₁. With help from (24), (28), (29), (30), we obtain

$$\begin{array}{@{}rcl@{}}\frac{\partial J}{\partial \alpha_{1}} & = & T \sum\limits_{\omega} |\chi_{0}|^{2} \int\limits^{\infty}_{- \infty} \frac{d \xi}{(2 \pi \hbar)^{2}} \frac{\partial}{\partial \alpha_{1}} \left\{ \left( \frac{2 \sqrt{\gamma_{1}}}{h} \frac{2}{1 - R_{2}} \right)^{1/2} \int\limits^{\infty}_{- \infty} dt \frac{1}{\xi^{2} + \frac{{\alpha^{2}_{1}}}{4} - \left[ i \omega - \left( t^{2} + \frac{h}{2 \sqrt{\gamma_{1}}} \frac{\delta \gamma_{1}}{\gamma_{1}} \right) \right]^{2}} \right. \\ && \left. -2 \left( \frac{2 |R_{2}|}{1 - R_{2}} \frac{\sqrt{\gamma_{1}}}{h \sqrt{1 - {R^{2}_{2}}}} \right) \int\limits^{\infty}_{- \infty} dt \frac{1}{\left( t - i \omega + \sqrt{\xi^{2} + {\alpha^{2}_{1}} /4} \right) \left( t - i \omega - \sqrt{\xi^{2} + {\alpha^{2}_{1}} /4} \right)} \right\} ~~. \end{array} $$

(31)

The last integral in (31) is equal to 0. That is, the main contribution arises only from term with a₋ in denominator. The same situation takes place for integrals {J₁, J₂}. We have

$$\begin{array}{@{}rcl@{}} - \frac{2}{\alpha_{1}} \frac{\partial}{\partial \alpha_{1}} \left( \frac{2}{\alpha_{1}} \frac{\partial J_{1}}{\partial \alpha_{1}} \right) & = & T \sum\limits_{\omega} |\chi_{0} (z)|^{2} \int\limits^{\infty}_{- \infty} \frac{d \xi}{(2 \pi \hbar)^{2}} \int\limits^{2 \pi}_{0} d \varphi \frac{\xi^{2} - (i \omega + a_{-})^{2}}{\xi^{2} - \left( i \omega - \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2}} \\[-1pt] && \cdot \frac{2}{\left( \xi^{2} + \frac{{\alpha^{2}_{1}}}{4} - \left( i \omega + a_{-} \right)^{2} \right)^{3}} \\[-1pt] - \frac{2}{\alpha_{1}} \frac{\partial}{\partial \alpha_{1}} \left( \frac{2}{\alpha_{1}} \frac{\partial J_{2}}{\partial \alpha_{1}} \right) & = & T \sum\limits_{\omega} |\chi_{0} (z)|^{2} \int\limits^{\infty}_{- \infty} \frac{d \xi}{(2 \pi \hbar)^{2}} \int\limits^{2 \pi}_{0} d \varphi \frac{ -i \omega + \xi - a_{-}}{- i \omega + \xi + \frac{3(\mathbf{PQ})}{2m} + \lambda} \\[-1pt] && \cdot \frac{2}{\left( \xi^{2} + \frac{{\alpha^{2}_{1}}}{4} - \left( i \omega + a_{-} \right)^{2} \right)^{3}}~~. \end{array} $$

(32)

Main contribution in the integral value arises from the point $(\varphi = \frac {\pi }{2})$, where we will put

$$ \varphi = \frac{\pi}{2} + \delta \varphi , \,\,\, \sin \varphi \!=\! 1 - (\delta \varphi)^{2} /2, \,\,\, \delta \varphi \!=\! \left( \frac{2 \sqrt{\gamma_{1}}}{h} \frac{2}{1 - R_{2}} \right)^{1/2} t~~. $$

(33)

Equations (31), (32), and (33) enable us to calculate the value of all integrals (J, J₁, J₂) and complete estimation of equation for order parameter in low-temperature region.

4 Subregion of Low-Temperature Region α ₁ > 2π T β

The low-temperature region P_FQ/m ≫ πT decays on two subregions: α₁ > 2πTβ and α₁ ≪ 2πTβ. We will consider first the region α₁ > 2πTβ. In this region, we obtain from (31)

$$\begin{array}{@{}rcl@{}} \frac{\partial J}{\partial \alpha_{1}} \!& = & \left< |\chi_{0} (z)|^{2} \right> \left( \frac{2 \sqrt{\gamma_{1}}}{h} \frac{2}{1 - R_{2}} \right)^{1/2} \frac{\partial}{\partial \alpha_{1}} \int\limits^{\infty}_{- \infty} \frac{d \xi}{(2 \pi \hbar)^{2}} \int\limits^{\infty}_{- \infty} dt\\ &&\times\left\{ \frac{1}{2 \pi} \int\limits^{\infty}_{- \infty} d \omega \frac{1}{\xi^{2} + \frac{{\alpha^{2}_{1}}}{4} + \left( \omega + i \left( t^{2} - \pi T \beta \right) \right)^{2}} \right. \\ && + \left[ \frac{T}{2i} \int\limits_{\overrightarrow{.....}} dy (tg (\pi y) -i) + \frac{T}{2i} \int\limits_{\underleftarrow{.....}} dy (tg (\pi y) +i) \right] \\ &&\left.\times\frac{1}{\xi^{2} + \frac{{\alpha^{2}_{1}}}{4} - \left[ i 2 \pi Ty - \left( t^{2} - \pi T \beta \right) \right]^{2}} \right\}~~. \end{array} $$

(34)

Here the arrow means the integration contour and points indicate the poles of tg(πy).

In the range α₁ > 2πTβ, we obtain from (34)

$$\begin{array}{@{}rcl@{}} \frac{\partial J}{\partial \alpha_{1}} \!& = & \left< |\chi_{0} (z)|^{2} \right> \left( \frac{2 \sqrt{\gamma_{1}}}{h} \frac{2}{1 - R_{2}} \right)^{1/2} \int\limits^{\infty}_{- \infty} \frac{d \xi}{(2 \pi \hbar)^{2}} \frac{\partial}{\partial \alpha_{1}} \\ && \left\{\! \frac{R}{\sqrt{\xi^{2} + {\alpha^{2}_{1}} /4}} -\! {\int\limits^{R}_{0}}\! \frac{dt}{\sqrt{\xi^{2} + {\alpha^{2}_{1}} /4}} \frac{\exp \left( - \left( R^{2} - t^{2} \right) / T \right)}{1 + \exp \left( -\! \left( R^{2} \!-\! t^{2} \right) \!/ T \right)} \right. \!\!\! \\ && \left. -\! \int\limits^{\infty}_{0}\! \frac{dt}{\sqrt{\xi^{2} \!+\! {\alpha^{2}_{1}} /4}} \frac{\exp \left( - \left( t^{2} + \sqrt{\xi^{2} + \frac{{\alpha^{2}_{1}}}{4}} - \pi T \beta \right) / T \right)}{1 + \exp \left( \!-\! \left( t^{2} \!+\! \sqrt{\xi^{2} \!+\! \frac{{\alpha^{2}_{1}}}{4}} \!-\! \pi T \beta \right) \!/ T\! \right)} \right.\!\!\!\! \\ && \left. + \int\limits^{\infty}_{R} \frac{dt}{\sqrt{\xi^{2} + {\alpha^{2}_{1}} /4}} \frac{\exp \left( - \left( t^{2} - R^{2} \right) / T \right)}{1 + \exp \left( - \left( t^{2} - R^{2} \right) / T \right)} \right\} \end{array} $$

(35)

where $R = \left (\sqrt {\xi ^{2} + {\alpha ^{2}_{1}} /4} + \pi T \beta \right )^{1/2}$.

The second and fourth terms in (35) strongly cancel each other. Expansion is going over powers of (T/R²). As result, we obtain

$$\begin{array}{@{}rcl@{}} &&\!\!\!- {\int\limits^{R}_{0}} dt \frac{\exp \left( - \left( R^{2} - t^{2} \right) / T \right)}{1 + \exp \left( - \left( R^{2} - t^{2} \right) / T \right)}\\ &&\!\!\!+ \int\limits^{\infty}_{R} dt \frac{\exp \left( - \left( t^{2} - R^{2} \right) / T \right)}{1 + \exp \left( - \left( t^{2} - R^{2} \right) / T \right)} = - \frac{T^{2}}{4 \cdot R^{3}} \zeta_{(2)}, \\[1ex] &&\!\!\!\int\limits^{\infty}_{- \infty} d \xi \int\limits^{\infty}_{0} \!\frac{dt}{\sqrt{\xi^{2} + {\alpha^{2}_{1}} /4}} \frac{\exp \left( \!-\! \left( t^{2} + \sqrt{\xi^{2} + {\alpha^{2}_{1}} /4} - \pi T \beta \right) \!/ T\! \right)}{1 \!+\! \exp \left( \!-\! \left( \! t^{2} \!+\! \sqrt{\xi^{2} \!+\! {\alpha^{2}_{1}} /4} \!-\! \pi T \beta \right) \!/ T \!\right)}\\ && \!\!\!= \frac{\pi T}{\sqrt{\alpha_{1}}} \ln \left( 1 + \exp \left( -\left( \frac{\alpha_{1}}{2T} - \pi \beta\right) \right) \right) \end{array} $$

(36)

Final answer for the function J in the region α₁ > 2πTβ is

$$\begin{array}{@{}rcl@{}} J & = & \left< |\chi_{0} (z)|^{2} \right> \left( \frac{2 \sqrt{\gamma_{1}}}{h} \frac{2}{1 - R_{2}} \right)^{1/2} \frac{1}{(2 \pi \hbar)^{2}} \\ && \cdot \left\{\vphantom{\int\limits^{\infty}_{0}} J^{(2)} - \frac{\pi T}{\sqrt{\alpha_{1}}} \ln \left[ 1 + \exp \left( - \left( \frac{\alpha_{1}}{2T} - \pi \beta \right) \right) \right]\right.\\ &&\left.- \frac{\zeta_{(2)} T^{2}}{2} \int\limits^{\infty}_{0} \frac{d \xi}{R^{3} \sqrt{\xi^{2} + \frac{{\alpha^{2}_{1}}}{4}}} \right\} \end{array} $$

(37)

here ζ_(x)—is Riemann ζ function, and function J⁽²⁾ is given by equation

$$\begin{array}{@{}rcl@{}} \frac{\partial J^{(2)}}{\partial \alpha_{1}} \!&=& 2 \int\limits^{\infty}_{0} d \xi \frac{\partial}{\partial \alpha_{1}} \left( \frac{R}{\sqrt{\xi^{2} + {\alpha^{2}_{1}} /4}} \right)\\ \!&=&\! - \frac{1}{\sqrt{2 \alpha_{1}}} \int\limits^{\infty}_{0} dy \frac{\sqrt{y^{2} + 1} + 4 \pi T \beta / \alpha_{1}}{(y^{2} + 1)^{3/2} \left( \sqrt{y^{2} + 1} + 2 \pi T \beta / \alpha_{1} \right)^{1/2}}.\\ \end{array} $$

(38)

It has series expansion over parameter (2πTβ/α₁)

$$ J^{(2)} = - \sqrt{2 \alpha_{1}} C_{1} + \sqrt{2 \alpha_{1}} \cdot \frac{3 \pi T \beta}{\alpha_{1}} C_{2} + \dots $$

(39)

with coefficients C₁, C₂ equal to

$$\begin{array}{@{}rcl@{}} C_{1} & = & \int\limits^{\infty}_{0} \frac{dy}{(y^{2} + 1)^{5/4}} \!=\! \sqrt{2} \left[ (2 \!-\! \sqrt{2}) +\! \sum\limits^{\infty}_{K = 1} B_{K} \right] \!=\! 1.198134;\\ B_{1} &=& \frac{1}{3}\ , \ B_{K} = -B_{K-1} \frac{(K-5/4)(K-1/2)}{K(K-1/4)} \ \ ; \\ C_{2} & = & \int\limits^{\infty}_{0} \frac{dy}{(y^{2} + 1)^{7/4}} = \frac{\sqrt{2}}{3} \sum\limits^{\infty}_{K = 0} \tilde{B}_{K} = 0.874015 ; \end{array} $$

$$\begin{array}{@{}rcl@{}} \tilde{B}_{0} &=& 2 , \tilde{B}_{K}=-\tilde{B}_{K-1} \cdot \frac{(K-1/2)}{K} \cdot \frac{(K-3/4)}{K + 1/4} \ \ ; K \geqslant 1 \\ && \int\limits^{\infty}_{0} \frac{dy}{(y^{2} + 1)^{9/4}} = \frac{3}{5} C_{1}\ \ ; \int\limits^{\infty}_{0} \frac{dy}{(y^{2} + 1)^{11/4}} = \frac{5}{7} C_{2}\ \ ; \ \dots \\ \end{array} $$

(40)

The last integral in (37) also has series expansion over parameter 2πTβ/α₁.

$$\begin{array}{@{}rcl@{}} \int\limits^{\infty}_{0} \frac{d \xi}{R^{3} \sqrt{\xi^{2} + {\alpha^{2}_{1}} /4}} \!&=&\! \left( \! \frac{2}{\alpha_{1}} \!\right)^{3/2} \!\int\limits^{\infty}_{0} \!\frac{dy}{(y^{2} + 1)^{5/4} \left( \! 1 \!+\! \frac{2 \pi T \beta}{\alpha_{1} \sqrt{y^{2} + 1}}\! \right)^{3/2}} \\ &=& \left( \frac{2}{\alpha_{1}} \right)^{3/2} \left[ C_{1} - \frac{3 \pi T \beta}{\alpha_{1}} C_{2} \dots \right]~~.\\ \end{array} $$

(41)

In the same way, we calculate the value of integrals {J₁, J₂} from (32). We have

$$\begin{array}{@{}rcl@{}} J_{1} & = & \left< |\chi_{0} (z)|^{2} \right> \cdot \left( \frac{2 \sqrt{\gamma_{1}}}{h} \frac{2}{1 - R_{2}} \right)^{1/2} \frac{1}{(2 \pi \hbar)^{2}} \left( \frac{m}{2 P_{F} Q} \right)^{2} \\ && \left\{ \frac{{\alpha^{2}_{1}}}{4} \left( \frac{T^{2} \zeta_{(2)}}{2} \int\limits^{\infty}_{0} \frac{d \xi}{R^{3} \sqrt{\xi^{2} + {\alpha^{2}_{1}} /4}}\right.\right.\\ &&\left.\left. + \frac{\pi T}{\sqrt{\alpha_{1}}} \ln \left[ 1 + \exp \left( - \left( \frac{\alpha_{1}}{2T} - \pi \beta \right) \right) \right] \vphantom{\int\limits^{\infty}_{0}}\right) + J^{(2)}_{1} \right\}\\ \end{array} $$

(42)

where function $J^{(2)}_{1}$ is solution of equation

$$\begin{array}{@{}rcl@{}} \frac{2}{\alpha_{1}} \frac{\partial}{\partial \alpha_{1}} \left( \frac{2}{\alpha_{1}} \frac{\partial J^{(2)}_{1}}{\partial \alpha_{1}} \right) &=& 2 \left( \frac{2}{\alpha_{1}} \right)^{3/2} \int\limits^{\infty}_{0} \frac{dy}{(y^{2} + 1)^{5/2}}\\ &&\times (y^{2} \!+\! 1/4)\! \left( \sqrt{y^{2} + 1} + \frac{2 \pi T \beta}{\alpha_{1}} \right)^{1/2}\\ \end{array} $$

(43)

Function $J^{(2)}_{1}$ has series expansion over parameter 2πTβ/α₁. We obtain from (43)

$$ J^{(2)}_{1} = \frac{22}{50} C_{1} \sqrt{2} \alpha^{5/2}_{1} - \frac{13}{21} \sqrt{2} \pi T \beta C_{2} \cdot \alpha^{3/2}_{1} + \dots $$

(44)

Function J₂ is equal to (in the range α₁ > 2πTβ)

$$\begin{array}{@{}rcl@{}} J_{2} & = & - \left< |\chi_{0} (z)|^{2} \right> \frac{1}{(2 \pi \hbar)^{2}} \left( \frac{2 \sqrt{\gamma_{1}}}{h} \frac{2}{1 - R_{2}} \right)^{1/2} \frac{m}{2 P_{F} Q} \\ && \left\{ - \frac{T^{2} \zeta_{(2)}}{2} \int\limits^{\infty}_{0} \frac{d \xi}{R^{3}} + \frac{\pi T \sqrt{\alpha_{1}}}{2} \ln\right.\\ &&\left.\left[ 1 + \exp \left( - \left( \frac{\alpha_{1}}{2T} - \pi \beta \right)\right) \right] + J^{(2)}_{2} \vphantom{\int\limits^{\infty}_{0}}\right\}\quad; J^{(2)}_{2} = 0\\ \end{array} $$

(45)

The integral in (45) is equal to

$$\begin{array}{@{}rcl@{}} \int\limits^{\infty}_{0} \frac{d \xi}{R^{3}} &=& \left( \frac{2}{\alpha_{1}} \right)^{1/2} \int\limits^{\infty}_{0} \frac{dy}{\left( \sqrt{y^{2} + 1} + \frac{2 \pi T \beta}{\alpha_{1}} \right)^{3/2}}; \\ \int\limits^{\infty}_{0} \frac{dy}{(y^{2} + 1)^{3/4}} &=& 3 \int\limits^{\infty}_{0} \frac{dy}{(y^{2} + 1)^{7/4}} = 3C_{2}. \end{array} $$

(46)

5 Subregion α ₁ < 2π T β

Consider now the low-temperature subregion restricted by condition α₁ < 2πTβ. In this case, we will use the equation, estimated in [4].

$$\begin{array}{@{}rcl@{}} && \frac{i}{2} \int\limits^{\infty}_{- \infty} dt \left\{ \psi^{\prime} \left( \frac{1}{2} + \frac{i}{2} (t^{2} - \beta) \right) - \psi^{\prime} \left( \frac{1}{2} - \frac{i}{2} (t^{2} - \beta) \right)\right\}\\ & = & 2 \pi^{2} \sum\limits^{\infty}_{K = 1} (-)^{K + 1} \sqrt{K} \exp (- \pi K \beta) \\ &&\int\limits^{\infty}_{- \infty} dt \left\{ \psi^{\prime\prime} \left( \frac{1}{2} + \frac{i}{2} (t^{2} - \beta) \right) + \psi^{\prime\prime} \left( \frac{1}{2} - \frac{i}{2} (t^{2} - \beta) \right)\right\}\\ &=& 8 \pi^{3} \sum\limits^{\infty}_{K = 1} (K)^{3/2} (-)^{K} \exp (- \pi K \beta) \end{array} $$

(47)

In main approximation over parameter α₁/(2πTβ), we obtain from (30)

$$\begin{array}{@{}rcl@{}} J & = & \frac{{\alpha^{2}_{1}}}{32} \frac{\sqrt{\pi T}}{(2 \pi T)^{2}} \left< |\chi_{0} (z)|^{2} \right> \frac{1}{(2 \pi \hbar)^{2}} \left( \frac{2 \sqrt{\gamma_{1}}}{h} \frac{2}{1 - R_{2}} \right)^{1/2} \\ && \int\limits^{\infty}_{- \infty} dt \left\{ \psi^{\prime\prime} \left( \frac{1}{2} + \frac{i}{2} (t^{2} - \beta) \right) \!+\! \psi^{\prime\prime} \!\left( \frac{1}{2} - \frac{i}{2} (t^{2} - \beta) \!\right) \right\}.\\ \end{array} $$

(48)

The quantity β is solution of equation [4]

$$ \sum\limits^{\infty}_{K = 1}(-)^{K + 1} \sqrt{K} \exp (- \pi K \beta) \!=\! \frac{1 - R_{2}}{\pi} \sqrt{Z} \quad; \,\,\, Z \!=\! \frac{4 \pi T \sqrt{\gamma_{1}}}{h (1 - R_{2})}~~. $$

(49)

From (47), (48), and (49), we obtain

$$ J = - \frac{\pi {\alpha^{2}_{1}}}{4 T} \left( \frac{\sqrt{\gamma_{1}}}{h} \right) \frac{\left< |\chi_{0} (z)|^{2} \right>}{(2 \pi \hbar)^{2}} \qquad; \qquad \alpha_{1} \ll 2 \pi T \beta~~. $$

(50)

From (32), it follows that

$$\begin{array}{@{}rcl@{}} &&-\frac{2}{\alpha_{1}} \frac{\partial}{\partial \alpha_{1}} \left( \frac{2}{\alpha_{1}} \frac{\partial J_{1}}{\partial \alpha_{1}} \right) = J \cdot \frac{8}{{\alpha^{2}_{1}}} \left( \frac{m}{2P_{F}Q} \right)^{2} ; \\ && J_{1} = \frac{\pi {\alpha^{4}_{1}}}{16 T} \left( \frac{m}{2P_{F}Q} \right)^{2} \frac{\left< |\chi_{0}(z)|^{2} \right>}{(2 \pi \hbar)^{2}} \left( \frac{\sqrt{\gamma_{1}}}{h} \right)~~. \end{array} $$

(51)

In the same approximation, we obtain the next equation for function J₂

$$\begin{array}{@{}rcl@{}} J_{2} \!& = &\! - \left< |\chi_{0}(z)|^{2} \right>\! \left( \! \frac{m}{2P_{F}Q} \!\right) \frac{{\alpha^{2}_{1}}}{16 \sqrt{\pi T}}\! \left( \! \frac{2 \sqrt{\gamma_{1}}}{h} \!\cdot\! \frac{2}{1-R_{2}} \!\right)^{1/2}\! \frac{1}{(2 \pi \hbar)^{2}} \frac{i}{2} \\ && \cdot \int\limits^{\infty}_{- \infty} dt \left( \psi^{\prime} \left( \frac{1}{2} + \frac{i}{2} (t^{2} \!-\! \beta) \right) \!-\! \psi^{\prime} \left( \frac{1}{2} - \frac{i}{2} (t^{2} \!-\! \beta) \right) \right)~. \end{array} $$

(52)

Final expression for value of quantity J₂, following from (47) and (52), is

$$ J_{2} = - \left< |\chi_{0}|^{2} \right> \frac{1}{(2 \pi \hbar)^{2}} \left( \frac{m}{2P_{F}Q} \right) \frac{\pi {\alpha^{2}_{1}}}{2} \left( \frac{\sqrt{\gamma_{1}}}{h} \right)~~. $$

(53)

As a result, in low-temperature region πT ≪ P_FQ/2m, the (20) for order parameter acquires the form

$$\begin{array}{@{}rcl@{}} \alpha_{1} \cos \left( \frac{(\mathbf{Qr})}{\hbar} \right) + \alpha_{2} \cos \left( \frac{3(\mathbf{Qr})}{\hbar} \right) & = & |V_{0}| m \left\{ \cos \left( \frac{(\mathbf{Qr})}{\hbar} \right) \cdot \alpha_{1} (0.5 \cdot J - J_{2}) - \frac{\alpha_{1}}{2} J_{1} \cos \left( \frac{(3 \mathbf{Qr})}{\hbar} \right) \right\} \\[-1pt] && + \frac{|V_{0}| m \left< |\chi_{0}(z)|^{2} \right>}{4 (2 \pi \hbar)^{2}} \left\{ \alpha_{1} \cos \left( \frac{(\mathbf{Qr})}{\hbar} \right) \left[ 4 \pi \ln \left( \frac{\gamma_{1}(T = 0) {\omega^{2}_{D}}}{h^{2}} \right) \right. \right. \\[-1pt] && \left. \left. + 8 \pi \ln 2 - 2 (1-R_{2}) Z \cdot \left( 1 + \ln \left( \frac{\pi}{(1-R_{2}) \sqrt{Z}} \right) \right) \right] \right. \\[-1pt] && \left. + \alpha_{2} \cos \left( \frac{3(\mathbf{Qr})}{\hbar} \right) \left[ 4 \pi \ln \left( \frac{\gamma_{1} {\omega^{2}_{D}}}{9 h^{2}} \right) + 8 \pi \ln 2 \right] \right\} \end{array} $$

(54)

To obtain (54), we used the value of linear on α₁ terms from paper [4] and next equation

$$\begin{array}{@{}rcl@{}} && T \sum\limits_{\omega} \int\limits^{\infty}_{- \infty} \frac{d \xi}{(2 \pi \hbar)^{2}} \int\limits^{2 \pi}_{0} d \varphi \left[ \frac{1}{\xi^{2} - \left( i \omega - \left( \frac{3 (\mathbf{PQ})}{2m} + \lambda \right) \right)^{2}} + \frac{1}{\xi^{2} - \left( i \omega + \left( \frac{3 (\mathbf{PQ})}{2m} - \lambda \right) \right)^{2}} \right] \\[-1pt] && = \frac{1}{2 (2 \pi \hbar)^{2}} \left\{ 4 \pi \ln \left( \frac{\gamma_{1} {\omega^{2}_{D}}}{9 h^{2}} \right) + 8 \pi \ln 2\right\}~~. \end{array} $$

(55)

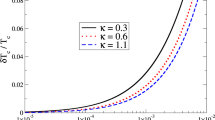
Equation (54) gives temperature, magnetic field, and spin-orbit interaction dependence of the order parameter Δ(r) in low-temperature region πT ≪ P_FQ/2m near the critical surface.

6 Conclusions

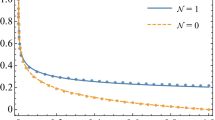
We obtain explicit expression for the order parameter in two-dimensional space (thin films) in inhomogeneous state near the critical surface in low-temperature region πT ≪ (P_FQ)/2m. Low-temperature region πT ≪ (P_FQ)/2m separated for two subregions: α₁ > 2πTβ and α₁ < 2πTβ. In full subregion α₁ > 2πTβ, the relative simple general equations for all coefficients are found. In this subregion, series expansion is going over parameter 2πβT/α₁. All functions are singular in this subregion. Usual expansion over order parameter Δ near the critical point is going over integer powers of Δ similar to that as it takes place in Ginzburg-Landau approximation: after linear term, cubic term is going. In the considered region, next term has power 3/2 (see (37, 39, 50)). Such singularity will present special interest for experimental investigation. Equation (34) is valid also in subregion α₁ < 2πβT. In this subregion, some additional restrictions arise for integration over ξ, t. Partially, in first integral (34), we obtain restriction from down $\pi T \beta -\sqrt {(\alpha _{1})^{2}/4+(\xi )^{2}}<(t)^{2}< (R)^{2}$. In subregion α₁ << 2πTβ, expansion of all coefficients is going over parameter α₁/2πTβ. Nontrivial key equation that enables us to relatively easily find expansion coefficients in this region is given by (47).

We present also the general equation for inhomogeneous state in full temperature region near the critical surface. The reconstruction of free energy functional on the subspace of periodic functions can be made with the help of (20).

Appendix

With help from (14), (17), (18), we obtain the next expression for function Tr$\hat {F}(\mathbf {r}, \mathbf {r}, \tau = \tau ^{\prime })$ inside fifth order perturbation theory over quantity α₁

$$\begin{array}{@{}rcl@{}} Tr \hat{F}(\mathbf{r}, \mathbf{r}, \tau = \tau^{\prime}) &=& F_{1} (\mathbf{r}, \mathbf{r}, \tau = \tau^{\prime}) + F_{2} (\mathbf{r}, \mathbf{r}, \tau = \tau^{\prime})\\ &&+ F_{3} (\mathbf{r}, \mathbf{r}, \tau = \tau^{\prime}) \end{array} $$

(A1)

where

$$\begin{array}{@{}rcl@{}} F_{1} (\mathbf{r}, \mathbf{r}, \tau = \tau^{\prime}) & = & T \sum\limits_{\omega} \chi_{0} (z) \chi^{*}_{0} (z) \int\limits^{\infty}_{- \infty} \frac{m d \xi}{(2 \pi \hbar)^{2}} \int\limits^{2 \pi}_{0} d \varphi \\[-1pt] && \cdot \left\{ \frac{\alpha_{1}}{2} \cos \left( \frac{(\mathbf{Qr})}{\hbar} \right) \left[ \frac{1}{\xi^{2} - \left( i \omega - \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2}} \left( 1 - \frac{{\alpha^{2}_{1}} / 4}{\xi^{2} - \left( i \omega - \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2}} \right. \right. \right. \\[-1pt] && + \left. \left. \left. \frac{\left( {\alpha^{2}_{1}} / 4 \right)^{2}}{\left( \xi^{2} - \left( i \omega - \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2}\right)^{2}} \right) + \frac{1}{\xi^{2} - \left( i \omega + \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2}} \right. \right. \\[-1pt] && \left. \left. \cdot \left( 1 - \frac{{\alpha^{2}_{1}} / 4}{\xi^{2} - \left( i \omega + \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2}} + \frac{\left( {\alpha^{2}_{1}} / 4 \right)^{2}}{\left( \xi^{2} - \left( i \omega + \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2}\right)^{2}} \right) \right] \right. \\[-1pt] && \left. + \frac{\alpha_{2}}{2} \cos \left( \frac{3(\mathbf{Qr})}{\hbar} \right) \left( \frac{1}{\xi^{2} - \left( i \omega - \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2}} + \frac{1}{\xi^{2} - \left( i \omega + \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2}} \right) \right. \\[-1pt] && \left. + \frac{\alpha_{3}}{2} \cos \left( \frac{5(\mathbf{Qr})}{\hbar} \right) \left( \frac{1}{\xi^{2} - \left( i \omega - \frac{5(\mathbf{PQ})}{2m} - \lambda \right)^{2}} + \frac{1}{\xi^{2} - \left( i \omega + \frac{5(\mathbf{PQ})}{2m} - \lambda \right)^{2}} \right) \right\} \\[-1pt] && - T \sum\limits_{\omega} \chi_{0} (z) \chi^{*}_{0} (z) \int\limits^{\infty}_{- \infty} \frac{m d \xi}{(2 \pi \hbar)^{2}} \int\limits^{2 \pi}_{0} d \varphi \\[-1pt] && \cdot \left\{ \frac{{\alpha^{3}_{1}}}{8} \cos \left( \frac{3(\mathbf{Qr})}{\hbar} \right) \left[ \frac{1}{\xi^{2} \!-\! \left( i \omega \!-\! \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2}} \!\left( \! \frac{1}{\xi^{2} \!-\! \left( i \omega \!+\! \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2}} \!-\! \frac{{\alpha^{2}_{1}} / 4}{\left( \! \xi^{2} \!-\! \left( \! i \omega + \frac{(\mathbf{PQ})}{2m} - \lambda \!\right)^{2}\right)^{2}} \right) \right. \right. \\ && \left. \left. + \frac{1}{\xi^{2} - \left( i \omega + \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2}} \cdot \left( \frac{1}{\xi^{2} - \left( i \omega - \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2}} - \frac{{\alpha^{2}_{1}} / 4}{\left( \xi^{2} - \left( i \omega - \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2}\right)^{2}} \right) \right] \right. \\[-1pt] && \left. + \frac{{\alpha^{3}_{1}}}{4} \cos \left( \frac{(\mathbf{Qr})}{\hbar} \right) \left[ \frac{1}{\left( - i \omega + \xi - \frac{3(\mathbf{PQ})}{2m} + \lambda \right) \left( i \omega + \xi - \frac{(\mathbf{PQ})}{2m} - \lambda \right)} \left( \frac{1}{\xi^{2} - \left( i \omega - \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2}} \right. \right. \right. \\[-1pt] && \left. \left. \left. - \frac{{\alpha^{2}_{1}} / 4}{\left( \xi^{2} - \left( i \omega - \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right)^{2}} \right) + \frac{1}{\left( - i \omega + \xi + \frac{3(\mathbf{PQ})}{2m} + \lambda \right) \left( i \omega + \xi + \frac{(\mathbf{PQ})}{2m} - \lambda \right)} \right. \right. \\[-1pt] && \left. \left. \cdot \left( \frac{1}{\xi^{2} - \left( i \omega + \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2}} - \frac{{\alpha^{2}_{1}} / 4}{\left( \xi^{2} - \left( i \omega + \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right)^{2}} \right) \right] \right\} \end{array} $$

(A2)

$$\begin{array}{@{}rcl@{}} && F_{2} (\mathbf{r}, \mathbf{r}, \tau = \tau^{\prime}) = T \sum\limits_{\omega} \chi_{0} (z) \chi^{*}_{0} (z) \int\limits^{\infty}_{- \infty} \frac{m d \xi}{(2 \pi \hbar)^{2}} \int\limits^{2 \pi}_{0} d \varphi \frac{{\alpha^{5}_{1}}}{32} \left\{ \ \cos \left( \frac{(\mathbf{Qr})}{\hbar} \right) \right. \\ && \left. \cdot \left[ 4 \cdot \left( \frac{1}{\left( i \omega + \xi - \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{3} \left( - i \omega + \xi + \frac{(\mathbf{PQ})}{2m} + \lambda \right)^{2} \left( - i \omega + \xi - \frac{3(\mathbf{PQ})}{2m} + \lambda \right)} \right. \right. \right. \\ && \left. \left. \left. + \frac{1}{\left( i \omega + \xi + \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{3} \left( - i \omega + \xi - \frac{(\mathbf{PQ})}{2m} + \lambda \right)^{2} \left( - i \omega + \xi + \frac{3(\mathbf{PQ})}{2m} + \lambda \right)} \right) \right. \right. \\ && \left. \left. + 3 \left( \frac{1}{\xi^{2} - \left( i \omega - \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2} \left( \xi^{2} - \left( i \omega + \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right)^{2}} \right. \right. \right. \\ && \left. \left. \left. + \frac{1}{\left( \xi^{2} - \left( i \omega + \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right) \left( \xi^{2} - \left( i \omega - \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right)^{2}} \right) \right] + 2 \cos \left( \frac{3(\mathbf{Qr})}{\hbar} \right) \right. \\ && \left. \cdot \left[ \left( \frac{1}{\left( i \omega + \xi + \frac{5(\mathbf{PQ})}{2m} - \lambda \right)\left( \xi^{2} - \left( i \omega - \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right)\left( -i \omega + \xi + \frac{3(\mathbf{PQ})}{2m} + \lambda \right)\left( \xi^{2} - \left( i \omega + \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right)} \right. \right. \right. \\ && \left. \left. + \frac{1}{\left( i \omega + \xi - \frac{5(\mathbf{PQ})}{2m} - \lambda \right)\left( \xi^{2} - \left( i \omega + \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right) \left( -i \omega + \xi - \frac{3(\mathbf{PQ})}{2m} + \lambda \right)\left( \xi^{2} - \left( i \omega - \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right)} \right. \right) \\ && \left. \left. + \left( \frac{1}{\left( \xi^{2} - \left( i \omega - \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right) \left( \xi^{2} - \left( i \omega + \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right) \left( -i \omega + \xi + \frac{3(\mathbf{PQ})}{2m} + \lambda \right) \left( i \omega + \xi + \frac{(\mathbf{PQ})}{2m} - \lambda \right)} \right. \right. \right. \\ && \left. \left. + \frac{1}{\left( \xi^{2} - \left( i \omega + \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right) \left( \xi^{2} - \left( i \omega - \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right) \left( -i \omega + \xi - \frac{3(\mathbf{PQ})}{2m} + \lambda \right) \left( i \omega + \xi - \frac{(\mathbf{PQ})}{2m} - \lambda \right)} \right) \right] \\ && \left. + \cos \left( \frac{5(\mathbf{Qr})}{\hbar} \right) \left( \frac{1}{\left( \xi^{2} - \left( i \omega - \frac{5(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right) \left( \xi^{2} - \left( i \omega + \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right) \left( \xi^{2} - \left( i \omega - \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right)} \right. \right. \\ && \left. \left. + \frac{1}{\left( \xi^{2} - \left( i \omega + \frac{5(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right) \left( \xi^{2} - \left( i \omega - \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right) \left( \xi^{2} - \left( i \omega + \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right)} \right) \right\} \end{array} $$

(A3)

$$\begin{array}{@{}rcl@{}} && F_{3} (\mathbf{r}, \mathbf{r}, \tau = \tau^{\prime}) = -T \sum\limits_{\omega} \chi_{0} (z) \chi^{*}_{0} (z) \int\limits^{\infty}_{- \infty} \frac{m d \xi}{(2 \pi \hbar)^{2}} \int\limits^{2 \pi}_{0} d \varphi \frac{{\alpha^{2}_{1}} \alpha_{2}}{8} \left\{ \ 3 \cos \left( \frac{(\mathbf{Qr})}{\hbar} \right) \right. \\ && \left. \cdot \left( \frac{1}{\left( \xi^{2} - \left( i \omega + \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right) \left( \xi^{2} - \left( i \omega - \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right)} + \frac{1}{\left( \xi^{2} \!-\! \left( i \omega - \frac{(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right) \left( \xi^{2} - \left( i \omega + \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right)} \right) \right.\!\! \\ && \left. + 2 \cos \left( \frac{3(\mathbf{Qr})}{\hbar} \right) \left[ \frac{1}{\xi^{2} - \left( i \omega - \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2}} \cdot \left( \frac{1}{\left( i \omega + \xi + \frac{5(\mathbf{PQ})}{2m} - \lambda \right)\left( -i \omega + \xi - \frac{(\mathbf{PQ})}{2m} + \lambda \right)} \right. \right. \right. \\ && \left. \left. \left. + \frac{1}{\left( i \omega + \xi - \frac{3(\mathbf{PQ})}{2m} - \lambda \right)\left( -i \omega + \xi - \frac{(\mathbf{PQ})}{2m} + \lambda \right)} + \frac{1}{\left( i \omega + \xi - \frac{3(\mathbf{PQ})}{2m} - \lambda \right) \left( -i \omega + \xi - \frac{5(\mathbf{PQ})}{2m} + \lambda \right)} \right) \right. \right. \\ && \left. \left. + \frac{1}{\xi^{2} - \left( i \omega + \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2}} \cdot \left( \frac{1}{\left( i \omega + \xi - \frac{5(\mathbf{PQ})}{2m} - \lambda \right) \left( -i \omega + \xi + \frac{(\mathbf{PQ})}{2m} + \lambda \right)} \right. \right. \right. \\ && \left. \left. \left. + \frac{1}{\left( i \omega + \xi + \frac{3(\mathbf{PQ})}{2m} - \lambda \right) \left( -i \omega + \xi + \frac{(\mathbf{PQ})}{2m} + \lambda \right)} + \frac{1}{\left( i \omega \!+ \xi + \frac{3(\mathbf{PQ})}{2m} \!-\! \lambda \right) \left( -i \omega + \xi + \frac{5(\mathbf{PQ})}{2m} + \lambda \right)} \right) \right] \!+\! \cos \left( \frac{5(\mathbf{Qr})}{\hbar} \right) \right. \! \\ && \left. \cdot \left[ \left( \frac{1}{\left( \xi^{2} - \left( i \omega - \frac{5(\mathbf{PQ})}{2m} \!-\! \lambda \right)^{2} \right) \left( \xi^{2} \!-\! \left( i \omega + \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right)} \!+\! \frac{1}{\left( \xi^{2} \!-\! \left( i \omega + \frac{5(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right) \left( \xi^{2} \!-\! \left( i \omega - \frac{3(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right)} \!\right) \right. \right.\!\! \\ && \left. \left. + 2 \left( \frac{1}{\left( \xi^{2} - \left( i \omega - \frac{5(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right) \left( -i \omega + \xi - \frac{3(\mathbf{PQ})}{2m} + \lambda \right) \left( i \omega + \xi - \frac{(\mathbf{PQ})}{2m} - \lambda\right)} \right. \right. \right. \\ && \left. \left. \left. + \frac{1}{\left( \xi^{2} - \left( i \omega + \frac{5(\mathbf{PQ})}{2m} - \lambda \right)^{2} \right) \left( -i \omega + \xi + \frac{3(\mathbf{PQ})}{2m} + \lambda \right) \left( i \omega + \xi + \frac{(\mathbf{PQ})}{2m} - \lambda \right)} \right) \right] \right\} \end{array} $$

(A4)