Skip to main content
SpringerLink
Log in

Geometric analysis and onset of chaos for the resonant nonlinear Schrödinger system

  • Regular Article
  • Published:
The European Physical Journal Special Topics Aims and scope Submit manuscript

This article has been updated

Abstract

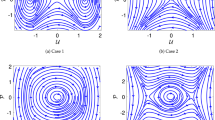
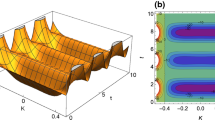
In this paper, Jacobi stability of a resonant nonlinear Schrödinger (RNS) system is studied by the KCC theory, which is also called differential geometric method. The RNS system is transformed into an equivalent planar differential system by traveling wave transformation, then the Lyapunov stability of equilibrium points of the planar system is analyzed. By constructing geometric invariants, we analyze and discuss the Jacobi stability of three equilibrium points. The results show that the zero point is always Jacobi stable, while the Jacobi stability of the other nonzero equilibrium points are determined by the values of the parameters. In addition, the focusing tendency towards trajectories around the equilibrium points are studied by the dynamical behavior of deviation vector. Finally, numerical results show that the system presents quasi-periodic and chaotic phenomena under periodic disturbances.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9

Similar content being viewed by others

Change history

  • 13 January 2022

    This article was revised due to wrong given names for two of the authors of reference 19 in the reference list.

References

  1. E. Schrödinger, An undulatory theory of the mechanics of atoms and molecules. Phys. Rev. 28, 1049–1070 (1926)

    Article  ADS  Google Scholar 

  2. G P. Agrawal, Nonlinear Fiber Optics 4th edn. San Diego, CA (1995)

  3. A. Hasegawa, Y. Kodama, Solitons in Optical Communications (Oxford University Press, Oxford, 1995)

    MATH  Google Scholar 

  4. M. Eslami, M. Mirzazadeh, B. Fathi Vajargah, A. Biswas, The first integral method to study the resonant nonlinear Schrödinger’s equation with time-dependent coeffificients. Optik 125, 3107–3116 (2014)

    Article  ADS  Google Scholar 

  5. H. Triki, T. Hayat, O.M. Aldossary, A. Biswas, Bright and dark solitons for the resonant nonlinear Schrödinger’s equation with time-dependent coeffificients. Opt. Laser Technol. 44, 2223–2231 (2012)

    Article  ADS  Google Scholar 

  6. C. Chen, Y. Jiang, Z. Wang, J. Wu, Dynamical behavior and exact solutions for time-fractional nonlinear Schrödinger equation with parabolic law nonlinearity. Optik 222, 165331 (2020)

    Article  ADS  Google Scholar 

  7. D.D. Kosambi, Parallelism and path-spaces. Math. Z. 37, 608–618 (1933)

    Article  MathSciNet  Google Scholar 

  8. E. Cartan, D.D. Kosambi, Observations sur le mémoireprécédent. Math. Z. 37, 619–622 (1933)

    Article  MathSciNet  Google Scholar 

  9. S.S. Chern, Sur la géométrie d’un systéme d’équations différentielles du second order. Bull. Sci. Math. 63, 206–212 (1939)

    MathSciNet  MATH  Google Scholar 

  10. S.V. Sabau, Some remarks on Jacobi stability. Nonlinear Analysis: Theory, Methods with Applications 63, e143–e153 (2005)

    Article  Google Scholar 

  11. C.G. Böhmer, T. Harko, S.V. Sabau, Jacobi stability analysis of dynamical systems applications in gravitation and cosmology. Adv. Theor. Math. Phys. 16, 1145–1196 (2012)

    Article  MathSciNet  Google Scholar 

  12. P.L. Antonelli, S.F. Rutz, V.S. Sabau, A transient-state analysis of Tyson’s model for the cell division cycle by means of kcc-theory. Open Systems with Information Dynamics 9, 223–238 (2002)

    Article  MathSciNet  Google Scholar 

  13. K. Yamasaki, T. Yajima, Lotka-Volterra system and KCC theory: Differential geometric structure of competitions and predations. Nonlinear Anal. Real World Appl. 14, 1845–1853 (2013)

    Article  MathSciNet  Google Scholar 

  14. K. Yamasaki, T. Yajima, Differential geometric structure of non-equilibrium dynamics in competition and predation: Finsler geometry and KCC theory. Journal of Dynamical Systems and Geometric Theories 14, 137–153 (2016)

    Article  MathSciNet  Google Scholar 

  15. K. Yamasaki, T. Yajima, KCC analysis of a one-dimensional system during catastrophic shift of the Hill function: Douglas tensor in the nonequilibrium region. Int. J. Bifurcat. Chaos 30, 13 (2020)

    Article  MathSciNet  Google Scholar 

  16. C.Y. Leung, C.S. Harko, T. Ho, S. Yip, Jacobi stability analysis of the Lorenz system. Int. J. Geom. Methods Modern Phys. 12, 55–72 (2015)

    MathSciNet  MATH  Google Scholar 

  17. M.K. Gupta, C.K. Yadav, Jacobi stability analysis of Rossler system. Int. J. Bifurcat. Chaos 27, 63–76 (2017)

    Article  Google Scholar 

  18. H. Abolghasem, Jacobi stability of Hamiltonian system. Int. J. Pure Appl. Math. 87, 18–194 (2013)

    Article  Google Scholar 

  19. Q. Huang, A. Liu, Y. Liu, Jacobi stability analysis of Chen system. Int. J. Bifurcat. Chaos 29, 1950139 (2019)

    Article  MathSciNet  Google Scholar 

  20. Y. Liu, H. Chen, X. Lu, C. Feng, A. Liu, Homoclinic orbits and Jacobi stability on the orbits of Maxwell-Bloch system. Appl. Anal. pp 1–20 (2020)

  21. A. Liu, B. Chen, Y. Wei, Jacobi analysis of a disc dynamo system. Int. J. Geometr. Methods Modern Phys. 17, 2050205 (2020)

  22. C.G. Boehmer, T. Harko, S.V. Sabau, Jacobi stability analysis of dynamical systems applications in gravitation and cosmology. Adv. Theor. Math. Phys. 16, 1145–1196 (2012)

    Article  MathSciNet  Google Scholar 

  23. M. Kumar, T.N. Mishra, B. Tiwari, Stability analysis of Navier–Stokes system. Int. J. Geom. Methods Modern Phys. 16, 1950157 (2019)

    Article  ADS  MathSciNet  Google Scholar 

  24. M.K. Gupta, C.K. Yadav, Jacobi stability analysis of Rikitake system. Int. J. Geom. Methods Modern Phys. 13, 1650098 (2016)

    Article  ADS  MathSciNet  Google Scholar 

  25. O.K. Pashaev, J.H. Lee, Resonance solitons as black holes in Madelung fluid. Mod. Phys. Lett. A 17, 1601–1619 (2002)

    Article  ADS  Google Scholar 

  26. W. Krolikowski, O. Bang, Solitons in nonlocal nonlinear media: exact solutions. Phys. Rev. E Stat. Nonlinear Soft Matter Phys. 63, 016610 (2001)

  27. A. Biswas, S. Konar, Introduction to non-Kerr Law Optical Solitons (CRC Press, Boca Raton, 2006)

    Book  Google Scholar 

  28. C. Udrişte, I.R. Nicola, Jacobi stability for geometric dynamics. J. Dyn. Syst. Geom. Theor. 5, 85–95 (2007)

    MathSciNet  MATH  Google Scholar 

  29. S.V. Sabau, Systems biology and deviation curvature tensor. Nonlinear Anal. Real World Appl. 6, 563–587 (2005)

    Article  MathSciNet  Google Scholar 

  30. P. L. (ed.) Antonelli. Handbook of Finsler Geometry. Kluwer Academic, Dordrecht (2003)

  31. M.A. Malkov, Spatial chaos in weakly dispersive and viscous media: A nonperturbative theory of the driven KdV-Burgers equation. Physica D 95, 62–80 (1996)

    Article  ADS  Google Scholar 

  32. T. V. Laptyeva, J. D. Bodyfelt, D. O. Krimer, Ch. Skokos, S. Flach. The crossover from strong to weak chaos for nonlinear waves in disordered systems. EPL (Europhysics Letters), 91:30001(2010)

  33. M. Mulansky, K. Ahnert, A. Pikovsky, D. L. Shepelyansky, Strong and weak chaos in weakly nonintegrable Many-Body Hamiltonian systems. J. Stat. Phys

  34. D.M. Basko, Weak chaos in the disordered nonlinear Schrödinger chain: Destruction of Anderson localization by Arnold diffusion. Ann. Phys. 326, 1577–1655 (2011)

    Article  ADS  MathSciNet  Google Scholar 

  35. N.M. Ryskin, V.N. Titov. Transition to fully developed chaos in a system of two unidirectionally coupled backward-wave oscillators. Technical Physics

  36. C.C. Lalescu, C. Meneveau, G.L. Eyink, Synchronization of chaos in fully developed turbulence. Phys. Rev. Lett. 110, 084102 (2013)

    Article  ADS  Google Scholar 

Download references

Acknowledgements

The authors thank the reviewers for their constructive and pertinent suggestions for improving the manuscript. This work is supported by the National Natural Science Foundation of China (Grant Nos. 11961074, 11971414), Natural Science Foundation of Guangxi Province (Grant No. 2018GXNSFDA281028), the Excellent Youth Foundation of SINOPEC (P20009), the High Level Innovation Team Program from Guangxi Higher Education Institutions of China (Document No. [2018] 35) and the research ability enhancement project of young and middle-aged teachers in Guangxi colleges and universities (Grant No. 2020KY14008).

Author information

Authors and Affiliations

  1. School of Mathematics and Computational Science, Xiangtan University, Xiangtan, 411105, People’s Republic of China

    Ting Lai & Chunsheng Feng

  2. Guangxi Colleges and Universities Key Laboratory of Complex System Optimization and Big Data Processing, Yulin Normal University, Yulin, 537000, People’s Republic of China

    Yongjian Liu & Aimin Liu

Authors
  1. Ting Lai
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Chunsheng Feng
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Yongjian Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Aimin Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yongjian Liu.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Lai, T., Feng, C., Liu, Y. et al. Geometric analysis and onset of chaos for the resonant nonlinear Schrödinger system. Eur. Phys. J. Spec. Top. 231, 2133–2142 (2022). https://doi.org/10.1140/epjs/s11734-021-00398-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1140/epjs/s11734-021-00398-1

Search

Navigation