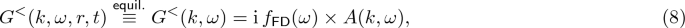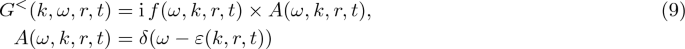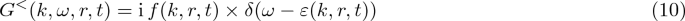Skip to main content
SpringerLink
Log in

Relation between full NEGF, non-Markovian and Markovian transport equations

  • Review
  • Published:
The European Physical Journal Special Topics Aims and scope Submit manuscript

Abstract

This article addresses the problem of an efficient description of the transient electron transport in (primarily small open) quantum systems out of equilibrium. It provides an overview and critical review of the use of causal Ansatzes with the accent on derivation of (quantum) transport equations from the standard Kadanoff–Baym (KB) equations for the non-equilibrium Green’s functions (NEGF). The family of causal Ansatzes originates from the well-known Generalized Kadanoff–Baym Ansatz (GKBA). The Ansatz technique has been fairly successful in practice. Recently, the scope of the method has been extended towards more “difficult” cases and its success can be assessed more precisely. This general picture is demonstrated and analyzed in detail for a variant of the generic molecular island model, an Anderson impurity linked between two bulk metallic leads by tunneling junctions. First, the KB equations are reduced to a non-Markovian generalized master equation (GME) by means of a general causal Ansatz. Further reduction to a Markovian master equation is achieved by partly relaxing the strictly causal character of the theory. For the model narrowed down to ferromagnetic leads, the transient currents are spin polarized and the tunneling functions have a complex spectral structure. This has prompted deriving explicit conditions for the use of an Ansatz. To extend the applicability range of the GME, approximate vertex corrections to the Ansatz were introduced and used with success. Finally, the relation of the GME description to possible non-equilibrium generalizations of the fluctuation–dissipation theorem is shown, extended beyond the present model within the NEGF formalism and physically interpreted in terms of a simplified kinetic theory of non-equilibrium electrons in open quantum systems.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Fig. 1
Fig. 2

Similar content being viewed by others

Notes

  1. The commonly known partitioning dealing with the projection of the whole many body density matrix on the relevant system, e.g., the Nakajima–Zwanzig approach [99, 100], is unrelated to the present discussion.

  2. We can see from the time arguments of KBA that this Ansatz exhibits anticausal features. This corresponds to the construction of the scattering integrals (completed collisions) in the true Boltzmann equation for a quasiparticle distribution function [65]

  3. The above symbolic forms of the KBA and GKBA seem to be nearly equivalent in an equilibrium state—homogeneous in time. In fact, the relation between the KBA proper and the GKBA is far more complicated. To see it, let us go to the Wigner representation using variables \((\omega ,k,r,t)\). Kadanoff and Baym suggested that the form of the exact equilibrium Green’s function fluctuation–dissipation theorem (FDT), cf. para. 5.3.1 below,

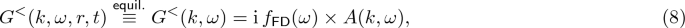
    figure a

    is approximately valid also in near equilibrium situations. In addition they also assumed the quasiparticle behavior of the system and they used the spectral function centered around the quasiparticle energy. Correspondingly, they suggested to use for the derivation of quantum transport equations the couple of the following approximate relations:

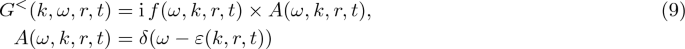
    figure b

    where \( \varepsilon (k,r,t)\) is the quasiparticle energy. This provides the possibility to express the energy argument of f via quasiparticle energy \(\varepsilon (k,r,t)\) and to arrive at the KBA proper:

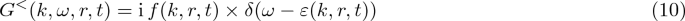
    figure c

    Thus, the KBA is based on the use of the quasiparticle distribution function f, which, in general, is not equal to the single particle density matrix \(\rho \) used in the GKBA. Therefore, in general the KBA and the GKBA do not coincide. They also do not represent exact identities even in equilibrium. For details about the Wigner representation and the differences between the KBA and the GKBA, see [65, 126, 134, 179].

  4. Thus, we leave the idea of anticausal Ansatzes [45, 126, 139] and adopt different approach motivated by the causal structure of NEGF, so we will discuss causal approximations leading to effective transport theories. By this step we will leave aside equations for quasiparticle distribution function. This approach was the original approach designed already by KB, which was, however, limited to the quasiclassical approximation, moderate changes in time and long time asymptotics. We will construct transport equations of the single particle density matrix which covers also fast and abrupt dynamical processes

  5. That may be changed in the future, at least for certain problems, for which a fast algorithm of a direct solution of the KB equations was reported in Ref. [226].

  6. the following decomposition of selfenergy is exactly of the form first given by Danielewicz in [230]. \({}^{\,}_{\ \circ }\varSigma ^<_{\bullet }\) and \({}^{\,}_{\ \bullet }\varSigma ^<_{\circ }\) in (49) are his famous \({\varSigma }^\mathsf{c}\) and \({\varSigma }_\mathsf{c}\).

Abbreviations

GF:

Green functions

NEGF:

Non-equilibrium green functions

OPDM:

One-particle density matrix

FDT:

Fluctuation–dissipation theorem

NE FDT:

Non-equilibrium fluctuation–dissipation theorem

IC:

Initial condition

KB:

Kadanoff–Baym

HF:

Hartree–Fock

KBA:

Kadanoff–Baym Ansatz

GKBA:

Generalized Kadanoff–Baym Ansatz

FGKBA:

Free-particle (propagators) generalized Kadanoff–Baym Ansatz

QGKBA:

Quasiparticle (propagators) generalized Kadanoff–Baym Ansatz

RQGKBA:

Renormalized quasiparticle generalized Kadanoff–Baym Ansatz

XGKBA:

Causal Ansatz (GKBA-like) with propagators \(G^R_X\) and \(G^A_X\)

CGKBA:

Corrected generalized Kadanoff–Baym Ansatz

EOM:

Equations of motion

DE:

Dyson equation

RE:

Reconstruction equations

KBE:

Kadanoff–Baym equation

GKBE:

Generalized Kadanoff–Baym equation

PQKE:

Precursor quantum kinetic equation

PQTE:

Precursor quantum transport equation

QTE:

Quantum transport equation

QKE:

Quantum kinetic equation

QBE:

Quantum Boltzmann equation

GME:

Generalized master equation

ME:

Master equation

References

  1. M. Bonitz, Quantum Kinetic Theory (Teubner, Stuttgart, 1998)

    MATH  Google Scholar 

  2. M. Bonitz, Quantum Kinetic Theory, (Teubner-Texte zur Physik), 2nd edn. (Springer, New York, 2016)

    Book  Google Scholar 

  3. M. Bonitz, M. Scharnke, N. Schlünzen, Time reversal invariance of quantum kinetic equations II: density matrix formalism. Contrib. Plasma Phys. 58, 1036 (2018)

    Article  ADS  MATH  Google Scholar 

  4. I. Knezevic, D.K. Ferry, Partial-trace-free time-convolutionless equation of motion for the reduced density matrix. Phys. Rev. E 66, 016131 (2002)

    Article  ADS  Google Scholar 

  5. I. Knezevic, D.K. Ferry, Memory effects and nonequlibrium transport in open many-particle systems for the reduced density matrix. Phys. Rev. E 67, 066122 (2003)

    Article  ADS  Google Scholar 

  6. I. Knezevic, D.K. Ferry, Quantum transport and memory effects in mesoscopic structures for the reduced density matrix. Phys. E 19, 71 (2003)

    Article  Google Scholar 

  7. I. Knezevic, D.K. Ferry, Open system evolution and memory dressing. Phys. Rev. A 69, 012104 (2004)

    Article  ADS  Google Scholar 

  8. D. Joubert (ed.), Density Functionals: Theory and Applications, Lecture Notes in Phys., vol. 500 (Springer, Berlin, 1998)

    Google Scholar 

  9. D.S. Sholl, J.A. Steckel, Density Functional Theory: A Practical Introduction (Wiley, New Jersey, 2009)

    Book  Google Scholar 

  10. E. Runge, E.K.U. Gross, Density-functional theory for time-dependent systems. Phys. Rev. Lett. 52, 997 (1984)

    Article  ADS  Google Scholar 

  11. K. Burke, E.K.U. Gross, A guided tour of time-dependent density functional theory, in Density Functionals: Theory and Applications, Lecture Notes in Phys., ed. by D. Joubert vol. 500, p. 116 (Springer, Berlin 1998)

  12. R. Van Leeuwen, Key concepts in time-dependent density-functional theory. Int. J. Mod. Phys. B 15, 1969 (2001)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  13. G. Onida, L. Reining, A. Rubio, Electronic excitations: density-functional versus many-body Green’s functional approaches. Rev. Mod. Phys. 74, 601 (2002)

    Article  ADS  Google Scholar 

  14. G. Stefanucci, C.-O. Almbladh, Time-dependent quantum transport: an exact formulation based on TDDFT. Europhys. Lett. 67, 14 (2004)

    Article  ADS  Google Scholar 

  15. S. Kurth, G. Stefanucci, C.-O. Almbladh, A. Rubio, E.K.U. Gross, Time-dependent quantum transport: a practical scheme using density functional theory. Phys. Rev. B 72, 035308 (2005)

    Article  ADS  Google Scholar 

  16. C. Verdozzi, D. Karlsson, M. Puig von Friesen, C.-O. Almbladh, U. von Barth, Some open questions in TDDFT: clues from lattice models and Kadanoff–Baym dynamics. Chem. Phys. 391, 37 (2011)

    Article  Google Scholar 

  17. C. Ullrich, Time-Dependent Density-Functional Theory: Concepts and Applications (Oxford University Press, Oxford, 2012)

    MATH  Google Scholar 

  18. J.K. Freericks, V.M. Turkowski, V. Zlatic, Nonequilibrium dynamical mean-field theory. Phys. Rev. Lett 97, 266408 (2006)

    Article  ADS  Google Scholar 

  19. V.M. Turkowski, J.K. Freericks, Spectral moment sum rules for strongly correlated electrons in time-dependent electric fields. Phys. Rev. B 73, 075108 (2006)

    Article  ADS  Google Scholar 

  20. O.P. Matveev, A.M. Shvaika, J.K. Freericks, Optical and dc-transport properties of a strongly correlated charge-density-wave system: exact solution in the ordered phase of the spinless Falicov-Kimball model with dynamical mean-field theory. Phys. Rev. B 77, 035102 (2008)

    Article  ADS  Google Scholar 

  21. H. Aoki, N. Tsuji, M. Eckstein, M. Kollar, T. Oka, P. Werner, Nonequilibrium dynamical mean-field theory and its applications. Rev. Mod. Phys. 86, 779 (2014)

    Article  ADS  Google Scholar 

  22. J.K. Freericks, Transport in Multilayered Nanostructures: The Dynamical Mean-Field Theory Approach, 2nd edn. (Imperial College Press, London, 2016)

    Book  MATH  Google Scholar 

  23. H.F. Fotso, J.K. Freericks, Characterizing the non-equilibrium dynamics of field-driven correlated quantum systems. Front. Phys. 8, 324 (2020)

    Article  Google Scholar 

  24. M. Schüler, M. Eckstein, P. Werner, Truncating the memory time in nonequilibrium DMFT calculations. Phys. Rev. B 97, 245129 (2018). arXiv:1804.09576v1

  25. A. Picano, J. Li, M. Eckstein, A quantum Boltzmann equation for strongly correlated electrons (2021). arXiv:2101.09037v1 (cond-mat)

  26. M. Grifoni, P. Hänggi, Driven quantum tunneling. Phys. Rep. 304, 229 (1998)

    Article  ADS  MathSciNet  Google Scholar 

  27. S. Kohler, J. Lehmann, P. Hänggi, Driven quantum transport on the nanoscale. Phys. Rep. 406, 379 (2005)

    Article  ADS  Google Scholar 

  28. N. Tsui, T. Oka, H. Aoki, Correlated electron systems periodically driven out of equilibrium: Floquet+DMFT formalism. Phys. Rev. B 78, 235124 (2008)

    Article  ADS  Google Scholar 

  29. T.A. Costi, Renormalization group approach to non-equilibrium Green’s function. Phys. Rev. B 55, 3003 (1997)

    Article  ADS  Google Scholar 

  30. M. Keil, H. Schoeller, The real-time renormalization group approach for the spin-boson model in nonequilibrium. Chem. Phys. 268, 11 (2001)

    Article  Google Scholar 

  31. M.A. Cazalila, J.B. Marston, Time-dependent density-matrix renormalization group. Phys. Rev. Lett. 88, 256403 (2002)

    Article  ADS  Google Scholar 

  32. A.J. Daley, C. Kollath, U. Schollwöck, G. Vidal, Time-dependent density-matrix renormalization-group using adaptive effective Hilbert spaces. J. Stat. Mech. P04005 (2004)

  33. P. Schmitteckert, Nonequilibrium electron transport using the density matrix renormalization group. Phys. Rev. B 70, 121302 (2004)

    Article  ADS  Google Scholar 

  34. S.G. Jakobs, V. Meden, H. Schoeller, Nonequilibrium functional renormalization group for interacting quantum systems. Phys. Rev. B 70, 121302 (2004)

    Google Scholar 

  35. F.B. Anders, Steady-state currents through nanodevices: a scattering states numerical renormalization group approach to open quantum systems. Phys. Rev. Lett. 101, 066804 (2008)

    Article  ADS  Google Scholar 

  36. N. Schlünzen, J.P. Joost, F. Heidrich-Meisner, M. Bonitz, Nonequilibrium dynamics in the one dimensional Fermi–Hubbard model: comparison of the nonequilibrium Green-functions approach and the density matrix renormalization group method. Phys. Rev. B 95, 165139 (2017)

    Article  ADS  Google Scholar 

  37. E. Gull, A.J. Millis, A.I. Lichtenstein, A.N. Rubtsov, M. Troyer, P. Werner, Continuous-time Monte Carlo methods for quantum impurity models. Rev. Mod. Phys. 83, 349 (2011)

    Article  ADS  Google Scholar 

  38. M. Dowling, M.J. Davis, P. Drummond, J. Corney, Monte Carlo techniques for real-time quantum dynamics. J. Comput. Phys. 220, 549 (2020)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  39. J. Schwinger, Quantum electrodynamics III. The electromagnetic properties of the electron-radiative corrections to scattering. Phys. Rev. 76, 796 (1949)

  40. P.C. Martin, J. Schwinger, Theory of many particle systems I. Phys. Rev. 115, 1342 (1959)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  41. J. Schwinger, Brownian motion of a quantum oscillator. J. Math. Phys. 2, 407 (1961)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  42. F. Cooper, Nonequilibrium problems in quantum field theory and Schwinger’s closed time path formalism (1995). arXiv:hep-th/9504073

  43. P.C. Martin, Quantum kinetic equations, in Progress in Non-equilibrium Green’s Functions Bonitz M. (ed.) (World Scientific, Singapore, 2000), p. 2

  44. S.S. Schweber, The sources of Schwinger’s Green’s functions. PNAS 102, 7783 (2005)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  45. L.P. Kadanoff, G. Baym, Quantum Statistical Mechanics (Benjamin, New York, 1962)

    MATH  Google Scholar 

  46. G. Baym, Conservation laws and the quantum theory of transport: the early days, in Progress in Non-equilibrium Green’s Functions, ed. by M. Bonitz (World Scientific, Singapore, 2000), p.17

  47. R.E. Prange, L.P. Kadanoff, Transport theory for electron-phonon interactions in metals. Phys. Rev. 134, A566 (1964)

    Article  ADS  MATH  Google Scholar 

  48. R.E. Prange, A. Sachs, Transport phenomena in the simple metals. Phys. Rev. 158, 672 (1967)

    Article  ADS  Google Scholar 

  49. O.V. Konstantinov, V.I. Perel, A diagram technique for evaluating transport quantities. Sov. Phys. JETP 12, 142 (1961)

    MathSciNet  MATH  Google Scholar 

  50. L.V. Keldysh, Diagram technique for nonequilibrium processes. Sov. Phys. JETP 20, 1018 (1965)

    MathSciNet  Google Scholar 

  51. L.V. Keldysh, Real-time nonequilibrium Green’s functions, in Progress in Non-equilibrium Green’s Functions II, ed. by M. Bonitz, D. Semkat (World Scientific, Singapore, 2003), p. 2

  52. V. Korenman, Non-equilibrium quantum statistics: applications to the laser. Ann. Phys. 39, 72 (1966)

    Article  ADS  Google Scholar 

  53. R.A. Craig, Perturbation expansion for real time Green’s functions. J. Math. Phys. 9, 605 (1968)

    Article  ADS  Google Scholar 

  54. R. Mills, Propagators for Many-particle Systems (Gordon and Branch, New York, 1969)

    Google Scholar 

  55. D.C. Langreth, G. Wilkins, Theory of spin resonance in dilute magnetic alloys. Phys. Rev. B 6, 3189 (1972)

    Article  ADS  Google Scholar 

  56. D.C. Langreth, Linear and non-linear response theory with applications, in Linear and Nonlinear Electron Transport in Solids, ed. by J.T. Devreese, E. van Boren (Plenum, New York, 1976)

  57. E.M. Lifshitz, L.P. Pitaevskii, Physical Kinetics, L.D. Landau and E.M. Lifshitz Course of Theoretical Physics, vol. 10 (Pergamon Press, 1981)

  58. K. Chou, Z. Su, B. Hao, L. Yu, Equilibrium and nonequilibrium formalism made unified. Phys. Rep. 118, 1 (1985)

  59. J. Rammer, H. Smith, Quantum field-theoretical methods in transport theory of metals. Rev. Mod. Phys. 58, 323 (1986)

    Article  ADS  Google Scholar 

  60. G. Strinati, Application of the Green’s functions method to the study of the optical properties of semiconductors. Riv Il Nuovo Cim 11, 1 (1988)

    Article  ADS  Google Scholar 

  61. W. Botermans, R. Malfliet, Quantum transport theory of nuclear matter. Phys. Rep. 198, 115 (1990)

    Article  ADS  Google Scholar 

  62. H. Haug, A.P. Jauho, Quantum Kinetics in Transport and Optics of Semiconductors (Springer, Berlin, 1997)

    Google Scholar 

  63. H. Haug, A.P. Jauho, Quantum Kinetics in Transport and Optics of Semiconductors, 2nd edn. (Springer, Berlin, 2008)

    Google Scholar 

  64. D. Kremp, M. Schlanges, W.D. Kraeft, Quantum Statistics of Nonideal Plasmas (Springer, Berlin, 2005)

    MATH  Google Scholar 

  65. V. Špička, B. Velický, A. Kalvová, Long and short time dynamics: I. Between Green’s functions and transport equations, II. Kinetic regime, III. Transients. Phys. E 29, 154 (2005). ibid 175, ibid 196

  66. M. Bonitz, D. Semkat (eds.), Introduction to Computational Methods in Many Body Physics (Rinton Press Inc, New Jersey, 2006)

    MATH  Google Scholar 

  67. V. Špička, A. Kalvová, B. Velický, Dynamics of mesoscopic systems: non-equilibrium Green’s functions approach. Phys. E 42, 525 (2010)

    Article  MATH  Google Scholar 

  68. T. Kita, Introduction to nonequilibrium statistical mechanics with quantum field mechanics. Prog. Theor. Phys. 123, 581 (2010)

    Article  ADS  MATH  Google Scholar 

  69. A. Kamenev, Field Theory of Non-Equilibrium Systems (Cambridge University Press, Cambridge, 2011)

    Book  MATH  Google Scholar 

  70. K. Balzer, M. Bonitz, Nonequilibrium Green’s Functions Approach to Inhomogeneous Systems (Springer, Berlin, 2013)

    Book  MATH  Google Scholar 

  71. G. Stefanucci, R. van Leeuwen, Nonequilibrium Many-Body Theory of Quantum Systems: A Modern Introduction (Cambridge University Press, Cambridge, 2013)

    Book  MATH  Google Scholar 

  72. V. Špička, B. Velický, A. Kalvová, Electron systems out of equilibrium: nonequilibrium Green’s functions approach. Int. J. Mod. Phys. B 28, 1430013 (2014)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  73. N.J.M. Horing, Quantum Statistical Field Theory: An Introduction to Schwinger’s Variational Method with Green’s Function Nanoapplications, Graphene and Superconductivity (Oxford University Press, Oxford, 2017)

    Book  MATH  Google Scholar 

  74. C. Aron, G. Biroli, L.F. Cugliandolo, (Non) equilibrium dynamics: a (broken) symmetry of the Keldysh generating functional. SciPost Phys. 4, 008 (2018)

    Article  ADS  Google Scholar 

  75. M.R. Hirsbrunner, T.M. Philip, B. Basa, Y. Kim, M.J. Park, M.J. Gilbert, A review of modeling interacting transient phenomena with non-equilibrium Green functions. Rep. Prog. Phys. 82, 046001 (2019)

    Article  ADS  MathSciNet  Google Scholar 

  76. M. Bonitz, K. Balzer, N. Schlünzen, M.R. Rasmussen, J-P. Joost, Ion impact induced ultrafast electron dynamics in correlated materials and finite graphene clusters. Phys. Status Solidi B 256, 1800490 (2019). arXiv:1808.07868v1

  77. N. Schlünzen, S. Hermanns, M. Scharnke, M. Bonitz, Ultrafast dynamics of strongly correlated fermions—nonequilibrium green functions and selfenergy approximations. J. Phys. Condens. Matter 32, 103001 (2020)

  78. M. Esposito, M.A. Ochoa, M. Galperin, Quantum thermodynamics: a nonequilibrium Green’s function approach. Phys. Rev. Lett. 114, 080602 (2015)

    Article  ADS  Google Scholar 

  79. N. Bergmann, M. Galperin, Green’s functions perspective on nonequilibrium thermodynamics of open quantum systems strongly coupled to baths. arXiv:2004.05175v1 [cond-mat.mes-hall]

  80. W. Dou, J. Batge, A. Levy, M. Thoss, Universal approach to quantum thermodynamics of strongly coupled systems under nonequilibrium conditions and external driving. Phys. B 101, 184304 (2020)

    Google Scholar 

  81. A.L. Fetter, J.D. Walecka, Quantum Theory of Many Particle Systems (McGraw-Hill, New York, 1971)

    Google Scholar 

  82. G.D. Mahan, Many Particle Physics (Plenum Press, New York, 1990)

    Book  Google Scholar 

  83. X.-G. Weng, Quantum Field Theory of Many-Body Systems (Oxford University Press, Oxford, 2004)

    Google Scholar 

  84. H. Bruus, K. Flensberg, Many Body Quantum Theory in Condensed Matter Physics (Oxford Un. Press, Oxford, 2004)

    Google Scholar 

  85. H. Kleinert, Path Integrals in Quantum Mechanics, Statistics, Polymer Physics, and Financial Markets, 5th edn. (World Scientific, Singapore, 2009)

    Book  MATH  Google Scholar 

  86. E. Fradkin, Field Theories of Condensed Matter Physics, 2nd edn. (Cambridge University Press, Cambridge, 2013)

    Book  MATH  Google Scholar 

  87. P. Coleman, Introduction to Many-Body Physics (Cambridge University Press, Cambridge, 2015)

    Book  MATH  Google Scholar 

  88. R. Kubo, Statistical-mechanical theory of irreversible processes. I. General theory and simple applications to magnetic and conduction problems. J. Phys. Soc. Jpn. 12, 570 (1957)

  89. R. Kubo, The fluctuation-dissipation theorem. Rep. Prog. Phys. 29, 255 (1966)

    Article  ADS  MATH  Google Scholar 

  90. R. Kubo, M. Toda, N. Hashitsume, Statistical Physics II, 2nd edn. (Springer, Berlin, 1991)

    Book  MATH  Google Scholar 

  91. N.N. Bogolyubov, in Studies in Statistical Mechanics, Vol.1, ed. by G. Uhlenbeck, J. DeBoer (North-Holland, Amsterdam, 1962)

  92. G.V. Chester, The theory of irreversible process. Rep. Prog. Phys. 26, 411 (1963)

    Article  ADS  Google Scholar 

  93. R. Balescu, Equilibrium and Nonequilibrium Statistical Mechanics (Wiley, New York, 1975)

    MATH  Google Scholar 

  94. A.I. Akhiezer, S.V. Peletminskij, Methods of Statistical Physics (Pergamon, New York, 1980)

    Google Scholar 

  95. P. Hänggi, H. Thomas, Stochastic processes: time evolution, symmetries, and linear response. Phys. Rep. 88, 207 (1982)

    Article  ADS  MathSciNet  Google Scholar 

  96. R.L. Liboff, Kinetic Theory, Classical, Quantum and Relativistic Descriptions, 3rd edn. (Prentice Hall, London, 1990)

    Google Scholar 

  97. R. Balian, From Microphysics to Macrophysics, vol. II (Springer, Berlin, 1992)

    MATH  Google Scholar 

  98. T. Dittrich, P. Hänggi, G.-L. Ingold, B. Kramer, G. Schön, W. Zwerger, Quantum Transport and Dissipation (Wiley-WCH, Weinheim, 1998)

    MATH  Google Scholar 

  99. R. Zwanzig, Nonequilibrium Statistical Mechanics (Oxford University Press, New York, 2001)

    MATH  Google Scholar 

  100. H.P. Breuer, F. Petruccione, The Theory of Open Quantum Systems (Oxford, New York, 2002)

  101. F. Benatti, R. Floreanini (ed.), Irreversible Quantum Dynamics (Springer, 2003)

  102. C.M. Van Vliet, Equilibrium and Non-equilibrium Statistical Mechanics (World Scientific, Singapore, 2008)

    Book  MATH  Google Scholar 

  103. U.M.B. Marconia, A. Puglisi, L. Rondonic, A. Vulpiani, Fluctuation–dissipation: response theory in statistical physics. Phys. Rep. 461, 111 (2008)

    Article  ADS  Google Scholar 

  104. M. Di Ventra, Electrical Transport in Nanoscale Systems (Oxford University Press, Oxford, 2008)

    Book  Google Scholar 

  105. Y.V. Nazarov, Y.M. Blunter, Quantum Transport (Cambridge University Press, Cambridge, 2009)

    Book  Google Scholar 

  106. J.C. Cuevas, E. Scheer, Molecular Electronics (World Scientific, Singapore, 2010)

    Book  Google Scholar 

  107. M. Campisi, P. Hänggi, P. Talkner, Colloquium: quantum fluctuation relations: foundations and applications. Rev. Mod. Phys. 83, 771 (2011)

    Article  ADS  MATH  Google Scholar 

  108. R. Klages, W. Just, C. Jarzynski (eds.), Nonequilibrium Statistical Physics of Small Systems: Fluctuation Relations and Beyond (Wiley-VCH, Weinheim, 2012)

  109. S. Datta, Lessons from Nanoelectronics (World Scientific, Singapore, 2012)

    Book  MATH  Google Scholar 

  110. D.K. Ferry, An Introduction to Quantum Transport in Semiconductors (Stanford Publishing, Singapore, 2018)

  111. A.D. Zaikin, D.S. Golubev, Dissipative Quantum Mechanics of Nanostructures (Jenny Stanford Publishing, Singapore, 2019)

  112. C.F. Li, G.C. Guo, J. Piilo, Non-Markovian quantum dynamics: what does it mean? Europhys. Lett. 127, 5001 (2019)

    Article  Google Scholar 

  113. V. Špička, A. Kalvová, B. Velický, Fast dynamics of molecular bridges. Phys. Scr. T 151, 014037 (2012)

    Article  ADS  Google Scholar 

  114. J.E. Han, Solution of electric-field-driven tight-binding lattice coupled to fermion reservoirs. Phys. Rev. B 87, 085119 (2013)

    Article  ADS  Google Scholar 

  115. L. Del Re, B. Rost, A.F. Kemper, J.K. Freericks, Driven-dissipative quantum mechanics on a lattice: simulating a fermionic reservoir on a quantum computer. Phys. Rev. B 102, 125112 (2020)

    Article  ADS  Google Scholar 

  116. D. Manzano, A short introduction to the Lindblad master equation. AIP Adv. 10(2), 025106 (2020). arXiv:1906.04478

  117. P. Talkner, P. Hänggi, Colloquium: statistical mechanics and thermodynamics at strong coupling: quantum and classical. Rev. Mod. Phys. 92, 041002 (2020)

    Article  ADS  MathSciNet  Google Scholar 

  118. M. Bonitz, R. Nareyka, D. Semkat (eds.), Progress in Nonequilibrium Green’s Functions (World Scientific, Singapore, 2000)

    MATH  Google Scholar 

  119. M. Bonitz, D. Semkat (eds.), Progress in Nonequilibrium Green’s Functions II (World Scientific, Singapore, 2003)

    MATH  Google Scholar 

  120. M. Bonitz, A. Filinov (eds.), Progress in Nonequilibrium Green’s Functions III. Journal of Physics: Conference Series, vol. 35, no. 1 (2006)

  121. M. Bonitz, K. Balzer (eds.), Progress in Nonequilibrium Green’s Functions IV. Journal of Physics: Conference Series, vol. 220, no. 1, p. 011001 (2010)

  122. R. van Leeuwen, R. Tuovinen, M. Bonitz (eds.), Progress in Nonequilibrium Green’s Functions V. Journal of Physics: Conference Series, vol. 427, no. 1, p. 011001 (2013)

  123. C. Verdozzi, A. Wacker, C.O. Almbladh, M. Bonitz (eds.), Progress in Nonequilibrium Green’s Functions VI. Journal of Physics: Conference Series, vol. 696, no. 1, p. 011001 (2016)

  124. G. Stefanucci, A. Marini, S. Bellucc (eds.), Progress in Nonequilibrium Green’s Functions VII, Phys. Stat. Sol. B7, vol. 256, p. 1900335 (2019)

  125. V. Špička, P. Lipavský, Quasi-particle Boltzmann equation in semiconductors. Phys. Rev. Lett 73, 3439 (1994)

    Article  ADS  Google Scholar 

  126. V. Špička, P. Lipavský, Quasi-particle Boltzmann equation in semiconductors. Phys. Rev. B 29, 14615 (1995)

    Article  ADS  Google Scholar 

  127. Th Bornath, D. Kremp, W.D. Kraeft, M. Schlanges, Kinetic equations for a nonideal quantum system. Phys. Rev. E 54, 3274 (1996)

    Article  ADS  Google Scholar 

  128. D. Kremp, M. Bonitz, W.D. Kraeft, M. Schlanges, Non-Markovian Boltzmann equation. Ann. Phys. 258, 320 (1997)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  129. V. Špička, P. Lipavský, K. Morawetz, Quasi-particle transport equation with collision delay: I. Phenomenological approach. Phys. Rev. B 55, 5084 (1997)

  130. V. Špička, P. Lipavský, K. Morawetz, Quasi-particle transport equation with collision delay: II. Microscopic theory. Phys. Rev. B 55, 5095 (1997)

  131. V. Špička, P. Lipavský, K. Morawetz, Space-time versus particle-hole symmetry in quantum Enskog equations. Phys. Rev. E 64, 046107 (2001)

    Article  ADS  Google Scholar 

  132. V. Špička, P. Lipavský, K. Morawetz, Nonlocal corrections to the Boltzmann equation for dense Fermi systems. Phys. Lett. A 240, 160 (1998)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  133. J. Koide, Quantum kinetic equation in the closed-time-path formalism. Phys. Rev. E 62, 5953 (2000)

    Article  ADS  Google Scholar 

  134. P. Lipavský, K. Morawetz, V. Špička, Kinetic equation for strongly interacting dense Fermi systems. Ann. Phys. Fr. 26, 1 (2001)

  135. P. Lipavský, V. Špička, B. Velický, Generalized Kadanoff–Baym ansatz for deriving quantum transport equations. Phys. Rev. B 29, 6933 (1986)

    Article  ADS  Google Scholar 

  136. H. Chim Tso, N.J.M. Horing, Exact functional differential variant of the generalized Kadanoff–Baym ansatz. Phys. Rev. B 44, 1451 (1991)

  137. M. Bonitz, D. Kremp, D.C. Scott, R. Binder, W.D. Kraeft, H.S. Kohler, Numerical analysis of non-Markovian effects in charge-carrier scattering: one-time versus two-time kinetic equations. J. Phys. Cond. Matter 8, 6057 (1996)

  138. D. Kremp, Th Bornath, M. Bonitz, M. Schlanges, Quantum kinetic theory of plasmas in strong laser fields. Phys. Rev. B 60, 4725 (1999)

    Article  ADS  Google Scholar 

  139. P. Lipavský, V. Špička, K. Morawetz, Approximative constructions of the double-time correlation function from the single-time distribution function, in Progress in Non-equilibrium Green’s Functions, ed. by M. Bonitz (World Scientific, Singapore, 2000), p.63

  140. H. Haug, Interband quantum kinetics with LO-phonon scattering in a laser-pulse-excited semiconductor. Phys. Stat. Sol. (b) 173, 139 (1992)

  141. L. Banyai, D.B. Tran Thoai, C. Remling, H. Haug, Interband quantum kinetics with LO-phonon scattering in a laser-pulse-excited semiconductor II. Numerical studies. Phys. Stat. Sol. (b) 173, 149 (1992)

  142. H. Haug, C. Ell, Coulomb quantum kinetics in a dense electron gas. Phys. Rev. B 46, 2126 (1992)

    Article  ADS  Google Scholar 

  143. K. El Sayed, S. Schuster, H. Haug, F. Herzel, K. Henneberger, Subpicosecond plasmon response—buildup of screening. Phys. Rev. B 49, 7337 (1994)

    Article  ADS  Google Scholar 

  144. L. Banyai, D.B. Tran Thoai, E. Reitsamer, H. Haug, D. Steinbach, M.U. Wehner, M. Wegener, T. Marschner, W. Stolz, Exciton—KLO-phonon quantum kinetics: evidence of memory effects in bulk GaAs. Phys. Rev. Lett. 75, 2188 (1995)

  145. H. Haug, L. Banyai, Improved spectral functions for quantum kinetics. Sol. State Commun. 100, 303 (1996)

    Article  ADS  Google Scholar 

  146. P. Gartner, L. Banyai, H. Haug, Two-time electron-LO-phonon quantum kinetics and the generalized Kadanoff–Baym approximation. Phys. Rev. B 60, 14234 (1999)

    Article  ADS  MATH  Google Scholar 

  147. P. Gartner, L. Banyai, H. Haug, Two-time electron-LO-phonon quantum kinetics and the test of the generalized Kadanoff–Baym relation, in Progress in Non-equilibrium Green’s Functions, ed. by M. Bonitz (World Scientific, Singapore, 2000), p.464

  148. J. Seebeck, T.R. Nielsen, P. Gartner, F. Jahnke, Polarons in semiconductor quantum dots and their role in the quantum kinetics of carrier relaxation. Phys. Rev. B 71, 125327 (2005)

    Article  ADS  Google Scholar 

  149. M. Lorke, T.R. Nielsen, J. Seebeck, P. Gartner, F. Jahnke, Quantum kinetic effects in the optical absorption of semiconductor quantum-dot systems. J. Phys. Conf. Series 35, 182 (2006)

  150. M. Bonitz, T. Bornath, D. Kremp, M. Schlanges, W.D. Kraeft, Quantum kinetic theory for laser plasmas. Dynamical screening in strong fields. Contrib. Plasma Phys. 39, 329 (1999)

  151. D. Kremp, T. Bornath, M. Bonitz, Quantum kinetic theory of plasmas in strong laser fields. Phys. Rev. E 60, 4725 (1999)

    Article  ADS  Google Scholar 

  152. H. Haberland, M. Bonitz, D. Kremp, Harmonics generation in electron-ion collisions in a short laser pulse. Phys. Rev. E 64, 026405 (2001)

    Article  ADS  Google Scholar 

  153. M. Bonitz, S. Hermanns, K. Balzer, Dynamics of Hubbard nano-clusters following strong excitation. Contrib. Plasma Phys. 53, 778 (2013)

    Article  ADS  Google Scholar 

  154. K. Balzer, S. Hermanns, M. Bonitz, The generalized Kadanoff–Baym ansatz. Computing nonlinear response properties of finite systems. J. Phys. Conf. Ser. 427, 012006 (2013)

  155. S. Hermanns, K. Balzer, M. Bonitz, Few-particle quantum dynamics comparing nonequilibrium Green functions with the generalized Kadanoff–Baym ansatz to density operator theory. J. Phys. Conf. Ser. 427, 012008 (2013)

  156. N. Schlünzen, J.P. Joost, M. Bonitz, Achieving the scaling limit for nonequilibrium Green functions simulations. Phys. Rev. Lett. 124, 076601 (2020)

    Article  ADS  MathSciNet  Google Scholar 

  157. J.P. Joost, N. Schlünzen, J.P. Joost, M. Bonitz, G1–G2 scheme: dramatic acceleration of nonequilibrium Green functions simulations within the Hartree–Fock generalized Kadanoff–Baym ansatz. Phys. Rev. B 101, 245101 (2020)

    Article  ADS  Google Scholar 

  158. S. Hermanns, K. Balzer, M. Bonitz, The non-equilibrium Green function approach to inhomogeneous quantum many-body systems using the generalized Kadanoff–Baym ansatz. Phys. Scr. T 151, 014036 (2012)

    Article  ADS  Google Scholar 

  159. K. Balzer, N. Schlünzen, M. Bonitz, Stopping dynamics of ions passing through correlated honeycomb clusters. Phys. Rev. B 94, 245118 (2016)

    Article  ADS  Google Scholar 

  160. K. Balzer, M.R. Rasmussen, N. Schlünzen, J-P. Joost, M. Bonitz, Doublon formation by ions impacting a strongly correlated finite lattice systems. Phys. Rev. Lett. 121, 267602 (2018). arXiv:1801.05267v1

  161. R. Tuovinen, D. Golez, M. Eckstein, M.A. Sentef, Comparing the generalized Kadanoff–Baym ansatz with the full Kadanoff–Baym equations for an excitonic insulator out of equilibrium. Phys. Rev. B 102, 115157 (2020). arXiv:2007.0780 (cond-mat)

  162. F. Cosco, N.W. Talarico, R. Tuovinen, N. Lo Gullo, Spectral properties of correlated quantum wires and carbon nanotubes within the generalized Kadanoff–Baym Ansatz. arXiv:2007.0890 [cond-mat]

  163. D. Karlsson, R. van Leeuwen, Y. Pavlyukh, E. Perfetto, G. Stefanucci, Fast Green’s function method for ultrafast electron-boson dynamics (2021). arXiv:2006.14956 [cond-mat]

  164. N. Kwong, M. Bonitz, R. Binder, H. Köhler, Semiconductor Kadanoff Baym equation results for optically excited electron-hole plasmas in quantum wells. Phys. Status Solidi B 206, 197 (1998)

    Article  ADS  Google Scholar 

  165. M.P. von Friesen, C. Verdozzi, C.O. Almbladh, Successes and failures of Kadanoff–Baym dynamics in Hubbard nanoclusters. Phys. Rev. Lett. 103, 176404 (2009)

    Article  ADS  Google Scholar 

  166. M. Bonitz, S. Hermanns, K. Balzer, Dynamics of Hubbard nano-clusters following strong excitation. Contrib. Plasma Phys. 53, 778 (2013)

    Article  ADS  Google Scholar 

  167. S. Hermanns, N. Schlünzen, M. Bonitz, Hubbard nanoclusters far from equilibrium. Phys. Rev. B 90, 125111 (2014)

    Article  ADS  Google Scholar 

  168. S. Latini, E. Perfetto, A.M. Uimonen, R. van Leeuwen, G. Stefanucci, Charge dynamics in molecular junctions: nonequilibrium Green’s function approach made fast. Phys. Rev. B 89, 075306 (2014)

    Article  ADS  Google Scholar 

  169. E. Perfetto, A. Uimonen, R. van Leeuwen, S. Stefanucci, First principles nonequilibrium Green’s-function approach to transient photoabsorption: application to atoms. Phys. Rev. A 92, 033419 (2015)

    Article  ADS  Google Scholar 

  170. P.M.M.C. de Melo, A. Marini, Unified theory of quantized electrons, phonons, and photons out of equilibrium: a simplified ab initio approach based on the generalized Baym-Kadanoff ansatz. Phys. Rev. B 93, 155102 (2016)

    Article  ADS  Google Scholar 

  171. Jun Koide, Perturbative method for the derivation of quantum kinetic theory based on closed-time-path formalism. Phys. Rev. E 65, 026101 (2002)

    Article  ADS  Google Scholar 

  172. E. Perfetto, G. Stefanucci, CHEERS: a tool for correlated hole-electron evolution from real time simulation. J. Phys. Cond. Matt. 30, 465901 (2018)

  173. R. Tuovinen, R. van Leeuwen, E. Perfetto, G. Stefanucci, Electronic transport in molecular junctions: the generalized Kadanoff Baym ansatz with initial contact and correlations (2020). arXiv:2012.08247v1

  174. B. Velicky, A. Kalvova, Build-up and decoherence of optical transients in disordered semiconductors. Phys. Stat. Sol. (b) 188, 515 (1995)

  175. B. Velický, A. Kalvová, V. Špička, Between Green’s functions and transport equations: reconstruction theorems and the role of initial conditions. J. Phys. Conf. Ser. 35, 1 (2006)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  176. B. Velický, A. Kalvová, V. Špička, Quasiparticle states of electron systems out of equilibrium. Phys. Rev. B 75, 195125 (2007)

    Article  ADS  MATH  Google Scholar 

  177. B. Velický, A. Kalvová, V. Špička, Ward identity for nonequilibrium Fermi systems. Phys. Rev. B 77, 041201(R) (2008)

    Article  ADS  Google Scholar 

  178. B. Velický, A. Kalvová, V. Špička, Correlated initial condition for an embedded process by time partitioning. Phys. Rev. B 81, 235116 (2010)

    Article  ADS  Google Scholar 

  179. V. Špička, B. Velický, A. Kalvová, Non-equilibrium dynamics of open systems and fluctuation-dissipation theorems. Fortschritte der Physik 65, 1700032 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  180. A. Kalvová, B. Velický, V. Špička, Generalized master equation for a molecular bridge improved by vertex correction to the Generalized Kadanoff–Baym Ansatz. Eur. Phys. Lett 121, 67002 (2018)

    Article  ADS  Google Scholar 

  181. A. Kalvová, B. Velický, V. Špička, Beyond the generalized Kadanoff–Baym Ansatz. Phys. Sat. Sol. B 256, 1800594 (2019)

    Article  ADS  Google Scholar 

  182. R. Tuovinen, D. Golez, M. Schüler, P. Werner, M. Eckstein, M.A. Sentef, Adiabatic preparation of a correlated symmetry-broken initial state with the generalized Kadanoff–Baym Ansatz. Phys. Stat. Sol. B. 256, 1800469 (2019)

    Article  ADS  Google Scholar 

  183. M. Hopjan, C. Verdozzi, Initial correlated states for the generalized Kadanoff–Baym Ansatz without adiabatic switching-on of interactions in closed systems. Eur. Phys. J. Spec. Top. 227, 1939 (2019). arXiv:1808.03520

  184. D. Karlsson, R. van Leeuwen, E. Perfetto, G. Stefanucci, The generalized Kadanoff–Baym Ansatz with initial correlations. Phys. Rev. B. 98, 115–148 (2018). arXiv:1806.05639v1

  185. H. Feshbach, Unified theory of nuclear reactions. Ann. Phys. 5, 357 (1958)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  186. H. Feshbach, Unified theory of nuclear reactions. II. Ann. Phys. 19, 287 (1962)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  187. A. Messiah, Quantum Mechanics Two volumes in One (Dover Publications Inc., Mineola, New York, 2014)

  188. P.O. Löwdin, A note on the quantum-mechanical perturbation theory. J. Chem. Phys. 19, 1396 (1951)

    Article  ADS  MathSciNet  Google Scholar 

  189. P.O. Löwdin, Studies in perturbation theory. IV. Solution of Eigenvalue problem by projection operator formalism. J. Math. Phys. 3, 969 (1962)

  190. P.O. Löwdin, Studies in perturbation theory: part I. An elementary iteration-variation procedure for solving the Schrödinger equation by partitioning technique. J. Mol. Spectr. 10, 12 (1963)

  191. A.D. Bochevarov, C.D. Sherrill, Some simple results following from Löwdin’s partitioning technique. J. Math. Chem. 42, 59 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  192. L. Jin, Z. Song, Partitioning technique for discrete quantum systems. Phys. Rev. A 83, 062118 (2011)

    Article  ADS  Google Scholar 

  193. Y. Meir, N.S. Wingreen, Landauer formula for the current through an interacting electron region. Phys. Rev. Lett. 68, 2512 (1992)

    Article  ADS  Google Scholar 

  194. A.P. Jauho, N.S. Wingreen, Y. Meir, Time-dependent transport in interacting and noninteracting resonant tunneling systems. Phys. Rev. B 50, 5528 (1994)

    Article  ADS  Google Scholar 

  195. D.M. Newns, Self-consistent model of hydrogen chemisorption. Phys. Rev. 178, 1123 (1969)

    Article  ADS  Google Scholar 

  196. T.B. Grimley, Electronic structure of adsorbed atoms and molecules. J. Vac. Sci. Technol. 8, 31 (1971)

    Article  ADS  Google Scholar 

  197. M. Galperin, M.A. Ratner, A. Nitzan, Molecular transport junctions: vibrational effects. J. Phys. Condens. Matter 19, 10 (2007)

    Article  Google Scholar 

  198. N.A. Zimbovskaya, M.K. Pedersen, Electron transport through molecular junctions. Phys. Rep. 509, 1 (2011)

    Article  ADS  Google Scholar 

  199. F. Evers, R. Korytar, S. Tewari, J.M. van Ruitenbeek, Advances and challenges in single-molecule electron transport. Rev. Mod. Phys. 92, 035001–2 (2020)

    Article  ADS  Google Scholar 

  200. C. Caroli, R. Combescot, P. Nozieres, D. Saint-James, Direct calculation of the tunneling current. J. Phys. C Solid State Phys. 4, 916 (1971)

    Article  ADS  Google Scholar 

  201. C. Caroli, R. Combescot, D. Lederer, P. Nozieres, D. Saint-James, A direct calculation of the tunnelling current. II. Free electron description. J. Phys. C Solid State Phys. 4, 2598 (1971)

  202. M. Cini, Time-dependent approach to electron transport through junctions: General theory and simple applications. Phys. Rev. B 22, 5887 (1980)

    Article  ADS  Google Scholar 

  203. T.L. Schmidt, P. Werner, L. Mühlbacher, A. Komnik, Transient dynamics of the Anderson impurity model out of equilibrium. Phys. Rev. B 78, 235110 (2008)

    Article  ADS  Google Scholar 

  204. D.F. Urban, R. Avriller, A. Levy Yeyati, Nonlinear effects of phonon fluctuations on transport through nanoscale junctions. Phys. Rev. B 82, 121414(R) (2010)

  205. S. Latini, E. Perfetto, A.M. Uimonen, R. van Leeuwen, G. Stefanucci, Charge dynamics in molecular junctions: nonequilibrium Green’s function approach made fast. Phys. Rev. 89, 075306 (2014)

    Article  Google Scholar 

  206. R. Seoane Souto, R. Avriller, R. C. Monreal, A. Martín-Rodero, A. Levy Yeyati, Transient dynamics and waiting time distribution of molecular junctions in the polaronic regime. Phys. Rev. B 92, 125435 (2015)

  207. M. Ridley, A. MacKinnon, L. Kantorovich, Current through a multilead nanojunction in response to an arbitrary time-dependent bias. Phys. Rev. B 91, 125433 (2015)

    Article  ADS  Google Scholar 

  208. M.M. Odashima, C.H. Lewenkopf, Time-dependent resonant tunneling transport: Keldysh and Kadanoff–Baym nonequilibrium Green’s functions in an analytically soluble problem. Phys. Rev. B 95, 104301 (2017)

    Article  ADS  Google Scholar 

  209. B. Velický, A. Kalvová, V. Špička, Single molecular bridge as a testing ground for using NGF outside of the steady state current regime. Phys. E 42, 539 (2010)

    Article  Google Scholar 

  210. A. Kalvová, V. Špička, B. Velický, Fast transient current response to switching events in short chains of molecular islands. J. Superconduct. Novel Magn. 26, 773 (2013)

    Article  Google Scholar 

  211. A. Kalvová, V. Špička, B. Velický, Transient magnetic tunneling mediated by a molecular bridge in the junction region. EPJ Web Conf. 02004 (2014)

  212. A. Kalvová, V. Špička, B. Velický, Transient magnetic tunneling mediated by a molecular bridge. J. Supercond. Novel Magn. 28, 1087 (2015)

    Article  Google Scholar 

  213. A. Kalvová, B. Velický, V. Špička, Transient magnetic currents through a molecular bridge: limits to reduction of nonequilibrium Green’s functions to a generalized master equation. J. Supercond. Novel Magn. 30, 807 (2017)

    Article  MATH  Google Scholar 

  214. G. Cohen, M. Galperin, Green’s function methods for single molecule junctions. J. Chem. Phys. 152, 090901 (2020)

    Article  ADS  Google Scholar 

  215. G. Baym, L.P. Kadanoff, Conservation laws and correlation functions. Phys. Rev. 124, 287 (1961)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  216. G. Baym, Self-consistent approximation in many-body systems. Phys. Rev. 127, 1391 (1962)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  217. S. Hermans, N. Schlünzen, M. Bonitz, Hubbard nanoclusters far from equilibrium. Phys. Rev. B 90, 125111 (2014)

    Article  ADS  Google Scholar 

  218. N. Schlünzen, M. Bonitz, M. Scharnke, Time reversal invariance of quantum kinetic equations: nonequilibrium Green functions formalism. J. Math. Phys. 58, 061903 (2017)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  219. D. Karlsson, R. van Leeuwen, Partial self-consistency and analyticity in many-body perturbation theory: particle number conservation and a generalized sum rule. Phys. Rev. B 94, 125124 (2016)

    Article  ADS  Google Scholar 

  220. M. Revzen, T. Toyoda, Y. Takahashi, F.C. Khanna, Baym–Kadanoff criteria and the Ward–Takahashi relations in many-body theory. Phys. Rev. B 40, 769 (1989)

    Article  ADS  Google Scholar 

  221. A. Gonis, Theoretical Materials Science (Materials Research Society, Warrendale, 2000)

    Google Scholar 

  222. P. Gartner, L. Banyai, H. Haug, Coulomb screening in the two time Keldysh–Green-function formalism. Phys. Rev. B 62, 7116 (2000)

    Article  ADS  Google Scholar 

  223. R. Ramazashvili, Ward identities for disordered metals and superconductors. Phys. Rev. B 66, 220503(R) (2002)

    Article  ADS  Google Scholar 

  224. J. Peralta-Ramos, E. Calzetta, Two-particle irreducible effective action approach to nonlinear current-conserving approximations in driven systems. J. Phys. Condens. Matter 21, 215601 (2009)

    Article  ADS  Google Scholar 

  225. A.S. Kondratyev, N. Shahid, Different forms of the Kadanoff–Baym equations in quantum statistical mechanics. Low Temp. Phys. 37, 777 (2011)

    Article  ADS  Google Scholar 

  226. J. Kaye, D. Golež, Low rank compression in the numerical solution of the nonequilibrium Dyson equation (2021). arXiv:2010.06511v3 [cond-mat.str-el]

  227. S. Fujita, Resolution of the hierarchy of Green’s functions for fermions. Phys. Rev. A 4, 1114 (1971)

    Article  ADS  Google Scholar 

  228. A.G. Hall, Non-equilibrium Green functions: generalized Wick’s theorem and diagrammatic perturbation theory with initial correlations. J. Phys. A 8, 214 (1975)

    Article  ADS  Google Scholar 

  229. Yu. A. Kukharenko, S.G. Tikhodeev, Diagram technique in the theory of relaxation processes. Sov. Phys. JETP 56, 831 (1982)

  230. P. Danielewicz, Quantum theory of nonequilibrium processes, I, II. Ann. Phys. (N.Y.) 152, 239 (1984)

  231. M. Wagner, Expansions of nonequilibrium Green’s functions. Phys. Rev. B 44, 6104 (1991)

    Article  ADS  Google Scholar 

  232. D. Semkat, D. Kremp, M. Bonitz, Kadanoff–Baym equations with initial correlations. Phys. Rev. E 59, 1557 (1999)

    Article  ADS  MATH  Google Scholar 

  233. V.G. Morozov, G. Röpke, The “mixed” Green’s function approach to quantum kinetics with initial correlations. Ann. Phys. 278, 127 (1999)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  234. K. Morawetz, M. Bonitz, V.G. Morozov, G. Röpke, D. Kremp, Short-time dynamics with initial correlations. Phys. Rev. E 63, 020102(R) (2001)

    Article  ADS  Google Scholar 

  235. D. Semkat, D. Kremp, M. Bonitz, Kadanoff–Baym equations and non-Markovian Boltzmann equation in generalized T-matrix approximation. J. Math. Phys. 41, 7458 (2000)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  236. D. Semkat, M. Bonitz, D. Kremp, Relaxation of a quantum many-body system from a correlated initial state. A general and consistent approach. Contrib. Plasma Phys. 43, 321 (2003)

  237. D. Kremp, D. Semkat, M. Bonitz, Short-time kinetics and initial correlations in quantum kinetic theory. J. Phys. Conf. Ser. 11, 1 (2005)

  238. R. van Leeuwen, G. Stefanucci, Wick theorem for general initial states. Phys. Rev. B 85, 115119 (2012)

    Article  ADS  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute of Physics, CAS, 182 21, Praha 8, Czech Republic

    V. Špička, B. Velický & A. Kalvová

  2. Department of Condensed Matter Physics, Charles University, 121 16, Praha 2, Czech Republic

    B. Velický

Authors
  1. V. Špička
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. B. Velický
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. A. Kalvová
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to V. Špička.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Špička, V., Velický, B. & Kalvová, A. Relation between full NEGF, non-Markovian and Markovian transport equations. Eur. Phys. J. Spec. Top. 230, 771–808 (2021). https://doi.org/10.1140/epjs/s11734-021-00109-w

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1140/epjs/s11734-021-00109-w

Search

Navigation