Phonon trapping states as a witness for generation of phonon blockade in a hybrid micromaser system

Abstract

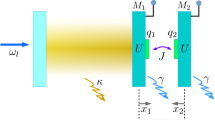
In a hybrid micromaser system consisting of an optical cavity with a moving mirror connected to a low-temperature thermal bath, we demonstrate, both analytically and numerically, that for certain interaction times between a random atomic flux and the optomechanical cavity, vacuum phonon trapping states are generated. Furthermore, under the approach of the master equation with independent phonon and photon thermal baths, we show that the trapping of the phonons and photons is achieved for the same interaction times. The results also indicate that by increasing the cavity-oscillator coupling, one may generate a coherent phonon state aside from the trapping states. Within the same hybrid system, but now connected to the squeezed phonon reservoir, a phonon blockade effect can be engineered. Moreover, we identify an interconnection between the trapping and blockade effects, particularly if one approaches the vacuum trapping state, strong phonon blockade can be achieved when the system is connected with a weakly squeezed phonon reservoir.

Appendices

Appendix A: Hamiltonian in the interaction picture

This appendix provides the derivation of the initial Hamiltonian Eq. 1 in the interaction picture. Let us introduce the operator counting the number of atom-cavity polaritonic excitations:

$$\begin{aligned} \hat{\mathcal {N}}={\hat{a}}^{\dag }{\hat{a}}+{\hat{\sigma }}_{z}/2 \end{aligned}$$

(A1)

We observe that this polariton number operator commutes with the Hamiltonian of the system, $[\hat{\mathcal {H}},\hat{\mathcal {N}}]=0$. Therefore, the Hamiltonian of the closed system is block-diagonal in the basis of eigenvectors of the polariton number operator. By considering the detuning $\delta =\omega _{a}-\omega _{c}$, the Hamiltonian in Eq. 1 can be written as

$$\begin{aligned} \hat{\mathcal {H}}=\hat{\mathcal {H}}_{0}+\hat{\mathcal {H}}_{I}, \end{aligned}$$

(A2)

where

$$\begin{aligned} \hat{\mathcal {H}}_{0}&=\omega _{m}{\hat{b}}^{\dag }{\hat{b}},\nonumber \\ \hat{\mathcal {H}}_{I}&=\delta \frac{{\hat{\sigma }}_{z}}{2}+ g_{ac}\left( {\hat{a}}{\hat{\sigma }}_{+}+{\hat{a}}^{\dag }{\hat{\sigma }}_{-}\right) -g_{cm}{\hat{a}}^{\dag }{\hat{a}}\left( {\hat{b}}^{\dag }+{\hat{b}}\right) . \end{aligned}$$

(A3)

Now, we calculate the Hamiltonian in the first interaction picture, that is

$$\begin{aligned} \hat{\mathcal {H}}'=e^{i \hat{\mathcal {H}}_{0} t}\hat{\mathcal {H}}_{I}e^{-i \hat{\mathcal {H}}_{0} t}. \end{aligned}$$

(A4)

Using the fact that

$$\begin{aligned} \mathcal {{\hat{U}}}f\left( \left\{ \mathcal {{\hat{X}}}_{i}\right\} \right) \mathcal {{\hat{U}}}^{\dag } =f\left( \left\{ \mathcal {{\hat{U}}}\mathcal {{\hat{X}}}_{i}{\hat{U}}^{\dag }\right\} \right) , \end{aligned}$$

(A5)

for any function f, unitary operator $\mathcal {{\hat{U}}}$ and arbitrary set of operators $\left\{ \mathcal {{\hat{X}}}_{i}\right\}$, so Eq. A4 takes the form

$$\begin{aligned} \hat{\mathcal {H}}'=\hat{\mathcal {H}}'_{0}+\hat{\mathcal {H}}'_{I}, \end{aligned}$$

(A6)

where

$$\begin{aligned} \hat{\mathcal {H}}'_{0}&=\delta \frac{{\hat{\sigma }}_{z}}{2}+ g_{ac}\left( {\hat{a}}{\hat{\sigma }}_{+}+{\hat{a}}^{\dag }{\hat{\sigma }}_{-}\right) ,\nonumber \\ \hat{\mathcal {H}}'_{I}&=-g_{cm}{\hat{a}}^{\dag }{\hat{a}}\left( {\hat{b}}^{\dag }e^{i\omega _{m}t}+{\hat{b}}e^{-i\omega _{m}t}\right) . \end{aligned}$$

(A7)

Now, we move to a second interaction picture, defined as

$$\begin{aligned} \hat{\tilde{\mathcal {H}}}=\exp {\left[ i\int \hat{\mathcal {H}}'_{0}dt\right] }\hat{\mathcal {H}}'_{I}\exp {\left[ -i\int \hat{\mathcal {H}}'_{0}dt\right] }. \end{aligned}$$

(A8)

The result of the transformation is easily calculated to be finally Eq. 2

Appendix B: Phonon gain superoperator

This section presents the derivation of the gain part of the maser ME for the MO operator. We consider an initial atom-cavity-oscillator operator as

$$\begin{aligned} {\hat{\rho }}(0)=\begin{pmatrix} 0 \quad &\quad 0\\ 0 \quad&\quad 1 \end{pmatrix}\otimes {\hat{\rho }}_{c}(0)\otimes {\hat{\rho }}_{m}(0), \end{aligned}$$

(B9)

where the atom was taken in the ground state. After the interaction time $\tau$, the total density operator evolves according to Eq. 3. After a straightforward calculation, one gets

$$\begin{aligned} {\hat{\rho }}(\tau )= \begin{pmatrix} g_{ac}^{2}\mathcal {\mathcal {{\hat{S}}}}{\hat{a}}e^{i\mathcal {{\hat{F}}}}{\hat{\rho }}_{c}(0)\otimes {\hat{\rho }}_{m}(0)e^{-i\mathcal {{\hat{F}}}}{\hat{a}}^{\dag }\mathcal {\mathcal {{\hat{S}}}} &{} -i g_{ac}\mathcal {\mathcal {{\hat{S}}}}{\hat{a}}e^{i\mathcal {{\hat{F}}}}{\hat{\rho }}_{c}(0)\otimes {\hat{\rho }}_{m}(0)\mathcal {{\hat{D}}}^{\dag }\\ ig_{ac}\mathcal {{\hat{D}}}{\hat{\rho }}_{c}(0)\otimes {\hat{\rho }}_{m}(0)e^{-i\mathcal {{\hat{F}}}}{\hat{a}}^{\dag }\mathcal {\mathcal {{\hat{S}}}} &{} \mathcal {{\hat{D}}}{\hat{\rho }}_{c}(0)\otimes {\hat{\rho }}_{m}(0)\mathcal {{\hat{D}}}^{\dag } \end{pmatrix}, \end{aligned}$$

(B10)

where $\mathcal {{\hat{D}}}^{\dag }$ is hermitian conjugate of $\mathcal {{\hat{D}}}$, see Eq. 5.

Next, tracing over the atom, the cavity-MO reduced operator reads

$$\begin{aligned} {\hat{\rho }}_{c,m}(\tau ) = {\hat{\rho }}_{m}(0)\mathcal {{\hat{D}}}{\hat{\rho }}_{c}(0)\mathcal {{\hat{D}}}^{\dag }+ g^{2}_{ac}e^{i\mathcal {{\hat{F}}}}{\hat{\rho }}_{m}(0)e^{-i\mathcal {{\hat{F}}}}\mathcal {\mathcal {{\hat{S}}}}{\hat{a}}{\hat{\rho }}_{c}(0){\hat{a}}^{\dag }\mathcal {\mathcal {{\hat{S}}}}. \end{aligned}$$

(B11)

In the following, we consider the cavity field initialized in a coherent state (Eq. 8). Hence, the above equation becomes

$$\begin{aligned} {\hat{\rho }}_{c,m}(\tau )= & {} {\hat{\rho }}_{m}(0)e^{-\vert \alpha \vert ^{2}}\sum _{n,m}\frac{\alpha ^{n}\alpha ^{*m}}{\sqrt{n!m!}}D_{n}D_{m}^{*}\vert n\rangle \langle m\vert \nonumber \\+ & {} g^{2}_{ac}e^{i\mathcal {{\hat{F}}}}{\hat{\rho }}_{m}(0)e^{-i\mathcal {{\hat{F}}}}e^{-\vert \alpha \vert ^{2}}\sum _{n,m}\frac{\alpha ^{n}\alpha ^{*m}}{\sqrt{n!m!}}\sqrt{nm}\nonumber \\\times & {} S_{n-1}S_{m-1}\vert n-1\rangle \langle m-1\vert , \end{aligned}$$

(B12)

where

$$\begin{aligned} D_{n}&=\cos {\left( \sqrt{\varphi _{n}}\tau \right) }+\frac{i\delta }{2}\frac{\sin {\left( \tau \sqrt{\varphi _{n}}\right) }}{\sqrt{\varphi _{n}}},\end{aligned}$$

(B13)

$$\begin{aligned} S_{n-1}&=\frac{\sin {\left( \tau \sqrt{\varphi _{n-1}+g_{ac}^{2}}\right) }}{\sqrt{\varphi _{n-1}+g_{ac}^{2}}}. \end{aligned}$$

(B14)

Finally tracing over the cavity, we find Eqs. 10, 11 and 12.

Appendix C: Solution of the Fokker-Planck equation

Here, we present the steps to get the solution 16 of the Fokker-Planck equation 15. Let us consider the MO initially is in a coherent state, i.e., in the Gaussian representation takes the form

$$\begin{aligned} P(\beta ,\beta ^{*},0)=(\pi \epsilon )^{-1}\exp {\left[ -\vert \beta -\beta _{0}\vert ^{2}/\epsilon \right] } \end{aligned}$$

(C15)

By considering a solution type $P(\beta ,\beta ^{*},t)=\exp {\left[ a(t)+b(t)\beta +c(t)\beta ^{*}+d(t)\beta \beta ^{*}\right] }$, one obtains a set of first-order differential equations

$$\begin{aligned} {\dot{a}}(t)&=\kappa _{b}\left( d(t)+{\bar{n}}_{th}d^{2}(t)\right) ,\end{aligned}$$

(C16)

$$\begin{aligned} {\dot{b}}(t)&=\kappa _{b}\left( \frac{1}{2}c(t)+{\bar{n}}_{th}c(t)d(t)\right) -\lambda B(\tau )rd(t),\end{aligned}$$

(C17)

$$\begin{aligned} {\dot{c}}(t)&=\kappa _{b}\left( \frac{1}{2}b(t)+{\bar{n}}_{th}b(t)d(t)\right) -\lambda B(\tau )rd(t),\end{aligned}$$

(C18)

$$\begin{aligned} {\dot{d}}(t)&=\kappa _{b}\left( 1+{\bar{n}}_{th}[c(t)b(t)+d(t)]\right) -\lambda B(\tau )r[c(t)+b(t)]. \end{aligned}$$

(C19)

For an initial thermal distribution, $\epsilon ={\bar{n}}_{th}$, $\beta _{0}=0$ and after a straightforward calculation, we get

$$\begin{aligned} P(\beta ,\beta ^{*},t) = \frac{1}{\pi {\bar{n}}_{th}}\text {exp}\left[ \text {ln}[\pi {\bar{n}}_{th}]-\frac{\beta _{1}^{2}}{{\bar{n}}_{th}}-\frac{\vert \beta \vert ^{2}}{{\bar{n}}_{th}}+\frac{\beta _{1}\beta }{{\bar{n}}_{th}}+\frac{\beta _{1}\beta ^{*}}{{\bar{n}}_{th}}\right] , \end{aligned}$$

(C20)

with $\beta _{1}=2\lambda r B(\tau )\kappa _{b}^{-1}(1-\exp {\left[ -\kappa _{b}t/2\right] })$. Finally, the last equation can be written compactly as Eq. 16.

Appendix D: Phonon trapping condition

The critical values of the pump parameter, $\Theta$, where the phonon trapping vacuum states occur, are calculated from the condition of the minimum value for the steady-state average phonon number: $\frac{\partial }{\partial \Theta }\langle {\hat{b}}^{\dag }{\hat{b}}\rangle =0$ with $\frac{\partial ^{2}}{\partial \Theta ^{2}}\langle {\hat{b}}^{\dag }{\hat{b}}\rangle >0$. So the derivative of Eq. 18 gives

$$\begin{aligned} \frac{\partial }{\partial \Theta }\langle {\hat{b}}^{\dag }{\hat{b}}\rangle= & {} \frac{8e^{-2\vert \alpha \vert ^{2}}g_{ac}\lambda ^{2}r^{3/2}}{\kappa _{b}^{2}\omega _{m}^{1/2}} \sum _{n}\frac{\vert \alpha \vert ^{2(n+1)}\sqrt{n+1}}{(n+1)!}\sin {\left( 2g_{ac}\Theta \sqrt{\frac{n+1}{\omega _{m}r}}\right) }\nonumber \\\times & {} \sum _{n}\frac{\vert \alpha \vert ^{2(n+1)}}{(n+1)!}\sin {\left( g_{ac}\Theta \sqrt{\frac{n+1}{\omega _{m}r}}\right) }^{2}. \end{aligned}$$

(D21)

Taking into account that $\left\{ \alpha ,g_{ac},r,\lambda ,\kappa _{b}\right\} >0$, the phonon trapping state is valid for

$$\begin{aligned} \sum _{n}\frac{\vert \alpha \vert ^{2(n+1)}\sqrt{n+1}}{(n+1)!}\sin {\left( 2g_{ac}\Theta \sqrt{\frac{n+1}{\omega _{m}r}}\right) }=0. \end{aligned}$$

(D22)

Finally, the above equation can be solved numerically, and the minimal values for $\langle {\hat{b}}^{\dag }{\hat{b}}\rangle$ are found, see vertical lines in Fig. 2b.

Appendix E: Calculation of $g_{b}^{(2)}(0)$

To calculate the second-order correlation function for phonons, $g_{b}^{(2)}(0)=\langle {\hat{b}}^{\dag }{\hat{b}}^{\dag }{\hat{b}}{\hat{b}}\rangle /\langle {\hat{b}}^{\dag }b\rangle ^{2}$, we consider convenient to use the moment-generating function

$$\begin{aligned} \mathcal {Q}(s)=\sum _{n=0}^{\infty }(1-s)^{n}P(n), \end{aligned}$$

(E23)

where $P(n)=\int d^{2}\beta P(\beta ,\beta ^{*},t)\vert \langle n\vert \beta \rangle \vert ^{2}$ is the probability to have n phonons in the MO and $P(\beta ,\beta ^{*},t)$ is defined in Eq. 16. After a straightforward calculation, one obtains

$$\begin{aligned} P(n)= \frac{1}{\pi {\bar{n}}_{th}n!} \int d^{2}\beta \vert \beta \vert ^{2n} e^{-\vert \beta \vert ^{2}-\frac{\vert \beta -\beta _{1}\vert ^{2}}{{\bar{n}}_{th}}}. \end{aligned}$$

(E24)

Finally, using the definition $g^{(2)}(0)=\frac{1}{\langle n\rangle }\frac{d^{2}\mathcal {Q}}{ds^{2}}\vert _{s=0}$, see [3], we get Eq. 23.