Propagation of dust acoustic waves in multi-components plasma with random parameters

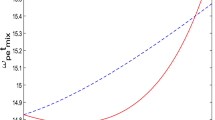
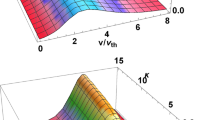
Abstract

In this article, contrary to what is known previously, a randomized dusty plasma model is introduced. In this new issue, the uncertain properties of the plasma nonlinear system are assumed as continuous random variables. Hence, a comprehensive probabilistic behavior of the interesting characteristics of the dusty plasma wave is presented through computing the first probability density functions and consequently the means, the variances and the confidence intervals. For this goal, a probabilistic transformational technique that is known as the random variable transformation is implemented. The obtained theoretical findings are investigated via some numerical results that showed acceptable consistency with the physical situations.

Appendices

Appendix

Deterministic model

2.1 Scaled system of nonlinear differential equations

The system of coupled nonlinear partial differential Eqs. (1–3) before scaling takes, respectively, the form [1, 2];

$$ \frac{{\partial N_{d} }}{\partial \tau } + \frac{\partial }{\partial \xi }(N_{d} U_{d} ) = 0, $$

(53)

$$ \frac{{\partial U_{d} }}{\partial \tau } + U_{d} \frac{{\partial U_{d} }}{\partial \xi } = \frac{{eZ_{d} }}{{m_{d} }}\frac{\partial \Phi }{{\partial \xi }}, $$

(54)

$$ \frac{{\partial^{2} \Phi }}{{\partial \xi^{2} }} = 4\pi e(Z_{d} N_{d} + N_{e} - N_{i} ). $$

(55)

In addition, Eq. (4) before scaling is

$$ N_{e} = n_{e0} \exp \left( {\frac{e\Phi }{{k_{B} T_{e} }}} \right),N_{i} = n_{i0} \exp \left( { - \frac{e\Phi }{{k_{B} T_{i} }}} \right). $$

(56)

In Eqs. (53–56), if the scaled variables $n_{d} = \frac{{N_{d} }}{{n_{d0} }},\,$$\,n_{i} = \frac{{N_{i} }}{{Z_{d} n_{d0} }},$$\,n_{e} = \frac{{N_{e} }}{{Z_{d} n_{d0} }},\,$$u_{d} = \frac{{U_{d} }}{{C_{d} }},$ $\phi = \frac{e\Phi }{{k_{B} T_{i} }},$ $x = \frac{\xi }{{\lambda_{D} }}$ and $t = \tau \omega_{pd} ,$ are introduced; we get, with some algebraic manipulations, Eqs. (1–4), where $n_{d0}$ is the unperturbed negative dust density, $C_{d} = (Z_{d} k_{B} T_{i} /m_{d} )^{1/2}$ is the negative dust sound speed and $m_{d} ,\,Z_{d}$, $e$, and $k_{B}$ are the dust mass, charge number, the electron charge and Boltzmann constant, respectively. In addition, $\lambda_{D} = \left( {k_{B} T_{i} /4\pi Z_{d} e^{2} n_{d0} } \right)^{1/2}$ is the negative dust Debye radius and $\omega_{pd}^{ - 1} = (m_{d} /4\pi Z_{d}^{2} e^{2} n_{d0} )^{1/2}$ is the negative ion plasma period.

2.2 Deterministic solution

The KdV equation, Eq. (13), can be derived from Eqs. (1–4), by employing the reductive perturbation method (RPM) [18]. In this method, the independent variables are stretched to $X = {\epsilon}^{1/2} (x - v_{0} t),\,\,\,T = {\epsilon}^{3/2} t$, and hence

$$ \frac{\partial }{\partial x} = \epsilon^{1/2} \frac{\partial }{\partial X},\frac{\partial }{\partial t} = \epsilon^{1/2} { - v_{0} \frac{\partial }{\partial X} + \epsilon \frac{\partial }{\partial T}}, $$

(57)

where $\epsilon$ is a small (real) parameter and $v_{0}$ is the phase velocity. The dependent variables are expanded according to Eq. (9). Substituting the variable stretching (57) and the expansions (9) in Eqs. (1–3), we get the following:

• (i) For the continuity Eq. (1)

$$ \begin{gathered} \epsilon^{1/2} \left( { - v_{0} \frac{\partial }{\partial X} + \epsilon \frac{\partial }{\partial T}} \right)(1 + \epsilon n_{d}^{(1)} + \epsilon^{2} n_{d}^{(2)} + ...) \hfill \\ + \epsilon^{1/2} \frac{\partial }{\partial X}(1 + \epsilon n_{d}^{(1)} + \epsilon^{2} n_{d}^{(2)} + ...)(\epsilon u_{d}^{(1)} + \epsilon^{2} u_{d}^{(2)} + ...) = 0. \hfill \\ \end{gathered} $$

(58)

Equating the coefficients of the first and the second orders of $\epsilon$ in (58) to zero gives, respectively,

$$ v_{0} \frac{{\partial n_{d}^{(1)} }}{\partial X} = \frac{{\partial u_{d}^{(1)} }}{\partial X}, $$

(59)

$$ v_{0} \frac{{\partial n_{d}^{(2)} }}{\partial X} = \frac{{\partial n_{d}^{(1)} }}{\partial T} + \frac{{\partial u_{d}^{(2)} }}{\partial X} + n_{d}^{(1)} \frac{{\partial u_{d}^{(1)} }}{\partial X} + u_{d}^{(1)} \frac{{\partial n_{d}^{(1)} }}{\partial X}. $$

(60)

• (ii) For the momentum Eq. (2)

$$ \begin{gathered} \epsilon^{1/2} \left( { - v_{0} \frac{\partial }{\partial X} + \epsilon \frac{\partial }{\partial T}} \right)(\epsilon u_{d}^{(1)} + \epsilon^{2} u_{d}^{(2)} + .) \hfill \\ + (\epsilon u_{d}^{(1)} + \epsilon^{2} u_{d}^{(2)} + ..)\epsilon^{1/2} \frac{\partial }{\partial X}(\epsilon u_{d}^{(1)} + \epsilon^{2} u_{d}^{(2)} + ...) \hfill \\ = \epsilon^{1/2} \frac{\partial }{\partial X}(\epsilon \phi^{(1)} + \epsilon^{2} \phi^{(2)} + ...). \hfill \\ \end{gathered} $$

(61)

Equating the first and the second orders of $\epsilon$ to zero gives, respectively,

$$ - v_{0} \frac{{\partial u_{d}^{(1)} }}{\partial X} = \frac{{\partial \phi^{(1)} }}{\partial X}, $$

(62)

$$ - v_{0} \frac{{\partial u_{d}^{(2)} }}{\partial X} + \frac{{\partial u_{d}^{(1)} }}{\partial T} = \frac{{\partial \phi^{(2)} }}{\partial X} - u_{d}^{(1)} \frac{{\partial u_{d}^{(1)} }}{\partial X}. $$

(63)

• (iii) In view of Eq. (4), the Poisson’s Eq. (3) can be rewritten as

$$ \frac{{\partial^{2} \phi }}{{\partial x^{2} }} = n_{d} + \mu_{e} (1 + \alpha \phi + \frac{1}{2}\alpha^{2} \phi^{2} + ...) - \mu_{i} (1 - \phi + \;\;\frac{1}{2}\phi^{2} - ...). $$

(64)

Substituting the variable stretching (57) and the expansions (9) in (64), we get

$$ \begin{gathered} \epsilon \frac{{\partial^{2} }}{{\partial X^{2} }}(\epsilon \phi^{(1)} + \epsilon^{2} \phi^{(2)} + ...) = (1 + \epsilon n_{d}^{(1)} + \epsilon^{2} n_{d}^{(2)} + ...) + \mu_{e} [1 + \alpha (\epsilon \phi^{(1)} + \epsilon^{2} \phi^{(2)} + ...) \hfill \\ + \frac{1}{2}\alpha^{2} (\epsilon \phi^{(1)} + \epsilon^{2} \phi^{(2)} + ...)^{2} + ...] - \mu_{i} [1 - (\epsilon \phi^{(1)} + \epsilon^{2} \phi^{(2)} + ...) \hfill \\ + \frac{1}{2}(\epsilon \phi^{(1)} + \epsilon^{2} \phi^{(2)} + ...)^{2} - ...]. \hfill \\ \end{gathered} $$

For the zero order of $\epsilon$, we have the neutrality condition:

$$ 1 + \mu_{e} - \mu_{i} = 0. $$

(65)

Equating the coefficients of the first and the second orders of $\epsilon$ to zero gives, respectively,

$$ n_{d}^{(1)} + \mu_{e} \alpha \phi^{(1)} + \mu_{i} \phi^{(1)} = 0, $$

(66)

$$ \frac{{\partial^{2} \phi^{(1)} }}{{\partial X^{2} }} = n_{d}^{(2)} + \alpha \mu_{e} \phi^{(2)} + \frac{1}{2}\alpha^{2} \mu_{e} \left[ {\phi^{(1)} } \right]^{2} \;\; + \mu_{i} \phi^{(2)} - \frac{1}{2}\mu_{i} \left[ {\phi^{(1)} } \right]^{2} . $$

(67)

Integrating (59) and (62), under the boundary conditions: $\,\,u(X) \to 0,$ $\phi^{(1)} (X) \to 0$ at $|X| \to \infty ,$ gives

$$ n_{d}^{(1)} = - \frac{1}{{v_{0}^{2} }}\phi^{(1)} , \, u_{d}^{(1)} = - \frac{1}{{v_{0} }}\phi^{(1)} . $$

(68)

Substituting the value of $n_{d}^{(1)}$ in (66) provides the compatibility condition

$$ \mu_{e} \alpha + \mu_{i} - \frac{1}{{v_{0}^{2} }} = 0. $$

This leads to the following expression for the phase velocity

$$ v_{0} = \left( {\frac{1}{{\mu_{e} \alpha + \mu_{i} }}} \right)^{1/2} . $$

(69)

Delete the term $\frac{{\partial u_{d}^{(2)} }}{\partial X}$ between Eqs. (60) and (63) to get

$$ \begin{gathered} - v_{0}^{2} \frac{{\partial n_{d}^{(2)} }}{\partial X} + v_{0} \frac{{\partial n_{d}^{(1)} }}{\partial T} + v_{0} n_{d}^{(1)} \frac{{\partial u_{d}^{(1)} }}{\partial X} + v_{0} u_{d}^{(1)} \frac{{\partial n_{d}^{(1)} }}{\partial X} \hfill \\ + \frac{{\partial u_{d}^{(1)} }}{\partial T} - \frac{{\partial \phi^{(2)} }}{\partial X} + u_{d}^{(1)} \frac{{\partial u_{d}^{(1)} }}{\partial X} = 0. \hfill \\ \end{gathered} $$

(70)

Substitute $n_{d}^{(1)}$ and $u_{d}^{(1)}$ from (68) in (70) to get

$$ \frac{{\partial n_{d}^{(2)} }}{\partial X} + \frac{2}{{v_{0}^{3} }}\frac{{\partial \phi^{(1)} }}{\partial T} - \frac{3}{{v_{0}^{4} }}\phi^{(1)} \frac{{\partial \phi^{(1)} }}{\partial X} + \frac{1}{{v_{0}^{2} }}\frac{{\partial \phi^{(2)} }}{\partial X} = 0. $$

(71)

Differentiate (67) to get

$$ \frac{{\partial n_{d}^{(2)} }}{\partial X} = \frac{{\partial^{3} \phi^{(1)} }}{{\partial X^{3} }} - \alpha \mu_{e} \frac{{\partial \phi^{(2)} }}{\partial X} - \alpha^{2} \mu_{e} \phi^{(1)} \frac{{\partial \phi^{(1)} }}{\partial X}\;\; - \mu_{i} \frac{{\partial \phi^{(2)} }}{\partial X} + \mu_{i} \phi^{(1)} \frac{{\partial \phi^{(1)} }}{\partial X}. $$

Substitute $\frac{{\partial n_{d}^{(2)} }}{\partial X}$ in Eq. (71) and arrange the terms to get the required KdV equation:

$$ \frac{{\partial \phi^{(1)} }}{\partial T} + \frac{{v_{0}^{3} }}{2}\left( { - \frac{3}{{v_{0}^{4} }} + \mu_{i} - \alpha^{2} \mu_{e} } \right)\phi^{(1)} \frac{{\partial \phi^{(1)} }}{\partial X} + \frac{{v_{0}^{3} }}{2}\frac{{\partial^{3} \phi^{(1)} }}{{\partial X^{3} }} = 0, $$

(72)

Or

$$ \frac{{\partial \phi^{(1)} }}{\partial T} + A\phi^{(1)} \frac{{\partial \phi^{(1)} }}{\partial X} + B\frac{{\partial^{3} \phi^{(1)} }}{{\partial X^{3} }} = 0, $$

(73)

where the coefficients of nonlinear and dispersion terms are given by Eq. (14).

In order to solve the KdV (73), analytically, we introduce the transformation $\zeta = X - u_{0} T$, where $\zeta$ is the transformed coordinate with respect to a frame moving with a speed $u_{0}$ normalized to $C_{d} .$ Setting $\phi^{(1)} (X,T) \equiv u(\zeta )$ in (73) and integrating the resulting equation under the boundary conditions (15) lead to Eq. (16). Multiply (16) by $\frac{du(\zeta )}{{d\zeta }}$ and integrate to get

$$ \frac{{ - u_{0} }}{2B}u^{2} + \frac{A}{6B}u^{3} + \frac{1}{2}\left( {\frac{du}{{d\zeta }}} \right)^{2} = 0. $$

or

$$ \frac{du}{{d\zeta }} = u\sqrt {\frac{{u_{0} }}{B} - \frac{A}{3B}u} . $$

(74)

Equation (74) is a separable first-order differential equation that can be easily solved to get the solution (17).