q-Cat states revisited: two families in a Fock representation space of q-oscillator algebra with different nonclassical behaviors

Abstract

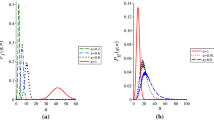
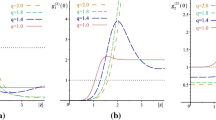
This paper has been motivated by our previous paper (Eur. Phys J Plus 135:253, 2020) on q-coherent states of the Arik–Coon q-oscillator. The quadratic powers of unbounded and bounded annihilation operators associated with the Arik–Coon q-oscillator are used to obtain two different families of the even and odd q-cat states for $0<q<1$ in even and odd subspaces of the Fock representation space of the system. The resolutions of the identity condition for q-cat states of the unbounded and bounded annihilation operators are realized by two appropriate positive definite q-measures in the q-integral on the whole complex plane and a disk in radius $1/\sqrt{1-q}$, respectively. It is shown that the antibunching effect and sub-Poissonian statistics as well as the bunching effect and super-Poissonian statistics are simultaneously exhibited by the first and second families of q-cat states, respectively. Otherwise, a fixed observation cannot be concluded. The strong and weak squeezing conditions for two different types of q-generalizations of the position and momentum quadratures, corresponding to the unbounded and bounded annihilation operators, on their associated even and odd q-cat states are considered. Using an example, we show that contrary to the odd q-cat states of the first family that exhibit both strong and weak squeezing effects by both quadratures, the ones of the second family demonstrate only the weak squeezing.

Appendix A: q-calculus

For $0<q<1$, the two known q-generalized exponential functions are

$$\begin{aligned}&{E_q(x)}:=\sum _{n=0}^{\infty }\frac{q^\frac{n(n-1)}{2}}{(q;q)_n}{x^n}, \end{aligned}$$

(A1a)

$$\begin{aligned}&{e_q(x)}:=\sum _{n=0}^{\infty }\frac{x^n}{(q;q)_n}, \end{aligned}$$

(A1b)

in which, the definition of q-Pochhammer symbol as $(a;q)_n:=(1-a)(1-aq)(1-aq^2)\cdots (1-aq^{n-1})$ for a nonzero complex number such as a has been used. Their convergence regions are the intervals $|x|<\infty$ and $|x|<1$, respectively, with an infinite product expansion for the second q-exponential function as $e_{q}(x)=1/\prod _{k=0}^\infty (1-q^{k} x)$ [58]. Also, they satisfy the relations ${E_q(x)}={e_{q^{-1}}(-{q^{-1}} x)}$, $e_q(x)E_q(-x)=1$, $\lim _{q\rightarrow 1} {e_q((1-q)x)}=\exp (x)$ and $\lim _{q\rightarrow 1}{E_q((1-q)x)}=\exp (x)$ [51, 59]. We fix the definition of q-hyperbolic functions $\sinh$ and $\cosh$ by using (A1a), as below

$$\begin{aligned}&{{\sinh _q}x}:=\frac{E_q((1-q)x) - E_q(-(1-q)x)}{2}, \end{aligned}$$

(A2a)

$$\begin{aligned}&{{\cosh _q}x}:=\frac{E_q((1-q)x) + E_q(-(1-q)x)}{2}. \end{aligned}$$

(A2b)

Moreover, the asymmetric and symmetric q-derivatives $D_{q,x}$ and ${\widetilde{D}}_{q,x}$ on an arbitrary continuous function such as f(x) are defined as

$$\begin{aligned}&D_{q,x}f(x)=\frac{f(x)-f(qx)}{(1-q)x}, \quad \end{aligned}$$

(A3a)

$$\begin{aligned}&{\widetilde{D}}_{q,x}f(x):=\frac{f(q x)-f(q^{-1}x)}{(q-q^{-1})x}. \quad \end{aligned}$$

(A3b)

Their relative q-analogues of Leibniz formula are

$$\begin{aligned}&{D_{q,x}}(f(x) g(x))=({D_{q,x}}f(x))\, g(qx)+f(x)\,D_{q,x}g(x) \quad \nonumber \\&\quad =({D_{q,x}}f(x))\, g(x)+f(qx)\,D_{q,x}g(x), \quad \end{aligned}$$

(A4a)

$$\begin{aligned}&{\widetilde{D}}_{q,x}(f(x) g(x))=({\widetilde{D}}_{q,x}f(x))\,g(q^{-1}x)+f(q x)\,{\widetilde{D}}_{q,x}g(x) \quad \nonumber \\&\quad =({\widetilde{D}}_{q,x}f(x))\,g(q x)+f(q^{-1} x)\,{\widetilde{D}}_{q,x}g(x).&\end{aligned}$$

(A4b)

Using the q-derivatives ${D_{q,x}}$ and ${\widetilde{D}}_{q,x}$ as the inverse operations, the q-integrals on the intervals $[0,\infty )$ and [0, c] with c as a positive real number are defined, respectively, as [51, 59]

$$\begin{aligned}&\int _{0}^{\infty }f(x)d_{q}x:=(1-q)\sum _{j=-\infty }^{\infty }q^j{f(q^j)}, \quad \end{aligned}$$

(A5a)

$$\begin{aligned}&\int _{0}^{c}f(x)d_{q}x:=(1-q)c\sum _{j=0}^{\infty }q^j{f(q^j{c})}, \quad \end{aligned}$$

(A5b)

and [60]

$$\begin{aligned}&\int _0^{\infty }f(x)\widetilde{d_q}x=(q^{-1}-q)\sum _{j=-\infty }^{\infty }q^{2j+1}f(q^{2j+1}), \quad \end{aligned}$$

(A6a)

$$\begin{aligned}&\int _0^{c}f(x)\widetilde{d_q}x=(q^{-1}-q)c\sum _{j=0}^{\infty }q^{2j+1}f(q^{2j+1}c). \quad \end{aligned}$$

(A6b)

For $0<q<1$, as two different generalizations of the integral representation of the ordinary factorial, the infinite and finite q-integral representations of the q- and $q^2$-factorials are obtained, respectively, as below [31, 32, 60]

$$\begin{aligned}&\int _{0}^{\infty }\frac{x^n}{E_{q^2}((q^{-1}-q)x)}{\widetilde{d}}_{q}x=q^{-n^2}[n]_{q^2}!, \quad \end{aligned}$$

(A7a)

$$\begin{aligned}&\int _{0}^{\frac{1}{1-q}}\frac{x^n}{{e_q}((1-q)x)}d_{q}x=[n]_q!. \quad \end{aligned}$$

(A7b)

The q-factorial is defined as $[0]_q!=1$ and $[n]_q!:=[1]_q [2]_q \cdots [n]_q$ with n as a positive integer number. One must note that the quantum number introduced in [60] is symmetric, and here it is written in terms of the asymmetric quantum number.