Skip to main content
SpringerLink
Log in

Numerical analysis of Galerkin meshless method for parabolic equations of tumor angiogenesis problem

  • Regular Article
  • Published:
The European Physical Journal Plus Aims and scope Submit manuscript

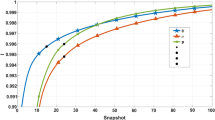
Abstract

The stability and convergence of the Galerkin method for differential equations with symmetric operators have been confirmed with numerical results, while this is not the case when dealing with unsymmetric operators. In the present study, a sort of transformation is used as a preconditioner to convert the unsymmetric operator to a symmetric one. This method is implemented on the capillary formation mathematical model of tumor angiogenesis problem. Then, a Galerkin meshfree method based on the radial basis functions is presented for the numerical solution of this problem. The proposed strategy is based on applying the Galerkin method and group preserving scheme for the spatial and time variables, respectively. Also, the stability and the convergence of proposed method is considered. In addition, some of the advantages of the proposed technique over existing methods are shown. Finally, some numerical results will be provided to validate the theoretical achievements.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

References

  1. K. Zennir, T. Miyasita, Lifespan of solutions for a class of pseudo-parabolic equation with weak-memory. Alex. Eng. J. 59(2), 957–964 (2020)

    Article  Google Scholar 

  2. N.A. Mbroh, S.C.O. Noutchie, R.Y.M.P. Massoukou, A robust method of lines solution for singularly perturbed delay parabolic problem. Alex. Eng. J. 59(4), 2543–2554 (2020)

    Article  Google Scholar 

  3. Z. Korpinar, M. Inc, E. Hncal, D. Baleanu, Residual power series algorithm for fractional cancer tumor models. Alex. Eng. J. 59(3), 1405–1412 (2020)

    Article  Google Scholar 

  4. H.M. Srivastava, U. Dey, A. Ghosh, J.P. Tripathi, S. Abbas, A. Taraphder, M. Roy, Growth of tumor due to Arsenic and its mitigation by black tea in Swiss albino mice. Alex. Eng. J. 59(3), 1345–1357 (2020)

    Article  Google Scholar 

  5. A.M. Mishra, S.D. Purohit, K.M. Owolabi, Y.D. Sharma, A nonlinear epidemiological model considering asymptotic and quarantine classes for SARS CoV-2 virus. Chaos Solitons Fractals 138, 109953 (2020)

    Article  ADS  MathSciNet  Google Scholar 

  6. K.M. Owolabi, A. Shikongo, Fractional operator method on a multi-mutation and intrinsic resistance model. Alex. Eng. J. 59(4), 1999–2013 (2020)

    Article  Google Scholar 

  7. K.M. Owolab, K.C. Patidar, Shikongo, A, Mathematical analysis and numerical simulation of a tumor-host model with chemotherapy application. Commun. Math. Biol. Neurosci. 1, 1–34 (2018)

    Google Scholar 

  8. K.M. Owolabi, K.C. Patidar, A. Shikongo, A fitted numerical method for a model arising in HIV related cancer-immune system dynamics. Commun. Math. Biol. Neurosci. 1, 1–23 (2019)

    Google Scholar 

  9. K.M. Owolabi, K.C. Patidar, A. Shikongo, A fitted operator method for tumor cells dynamics in their micro-environment. Commun. Math. Biol. Neurosci. 1, 1–44 (2019)

    Google Scholar 

  10. K.M. Owolabi, K.C. Patidar, A. Shikongo, A fitted operator method for a model arising in vascular tumor dynamics. Commun. Math. Biol. Neurosci. 1, 1–24 (2020)

    Google Scholar 

  11. K.M. Owolabi, K.C. Patidar, A. Shikongo, Numerical solution for a problem arising in angiogenic signalling. AIMS Math. 4(1), 43–63 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  12. H.A. Levine, S. Pamuk, B.D. Sleeman, M. Nilsen-Hamilton, Mathematical modeling of capillary formation and development in tumor angiogenesis: penetration into the stroma. Bull. Math. Biol. 63(5), 801–863 (2001)

    Article  MATH  Google Scholar 

  13. B. Davis, Reinforced random walk. Probab. Theory Related Fields 84(2), 203–229 (1990)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  14. A. Stevens, H.G. Othmer, Aggregation, blowup, and collapse: the ABC’s of taxis in reinforced random walks. SIAM J. Appl. Math. 57(4), 1044–1081 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  15. A. Saadatmandi, M. Dehghan, Numerical solution of a mathematical model for capillary formation in tumor angiogenesis via the tau method. Int. J. Numer. Method Biomed. Eng. 24(11), 1467–1474 (2008)

    MathSciNet  MATH  Google Scholar 

  16. S. Abbasbandy, H.R. Ghehsareh, I. Hashim, Numerical analysis of a mathematical model for capillary formation in tumor angiogenesis using a meshfree method based on the radial basis function. Eng. Anal. Bound. Elem. 36(12), 1811–1818 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  17. N. Gücüyenen, G. Tanoĝlu, Iterative operator splitting method for capillary formation model in tumor angiogenesis problem: Analysis and application. Int. J. Numer. Method Biomed. Eng. 27(11), 1740–1750 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  18. S. Pamuk, Qualitative analysis of a mathematical model for capillary formation in tumor angiogenesis. Math. Model Method Appl. Sci. 13(01), 19–33 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  19. S. Pamuk, A. Erdem, The method of lines for the numerical solution of a mathematical model for capillary formation: the role of endothelial cells in the capillary. Appl. Math. Comput. 186(1), 831–835 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  20. S. Pamuk, A mathematical model for capillary formation and development in tumor angiogenesis: a review. Chemotherapy 52(1), 35–37 (2006)

    Article  Google Scholar 

  21. E. Shivanian, A. Jafarabadi, Capillary formation in tumor angiogenesis through meshless weak and strong local radial point interpolation. Eng. Comput. 34(3), 603–619 (2018)

    Article  Google Scholar 

  22. K. Kormann, E. Larsson, A Galerkin radial basis function method for the Schrödinger equation. SIAM J. Scient. Comput. 35(6), 2832–2855 (2017)

    Article  MATH  Google Scholar 

  23. R.B. Lehoucq, S.T. Rowe, A radial basis function Galerkin method for inhomogeneous nonlocal diffusion. Comput. Methods Appl. Mech. Eng. 299, 366–380 (2016)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  24. A. Ortiz-Bernardin, A. Russo, N. Sukumar, Consistent and stable meshfree Galerkin methods using the virtual element decomposition. Int. J. Numer. Methods Eng. 112(7), 655–684 (2017)

    Article  MathSciNet  Google Scholar 

  25. V. Thomée, Galerkin Finite Element Methods for Parabolic Problems, vol. 1054 (Springer, Berlin, 1984)

    MATH  Google Scholar 

  26. H. Wendland, Meshless Galerkin methods using radial basis functions. Math. Comput. 68(228), 1521–1531 (1999)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  27. Y.C. Chiang, D.L. Young, J. Sladek, V. Sladek, Local radial basis function collocation method for bending analyses of quasicrystal plates. Appl. Math. l Model. 50, 463–483 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  28. M. Dehghan, M. Abbaszadeh, The space-splitting idea combined with local radial basis function meshless approach to simulate conservation laws equations. Alex. Eng. J. 57(2), 1137–1156 (2018)

    Article  Google Scholar 

  29. K. Parand, M. Hemami, Numerical study of astrophysics equations by meshless collocation method based on compactly supported radial basis function. Int. J. Appl. Comput. Math. 3(2), 1053–1075 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  30. L. Wang, Z. Wang, Z. Qian, A meshfree method for inverse wave propagation using collocation and radial basis functions. Comput. Methods Appl. Mech. Eng. 322, 311–350 (2017)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  31. M. Dehghan, V. Mohammadi, A numerical scheme based on radial basis function finite difference (RBF-FD) technique for solving the high-dimensional nonlinear Schrödinger equations using an explicit time discretization: Runge-Kutta method. Comput. Phys. Commun. 217, 23–34 (2017)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  32. B. Martin, B. Fornberg, Using radial basis function-generated finite differences (RBF-FD) to solve heat transfer equilibrium problems in domains with interfaces. Eng. Anal. Bound. Elem. 79, 38–48 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  33. V. Shankar, The overlapped radial basis function-finite difference (RBF-FD) method: A generalization of RBF-FD. J. Comput. Phys. 342, 211–228 (2017)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  34. C.S. Liu, Cone of non-linear dynamical system and group preserving schemes. Int. J. Non. Linear Mech. 36(7), 1047–1068 (2001)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  35. C.J. Budd, A. Iserles, Geometric integration: numerical solution of differential equations on manifolds. Philos. Trans. R. Soc. Lond. A 357, 945–956 (1999)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  36. E. Hairer, Geometric integration of ordinary differential equations on manifolds. BIT Numer. Math. 41, 996–1007 (2001)

    Article  MathSciNet  Google Scholar 

  37. H.C. Lee, C.K. Chen, C.I. Hung, A modified group-preserving scheme for solving the initial value problems of stiff ordinary differential equations. Appl. Math. Comput. 133, 445–459 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  38. H.C. Lee, C.S. Liu, The fourth-order group preserving methods for the integrations of ordinary differential equations. Comput. Model. Eng. Sci. 41, 1–26 (2009)

    MathSciNet  MATH  Google Scholar 

  39. S. Abbasbandy, M.S. Hashemi, Group preserving scheme for the Cauchy problem of the Laplace equation. Eng. Anal. Bound. Elem. 35(8), 1003–1009 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  40. C.S. Liu, Group preserving scheme for backward heat conduction problems. Int. J. Heat Mass Transf. 47(12–13), 2567–2576 (2004)

    Article  MATH  Google Scholar 

  41. S. Abbasbandy, R.A.V. Gorder, M. Hajiketabi, M. Mesrizadeh, Existence and numerical simulation of periodic traveling wave solutions to the Casimir equation for the ito system. Commun. Nonlinear Sci. Numer. Simul. 27, 254–262 (2015)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  42. E.A. Coutsias, T. Hagstrom, J.S. Hesthaven, D. Torres, Integration preconditioners for differential operators in spectral \(\tau \)-method. Houston J. math. 1, 21–38 (1996)

    Google Scholar 

  43. K. Atkinson, W. Han, Theoretical Numerical Analysis, vol. 39 (Springer, Berlin, 2005), p. xviii+-576

    Book  MATH  Google Scholar 

  44. V.A. Marchenko, Sturm–Liouville Operators and Their Applications. Kiev Izdatel Naukova Dumka (1977)

  45. S.N. Singh, The determination of eign-functions of a certain Sturm–Liouville equation and its application to problems of heat-transfer. Appl. Sci. Res. 7(4), 237–250 (1958)

    Article  MathSciNet  MATH  Google Scholar 

  46. J.D. Lambert, Numerical Methods for Ordinary Differential Systems: The Initial Value Problem (Wiley, New York, 1999)

    Google Scholar 

  47. S.Y. Zhang, Z.C. Deng, Group preserving schemes for nonlinear dynamic system based on RKMK methods. Appl. Math. Comput. 175, 497–507 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  48. M.D. Buhmann, Radial basis functions. Acta Numerica 91, 1–38 (2000)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

José Francisco Gómez Aguilar acknowledges the support provided by CONACyT: cátedras CONACyT para jóvenes investigadores 2014 and SNI-CONACyT.

Funding

None.

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, University of Manitoba, Winnipeg, R3T 5V6, Canada

    Hadi Jahanshahi

  2. Department of Mathematics, University of Kurdistan, Sanandaj, Iran

    Kamal Shanazari

  3. Department of Mathematics, Imam Khomeini International University, Qazvin, Iran

    Mehdi Mesrizadeh

  4. Faculty of Industry and Mining (Khash), University of Sistan and Baluchestan, Zahedan, Iran

    Samaneh Soradi-Zeid

  5. CONACyT-Tecnológico Nacional de México/CENIDET, Interior Internado Palmira S/N, Col. Palmira, C.P. 62490, Cuernavaca, Morelos, Mexico

    J. F. Gómez-Aguilar

Authors
  1. Hadi Jahanshahi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Kamal Shanazari
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Mehdi Mesrizadeh
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Samaneh Soradi-Zeid
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. J. F. Gómez-Aguilar
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

All authors have made equal contributions to the writing of this article. The final manuscript is read and approved by all authors.

Corresponding author

Correspondence to J. F. Gómez-Aguilar.

Ethics declarations

Conflict of interest

The authors state that there is no conflict of interest in the publication of this article.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Jahanshahi, H., Shanazari, K., Mesrizadeh, M. et al. Numerical analysis of Galerkin meshless method for parabolic equations of tumor angiogenesis problem. Eur. Phys. J. Plus 135, 866 (2020). https://doi.org/10.1140/epjp/s13360-020-00716-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1140/epjp/s13360-020-00716-x

Search

Navigation