Stark-Lorentz profiles of spectral lines of atoms and polar molecules in magnetized turbulent plasmas: analytical results

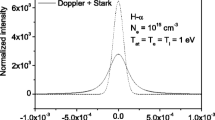
Abstract

We present analytical results for spectral line profiles of atoms and polar molecules in magnetized turbulent plasmas for the situation where the ion microfield is much smaller than both the field of the Low-frequency Electrostatic Turbulence (LET), developed along the magnetic field B, and the Lorentz field Bv/c. This situation arises, e.g., in magnetic fusion devices, solar plasmas, and white dwarfs plasmas. We obtain explicit analytical results for the line profiles in the universal form valid for any ratio p of the field of the LET and the Lorentz field and for any detuning from the unperturbed position of the spectral line. We find that the dependence of the line intensity on the ratio p at a fixed detuning is non-monotonic: as this ratio increases, the line intensity first increases, then reaches a maximum, and then decreases. For hydrogenic atoms or ions, we also provide the corresponding theoretical Stark-Lorentz profiles for the Lyman-beta, Lyman-delta, and Balmer-beta lines. From an experimental profile of the spectral line of an atom, an ion, or a polar molecule, the employment of our analytical results for the line profiles allows determining both the root-mean-square field $F_{0}$ of the LET and the product ${\textit{BT}}^{1/2}$, where B is the magnetic field and T is the temperature.

Graphic Abstract

Appendix A. Validity conditions

In plasmas where there is a large number of ions $\nu$ in the sphere of the Debye radius

$$\begin{aligned} \nu = [{\hbox {T}}_{\mathrm {e}}^{\mathrm {3}}/(36\pi {\hbox {N}}_{\mathrm {e}} {\hbox {e}}^{\mathrm {6}})]^{1/2} = 1377 [{\hbox {T}}_{\mathrm {e}}^{\mathrm {3}}/{\hbox {N}}_{\mathrm {e}} {\hbox {e}}^{\mathrm {6}}]^{1/2}> > 1,\qquad \end{aligned}$$

(A.1)

the most probable ion field is

$$\begin{aligned} {\hbox {E}}_{\mathrm {i}} = 1.608 {\hbox {E}}_{0}, \quad {\hbox {E}}_{0} = 2.603 {\hbox {eN}}_{\mathrm {e}}^{2/3} = 1.25\hbox {x}10^{-9} {\hbox {N}}_{\mathrm {e}}^{2/3}.\nonumber \\ \end{aligned}$$

(A.2)

In Eqs. (A.1) and (A.2), ${\hbox {N}}_{\mathrm {e}}$ is the electron density in ${\hbox {cm}}^{-3}$and ${\hbox {T}}_{\mathrm {e}}$ is the electron temperature in Kelvin.

The root-mean-square Lorentz field for thermal velocities

$$\begin{aligned} {\hbox {Bv}}_{0}/\hbox {c} = 4.28\hbox {x}10^{-3} \hbox {B(Tesla)}[\hbox {T}(\hbox {K})]^{1/2} \end{aligned}$$

(A.3)

is greater than the most probable ion microfield ${\hbox {E}}_{\mathrm {i}}$ when the magnetic field B is greater than the following critical value:

$$\begin{aligned} {\hbox {B}}_{\mathrm {c}} \hbox {(Tesla)} = 4.69\hbox {x}10^{-7} {\hbox {N}}_{\mathrm {e}}^{2/3}/ [\hbox {T(K)}]^{1/2}. \end{aligned}$$

(A.4)

The condition $\hbox {B} > {\hbox {B}}_{\mathrm {c}}$ can be satisfied, for example, for the edge plasmas of tokamaks. For example, for a low-density discharge in Alcator C-Mod [33], where there was ${\hbox {N}}_{\mathrm {e}} \sim 3\hbox {x}10^{13} \, {\hbox {cm}}^{-3}$ and $\hbox {T} \sim 5\hbox {x}10^{4}$ K, we get ${\hbox {B}}_{\mathrm {c}} = 4 \, \hbox {T}$, while the actual magnetic field was 8 T.

Another example: the chromosphere of the quiet Sun where the typical plasma parameters are ${\hbox {N}}_{\mathrm {e}} \sim 10^{11} \, {\hbox {cm}}^{-3}$ and $\hbox {T} \sim 10^{4} \, \hbox {K}$ – see, e.g., papers [34, 35]. In this case from Eq. (A.4) we get ${\hbox {B}}_{\mathrm {c}} = 0.1 \, \hbox {T}$. A more accurate estimate for this example can be obtained by taking into account that non-thermal velocities in the solar chromosphere can be several times higher than the thermal velocities. This lowers ${\hbox {B}}_{\mathrm {c}}$ to the value $\sim 0.03$ Tesla, while B can reach 0.4 T in sunspots.

Yet another example: atmospheres of DA white dwarfs, where the typical plasma parameters are ${\hbox {N}}_{\mathrm {e}} \sim 10^{17} \, {\hbox {cm}}^{-3}$ and $\hbox {T} \sim 10^{4} \, \hbox {K}$. In this case from Eq. (A.4) we get ${\hbox {B}}_{\mathrm {c}} \sim 10^{3} \, \hbox {T}$, while B can reach $\sim 10^{5}$ Tesla – see, e.g., papers [36, 37].

As for the LET, its energy density ${\text {F}}_{0}^{2}/(16 \pi {\hbox {N}}_{\mathrm {e}} {\hbox {T}}_{\mathrm {e}})$ can reach values $\sim 0.1$, so that the ratio ${\text {F}}_{0}/{\hbox {E}}_{\mathrm {i}}$ can be as high as

$$\begin{aligned} {\text {F}}_{0}/{\hbox {E}}_{\mathrm {i}} \sim \nu ^{1/3}> > 1, \end{aligned}$$

(A.5)

For example, such a strong field of the LET had been detected (by using a spectroscopic diagnostic) in tokamak T-10 [25].

Finally, let us bring up the following point. In the situations, where at the absence of the LET, the ion broadening of hydrogen lines was in the “impact” (more rigorously, fully dynamic) regime, the presence of a relatively strong LET can change the ion broadening to the quasistatic regime (i.e, to the regime where the overwhelming majority of ions can be considered quasistatic) under the following condition

$$\begin{aligned} {\text {v}}_{0}/{\text {r}}_{\mathrm {Ni}} < [3\hbar /(2{\hbox {m}}_{\mathrm {e}}\hbox {e})] {\hbox {nF}}_{0}, \end{aligned}$$

(A.6)

where

$$\begin{aligned} {\text {r}}_{\mathrm {Ni}} = [3/(4\pi {\hbox {N}}_{\mathrm {i}})]^{1/3} \end{aligned}$$

(A.7)

is the mean interionic distance. The left side of the inequality (A.6) is the characteristic frequency of the variation of the ion microfield, while the right side is the separation between Stark sublevels of hydrogen atoms of the principal quantum number n – the separation caused by the root-mean square field of the LET ${\text {F}}_{0}$. Physically, under the inequality (A.6), the ion microfield cannot cause virtual transitions between the Stark sublevels (leading to the “impact” broadening), so that the ion microfield becomes quasistatic.

The inequality (A.6) can be rewritten as

$$\begin{aligned} {\text {F}}_{0} > {\text {F}}_{\mathrm {crit}}, \end{aligned}$$

(A.8)

where the critical value ${\text {F}}_{\mathrm {crit}}$ of the LET is as follows:

$$\begin{aligned} {\text {F}}_{\mathrm {crit}} = [2{\hbox {m}}_{\mathrm {e}} \hbox {e}/(3\hbar \hbox {n})]({\hbox {T/M}}_{\mathrm {r}})^{1/2}(4\pi {\hbox {N}}_{\mathrm {i}}/3)^{1/3}. \end{aligned}$$

(A.9)

Here ${\hbox {M}}_{\mathrm {r}}$ is the reduced mass of the ion-radiator pair. For hydrogen plasmas, where ${\hbox {M}}_{\mathrm {r}} = {\hbox {M}}_{\mathrm {p}}/2$ (${\hbox {M}}_{\mathrm {p}}$ being the proton mass) and ${\hbox {N}}_{\mathrm {i}} = {\hbox {N}}_{\mathrm {e}}$, Eq. (A.9) can be represented in the following practical form:

$$\begin{aligned} {\text {F}}_{\mathrm {crit}}(\hbox {V/cm}) = 0.115[\hbox {T(eV)}]^{1/2} [{\hbox {N}}_{\mathrm {e}} ({\hbox {cm}}^{-3})]^{1/3}/\hbox {n}. \end{aligned}$$

(A.10)

Let us consider the following numerical examples. For edge plasmas of tokamaks, for ${\hbox {N}}_{\mathrm {e}} = 10^{\mathrm {14}} \, {\hbox {cm}}^{-3}$ and $\hbox {T} = 2 \, \hbox {eV}$, Eq. (A.10) yields ${\text {F}}_{\mathrm {crit}} = 1.5 \, \hbox {kV/cm}$ for the Lyman-delta line ($\hbox {n} = 5$), or ${\text {F}}_{\mathrm {crit}} = 2.5 \, \hbox {kV/cm}$ for the Lyman-beta line ($\hbox {n} = 3$), or ${\text {F}}_{\mathrm {crit}} =$ 1.9 kV/cm for the Balmer-beta line ($\hbox {n} = 4$). The field ${\text {F}}_{0}$ of the LET can significantly exceed ${\text {F}}_{\mathrm {crit}}$ (e.g, ${\text {F}}_{0} \sim 20 \, \hbox {kV/cm}$ was measured spectroscopically in tokamak T-10 [25]) and thus switch the ion broadening regime from “impact” to quasistatic – even for the spectral lines originating from levels of relatively low principal quantum number n (for sufficiently high excited lines, where $\text {n}> > 1$, the ion broadening is quasistatic even at the absence of the LET).

For solar flares at ${\hbox {N}}_{\mathrm {e}} = 10^{13} \, {\hbox {cm}}^{-3}$ and $\hbox {T} = 1 \, \hbox {eV}$, Eq. (A.10) yields ${\text {F}}_{\mathrm {crit}} = 165 \, \hbox {V/cm}$ for the Balmer line ${\hbox {H}}_{15}$ observed even in the most powerful solar flares (Balmer lines of $\hbox {n} > 15$ are observed in less powerful solar flares). The field ${\text {F}}_{0}$ of the LET can significantly exceed ${\text {F}}_{\mathrm {crit}}$: e.g, ${\text {F}}_{0} \sim (1 - 1.5) \, \hbox {kV/cm}$ was measured spectroscopically in solar flares (see, e.g., papers [26, 28]).

For flare stars at ${\hbox {N}}_{\mathrm {e}} = 10^{15} \, {\hbox {cm}}^{-3}$ and $\hbox {T} = 1 \, \hbox {eV}$, Eq. (A.10) yields ${\text {F}}_{\mathrm {crit}} = 1.9 \, \hbox {kV/cm}$ for the Balmer-delta line observed even in the most powerful stellar flares (Balmer lines of up to $\hbox {n} = 11$ are observed in less powerful stellar flares). The field ${\text {F}}_{0}$ of the LET can significantly exceed ${\text {F}}_{\mathrm {crit}}$: e.g, ${\text {F}}_{0}$ up to 30 kV/cm was measured spectroscopically in stellar flares (see, e.g., paper [28]).

As for plasmas of DA white dwarfs of typical parameters ${\hbox {N}}_{\mathrm {e}} \sim 10^{17} \, {\hbox {cm}}^{-3}$ and $\hbox {T} \sim 1 \, \hbox {eV}$, the ion broadening of all Balmer lines is quasistatic – regardless of whether any LET is present.