Skip to main content
SpringerLink
Log in

Precessional switching of thin nanomagnets: analytical study

  • Published:
The European Physical Journal B - Condensed Matter and Complex Systems Aims and scope Submit manuscript

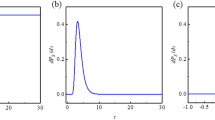
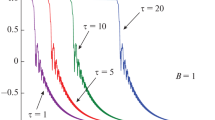
Abstract.

We study analytically the precessional switching of the magnetization of a thin macrospin. We analyze its response when subjected to an external field along its in-plane hard axis. We derive the exact trajectories of the magnetization. The switching versus non switching behavior is delimited by a bifurcation trajectory, for applied fields equal to half of the effective anisotropy field. A magnetization going through this bifurcation trajectory passes exactly along the hard axis and exhibits a vanishing characteristic frequency at that unstable point, which makes the trajectory noise sensitive. Attempting to approach the related minimal cost in applied field makes the magnetization final state unpredictable. We add finite damping in the model as a perturbative, energy dissipation factor. For a large applied field, the system switches several times back and forth. Several trajectories can be gone through before the system has dissipated enough energy to converge to one attracting equilibrium state. For some moderate fields, the system switches only once by a relaxation dominated precessional switching. We show that the associated switching field increases linearly with the damping parameter. The slope scales with the square root of the effective anisotropy. Our simple concluding expressions are useful to assess the potential application of precessional switching in magnetic random access memories.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. C.H. Back, Science 285, 864 (1999)

    Article  Google Scholar 

  2. Th. Gerrits, H.A.M. Van Den Berg, J. Hohlfeld, L. Bär, Th. Rasing, Nature 418, 6897 (2002)

    Article  Google Scholar 

  3. S. Kaka, S.E. Russek, Appl. Phys. Lett. 80, 2958 (2002)

    Article  Google Scholar 

  4. H.W. Schumacher, C. Chappert, R.C. Sousa, P.P. Freitas, J. Miltat, Phys. Rev. Lett. 90, 017204 (2003)

    Article  Google Scholar 

  5. Y. Acremann, C.H. Back, M. Buess, O. Portmann, A. Vaterlaus, D. Pescia, H. Melchior, Science 290, 492 (2000)

    Article  Google Scholar 

  6. M. Bauer, J. Fassbender, B. Hillebrands, R.L. Stamps, Phys. Rev. B 61, 3410 (2000)

    Article  Google Scholar 

  7. B.C. Choi, M. Belov, W.K. Hiebert, G.E. Ballentine, M.R. Freeman, Phys. Rev. Lett. 86, 728 (2001)

    Article  Google Scholar 

  8. R.H. Koch, Phys. Rev. Lett. 81, 4512 (1998)

    Google Scholar 

  9. W.K. Hiebert, L. Lagae, J. Das, J. Bekaert, R. Wirix-Speetjens, J. De Boeck, J. Appl. Phys. 93, 6906 (2003)

    Google Scholar 

  10. Spin Dynamics in Confined Magnetic Structures, Vols. I and II, edited by B. Hillebrands, K. Ounadjela (Springer, Berlin, 2001 and 2003)

  11. W. Wernsdorfer. Adv. Chem. Phys. 118, 99 (2003)

    Google Scholar 

  12. N.D. Rizzo, T.J. Silva, A.B. Kos, Phys. Rev. Lett. 83, 4876 (1999)

    Google Scholar 

  13. J. Miltat, G. Aburquerque, A. Thiaville in Spin Dynamics in Confined Magnetic Structures, Vol. I, edited by B. Hillebrands, K. Ounadjela (Springer, Berlin, 2001)

  14. L. He, W.D. Doyle, J. Appl. Phys. 79, 6489 (1996)

    Article  Google Scholar 

  15. Y. Acremann, C.H. Back, M. Buess, D. Pescia, V. Pobrovsky, Appl. Phys. Lett. 79, 2228 (2001)

    Google Scholar 

  16. L. Landau, E. Lifshitz, Phys. Z Sowjetunion 8, 153 (1935)

    MATH  Google Scholar 

  17. We use the convention that \(\gamma\) is positive

  18. Explicit 4th order Runge-Kutta integration of the LLG equation

  19. C. Kittel, Introduction to Solid State Physics, 5th edn. (Wiley, New York, 1976)

  20. E.C. Stoner, E.P. Wohlfarth, Philos. Trans. R. Soc. London, Ser. A 240, 599 (1948)

    MATH  Google Scholar 

  21. T. Devolder, C. Chappert, Spectral analysis of the precessional switching of the magnetization in an isotropic thin film, Sol. St. Com. (in press, 2003)

  22. Magnetization precession in confined geometry: physical and numerical aspects. Ph.D. thesis of Gonçalo M.B. Albuquerque, Orsay, July 2002. Available at http://www.lps.u-psud.fr/Collectif/gr\_25/Publications.htm

  23. The typical frequency \(\omega\) displayed in equation (14) is semi-quantitatively consistent with \(2\pi/\omega=4\tau_1+2\tau_2+4\tau_3\)

  24. In fact there is some margin: other values close to \(NT=0.5\) can result in a relaxation dominated precessional switching event. An uncertainty on this value will have the same impact that an uncertainty on the prefactor of \(\tau_3\), and will change the proportionality constant of equations (23, 24) in front of \(\alpha\). The exact required value of NT will arise from the comparison with exact computations done in Section 2.4

  25. We chose to take 1, but this factor is certainly less. In our formalism, both this term and the numerical prefactor of \(\tau_3\) end as a prefactor multiplying the damping parameter \(\alpha\) in equations (23, 24)

Download references

Author information

Authors and Affiliations

  1. Institut d’Électronique Fondamentale, UMR CNRS 8622,, Université Paris-Sud,, bâtiment 220,, 91405 , Orsay,, France

    T. Devolder & C. Chappert

Authors
  1. T. Devolder
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. C. Chappert
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to T. Devolder.

Additional information

Received: 2 October 2003, Published online: 19 November 2003

PACS:

75.40.Gb Dynamic properties (dynamic susceptibility, spin waves, spin diffusion, dynamic scaling, etc.) - 75.60.Jk Magnetization reversal mechanisms - 75.75. + a Magnetic properties of nanostructures

Rights and permissions

Reprints and permissions

About this article

Cite this article

Devolder, T., Chappert, C. Precessional switching of thin nanomagnets: analytical study. Eur. Phys. J. B 36, 57–64 (2003). https://doi.org/10.1140/epjb/e2003-00316-y

Download citation

  • Issue Date:

  • DOI: https://doi.org/10.1140/epjb/e2003-00316-y

Keywords

Search

Navigation