Absolute stability of the axially moving Kirchhoff string with a sector boundary feedback control

Abstract

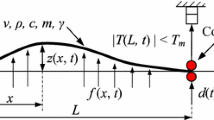
In this study, the absolute stability problem for the axially moving Kirchhoff string with nonlinear boundary feedback control has been investigated. Based on the generalized Hamilton’s variational principle, the nonlinear governing equations of the motion and boundary conditions have been deduced. The proposed boundary control, which satisfies a sector constraint condition, is a negative feedback of the transversal velocity at the right end of the string. Applying the integral-type multiplier method, the absolute stability of the axially moving Kirchhoff system is established. To validate the proposed theoretical results, numerical simulations are expressed by the finite element method.

Appendices

Appendix A. Variational analysis of motion.

Noticing that in the Hamilton principle (4), the variation variable $\delta y$ satisfy $\delta y(x,t_0)=\delta y(x,t_1)=0,$ and $\delta y(0,t)=0$, since the left eyelet $x=0$ of string is fixed. Thus, using integration by parts technique, we get from Eqs. (6) and (7),

$$\begin{aligned}&\int _{t_0}^{t_1} \delta T{\text{ d }}t\nonumber \\&\quad = \int _{t_0}^{t_1}v\rho A \left[ vy_x(l,t)+y_t(l,t)\right] \delta y(l,t){\text{ d }}t\nonumber \\&\qquad -\int _{t_0}^{t_1} \int _0^l \rho A\left[ vy_{xt}+y_{tt}\right] \delta y\nonumber \\&\qquad +v\rho A\left[ vy_{xx}+y_{tx}\right] \delta y{\text{ d }}x{\text{ d }}t\nonumber \\&\quad = \int _{t_0}^{t_1}v\rho A\left[ vy_x(l,t)+y_t(l,t)\right] \delta y(l,t){\text{ d }}t\nonumber \\&\qquad -\int _{t_0}^{t_1} \int _0^l \rho A \left[ y_{tt}+2 vy_{xt}+ v^2y_{xx}\right] \delta y{\text{ d }}x{\text{ d }}t,\nonumber \\ \end{aligned}$$

(41)

and

$$\begin{aligned}&\int _{t_0}^{t_1} \delta U{\text{ d }}t\nonumber \\&\quad = \int _{t_0}^{t_1}T_0 y_x(l,t)\delta y(l,t){\text{ d }}t-\int _{t_0}^{t_1} \int _0^l T_0 y_{xx}\delta y{\text{ d }}x{\text{ d }}t\nonumber \\&\qquad +\int _{t_0}^{t_1}\left[ \frac{EA}{2}\int _0^l y_x^2{\text{ d }}x \right] y_x(l,t) \delta y(l,t){\text{ d }}t\nonumber \\&\quad \quad -\int _{t_0}^{t_1}\int _0^l\left[ \frac{EA}{2}\int _0^l y_x^2{\text{ d }}x \right] y_{xx}\delta y{\text{ d }}x{\text{ d }}t\nonumber \\&\quad =\int _{t_0}^{t_1}\left[ T_0+\frac{EA}{2}\int _0^l y_x^2{\text{ d }}x \right] y_x(l,t) \delta y(l,t){\text{ d }}t\nonumber \\&\quad \quad -\int _{t_0}^{t_1}\int _0^l\left[ T_0+\frac{EA}{2}\int _0^l y_x^2{\text{ d }}x \right] y_{xx}\delta y{\text{ d }}x{\text{ d }}t. \end{aligned}$$

(42)

Substituting Eqs. (5), (41), and (42) into Hamilton principle (4), we get

$$\begin{aligned}&0=\int _{t_0}^{t_1} \left\langle v\rho A\left[ vy_x(l,t)+y_t(l,t)\right] +F_\mathrm{c}(t)\right. \nonumber \\&\quad \left. -\left[ T_0+\frac{EA}{2}\int _0^l y_x^2{\text{ d }}x \right] y_x(l,t)+F_\mathrm{c}(t)\right\rangle \delta y(l,t){\text{ d }}t\nonumber \\&\quad -\int _{t_0}^{t_1} \int _0^l\left\langle \rho A \left[ y_{tt}+2 vy_{xt}+ v^2y_{xx}\right] \right. \nonumber \\&\quad \left. -\left[ T_0+\frac{EA}{2}\int _0^l y_x^2{\text{ d }}x \right] y_{xx}\right\rangle \delta y{\text{ d }}x{\text{ d }}t. \end{aligned}$$

(43)

Since above equation must hold for arbitrary variation variable $\delta y$, the term in front of variables $\delta y$ and $\delta y(l,t)$ in Eq. (43) should be zero, respectively. It means that the governing Eq. (8) and the boundary condition (9) are derived.

Appendix B. Proof of Lemma 1.

Proof

To avoid repetition, we just give here the proof of Eqs. (13) and (15) in Lemma 1. And for detailed proof of Eqs. (14), (16), and (17) in Lemma 1, we refer to Lemma 3.1 of [35]. Based on the boundary condition (11c) at left eyelet $x=0,$ we easily get

$$\begin{aligned} y_t(0,t)=0,\quad \text{ for } \text{ all }\; t\ge 0. \end{aligned}$$

(44)

Then, using integration by parts and (44), we obtain for all $t\ge 0,$

$$\begin{aligned} 2\int _0^ly_{xt}(x,t)y_t(x,t){\text{ d }}x=\int _0^l[y_t^2(x,t)]_x{\text{ d }}x=y^2_t(l,t). \end{aligned}$$

That means Eq. (13) holds. For any $x\in [0,l], t\ge 0,$ we have

$$\begin{aligned} \frac{\partial }{\partial t}\big [xy_x^2(x,t)\big ]=2xy_x(x,t)y_{xt}(x,t). \end{aligned}$$

Integrating on both side of above equation with respect to $x$ over $[0,l]$, we get Eq. (15).$\square$

Appendix C. Proof of Proposition 1.

Proof

Differentiating the energy function (12) with respect to $t$, we get

$$\begin{aligned} E'(t)&= \int _0^ly_ty_{tt}{\text{ d }}x\nonumber \\&+\left[ (1-v^2)+b\int _0^ly_x^2{\text{ d }}x\right] \int _0^ly_xy_{xt}{\text{ d }}x.\nonumber \\ \end{aligned}$$

(45)

Combining boundary control (11d) with Eq. (14) in Lemma 1, we get

$$\begin{aligned}&\left[ (1-v^2)+b\int _0^ly_x^2(x,t){\text{ d }}x\right] \int _0^ly_xy_{xt}{\text{ d }}x\nonumber \\&\quad = \left[ F_\mathrm{c}(t)+vy_t(l,t)\right] y_t(l,t)\nonumber \\&\qquad -\left[ (1-v^2)+b\int _0^ly_x^2(x,t){\text{ d }}x\right] \int _0^ly_{xx}y_t{\text{ d }}x.\nonumber \\ \end{aligned}$$

(46)

Substituting Eq. (46) into Eq. (45) and observing (11a), we deduce

$$\begin{aligned} E'(t) =y_t(l,t)F_\mathrm{c}(t)+vy^2_t(l,t)-\int _0^l 2v y_{xt}y_t{\text{ d }}x, \end{aligned}$$

(47)

for all $t\ge 0.$ Finally, substituting Eq. (13) into above Eq. (47), we obtain Eq. (18).$\square$